Abstract
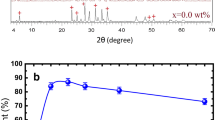
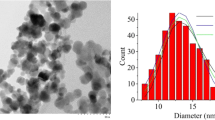
The addition of magnetic Co0.5Zn0.5Fe2O4 nanoparticles to the superconducting Cu0.5Tl0.5-1223 phase has been used to investigate the electrical resistivity behavior of the composite above the superconducting transition temperature T c. This was studied according to the opening of spin gap and fluctuation conductivity. The results indicated that the pseudogap temperature (T*) and superconducting fluctuation temperature (T scf) change by increasing the addition of Co0.5Zn0.5Fe2O4 nanoparticles. It was found that T* is related to hole carrier concentration P and it also depends on the antiferromagnetic fluctuation affected by magnetic nanoparticles. The excess-conductivity analysis showed four different fluctuation regions started from high temperature up to T c, and they were denoted by short wave (sw), two-dimensional (2D), three-dimensional (3D), and critical (cr) fluctuations. The crossover temperature between 3D and 2D (T 3D–2D) in the mean field region was decreased by increasing the addition of Co0.5Zn0.5Fe2O4 nanoparticles, in accordance with the decrease in T scf with x. The coherence length at 0 K along c-axis ξ c(0), effective layer thickness of the 2D system d, and inter-layer coupling strength J were estimated as a function of Co0.5Zn0.5Fe2O4 nanoparticle addition. Moreover, the thermodynamics, lower and upper critical magnetic fields, as well as critical current density have been calculated from the Ginzburg number N G. It was found that the low concentration of Co0.5Zn0.5Fe2O4 nanoparticles up to x = 0.08 wt% improves the superconducting parameters of Cu0.5Tl0.5-1223 phase. On the contrary, these parameters were deteriorated for (Co0.5Zn0.5Fe2O4) x /Cu0.5Tl0.5-1223 composite with x > 0.08 wt%.
Article PDF
Similar content being viewed by others
Avoid common mistakes on your manuscript.
References
Tokiwa K, Tanaka Y, Iyo A, et al. High pressure synthesis and characterization of single crystals of CuBa2Ca3Cu4Oy superconductor. Physica C 1998, 298: 209–216.
Takeuchi T, Iijima Y, Inoue K, et al. Effect of flat-roll forming on critical current density characteristics and microstructure of Nb3Al multifilamentary conductors. IEEE T Appl Supercon 1997, 7: 1529–1532.
Ihara H. New low anisotropic high-T c superconductors (Cu, Ag)Ba2Can−1CunO2n+4−y . In Advances in Superconductivity VII. Yamafuji K, Morishita T, Eds. Springer Japan, 1995: 255–260.
Ihara H, Sekita Y, Tateai H, et al. Superconducting properties of Cu1−x Tlx-1223 [Cu1−x Tlx(Ba,Sr)2Ca2Cu3O10–y] thin films. IEEE T Appl Supercon 1999, 9: 1551–1554.
Khan NA, Sekita Y, Tateai F, et al. Preparation of biaxially oriented TlCu-1234 thin films. Physica C 1999, 320: 39–44.
Aslamazove LG, Larkin AI. The influence of fluctuation pairing of electrons on the conductivity of normal metal. Phys Lett A 1968, 26: 238–239.
Thompson RS. Microwave, flux flow, and fluctuation resistance of dirty type-II superconductors. Phys Rev B 1970, 1: 327.
Lawrence WE, Doniach S. Theory of layer structure superconductor. In Proceedings of the 12th International Conference on Low-Temperature Physics. Kanda E, Ed. Keigaku, Tokyo, 1971: 361.
Hikami S, Larkin AI. Magnetoresistance of high temperature superconductors. Mod Phys Lett B 1988, 2: 693.
Qasim I, Waqee-ur-Rehman M, Mumtaz M, et al. Role of anti-ferromagnetic Cr nanoparticles in CuTl-1223 superconducting matrix. J Alloys Compd 2015, 649: 320–326.
Nadeem K, Hussain G, Mumtaz M, et al. Role of magnetic NiFe2O4 nanoparticles in CuTl-1223 superconductor. Ceram Int 2015, 41: 15041–15047.
Hussain G, Jabbar A, Qasim I, et al. Activation energy and excess conductivity analysis of (Ag)x/CuTl-1223 nano-superconductor composites. J Appl Phys 2014, 116: 103911.
Naqib SH. Effects of Zn on superconductivity, stripe order, and pseudogap correlations in YBa2(Cu1−y Zny)3O7−δ . Physica C 2012, 476: 10–14.
Emery VJ, Kivelson SA. Importance of phase fluctuations in superconductors with small superfluid density. Nature 1994, 374: 434–437.
Chen Q, Kosztin I, Jankó B, et al. Pairing fluctuation theory of superconducting properties in underdoped to overdoped cuprates. Phys Rev Lett 1998, 81: 4708.
Chubukov AV, Schmalian J. Temperature variation of the pseudogap in underdoped cuprates. Phys Rev B 1998, 57: R11085.
Anderson PW. The ‘spin gap’ in cuprate superconductors. J Phys: Condens Matter 1996, 8: 10083.
Lee PA. Pseudogaps in underdoped cuprates. Physica C 1999, 317–318: 194–204.
Tallon JL, Loram JW. The doping dependence of T*—what is the real high-T c phase diagram? Physica C 2001, 349: 53–68.
Khurram AA, Khan NA, Mumtaz M. Intercomparison of fluctuation induced conductivity of Cu0.5Tl0.5Ba2Ca n−1CunO2n+4−y (n = 2, 3, 4) superconductor thin films. Physica C 2009, 469: 279–282.
Abou Aly AI, Ibrahim IH, Awad R, et al. Stabilization of Tl-1223 phase by arsenic substitution. J Supercond Nov Magn 2010, 23: 1325–1332.
Koo JH, Cho G. The spin-gap in high T c superconductivity. J Phys: Condens Matter 2003, 15: L729.
Abou-Aly AI, Awad R, Ibrahim IH, et al. Excess conductivity analysis for Tl0.8Hg0.2Ba2Ca2Cu3O9−δ substituted by Sm and Yb. Solid State Commun 2009, 149: 281–285.
Naqib SH, Cooper JR, Tallon JL, et al. Doping phase diagram of Y1−x CaxBa2(Cu1−y Zny)3O7−δ from transport measurements: Tracking the pseudogap below T c. Phys Rev B 2005, 71: 054502.
Mohammadizadeh MR, Akhavan M. Pseudogap in Gd-based 123 HTSC. Physica B 2003, 336: 410–419.
Anderson PW. The resonating valence bond state in La2CuO4 and superconductivity. Science 1987, 235: 1196–1198.
Lee PA, Nagaosa N, Ng T-K, et al. SU(2) formulation of the t−J model: Application to underdoped cuprates. Phys Rev B 1998, 57: 6003.
François I, Jaekel C, Kyas G, et al. Influence of Pr doping and oxygen deficiency on the scattering behavior of YBa2Cu3O7 thin films. Phys Rev B 1996, 53: 12502.
Emery VJ, Kivelson SA, Zachar O. Spin-gap proximity effect mechanism of high-temperature superconductivity. Phys Rev B 1997, 56: 6120.
Presland MR, Tallon JL, Buckley RG, et al. General trends in oxygen stoichiometry effects on T c in Bi and Tl superconductors. Physica C 1991, 176: 95–105.
Ihara H, Tanaka K, Tanaka Y, et al. Mechanism of T c enhancement in Cu1−x Tlx-1234 and -1223 system with T c > 130 K. Physica C 2000, 341–348: 487–488.
Poddar A, Bandyopadhyay B, Chattopadhyay B. Effects of Co-substitution on superconductivity and transport in Tl2Ba2Ca1−x Yx(Cu1−y Coy)2O8+δ . Physica C 2003, 390: 120–126.
Passos CAC, Passamai Jr. JL, Orlando MTD, et al. An investigation of T* behavior on (Hg,Re)-1223 system. Physica C 2007, 460–462: 1086–1087.
Hohenberg PC, Halperin BI. Theory of dynamic critical phenomena. Rev Mod Phys 1977, 49: 435.
Lobb CJ. Critical fluctuations in high-T c superconductors. Phys Rev B 1987, 36: 3930.
Rahim M, Khan NA. Suppressed phonon density and Para conductivity of Cd doped Cu0.5Tl0.5Ba2Ca3Cu4−y CdyO12−δ (y = 0, 0.25, 0.5, 0.75) superconductors. J Alloys Compd 2012, 513: 55–60.
Bardeen J, Cooper LN, Schrieffer JR. Theory of superconductivity. Phys Rev 1957, 108: 1175.
Khurram AA, Khan NA. A search for a low anisotropic superconductor. J Electromagnetic Analysis & Applications 2010, 2: 63–74.
Geru II, Ghilan ZI, Dihor IT, et al. Moldavian Journal of the Physical Sciences 2002, N2: 53.
Snezhko A, Prozorov T, Prozorov R. Magnetic nanoparticles as efficient bulk pinning centers in type-II superconductors. Phys Rev B 2005, 71: 024527.
Abou-Aly AI, Awad R, Kamal M, et al. Excess conductivity analysis of (Cu0.5Tl0.5)-1223 substituted by Pr and La. J Low Temp Phys 2011, 163: 184–202.
Author information
Authors and Affiliations
Corresponding author
Additional information
This article is published with open access at Springerlink.com
Open Access The articles published in this journal are distributed under the terms of the Creative Commons Attribution 4.0 International License (http://creativecommons.org/licenses/by/4.0/), which permits unrestricted use, distribution, and reproduction in any medium, provided you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made.
Rights and permissions
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons licence, and indicate if changes were made.
The images or other third party material in this article are included in the article’s Creative Commons licence, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons licence and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder.
To view a copy of this licence, visit https://creativecommons.org/licenses/by/4.0/.
About this article
Cite this article
Barakat, M.M., Al-Sayyed, N., Awad, R. et al. Superconducting parameter determination for (Co0.5Zn0.5Fe2O4) x /Cu0.5Tl0.5-1223 composite. J Adv Ceram 5, 210–218 (2016). https://doi.org/10.1007/s40145-016-0192-2
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s40145-016-0192-2