Abstract
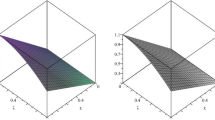
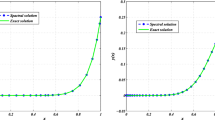
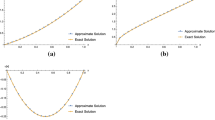
Here, we shed light on the fractional linear and nonlinear Klein–Gorden partial differential equations via Fractional Shifted Legendre Tau Method. With this objective, the operational matrices of fractional-order shifted Legendre functions (FSLFs) are derived and combined with the Tau method to convert the fractional-order differential equations to a system of solvable algebraic equations. The validity and the efficiency of the operational matrices are tested. Our findings yield an affirmative consequence, indicating applicability of the proposed method for nonlinear equations appearing in science and engineering.

Similar content being viewed by others
References
Mainardi, F.: Fractional Calculus and Waves in Linear Viscoelasticity: An Introduction to Mathematical Models. Imperial College Press, New York (2010)
Lu, D., Liang, J., Du, X., Ma, C., Gao, Z.: Fractional elastoplastic constitutive model for soils based on a novel 3d fractional plastic flow rule. Comput. Geotech. 105, 277–290 (2019)
Li, C., Guo, H., Tian, X., He, T.: Generalized thermoelastic diffusion problems with fractional order strain. Eur. J. Mech. -A/Solids 78, 103827 (2019)
Miles, P.R., Pash, G.T., Smith, R.C., Oates, W.S.: Global sensitivity analysis of fractional-order viscoelasticity models. In: Behavior and Mechanics of Multifunctional Materials XIII, Vol. 10968, p. 1096806. International Society for Optics and Photonics (2019)
Roy, R., Akbar, M.A., Wazwaz, A.M.: Exact wave solutions for the nonlinear time fractional Sharma-Tasso-olver equation and the fractional Klein-Gordon equation in mathematical physics. Opt. Quant. Electron. 50(1), 25 (2018)
Hosseini, V.R., Shivanian, E., Chen, W.: Local integration of 2-d fractional telegraph equation via local radial point interpolant approximation. Eur. Phys. J. Plus 130(2), 33 (2015)
Aslefallah, M., Shivanian, E.: Nonlinear fractional integro-differential reaction-diffusion equation via radial basis functions. Eur. Phys. J. Plus 130(47), 1–9 (2015)
Hosseini, V.R., Shivanian, E., Chen, W.: Local radial point interpolation (mlrpi) method for solving time fractional diffusion-wave equation with damping. J. Comput. Phys. 312, 307–332 (2016)
Shivanian, E.: Spectral meshless radial point interpolation (SMRPI) method to two-dimensional fractional telegraph equation. Math. Methods Appl. Sci. 39(7), 1820–1835 (2016)
Shivanian, E.: Analysis of the time fractional 2-d diffusion-wave equation via moving least square (mls) approximation. Int. J. Appl. Comput. Math. 3(3), 2447–2466 (2017)
Shivanian, E., Jafarabadi, A.: An improved spectral meshless radial point interpolation for a class of time-dependent fractional integral equations: 2d fractional evolution equation. J. Comput. Appl. Math. 325, 18–33 (2017)
Alqahtani, R.T.: Approximate solution of non-linear fractional Klein–Gordon equation using spectral collocation method. Appl. Math. 6, 2175–2181 (2015)
Wazwaz, A.: Compacton solitons and periodic solutions for some forms of nonlinear Klein-Gordon equations. Chaos Solitons Fract. 28, 1005–1013 (2006)
Elgarayhi, A.: New periodic wave solutions for the shallow water equations and the generalized Klein-Gordon equation. Commun. Nonlinear Sci. Numer. Simul. 13, 877–888 (2008)
Golmankhaneh, A.K., Golmankhaneh, A.K., Baleanu, D.: On nonlinear fractional Klein-Gordon equation. Signal Process. 91(3), 446–451 (2011)
Khader, M., Swetlam, N., Mahdy, A.: The Chebyshev collection method for solving fractional order Klein–Gordon equation. Wseas Trans. Math. 13, 31–38 (2014)
Ortiz, E., Samara, H.: An operational approach to the Tau method for the numerical solution of nonlinear differential equations. Computing 27, 15–25 (1981)
Ortiz, E., Samara, H.: Numerical solution of differential eigenvalue problems with an operational approach to the Tau method. Computing 31, 95–103 (1983)
Shaban, M., Shivanian, E., Abbasbandy, S.: Analyzing magneto-hydrodynamic squeezing flow between two parallel disks with suction or injection by a new hybrid method based on the tau method and the homotopy analysis method. Eur. Phys. J. Plus 128(11), 133 (2013)
Shaban, M., Kazem, S., Shivanian, E.: Fully discrete tau solution for some types of non-local heat transport equations. Appl. Anal. 1, 1–15 (2017)
Rida, S., Yousef, A.: On the fractional order Rodrigues formula for the Legendre polynomials. Adv. Appl. Math. Sci. 10, 509–518 (2011)
Klimek, M., Agrawal, O.P.: Fractional sturm-liouville problem. Comput. Math. Appl. 66(5), 795–812 (2013)
Kazem, S., Abbasbandy, S., Kumar, S.: Fractional-order Legendre functions for solving fractional-order differential equations. Appl. Math. Model. 37, 5498–5510 (2013)
Mokhtary, P.: Operational Tau method for nonlinear multi-order FDEs. Iranian J. Numer. Anal. Optim. 4, 43–55 (2014)
Kazem, S., Shaban, M., Rad, J.A.: Solution of Coupled Burger’s equation based on operational matrices of d–dimensional orthogonal functions. Z. Naturforsch 67(a), 267–274 (2007)
Wang, G., Hashemi, M.: Lie symmetry analysis and soliton solutions of time-fractional k(m, n) equation. Pramana 88(1), 7 (2017)
Hashemi, M., Baleanu, D.: Lie symmetry analysis and exact solutions of the time fractional gas dynamics equation 18, 3–4 (2016)
Kheybari, S., Darvishi, M.T., Hashemi, M.S.: Numerical simulation for the space-fractional diffusion equations. Appl. Math. Comput. 348, 57–69 (2019)
Hashemi, M.S., Inc, M., Yusuf, A.: On three-dimensional variable order time fractional chaotic system with nonsingular kernel. Chaos Solitons Fract 133, 109628 (2020)
Razzaghi, M., Oppenheimer, S., Ahmad, F.: Tau method approximation for radiative transfer problems in a slab medium. JQSRT 72, 439–447 (2002)
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
About this article
Cite this article
Tameh, M.S., Shivanian, E. Fractional shifted legendre tau method to solve linear and nonlinear variable-order fractional partial differential equations. Math Sci 15, 11–19 (2021). https://doi.org/10.1007/s40096-020-00351-8
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s40096-020-00351-8