Abstract
The generalized p-median problem is related with the task to find an optimal distribution of p facilities for given heterogeneous population distribution. In the real world, the meaning of what is optimal strongly depends on the type and the purpose of the facility: the optimal distribution of fire stations may not be the same as the ones for hospitals, coffee shops, and schools. We start from the variational approach in Gastner and Newman (Phys Rev E 74:016117, 2006) and generalize the objective function in such a way that the accessibility of a facility is an algebraic function of distance with an exponent \(\beta\). Applying the second-order variational approach, we find that the extremum of the objective function corresponds to either a minimum or a maximum, depending on the value of the exponent \(\beta\). We find that the optimal distribution of public (private) facilities minimizes (maximizes) the objective function. We also perform simulations for the real population distribution of South Korea to validate our results from the variational approach.




Similar content being viewed by others
References
S.M. Gusein-Zade, Geogr. Anal. 14, 246 (1982)
N. Megiddo, K.J. Supowit, SIAM J. Comput. 13, 182 (1984)
J. Mycielski, W. Trzeciakowski, J. Reg. Sci. 5, 59 (1963)
M.T. Gastner, Phys. Rev. E 84, 036112 (2011)
M.J. Lee, K. Kim, J. Son, D.-S. Lee, Sci. Rep. 10, 1 (2020)
A. Murat, V. Verter, G. Laporte, Comput. Oper. Res. 37, 123 (2010)
L. Cooper, Oper. Res. 11, 331 (1963)
S.L. Hakimi, Oper. Res. 13, 462 (1965)
C.S. ReVelle, R.W. Swain, Geogr. Anal. 2, 30 (1970)
G. Laporte, S. Nickel, F.S. da Gama, Location Science (Springer, 2015)
K.E. Rosing, Eur. J. Oper. Res. 58, 414 (1992)
E. Carrizosa, E. Conde, M. Munoz-Marquez, J. Puerto, RAIRO-Oper. Res. 29, 35 (1995)
Z. Drezner, H.W. Hamacher, Facility Location: Applications and Theory (Springer, 2002)
M.T. Gastner, M.E. Newman, Phys. Rev. E 74, 016117 (2006)
J. Um, S.-W. Son, S.-I. Lee, H. Jeong, B.J. Kim, Proc. Natl. Acad. Sci. USA. 106, 14236 (2009)
D. Kim, S.-W. Son, H. Jeong, J. Korean Phys. Soc. 60, 585 (2012)
O. Kwon, Physica A 503, 209 (2018)
A. Gomez-Lievano, H. Youn, L.M. Bettencourt, PLoS One 7, e40393 (2012)
V.C. Yang, A.V. Papachristos, D.M. Abrams, Phys. Rev. E 100, 032306 (2019)
A. Okabe, A. Suzuki, Eur. J. Oper. Res. 98, 445 (1997)
J.-H. Qian, C.-H. Yang, D.-D. Han, Y.-G. Ma, Physica A 391, 5146 (2012)
J.C. Leitao, J.M. Miotto, M. Gerlach, E.G. Altmann, R. Soc. Open Sci. 3, 150649 (2016)
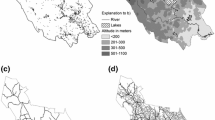
Population data are downloaded from Korean Statistical Information Service (https://kosis.kr), and the areas of counties from Ministry of Land, Infrastructure, and Transport (http://stat.molit.go.kr). We also use the boundary map data for each county downloaded from Statistical Geographic Information Service of Korea (https://sgis.kostat.go.kr)
Acknowledgements
This research was supported by a National Research Foundation of Korea (NRF) grant funded by the Korea government’s Ministry of Science and ICT (MSIT) Grant No. 2019R1A2C2089463.
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher’s Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
About this article
Cite this article
Park, J.I., Kim, B.J. Generalized p-median problem for the optimal distribution of facilities. J. Korean Phys. Soc. 80, 352–358 (2022). https://doi.org/10.1007/s40042-021-00361-2
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s40042-021-00361-2