Ferroquadrupolar Order in the Spin-1 Bilinear-Biquadratic Model up to the Second Nearest Neighbor

Abstract

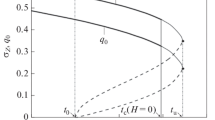
We have studied some ferroquadrupolar phases of the S = 1 Heisenberg model with bilinear and biquadratic exchange interactions on the square lattice up to the second nearest neighbor, using the SU(3) Schwinger bosons formalism in a mean field approximation. This technique is very convenient to treat nematic order. This technique has the advantage of using the fundamental representation of the SU(N) group instead of SU(2), designed to capture spin-quadrupolar order in addition to the dipolar magnetic order. We also present quadrupole structure factors that can be measured in future experiments. Our calculations can have implications in the study of iron-based superconductors.

Appendix A

Let us start with the calculation of the spin-spin correlation function. Using <t _z  > = t in Eq. (9) and Fourier transforming, we have

$$ \begin{array}{cc}\hfill {S}_q^{+}=\sqrt{2} t\left({d}_d+{u}_{- q}^{+}\right),\hfill & \hfill {S}_q^{-}=\sqrt{2} t\left({d}_q^{+}+{u}_{- q}\right)\hfill \end{array} $$

(A1)

Then we can write

$$ <{S}_q^{+}{S}_{- q}^{-}>=2{t}^2\left(<{d}_q{d}_d^{+}>+<{d}_q{u}_{- q}>+<{u}_{- q}^{+}{d}_q^{+}>+<{u}_{- q}^{+}{u}_{- q}>\right) $$

(A2)

Taking Eq. (14) into Eq. (A2), we obtain

$$ <{S}_q^{+}{S}_{- q}^{-}>=2{t}^2\left(<{\beta}_q{\beta}_q^{+}>+<{\alpha}_q^{+}{\alpha}_q>\right)\left({\chi}_q^2-2{\chi}_q{\rho}_q+{\rho}_q^2\right) $$

(A3)

Using the well know expression

$$ <{\alpha}_q^{+}{\alpha}_q>=<{\beta}_q^{+}{\beta}_q>={n}_q, $$

(A4)

leads to

$$ <{S}_q^{+}{S}_{- q}^{-}>=2{t}^2\left(1+2{n}_q\right)\left({\chi}_q^2-2{\chi}_q{\rho}_q+{\rho}_q^2\right). $$

(A5)

Finally, inserting Eq. (15) into Eq. (A5), we get Eq. (27).

Now, we turn to the calculation of the zz quadrupole structure factor. Equations (30) and (9) leads to

$$ \begin{array}{l}<{\left({S}_q^z\right)}^2{\left({S}_{- q}^z\right)}^2>=\sum_{k,{k}_1}\left(<{u}_{q- k}^{+}{u}_k{u}_{- q-{k}_1}^{+}{u}_{k_1}>+<{d}_{q- k}^{+}{d}_k{d}_{- q-{k}_1}^{+}{d}_{k_1}>\right.+\hfill \\ {}\left.<{u}_{q- k}^{+}{u}_k{d}_{- q-{k}_1}^{+}{d}_{k_1}>+<{d}_{q- k}^{+}{d}_k{u}_{- q-{k}_1}^{+}{u}_{k_1}>\right).\hfill \end{array} $$

(A6)

Let us consider the first term as an example. Using Eq. (14), we find

$$ <\left({\chi}_{q- k}{\alpha}_{q- k}^{+}-{\rho}_{q- k}{\beta}_{q- k}\right)\left({\chi}_k{\alpha}_k-{\rho}_k{\beta}_k^{+}\right)\left({\chi}_{q-{k}_1}{\alpha}_{q-{k}_1}^{+}-{\rho}_{q-{k}_1}{\beta}_{q-{k}_1}\right)\left({\chi}_{k_1}{\alpha}_{k_1}-{\rho}_{k_1}{\beta}_{k_1}^{+}\right)>. $$

(A7)

Using the standard procedure to decouple the four operators terms

$$ ABCD=< AB> CD+< AC> BD+< AD> BC+< BC> AD+< BD> AC+< CD> AB, $$

(A8)

and taking into account that the only non null averages are <α ⁺ α >  ,  < αα ⁺ > , <β ⁺ β > , and <ββ ⁺>, we get

$$ \begin{array}{l}\sum_k\left({\chi}_k^2\right.{\chi}_{q- k}^2<{\alpha}_{q- k}^{+}{\alpha}_{q- k}><{\alpha}_k{\alpha}_k^{+}>+{\chi}_{q- k}^2{\rho}_k^2<{\alpha}_{q- k}^{+}{\alpha}_{q- k}><{\beta}_k^{+}{\beta}_k>+\hfill \\ {}\left.{\chi}_k^2{\rho}_{q- k}^2<{\alpha}_k{\alpha}_k^{+}><{\beta}_{q- k}{\beta}_{q- k}^{+}>+{\rho}_k^2{\rho}_{q- k}^2<{\beta}_{q- k}{\beta}_{q- k}^{+}><{\beta}_k^{+}{\beta}_k>\right).\hfill \end{array} $$

(A9)

That can be written as

$$ \begin{array}{l}\sum_k\left[{\chi}_k^2\right.{\chi}_{q- k}^2{n}_{q- k}\left(1+{n}_k\right)+{\chi}_{q- k}^2{\rho}_k^2{n}_{q- k}{n}_k+\hfill \\ {}\left.{\chi}_k^2{\rho}_{q- k}^2\left(1+{n}_k\right)\left(1+{n}_{q- k}\right)+{\rho}_k^2{\rho}_{q- k}^2\left(1+{n}_{q- k}\right){n}_k\right].\hfill \end{array} $$

(A10)

On the other side, the fourth term leads to

$$ \begin{array}{l}\sum_k\left\{{\chi}_k{\chi}_{q- k}\right.{\rho}_k{\rho}_{q- k}{n}_{- q+ k}\left(1+{n}_k\right)+{\chi}_{q- k}{\chi}_k{\rho}_k{\rho}_{q- k}\left[{n}_{- q+ k}{n}_k+\left(1+{n}_{- q+ k}\right)\left(1+{n}_k\right)+\right.\\ {}\left.\left.\left(1+{n}_{- q+ k}\right){n}_k\right]\right\}.\end{array} $$

(A11)

Putting all the terms together, we find at T = 0:

$$ <{\left({S}_q^z\right)}^2{\left({S}_{- q}^z\right)}^2>=\sum_k2\left({\chi}_k^2{\rho}_{q- k}^2+{\chi}_k{\rho}_k{\chi}_{q- k}{\rho}_{q- k}\right). $$

(A12)

Eq. (15) gives

$$ {\chi}_k^2{\rho}_{q- k}^2=\frac{\left({\omega}_k+{\Lambda}_k\right)\left({\Lambda}_{q- k}-{\omega}_{q- k}\right)}{4{\omega}_k{\omega}_{q- k}} $$

(A13)

and

$$ {\chi}_k{\rho}_k{\chi}_{q- k}{\rho}_{q- k}=\frac{\sqrt{\left({\Lambda}_k^2-{\omega}_k^2\right)\left({\Lambda}_{q- k}^2-{\omega}_{q- k}^2\right)}}{4{\omega}_k{\omega}_{q- k}} $$

(A14)

Inserting (A13) and (A14) into (A12) and using ${\Lambda}_k^2-{\omega}_k^2={\Delta}_k^2$, we get Eq. (30).

Finally, we calculate the xy component of the quadrupole structure factor. We start with

$$ {Q}_n^{x y}={S}_n^x{S}_n^y+{S}_n^y{S}_n^x=-2 i\left({S}_n^{+}{S}_n^{+}-{S}_n^{-}{S}_n^{-}\right) $$

(A15)

Using Eq. (9) and taking the Fourier transform, we obtain

$$ \begin{array}{l}{Q}_q^{xy}=-4{it}^2\sum_k\left({d}_k{d}_{q- k}+{d}_k{u}_{- q+ k}^{+}+{u}_k^{+}{d}_{q+ k}+{u}_k^{+}{u}_{- q- k}^{+}\right.\hfill \\ {}\left.-{d}_k^{+}{d}_{- q- k}^{+}-{d}_k^{+}{u}_{q+ k}-{u}_k{d}_{- q+ k}^{+}-{u}_k{u}_{q- k}\right)\hfill \end{array} $$

(A16)

We follow the same procedure as we did for the zz component. The calculation is lengthy, but straightforward.