Skip to main content
SpringerLink
Log in

Uniformizations of stable \((\gamma ,n)\)-gonal Riemann surfaces

  • Published:
Analysis and Mathematical Physics Aims and scope Submit manuscript

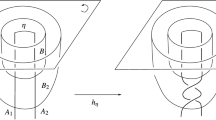
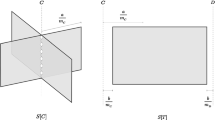
Abstract

A \((\gamma ,n)\)-gonal pair is a pair (S, f), where S is a closed Riemann surface and \(f:S \rightarrow R\) is a degree n holomorphic map onto a closed Riemann surface R of genus \(\gamma \). If the signature of (S, f) is of hyperbolic type, then it admits a uniformizing pair \((\varGamma ,G)\), where G is a Fuchsian group acting on the unit disc \({{\mathbb {D}}}\) containing \(\varGamma \) as an index n subgroup, such that f is induced by the inclusion \(\varGamma \le G\). The uniformizing pair is uniquely determined by (S, f), up to conjugation by holomorphic automorphisms of \({{\mathbb {D}}}\), and it permits to provide a natural complex orbifold structure on the Hurwitz space parametrizing (twisted) isomorphic classes of pairs topologically equivalent to (S, f). In order to produce certain compactifications of these Hurwitz spaces, one needs to consider the so called stable \((\gamma ,n)\)-gonal pairs, which are natural geometrical deformations of \((\gamma ,n)\)-gonal pairs. Due to the above, it seems interesting to search for uniformizations of stable \((\gamma ,n)\)-gonal pairs, in terms of certain class of Kleinian groups. In this paper we review such uniformizations by using noded Fuchsian groups, obtained from the noded Beltrami differentials of Fuchsian groups that were previously studied by Alexander Vasil’ev and the author, and which provide uniformizations of stable Riemann orbifolds. These uniformizations permit to obtain a compactification of the Hurwitz spaces together a complex orbifold structure, these being quotients of the augmented Teichmüller space of G by a suitable finite index subgroup of its modular group.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Abikoff, W.: On boundaries of Teichmüller spaces and on Kleinian groups, III. Acta Math. 134, 211–237 (1975)

    Article  MathSciNet  Google Scholar 

  2. Abikoff, W.: Degenerating families of Riemann surfaces. Ann. Math. (2) 105, 29–44 (1977)

    Article  MathSciNet  Google Scholar 

  3. Abikoff, W.: Augmented Teichmüller spaces. Bull. Am. Math. Soc. 82(2), 333–334 (1976)

    Article  MathSciNet  Google Scholar 

  4. Ahlfors, L.V.: Finitely generated Kleinian groups. Am. J. Math. 86, 413–429 (1964). correction ibid 87 (1965), 759

    Article  MathSciNet  Google Scholar 

  5. Ahlfors, L.V.: Some remarks on Teichmüller’s space of Riemann surfaces. Ann. Math. (2) 74, 171–191 (1961)

    Article  MathSciNet  Google Scholar 

  6. Ahlfors, L., Bers, L.: Riemann’s mapping theorem for variable metrics. Ann. Math. 72, 385–404 (1960)

    Article  MathSciNet  Google Scholar 

  7. Arfeux, M.: Berkovich spaces and Deligne–Mumford compactification. arXiv:1506.02552

  8. Bartolini, G., Costa, A.F., Izquierdo, M.: On automorphisms groups of cyclic \(p\)-gonal Riemann surfaces. J. Symb. Comput. 57, 61–69 (2013)

    Article  MathSciNet  Google Scholar 

  9. Beardon, A.E.: The Geometry of Discrete Groups. Graduate Texts in Mathematics, vol. 91. Springer, New York (1983)

    MATH  Google Scholar 

  10. Belyi, G.V.: On Galois extensions of a maximal cyclotomic field. Math. USSR Izv. 14, 247–265 (1980)

    Article  Google Scholar 

  11. Bers, L.: Simultaneous uniformization. Bull. Am. Math. Soc. 66(2), 94–97 (1960)

    Article  MathSciNet  Google Scholar 

  12. Bers, L.: Spaces of Kleinian groups. In: Several Complex Variables, Maryland. Lecture Notes in Mathematics, vol 155, pp. 9–34. Springer, Berlin (1970)

    Chapter  Google Scholar 

  13. Bers, L.: On boundaries of Teichmüller spaces and on Kleinian groups. I. Ann. Math. Second Ser. 91, 570–600 (1970)

    Article  Google Scholar 

  14. Bers, L.: On spaces of Riemann surfaces with nodes. Bull. Am. Math. Soc. 80, 1219–1222 (1974)

    Article  MathSciNet  Google Scholar 

  15. Bujalance, E., Costa, A.F.: Automorphism groups of cyclic \(p\)-gonal pseudo-real Riemann surfaces. J. Algebra 440, 531–544 (2015)

    Article  MathSciNet  Google Scholar 

  16. Clebsch, A.: Zur Theorie der Riemann’schen Fläche. Math. Ann. 6(2), 216–230 (1873)

    Article  MathSciNet  Google Scholar 

  17. Coelho, J., Sercio, F.: On the gonality of stable curves. arXiv:1507.07494v2.pdf

  18. Costa, A.F.: One example of \(1\)-dimensional Hurwitz space. In: López, J.L., Coronado, L.M.P. (eds.) Mathematical Contributions: Volume in Honor of Professor Joaquín Arregui Fernández (Spanish), pp. 127–134. Homenajes de la Universidad Complutense, Editorial Complutense, Madrid (2000)

  19. Costa, A.F., González-Aguilera, V.: Limits of equisymmetric 1-complex dimensional families of Riemann surfaces. Math. Scand. 121(1), 26–48 (2017)

    Article  MathSciNet  Google Scholar 

  20. Costa, A.F., Izquierdo, M., Parlier, H.: Connecting p-gonal loci in the compactification of moduli space. Rev. Mat. Complut. 28(2), 469–486 (2015)

    Article  MathSciNet  Google Scholar 

  21. Costa, A.F., Izquierdo, M., Riera, G.: One-dimensional Hurwitz spaces, modular curves, and real forms of Belyi meromorphic functions. Int. J. Math. Math. Sci. 2008, 1–18 (2008)

    Article  MathSciNet  Google Scholar 

  22. Deligne, P., Mumford, D.: The irreducibility of the space of curves of given genus. Inst. Hautes Études Sci. Publ. Math. 36, 75–109 (1969)

    Article  MathSciNet  Google Scholar 

  23. Diaz, R., González-Aguilera, V.: Limit points of branch locus of \({\rm M}_{g}\). Adv. Geom. (to appear)

  24. Earle, C.J.: Teichmüller spaces and complex manifolds. Warwick 1992. Teichmüller Theory Moduli Spaces 10, 5–33 (2010)

    MATH  Google Scholar 

  25. Eisenbud, D., Elkies, N., Harris, J., Speiser, R.: On the Hurwitz scheme and its monodromy. Compos. Math. 77(1), 95–117 (1981)

    MathSciNet  MATH  Google Scholar 

  26. Fried, M.: Fields of definition of function fields and Hurwitz families—groups as Galois groups. Commun. Algebra 5(1), 17–82 (1977)

    Article  MathSciNet  Google Scholar 

  27. Gromadzki, G., Weaver, A., Wootton, A.: On gonality of Riemann surfaces. Geom. Dedicata 149, 1–14 (2010)

    Article  MathSciNet  Google Scholar 

  28. Harris, J., Mumford, D.: On the Kodaira dimension of the moduli space of curves. Invent. Math. 67, 23–86 (1982)

    Article  MathSciNet  Google Scholar 

  29. Hidalgo, R.A.: The noded Schottky Space. Proc. Lond. Math. Soc. 73, 385–403 (1996)

    Article  MathSciNet  Google Scholar 

  30. Hidalgo, R.A.: Noded Fuchsian groups I. Complex Var. Theory Appl. 36, 45–66 (1998)

    MathSciNet  MATH  Google Scholar 

  31. Hidalgo, R.A., Vasil’ev, A.: Noded Teichmüller spaces. J. Anal. Math. 99(1), 89–107 (2006)

    Article  MathSciNet  Google Scholar 

  32. Hinich, V., Vaintrob, A.: Augmented Teichmüller spaces and orbifolds. Sel. Math. (N.S.) 16(3), 533–629 (2010)

    Article  Google Scholar 

  33. Hubbard, J.H., Koch, S.: An analytic construction of the Deligne–Mumford compactification of the moduli space of curves. J. Differ. Geom. 98, 261–313 (2014)

    Article  MathSciNet  Google Scholar 

  34. Hurwitz, A.: Über Riemann’sche Flächen, mit gegebenen Verzweigungspunkten. Math. Ann. 39(1), 1–60 (1891)

    Article  MathSciNet  Google Scholar 

  35. Keen, L., Maskit, B., Series, C.: Geometric finiteness and uniqueness for Kleinian groups with circle packing limit sets. J. Reine Angew. Math. 436, 209–219 (1993)

    MathSciNet  MATH  Google Scholar 

  36. Koebe, P.: Über die Uniformisierung der Algebraischen Kurven II. Math. Ann. 69, 1–81 (1910)

    Article  MathSciNet  Google Scholar 

  37. Kra, I.: On spaces of Kleinian groups. Comment. Math. Helv. 47, 53–69 (1972)

    Article  MathSciNet  Google Scholar 

  38. Kra, I., Maskit, B.: The deformation space of a Kleinian group. Am. J. Math. 103, 1065–1102 (1981)

    Article  MathSciNet  Google Scholar 

  39. Kra, I., Maskit, B.: Pinching two component Kleinian groups. In: Cazacu, C.A., Lehto, O.E., Rassias, T.M. (eds.) Analysis and Topology, pp. 425–465. World Scientific Press, London (1998)

    Chapter  Google Scholar 

  40. Letho, O.: Univalent Functions and Teichmüller Spaces. Graduate Texts in Mathematics. Springer, Berlin (1986)

    Google Scholar 

  41. Maskit, B.: Kleinian Groups. Grundlehren der Mathematischen Wissenschaften. Springer, Berlin (1988)

    MATH  Google Scholar 

  42. Maskit, B.: On boundaries of Teichmüller spaces II. Ann. Math. 91, 607–639 (1970)

    Article  MathSciNet  Google Scholar 

  43. Maskit, B.: On a class of Kleinian groups. Ann. Acad. Sci. Fenn. Ser. A I Math. 442 (1969). http://www.acadsci.fi/mathematica/1969/no442pp01-08.pdf

  44. Maskit, B.: Parabolic elements in Kleinian groups. Ann. Math. 117, 659–668 (1983)

    Article  MathSciNet  Google Scholar 

  45. Maskit, B.: Self-maps of Kleinian groups. Am. J. Math. 93, 840–856 (1971)

    Article  MathSciNet  Google Scholar 

  46. Masur, H.: Extension of the Weil–Petersson metric to the boundary of Teichmüller space. Duke Math. J. 43(3), 623–635 (1976)

    Article  MathSciNet  Google Scholar 

  47. Moore, R.L.: Concerning upper semi-continuous collection of continua. Trans. AMS 27, 412–428 (1925)

    Article  MathSciNet  Google Scholar 

  48. Morrey Jr., C.B.: On the solutions of quasi-linear elliptic partial differential equations. Trans. Am. Math. Soc. 43(1), 126–166 (1938)

    Article  MathSciNet  Google Scholar 

  49. Nag, S.: The Complex Analytic Theory of Teichmüller Spaces. Canadian Mathematical Society, Series of Monographs and Advances Texts. Wiley, New York (1988)

    MATH  Google Scholar 

  50. Natanzon, S.M.: Uniformization of spaces of meromorphic functions. Sov. Math. Dokl. 33, 487–490 (1986)

    MATH  Google Scholar 

  51. Natanzon, S.M.: Fuchsian groups and uniformization of Hurwitz spaces. Disertaciones del Seminario de Matemáticas Fundamentales, vol. 19, Universidad Nacional de Educación a Distancia. https://www.researchgate.net/publication/28272129 (1999)

  52. Ohshika, K.: Geometrically finite Kleinian groups and parabolic elements. Proc. Edinb. Math. Soc. (2) 41(1), 141–159 (1998)

    Article  MathSciNet  Google Scholar 

  53. Royden, H.L.: Intrinsic metrics on Teichmüller space. In: Proceedings of the International Congress of Mathematicians (Vancouver, B. C., 1974), vol. 2, pp. 217–221. Canadian Mathematical Congress, Montreal, Quebec (1975)

  54. Severi, F.: Vorlesungen über algebraische Geometrie. Teubner-Verlag, Leipzig (1921)

    Book  Google Scholar 

  55. Sullivan, D.: On the ergodic theory at infinity of an arbitrary discrete group of hyperbolic motions, Riemann surfaces and related topics. Ann. Math. Stud. 97, 465–496 (1981)

    Google Scholar 

  56. Thurston, W.P.: On the geometry and dynamics of diffeomorphisms of surfaces. Am. Math. Soc. Bull. New Ser. 19(2), 417–431 (1988)

    Article  MathSciNet  Google Scholar 

  57. Tromba, A.J.: On a natural algebraic affine connection on the space of almost complex structures and the curvature of Teichmüller space with respect to its Weil–Petersson metric. Manuscripta Math. 56(4), 475–497 (1986)

    Article  MathSciNet  Google Scholar 

  58. Wolpert, S.A.: Chern forms and the Riemann tensor for the moduli space of curves. Invent. Math. 85(1), 119–145 (1986)

    Article  MathSciNet  Google Scholar 

  59. Wolpert, S.A.: Geodesic length functions and the Nielsen problem. J. Differ. Geom. 25(2), 275–296 (1987)

    Article  MathSciNet  Google Scholar 

  60. Wolpert, S.A.: Geometry of the Weil–Petersson completion of the Teichmüller space. Surv. Differ. Geom. 8, 357–393 (2003)

    Article  Google Scholar 

Download references

Acknowledgements

The author is very grateful to the referee, in special for pointing out some references which improved the content and clarity in the presentation of this paper.

Author information

Authors and Affiliations

  1. Departamento de Matemática y Estadística, Universidad de La Frontera, Temuco, Chile

    Rubén A. Hidalgo

Authors
  1. Rubén A. Hidalgo
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Rubén A. Hidalgo.

Ethics declarations

Conflict of interest

The author declares that he has no conflicts of interest.

Additional information

To the memory of Alexander Vasil’ev.

Supported by Project Fondecyt 1150003 and Project Anillo ACT1415 PIA CONICYT.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Hidalgo, R.A. Uniformizations of stable \((\gamma ,n)\)-gonal Riemann surfaces. Anal.Math.Phys. 8, 655–677 (2018). https://doi.org/10.1007/s13324-018-0253-5

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s13324-018-0253-5

Keywords

Mathematics Subject Classification

Search

Navigation