Skip to main content
SpringerLink
Log in

Angle measures, general rotations, and roulettes in normed planes

  • Published:
Analysis and Mathematical Physics Aims and scope Submit manuscript

Abstract

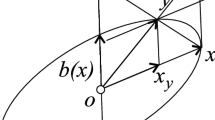
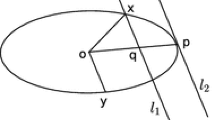
In this paper a special group of bijective maps of a normed plane (or, more generally, even of a plane with a suitable Jordan curve as unit circle) is introduced which we call the group of general rotations of that plane. It contains the isometry group as a subgroup. The concept of general rotations leads to the notion of flexible motions of the plane, and to the concept of Minkowskian roulettes. As a nice consequence of this new approach to motions the validity of strong analogues to the Euler-Savary equations for Minkowskian roulettes is proved.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7

Similar content being viewed by others

Notes

  1. As the Riemannian one.

  2. Normality means the so-called Birkhoff orthogonality of the Minkowski plane.

References

  1. Alonso, J., Martini, H., Wu, S.: On Birkhoff orthogonality and isosceles orthogonality in normed linear spaces. Aequ. Math. 83, 153–189 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  2. Balestro, V., Horváth, Á. G., Martini, H., Teixeira, R.: Angles in normed spaces. Aequ. Math. https://arxiv.org/pdf/1607.06938.pdf (to appear)

  3. Bliss, G.A.: A generalization of the notion of angle. Trans. Am. Math. Soc. 7, 184–196 (1906)

    Article  MathSciNet  MATH  Google Scholar 

  4. Brass, P.: Erdős distance problems in normed spaces. Comput. Geom. 6, 195–214 (1996)

    Article  MathSciNet  MATH  Google Scholar 

  5. Busemann, H.: Angular measure and integral curvature. Canad. J. Math. 1, 279–296 (1949)

    Article  MathSciNet  MATH  Google Scholar 

  6. Busemann, H.: The geometry of Finsler spaces. Bull. Am. Math. Soc. 56, 5–16 (1950)

    Article  MathSciNet  MATH  Google Scholar 

  7. Busemann, H.: The foundations of Minkowskian geometry. Comment. Math. Helv. 24, 156–187 (1950)

    Article  MathSciNet  MATH  Google Scholar 

  8. Chiang, C.H.: Spherical kinematics in contrast to planar kinematics. Mech. Mach. Theory 27(3), 243–250 (1992)

    Article  Google Scholar 

  9. Dekster, B.V.: An angle in Minkowski space. J. Geom. 80, 31–47 (2004)

    MathSciNet  MATH  Google Scholar 

  10. Dekster, B.V.: A metric space of directions in Minkowski space. J. Geom. 80, 48–64 (2004)

    MathSciNet  MATH  Google Scholar 

  11. Dekster, B.V.: Total angle around a point in Minkowski plane. J. Geom. 93, 38–45 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  12. Diminnie, C.R., Andalafte, E.Z., Freese, R.W.: Generalized angles and a characterization of inner product spaces. Houst. J. Math. 14, 475–480 (1988)

    MathSciNet  MATH  Google Scholar 

  13. Dragomir, S.S.: Semi-Inner Products and Applications. Nova Science Publishers Inc., Hauppauge, NY (2004)

  14. Düvelmeyer, N.: Angle measures and bisectors in Minkowski planes. Can. Math. Bull. 48(4), 523–534 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  15. Fankhänel, A.: I-measures in Minkowski planes. Beitr. Algebra Geom. 50, 295–299 (2009)

    MathSciNet  MATH  Google Scholar 

  16. Fankhänel, A.: On angular measures in Minkowski planes. Beitr. Algebra Geom. 52, 335–342 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  17. Finsler, P.: Über Kurven und Flächen in allgemeinen Räumen, Dissertation, Göttingen (1918)

  18. Finsler, P.: Über eine Verallgemeinerung des Satzes von Meusnier. Vierteljahrsschr. Naturf. Ges. Zürich 85, 155–164 (1940)

    MATH  Google Scholar 

  19. Graham, R.L., Witsenhausen, H.S., Zassenhaus, H.J.: On tightest packings in the Minkowski plane. Pac. J. Math. 41(3), 699–715 (1972)

    Article  MathSciNet  MATH  Google Scholar 

  20. Hilbert, D.: Grundlagen der Geometrie, Teubner-Archiv zur Mathematik. Teubner, Stuttgart (1999)

    Google Scholar 

  21. Horváth, Á.G., Lángi, Zs., Spirova, M.: Semi-inner products and the concept of semi-polarity. Res. Math. (2015). doi:10.1007/s00025-015-0510-y

  22. Horváth, Á.G.: Semi-indefinite inner product and generalized Minkowski spaces. J Geom. Phys. 60, 1190–1208 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  23. Horváth, Á.G.: Premanifolds. Note di Matematica 31(2), 17–51 (2011)

  24. Garcia-Roig, J.-L.: On the group of isometries and a system of functional equations of a real normed plane, Inner Product Spaces and Applications, Pitman Research Notes in Mathematics Series 376, pp. 42–53. Longman, Harlow (1997)

    Google Scholar 

  25. Gunawan, H., Lindiarni, J., Neswan, O.: \(P\)-,\(I\)-,\(g\)-, and \(D\)-angles in normed spaces. ITB J. Sci. 40(1), 24–32 (2008)

    Article  Google Scholar 

  26. Gungor, M.A., Pirdal, A.Z., Tosun, M.: The Euler-Savary formula for the Lorentzian planar homothetic motions. Int. J. Math. Comb. 2, 102–111 (2010)

    MATH  Google Scholar 

  27. Haantjes, J.: Distance geometry. Curvature in abstract metric spaces. Nederl. Akad. Wetensch Proc. 50, 496–508 (1947)

    MathSciNet  MATH  Google Scholar 

  28. Ikawa, T.: Euler-Savary’s formula in Minkowski geometry. Balk. J. Geom. 8(2), 31–36 (2003)

    MATH  Google Scholar 

  29. Kitson, D., Power, S.C.: Infinitesimal rigidity for non-Euclidean bar-joint frameworks. Bull. Lond. Math. Soc. 46(4), 685–697 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  30. Kitson, D., Power, S.C.: The rigidity of infinite graphs. Preprint (2013). https://arxiv.org/pdf/1310.1860v1.pdf

  31. Kitson, D.: Finite and infinitesimal rigidity with polyhedral norms. Discret. Comput. Geom. 54(2), 390–411 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  32. Kitson, D., Schulze, B.: Maxwell-Laman counts for bar-joint frameworks in normed spaces. Linear Algebra Appl. 15, 313–329 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  33. Laman, G.: On graphs and the rigidity of plane skeletal structures. J. Eng. Math. 4, 331–340 (1970)

    Article  MathSciNet  MATH  Google Scholar 

  34. Landsberg, G.: Über die Krümmung in der Variationsrechnung. Math. Ann. 65, 313–349 (1908)

    Article  MathSciNet  MATH  Google Scholar 

  35. Ling, J.M.: On Dekster’s angle measure in Minkowski spaces. J. Geom. 85, 72–76 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  36. Lippmann, H.: Zur Winkeltheorie in zweidimensionalen Minkowski- und Finsler-Räumen. Indag. Math. 60, 162–170 (1957)

    Article  MATH  Google Scholar 

  37. Lippmann, H.: Metrische Eigenschaften verschiedener Winkelmaße im Minkowski- und Finslerraum, I. Indag. Math. 61, 223–230 (1958)

    Article  MathSciNet  MATH  Google Scholar 

  38. Lippmann, H.: Metrische Eigenschaften verschiedener Winkelmaße im Minkowski- und Finslerraum, II. Indag. Math. 61, 231–238 (1958)

    Article  MathSciNet  MATH  Google Scholar 

  39. Lumer, G.: Semi-inner product spaces. Trans. Am. Math. Soc. 100, 29–43 (1961)

    Article  MathSciNet  MATH  Google Scholar 

  40. Martini, H., Spirova, M.: Reflections in strictly convex Minkowski planes. Aequ. Math. 78, 71–85 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  41. Martini, H., Spirova, M., Strambach, K.: Geometric algebra of strictly convex Minkowski planes. Aequ. Math. 88, 49–66 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  42. Martini, H., Swanepoel, K.: Antinorms and Radon curves. Aequ. Math. 71, 110–138 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  43. Martini, H., Swanepoel, K.J., Weiss, G.: The geometry of Minkowski spaces—a survey. Part I Expo. Math. 19, 97–142 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  44. Masal, M., Ersoy, S., Güngör, M.A.: The Euler-Savary formula for the homothetic motion in the complex plane. Ain Shams Eng. J. 5, 305–308 (2014)

    Article  Google Scholar 

  45. Menger, K.: Untersuchungen über allgemeine Metrik. Vierte Untersuchung. Math. Ann. 103, 466501 (1930)

    Article  Google Scholar 

  46. Milic̆ic, P.: On the \(B\)-angle and \(g\)-angle in a normed space. J. Inequal. Pure Appl. Math. 8/3, 9 (2007). (no. 4, Art. 99)

    Google Scholar 

  47. Önder, M., Hüseyin Uğurlu, H., Çalışkan, A.: The Euler-Savary analogue equations of a point trajectory in Lorentzian spatial motion. Proc. Nat. Acad. Sci. India Sect. A Phys. Sci. 83(2), 119–127 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  48. Rattan, S.S.: Theory of machines. Tata McGraw Hill, New Delhi (2009)

    MATH  Google Scholar 

  49. Sá Pereira, N.T., Ersoy, S.: Elliptical harmonic motion and the Euler-Savary formula. Adv. Appl. Clifford Algebras 26(2), 731–755 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  50. Thompson, A.C.: Minkowski Geometry, Encyclopedia of Mathematics and its Applications. Cambridge University Press, Cambridge (1996)

    Google Scholar 

  51. Underhill, A.L.: Invariants of the function \(F(x, y, x^{\prime }, y^{\prime })\) in the calculus of variations. Trans. Am. Math. Soc. 9, 316–338 (1908)

    MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. CEFET/RJ Campus Nova Friburgo, Nova Friburgo, Brazil

    Vitor Balestro

  2. Instituto de Matemática e Estatística, UFF, Niterói, Brazil

    Vitor Balestro

  3. Department of Geometry, Budapest University of Technology and Economics, 1521, Budapest, Hungary

    Ákos G. Horváth

  4. Fakultät für Mathematik, Technische Universität Chemnitz, 09107, Chemnitz, Germany

    Horst Martini

  5. Department of Applied Mathematics, Harbin University of Science and Technology, Harbin, 150080, China

    Horst Martini

Authors
  1. Vitor Balestro
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Ákos G. Horváth
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Horst Martini
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Vitor Balestro.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Balestro, V., Horváth, Á.G. & Martini, H. Angle measures, general rotations, and roulettes in normed planes. Anal.Math.Phys. 7, 549–575 (2017). https://doi.org/10.1007/s13324-016-0155-3

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s13324-016-0155-3

Keywords

Mathematics Subject Classification

Search

Navigation