Three-Player Games with Strategy-Dependent Time Delays

Abstract

We analyze replicator dynamics with strategy-dependent time delays in a certain three-player game with one pure and two mixed Nash equilibria. In such a model, new players are born from parents who interacted in the past. We show that stationary states depend on time delays. Moreover, at certain time delays, interior equilibria cease to exist.

Appendix

Proof of Theorem 1

Let us denote $\alpha =\frac{\tau _A}{\tau _A-\tau _B}$. The equation $F(x)=0$ is equivalent to $F_L(x)= F_R(x)$, where

$$\begin{aligned} F_L(x)= & {} \frac{\alpha }{\tau _A}\ln \left( \frac{x^2 + 2(1- x)^2}{4(1 - x)x + (1-x)^2}\right) , \\ F_R(x)= & {} \Bigl (x^2 + 2(1- x)^2\Bigr ) \left( \frac{4(1 - x)x + (1-x)^2}{x^2+ 2(1- x)^2}\right) ^{\alpha }. \end{aligned}$$

First, we consider the case $\tau _B>\tau _A$. We calculate the second derivative of $F_L$ with respect to x and we get

$$\begin{aligned} \begin{aligned} F_L'(x)&= -\frac{2\alpha }{\tau _A} \frac{3x^2-9x+4}{(1-x)(3x+1)\Bigl (\bigl (x\sqrt{3}-\frac{2}{\sqrt{3}}\bigr )^2+\frac{2}{3}\Bigr )}, \\ F_L''(x)&= \frac{\alpha }{\tau _A}\left( \frac{1}{(x-1)^2}+\frac{9}{(3x+1)^2}+\frac{8}{(3x^2-4x+2) ^2}-\frac{6}{3x^2-4x+2}\right) . \end{aligned} \end{aligned}$$

After some computations (reducing the expression in brackets to a common denominator and plotting—or estimating—a polynomial of the fifth degree of the numerator) it is possible to show that $F_L''(x)<0$ for all $x\in (0,1)$ and $\alpha <0$. Thus, $F_L$ is concave. On the other hand, the derivatives of $F_R$ read

$$\begin{aligned} \begin{aligned} F_R'(x)&= \frac{\bigl (4(1 - x)x + (1-x)^2\bigr )^{\alpha -1}}{\bigl (x^2 + 2(1- x)^2\bigr )^{\alpha }} \Bigl (2\alpha \bigl (x^2-5x^2+2\bigr )+2(x(x-1)(3x+1)\Bigr ),\\ F_R''(x)&= 2\frac{\bigl (4(1 - x)x + (1-x)^2\bigr )^{\alpha -2}}{\bigl (x^2 + 2(1- x)^2\bigr )^{\alpha +1}} h(x), \end{aligned} \end{aligned}$$

where

$$\begin{aligned} h(x)= & {} 2 \alpha ^2 \left( 3 x^2-9 x+4\right) ^2-\alpha \left( 54 x^5-171 x^4+120 x^3+111 x^2-156 x+50\right) \\&+\,3 (x-1)^2 \left( 3 x^2-4 x+2\right) (3 x+1)^2. \end{aligned}$$

Plotting the polynomial of the fifth degree shows that the coefficient in $\alpha$ is positive for $x\in (0,1)$, and therefore, $h(x)<0$ for $x\in (0,1)$. Thus, $F_R$ is convex. This proves that equation $F_L(x)=F_R(x)$ has at most two solutions in (0, 1).

Note that in this case ($\tau _A<\tau _B$), we have

$$\begin{aligned} \lim _{x\rightarrow 0+} F_L(x) = -\frac{|\alpha |}{\tau _A}\ln 2<0, \quad \lim _{x\rightarrow 1-} F_L(x) = -\infty , \end{aligned}$$

and

$$\begin{aligned} \lim _{x\rightarrow 0+} F_R(x) = 2^{1-\alpha }>0, \quad \lim _{x\rightarrow 1-} F_R(x) = 0. \end{aligned}$$

If we compare the value of $F_L$ and $F_R$ at $x=\frac{1}{2}$, we deduce that if

$$\begin{aligned} \tau _A<\tau _B<\tau _A+\frac{4}{3}\ln \frac{5}{3}, \end{aligned}$$

then $F_L(\frac{1}{2}) > F_R(\frac{1}{2})$ so there are exactly two solutions of $F_L(x)=F_R(x)$ in the interval (0, 1).

On the other hand, one can easily estimate that

$$\begin{aligned} F_L(x) \le \frac{\alpha }{\tau _A} \ln \frac{\sqrt{33}-1}{8} \end{aligned}$$

for all $x\in (0,1)$. Because $(1-x)^2+4x(1-x)\le 4/3$ and $x^2 + 2(1- x)^2\ge \frac{2}{3}$, one deduce that $F_R(x) \ge \frac{1}{2}$. This completes the proof of part (ii) of the theorem.

Now, assume that $\tau _A>\tau _{B}$ which implies that $\alpha >1$. In this case, it is convenient to change variables. Let

$$\begin{aligned} y = \frac{1-x}{x} \; \Longleftrightarrow \; x = \frac{1}{1+y}. \end{aligned}$$

The problem of finding solution of $F_L(x)=F_R(x)$ for $x\in (0,1)$ transforms to the problem of finding solution of $G_L(y)=G_R(y)$ for $y>0$, where

$$\begin{aligned} G_L(y) = -\frac{\alpha }{\tau _A} \ln \left( \frac{4y+y^2}{1+2y^2}\right) , \quad G_R(y) = \frac{1+2y^2}{(1+y)^2}\left( \frac{4y+y^2}{1+2y^2}\right) ^\alpha . \end{aligned}$$

It is very easy to calculate limits of $G_L$ and $G_R$ at 0 and at $+\infty$ and get

$$\begin{aligned} \begin{aligned} \lim _{y\rightarrow 0+} G_L(y)&= +\infty ,&\qquad \lim _{y\rightarrow +\infty } G_L(y)&= \frac{\alpha }{2}\ln 2, \\ \lim _{y\rightarrow 0+} G_R(y)&= 0,&\lim _{y\rightarrow +\infty } G_R(y)&= 2^{1-\alpha }. \end{aligned} \end{aligned}$$

We calculate the derivative of $G_L$ and we get

$$\begin{aligned} G_L'(y) = \frac{2\alpha }{\tau _{A}}\cdot \frac{4y^2-y-2}{y(4+y)(1+2y^2)}. \end{aligned}$$

It is easy to see that for $\alpha >1$, the function $G_L$ is decreasing for $y\in (0,{\tilde{y}})$ with $\displaystyle {\tilde{y}} = \frac{1+\sqrt{33}}{8}$, and it is increasing for $y>{\tilde{y}}$. Note also that

$$\begin{aligned} G_L({\tilde{y}}) = -\frac{\alpha }{\tau _{A}}\ln \left( \frac{2{\tilde{y}}({\tilde{y}}+2{\tilde{y}}^2)+{\tilde{y}}(2+{\tilde{y}}-4{\tilde{y}}^2)}{1+2{\tilde{y}}^2}\right) = -\frac{\alpha }{\tau _{A}}\ln (2{\tilde{y}}) <0, \end{aligned}$$

while $G_R(Y)>0$ for $y>0$. Now, we prove that $G_R$ is increasing on $(0,{\tilde{y}})$. We calculate the derivative of $G_R$ with respect to y and we obtain

$$\begin{aligned} G_R'(y) = \frac{2(4y+y^2)^{\alpha -1}}{(1+2y^2)^\alpha (1+y)^3} g(y) \end{aligned}$$

with

$$\begin{aligned} g(y) = (1+2y^2)(2-y) + (1+y)(2+y-4y^2)(\alpha -1). \end{aligned}$$

Because ${\tilde{y}}<2$ and $\alpha >1$, we easily see that $g(y)>0$ for $y\in (0,{\tilde{y}})$. Thus, $G_R$ is increasing in $(0,{\tilde{y}})$, and therefore, there exists exactly one solution of $G_L(y)=G_R(y)$ in $(0,{\tilde{y}})$.

One can see that $G_L(x) =0$ for ${\hat{y}}_1=2-\sqrt{3}$ and ${\hat{y}}_2=2+\sqrt{3}$. As ${\hat{y}}_2>2>{\tilde{y}}$, we see that $g(y)<0$ for $y>{\hat{y}}_2$, thus $G_R$ is decreasing while $G_L$ is increasing for $y>{\hat{y}}_2$. Thus, the second solution to $G_L(y)=G_R(y)$ exists in the interval $({\tilde{y}}, +\infty )$ if and only if $G_L(+\infty )>G_R(+\infty )$, that is $\alpha \ln 2 > 2^{1-\alpha }$. Some simple algebra finishes the proof of the theorem. $\square$

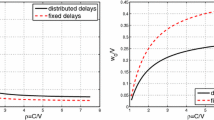
1.1 Formula Used for Drawing Stationary Solutions

Here, we derive a formula that gives us a better relation between $\tau _A$, $\tau _B$, and ${\bar{x}}$ than the equation $F(x)=0$. As before, let us denote

$$\begin{aligned} \alpha =\frac{\tau _A}{\tau _A-\tau _B}, \quad U_A = x^2 + 2(1- x)^2, \quad U_B = 4(1 - x)x + (1-x)^2. \end{aligned}$$

The equation $F(x)=0$ is then equivalent to

$$\begin{aligned} \frac{1}{\tau _{A}}\alpha \ln \frac{U_A}{U_B} = U_A \biggl (\frac{U_A}{U_B}\biggr )^\alpha \; \Longleftrightarrow \; \tau _A U_A = \ln \biggl (\frac{U_A}{U_B}\biggr )^\alpha \exp \Biggl (\ln \biggl (\frac{U_A}{U_B}\biggr )^\alpha \Biggr ). \end{aligned}$$

Thus,

$$\begin{aligned} \ln \biggl (\frac{U_A}{U_B}\biggr )^\alpha = W_L(\tau _A U_A) \; \Longleftrightarrow \; \alpha = \frac{ W_L(\tau _A U_A)}{\ln \frac{U_A}{U_B}}, \end{aligned}$$

where $W_L$ is a Lambert W function, that is $x = W_L(x)\exp \bigl (W_L(x)\bigr )$. Now, using the definition of $\alpha$ we get

$$\begin{aligned} \frac{\tau _{A}}{\tau _A-\tau _B} = \frac{ W_L(\tau _A U_A)}{\ln \frac{U_A}{U_B}} \; \Longleftrightarrow \; \tau _{B} = \tau _{A}\Biggl (1-\frac{\ln \frac{U_A}{U_B}}{W_L(\tau _AU_A)}\Biggr ). \end{aligned}$$

In this manner, we get a relation between $\tau _A$ and $\tau _B$ at the stationary state x, namely

$$\begin{aligned} \tau _{B} = \tau _{A}\Biggl (1-\frac{\ln \frac{x^2 + 2(1- x)^2}{4(1 - x)x + (1-x)^2}}{W_L\bigl ((x^2 + 2(1- x)^2)\tau _A\bigr )}\Biggr ). \end{aligned}$$

(17)

In an analogous manner—denoting $\beta = \frac{\tau _B}{\tau _A+\tau _B}$—we get another relation between $\tau _A$ and $\tau _B$ at the stationary state x, namely

$$\begin{aligned} \tau _{A} = \tau _{B}\Biggl (1+\frac{\ln \frac{x^2 + 2(1- x)^2}{4(1 - x)x + (1-x)^2}}{W_L\bigl ((4(1 - x)x + (1-x)^2)\tau _B\bigr )}\Biggr ). \end{aligned}$$

(18)

We used formulas (17) and (18) to draw Fig. 2a.