Abstract
Most carbonate reservoirs in Middle East are characterized as porous fractured reservoirs. Estimation of relative permeability of these highly heterogeneous reservoirs is challenging due to the existence of discontinuity in the fluid flow fractured porous media. Although relative permeability is an essential data for simulation of flow in fractured media, few attempts have so far been made to estimate the relative permeability curves. Most notable are the studies by Akin (J Pet Sci Eng 30(1)1–14, 2001), Al-sumaiti and Kazemi (2012), and Fahad (2013). This paper presents an integrated approach to history matching the oil drainage tests, which were carried out by unsteady state, on glass bed models with a single fracture at different orientations and to estimate the relative permeability curve. The integrated approach includes an inversion algorithm coupled with forward numerical modeling of fluid flow. The history matching of the displacement test data was obtained by using the Levenberg–Marquardt algorithm to minimize the error between the simulated and experimental data. In this algorithm, Corey-type power law is used to create relative permeability curves during the optimization procedures. The forward modeling is a 3D multiphase fluid simulator for flow through discrete fractures. Numerical results of fluid flow profiles and the optimized relative permeability curves for single fracture with different orientations and experimental validation with oil drainage tests are presented. The results of the optimized relative permeability data for single fracture are in a good agreement with the data derived by the correlation of Fahad (2013). These results prove that the presented approach can be used to upscale the relative permeability curve from laboratory scale to reservoir grid scale. The work on the upscaling of the estimated relative permeability curve of fractured porous media is under preparation and will be published soon.



















Similar content being viewed by others
References
Akin S (2001) Estimation of fracture relative permeabilities from unsteady state core floods. J Pet Sci Eng 30(1):1–14
Al-sumaiti AM, Kazemi H (2012) Experimental and numerical modeling of double displacement oil recovery process in tight fractured carbonate reservoirs. In: Abu Dhabi international petroleum conference and exhibition. Society of Petroleum Engineers
Arnold DN, Brezzi F, Cockburn B, Marini LD (2002) Unified analysis of discontinuous Galerkin methods for elliptic problems. SIAM J Numer Anal 39(5):1749–1779
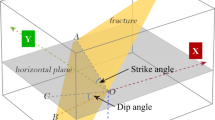
Bandis S, Lumsden A, Barton N (1983) Fundamentals of rock joint deformation. Int J Rock Mech Min Sci Geomech Abs 20(6):249–298
Buckley SE, Leverett M (1942) Mechanism of fluid displacement in sands. Trans AIME 146(01):107–116
Chima A, Chavez Iriarte EA, Carrillo C, Himelda Z (2010) An Equation to predict two-phase relative permeability curves in fractures. Paper presented at the SPE Latin American and Caribbean petroleum engineering conference
De la Porte JJ, Kossack CA, Zimmerman RW (2005) The effect of fracture relative permeabilities and capillary pressures on the numerical simulation of naturally fractured reservoirs. Paper presented at the SPE annual technical conference and exhibition
Fahad M (2013) Simulation of fluid flow and estimation of production from naturally fractured reservoirs. PhD thesis
Hassler G, Brunner E (1945) Measurement of capillary pressures in small core samples. Trans AIME 160(01):114–123
Hoteit H, Firoozabadi A (2005) Multicomponent fluid flow by discontinuous Galerkin and mixed methods in unfractured and fractured media. Water Resour Res 41(11):1–15
Hoteit H, Firoozabadi A (2008) Numerical modeling of two-phase flow in heterogeneous permeable media with different capillarity pressures. Adv Water Resour 31(1):56–73
Karimi-Fard M, Durlofsky L, Aziz K (2004) An efficient discrete-fracture model applicable for general-purpose reservoir simulators. SPE J 9(02):227–236
Kazemi H, Merrill L Jr, Porterfield K, Zeman P (1976) Numerical simulation of water–oil flow in naturally fractured reservoirs. Soc Petrol Eng J 16(06):317–326
Lewis RW, Ghafouri HR (1997) A novel finite element double porosity model for multiphase flow through deformable fractured porous media. Int J Numer Anal Methods Geomech 21(11):789–816
Moinfar A, Varavei A, Sepehrnoori K, Johns RT (2014) Development of an efficient embedded discrete fracture model for 3D compositional reservoir simulation in fractured reservoirs. SPE J 19(2):289–303
Monteagudo J, Firoozabadi A (2004) Control‐volume method for numerical simulation of two‐phase immiscible flow in two‐and three‐dimensional discrete‐fractured media. Water Resour Res 40(7):W07405
Monteagudo JE, Firoozabadi A (2007) Control-volume model for simulation of water injection in fractured media: incorporating matrix heterogeneity and reservoir wettability effects. SPE J 12(03):355–366
Monteagudo J, Rodriguez A, Florez H (2011) Simulation of flow in discrete deformable fractured porous media. Paper presented at the SPE reservoir simulation symposium, The Woodlands, TX
Persoff P, Pruess K, Myer L (1991) Two-phase flow visualization and relative permeability measurement in transparent replicas of rough-walled rock fractures. Lawrence Berkeley Lab
Pieters DA, Graves RM (1994) Fracture relative permeability: linear or non-linear function of saturation. In: International Petroleum Conference and Exhibition of Mexico. Society of Petroleum Engineers
Piggott AR, Elsworth D (1991) A hydromechanical representation of rock fractures. Paper presented at the proceedings of the 32nd US symposium on rock mechanics, University of Oklahoma
Pruess K, Tsang Y (1990) On two-phase relative permeability and capillary pressure of rough-walled rock fractures. Water Resour Res 26(9):1915–1926
Raviart P-A, Thomas J-M (1977) A mixed finite element method for 2-nd order elliptic problems mathematical aspects of finite element methods. Springer, Berlin, pp 292–315
Romm E (1966) Flow characteristics of fractured rocks. Nedra, Moscow 283
Thomas LK, Dixon TN, Pierson RG (1983) Fractured reservoir simulation. SPEJ 23(1):42–54. doi:10.2118/9305-PA
Vohralík M (2007) A posteriori error estimates for lowest-order mixed finite element discretization of convection–diffusion-reaction equations. SIAM J Numer Anal 45(4):1570–1599
Warren J, Root PJ (1963) The behavior of naturally fractured reservoirs. SPE J 3(3):245–255
Welge HJ (1952) A simplified method for computing oil recovery by gas or water drive. J Petrol Technol 4(04):91–98
Author information
Authors and Affiliations
Corresponding author
Appendix: Derivation of multiphase flow equations in a poroelastic framework
Appendix: Derivation of multiphase flow equations in a poroelastic framework
The mathematical equations of multiphase fluid flow through matrix and fracture system are derived and then reformulated using finite element technique. The combining of mass and momentum balance equations is used in the derivation process with appropriate boundary conditions and a set of assumptions. These assumptions include: (1) The system is only for water and oil fluid flow, and (2) the fluid is isothermal and fluid phases are in a thermodynamic equilibrium state.
Mass conversation equation
The conversation equations of masses of fluid and solid for a representative element volume (V) in a porous medium will be derived separately. The mass of solid component of the medium can be expressed as a volume fraction of the porous solid as the following equation shows,
where \(\phi\) is the sum of fracture and matrix porosities and \(\rho_{\text{s}}\) is the solid density
The mass conversation of solid constituent can be written as;
Equation (18) can be simplified if the continuum mechanism is assumed to be revealed;
Mass conversation for oil and water phases in rock and fracture systems can be derived similarly as follows:
The mass of solid component of the medium is expressed as:
The mass conversation of fluid constituent can be written as;
where \(\psi\) stands for oil and water phases, \(S_{\psi }\) is the saturation of fluid phase (oil and water), \(\rho_{\psi }\) is the fluid density, and \(q_{\psi }\) is the rate of fluid exchange between matrix and fracture system. Equation (21) can be written separately for oil and water fluid phases separately by assuming that the continuum mechanism is prevailing as follows:
where \(U_{\text{w}}\) and \(U_{\text{o}}\) are the intrinsic velocities for water and oil phases in matrix and fractured system, respectively. The assumption of quasi-steady-state flux between the fracture network and matrix is used; therefore, flux rate can be expressed as:
where \(k_{1}\) is referring to the permeability of the matrix and \(p_{1\psi } ,p_{2\psi }\) are the pressure in the matrix and fracture system, respectively.
Darcy velocities for oil and water phases in matrix and fracture system are defined as:
From Eq. (25) intrinsic phase velocities can be expressed as:
Substituting Eq. (26) into Eq. (23) and then the equation reformulated as follows:
Expanding the derivatives of Eqs. (19) and (27)
Equations (29) and (30) can be reformulated and written as follows:
By considering the total derivative as:
Equations (31) and (32) can be written by considering the total derivative form as:
From Eq. (34), the expression of change of porosity with time can be written as:
Substituting Eq. (36) into Eq. (35), the following equation can be got:
Or.
The relationships between the change of fluid and rock densities with fluid and rock bulk modulus are given as:
where Kw and Ko are the bulk modulus of water and oil, respectively. Ks and Kns are the bulk modulus of solid rock.
Substituting Eq. (39) into Eq. (38) gets the following equation:
In order to simplify Eq. (40) and get the final form of the two-phase fluid flow equation, the following assumptions are used as:
-
The total derivative is considered as:
$$\frac{{{\text{D}}(*)}}{{{\text{D}}t}} = \frac{\partial (*)}{\partial t} + u\frac{\partial (*)}{\partial x}$$ -
Combine Eq. (C.24) with Darcy’s law:
$$u_{\psi } = - \frac{{k_{\psi } }}{{\mu_{\psi } }}k_{r\psi } \left( {P_{\psi } + \rho_{\psi } gh} \right)$$(41) -
Solid velocity is small and can be neglected
where \(\psi\) is standing for oil and water phase, \(k_{r\psi }\) is phase relative permeability, \(\rho_{\psi }\) is phase fluid density, g is the gravitational acceleration, and \(h\) is the height above reference level.
The water-phase flow governing equation will be as follows:
The oil-phase fluid flow governing equation can be written as:
where \(P_{\text{wm}}\) and \(P_{\text{wf}}\) are the water pressures inside matrix and fractures, respectively.
Momentum balance equation
The relationship between total applied stresses \(\sigma_{ij}\) and intergranular (effective) stresses \(\sigma_{ij}^{\prime}\) is given by:
where \(\alpha\) is pore pressure ratio factor, and \(\delta_{ij}\) is the Kronecker delta.The linear constitutive relationships of the system can be expressed as:
where \(D_{ijkl}\) is the elasticity matrix.
The equilibrium equation of motion for a solid can be defined as:
where \(F\) is the vector of tractions applied on the body. The strain–displacement relationship is defined as:
Rearranging Eq. 42 for water phase and introduce strain, then same procedure for oil phase:
Finite element discretization
In this section the finite element technique is used to derive the integral formulation of the derived coupled fluid flow and rock deformation equations through fractured system. In addition, the finite element technique is used to discretize the problem domain into nodes and elements. In this paper, four-node tetrahedral elements were used to represent the rock matrix in a 3D space, while the discrete fractures are represented by triangle elements in a 2D space. The value of the material properties is assumed to be constant within the element and allowed to vary from one element to the next. For the three-dimensional four-node tetrahedral element, the shape functions have the following form:
where \(\left( {\xi ,\eta ,\zeta } \right)\) are the element local coordinates system. In order to transform the element geometry from the global system coordinates (x, y, and z) to the local coordinates \(\left( {\xi ,\eta ,\zeta } \right)\), the following equations were used as:
where J is the Jacobin matrix and can be expressed as:
Shape functions are used to obtain the variation of unknown variables within the element. These variables are approximated by using the interpolation function in finite element space as follows:
where N is the corresponding shape function, \(\bar{u}\), \(\bar{P}_{\text{w}}\), and \(\bar{P}_{\text{o}}\) are the nodal unknown variables. B is the strain displacement matrix and can be defined as:
Equilibrium equation can be written in its general form as follows:
where \(\partial f\) is the load vector applied on the boundary and V is the volume of the element.
Equation (57) can be written as:
Average pore pressure (P) can be defined as:
Substituting Eq. (58) into Eq. (59);
Equation (60) can be modified by inserting the capillary pressure as:
Discretization form for water-phase flow equation through fractured porous media is given below as:
The process is repeated for oil-phase flow in fractured porous media. All of the previous equations are used for fluid flow through discrete fractures, but in 2D space the final flow equation through fracture network and matrix is given as:
where \(\bar{\varOmega }_{\text{f}}\) represents the fracture part of the domain as a 2D entity, and \(\varOmega_{\text{m}}\) represents matrix domain and \(\varOmega\) is the entire domain.
Rights and permissions
About this article
Cite this article
Abdelazim, R. An integrated approach for relative permeability estimation of fractured porous media: laboratory and numerical simulation studies. J Petrol Explor Prod Technol 10, 1–18 (2020). https://doi.org/10.1007/s13202-016-0250-x
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s13202-016-0250-x