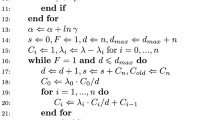Skip to main content
SpringerLink
Log in

Discrete lognormal distributions with application to insurance data

  • Original article
  • Published:
International Journal of System Assurance Engineering and Management Aims and scope Submit manuscript

Abstract

The continuous lognormal distribution has been used to model discrete count data, which is clearly not appropriate. In this paper, we introduce two discrete versions of the continuous lognormal distribution. We study their mathematical properties and estimation issues. Two real data applications show superior performance of the discrete versions over the continuous counter parts.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10

Similar content being viewed by others

References

  • Aitkin M, Anderson D, Francis B, Hinde J (1989) Stat Model GLIM. Oxford University Press, New York

    MATH  Google Scholar 

  • Alamatsaz MH, Dey S, Dey T, Shams Harandi S (2016) Discrete generalized Rayleigh distribution. Pak J Stat 32:1–20

    MathSciNet  Google Scholar 

  • Baxter LA, Coutts SM, Ross GAF (1980) Applications of linear models in motor insurance. In: Proceedings of the 21st international congress of actuaries, Zurich, pp 11–29

  • Chakraborty S (2015) Generating discrete analogues of continuous probability distributions-a survey of methods and constructions. J Stat Distrib Appl 2(6)

  • Cooray K, Cheng C-I (2015) Bayesian estimators of the lognormal-Pareto composite distribution. Scand Act J 500–515

  • James G, Witten D, Hastie T, Tibshirani R (2013) An introduction to statistical learning with applications in R. Springer Verlag, New York

    Book  Google Scholar 

  • Kim B, Noh J, Baek C (2016) Threshold estimation for the composite lognormal-GPD models. Korean J Appl Stat 29:807–822

    Article  Google Scholar 

  • Leirness JB, Kinlan BP (2018). Additional statistical analyses to support guidelines for marine avian sampling. Sterling (VA): US Department of the Interior, Bureau of Ocean Energy Management. OCS Study BOEM 2018-063

  • Luckstead J, Devadoss S (2017) Pareto tails and lognormal body of US cities size distribution. Phys A Stat Mech Appl 465:573–578

    Article  Google Scholar 

  • Moreira JAG, Zeng XHT, Amaral LAN (2015) The distribution of the asymptotic number of citations to sets of publications by a researcher or from an academic department are consistent with a discrete lognormal model. Plos One 10(e0143108)

  • Mullen RE, Gokhale SS (2005) Software defect rediscoveries: a discrete lognormal model. In: International symposium on software reliability engineering 203–212

  • Mutali S, Vernic R (2020) On the composite lognormal-Pareto distribution with uncertain threshold. Commun Stat Simul Comput. https://doi.org/10.1080/03610918.2020.1743860

    Article  Google Scholar 

  • Oliveira SLJ, Amaral Turkman MA, Pereira JMC (2012) An analysis of fire frequency in tropical savannas of northern Australia, using a satellite-based fire atlas. Int J Wildl Fire 22:479–492

    Article  Google Scholar 

  • Park S, Baek C (2018) Time-varying modeling of the composite LN-GPD. Korean J Appl Stat 31:109–122

    Google Scholar 

  • Pellegrin B, Mezzari F, Hanafi Y, Szymczyk A, Remigy JC, Causserand C (2015) Filtration performance and pore size distribution of hypochlorite aged PES/PVP ultrafiltration membranes. J Membr Sci 474:175–186

    Article  Google Scholar 

  • Pigeon M, Denuit M (2011) Composite lognormal-Pareto model with random threshold. Scand Actuar J 177–192

  • R Development Core Team (2020) R: a language and environment for statistical computing. R Foundation for Statistical Computing, Vienna, Austria

    Google Scholar 

  • Stringer MJ, Sales-Pardo M, Nunes Amaral LA (2010) Statistical validation of a global model for the distribution of the ultimate number of citations accrued by papers published in a scientific journal. J Am Soc Inf Sci Technol 61:1377–1385

    Article  Google Scholar 

  • Thelwall M, Wilson P (2014) Regression for citation data: an evaluation of different methods. J Informet 8:963–971

    Article  Google Scholar 

Download references

Acknowledgements

The authors would like to thank the editor and the three referees for careful reading and comments which greatly improved the paper.

Funding

No funds received for this research.

Author information

Authors and Affiliations

  1. Department of Mathematics, University of Manchester, Manchester, M13 9PL, UK

    Jiahang Lyu & Saralees Nadarajah

Authors
  1. Jiahang Lyu
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Saralees Nadarajah
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Saralees Nadarajah.

Ethics declarations

Conflict of interest

The authors declare that they have no conflict of interest.

Human participants and animals

We have complied with all ethical standards. Research did not involve human participants and/or animals.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Lyu, J., Nadarajah, S. Discrete lognormal distributions with application to insurance data. Int J Syst Assur Eng Manag 13, 1268–1282 (2022). https://doi.org/10.1007/s13198-021-01443-x

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s13198-021-01443-x

Keywords

Search

Navigation