Skip to main content
SpringerLink
Log in

Inventory policy for deteriorating items under trade credit when time of payment is uncertain

  • Theoretical Article
  • Published:
OPSEARCH Aims and scope Submit manuscript

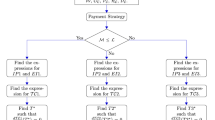
Abstract

In this paper, an inventory system with a trade credit policy when payment point is not deterministic in nature is studied for deteriorating items. Under trade credit policies, the retailers have to pay at trade credit point or at a predetermined point. In practice, it is not always possible for a retailer to strictly adhere to the same payment policy every time, may be because of uncertainty of cash in hand at respective time points or for other reasons. The optimal policies are derived by taking the possibility of not making the payment in a single point every time into consideration. Two scenarios of the policies of the supplier’s conditions are considered for which retailer may respond in different ways. Three types of retailer’s response are considered in this paper. Depending on payment pattern specific to a retailer a probability distribution for the duration of payment delay is incorporated. For all these situations the expected net present value of the total cost is derived and hence optimal policies are determined. These models are illustrated through numerical examples. The sensitivity analysis is carried out with respect to various parameters. The total costs which are optimally obtained give the actual expected cost and hence minimize budgetary variance.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Goyal, S.K.: EOQ under conditions of permissible delay in payments. J. Oper. Res. Soc. 36, 335–338 (1985)

    Article  Google Scholar 

  2. Aggarwal, S.P., Jaggi, C.K.: Ordering policies of deteriorating items under permissible delay in payments. J. Oper. Res. Soc. 46, 658–662 (1995)

    Article  Google Scholar 

  3. Chung, K.H.: Inventory control and trade credit revisited. J. Oper. Res. Soc. 40, 495–498 (1989)

    Article  Google Scholar 

  4. Arcelus, F.J., Shah, N.H., Srinivasan, G.: Retailer’s response to special sales: price discount vs. trade credit. OMEGA 29, 417–428 (2001)

    Article  Google Scholar 

  5. Chung, K.J., Liao, J.J.: The optimal ordering policy in a DCF analysis for deteriorating items when trade credit depends on the order quantity. Int. J. Prod. Econ. 100, 116–130 (2006)

    Article  Google Scholar 

  6. Hwang, H., Shinn, S.W.: Retailer’s pricing and lot sizing policy for exponentially deteriorating products under the condition of permissible delay in payments. Comput. Ind. Eng. 24(6), 539–547 (2003)

    Google Scholar 

  7. Goyal, S.K., Giri, B.C.: Recent trends in modeling of deteriorating inventory. Eur. J. Oper. Res. 134(1), 1–16 (2001)

    Article  Google Scholar 

  8. Jaggi, C.K., Aggarwal, K.K., Verma, P.: Inventory and pricing strategies for deteriorating items with limited capacity and time-proportional backlogging rate. Int. J. Oper. Res. 8(3), 331–354 (2010)

    Article  Google Scholar 

  9. Tripathi, R.P., Misra, S.S.: Credit financing in economic ordering policies of non- deteriorating items with time dependent demand rate. Int. Rev. Bus. Finance 2, 47–55 (2010)

    Google Scholar 

  10. Jaggi, C., Aggarwal, S.: Credit financing in economic ordering policies deteriorating items. Int. J. Prod. Econ. 34, 151–155 (1994)

    Article  Google Scholar 

  11. Roy, A.: An inventory model for deteriorating items with price dependent demand and time varying holding cost. Adv. Model. Optimization 10, 25–37 (2008)

    Google Scholar 

  12. Soni, H., Shah, N.H., Jaggi, C.K.: Inventory models and trade credit: a review. Control. Cybern. 39(3), 867–882 (2010)

    Google Scholar 

  13. Chaudhuri, K.S., Roy, T.: An inventory model for a deteriorating item with price-dependent demand and special sale. Int. J. Oper. Res. 2(2), 173–187 (2007)

    Article  Google Scholar 

  14. Chang, C.T., Wu, S.J., Chen, L.C.: Optimal payment time with deteriorating items under inflation and permissible delay in payments. Int. J. Syst. Sci. 40(10), 985–993 (2009)

    Article  Google Scholar 

  15. Jamal, A.M.M., Sarker, B.R., Wang, S.: An ordering policy for deteriorating items with allowable shortages and permissible delay in payment. J. Oper. Res. Soc. 48, 826–833 (1997)

    Article  Google Scholar 

  16. Liao, J.-J., Haung, K.-N.: An inventory model for deteriorating items with two levels of trade credit taking account of time discounting. J. Oper. Res. Soc. 30, 35–50 (2008)

    Google Scholar 

  17. Liao, H.C., Tsai, C.H., Su, C.T.: An inventory model with deteriorating items under inflation when a delay in payment is permissible. Int. J. Prod. Econ. 63, 207–214 (2000)

    Article  Google Scholar 

  18. Mandal, B.N., Phaujdar, S.: An inventory model for deteriorating items and stock-dependent consumption rate. J. Oper. Res. Soc. 40(5), 483–488 (1989)

    Article  Google Scholar 

  19. Ouyang, L.Y., Teng, J.T., Goyal, S.K., Yang, C.T.: An economic order quantity model for deteriorating items with partially permissible delay in payments linked to order quantity. Eur. J. Oper. Res. 194(2), 418–431 (2009)

    Article  Google Scholar 

  20. Roy, T., Chaudhuri, K.S.: A production-inventory model under stock dependent demand, Weibull distribution deterioration and shortage. Int. Trans. Oper. Res. 16(3), 325–346 (2009)

    Article  Google Scholar 

  21. Tsao, Y.C., Sheen, G.J.: Joint pricing and replenishment decisions for deteriorating items with lot-size and time-dependent purchasing cost under credit period. Int. J. Syst. Sci. 38(7), 549–561 (2007)

    Article  Google Scholar 

  22. Mohini, K., Pakkala, T.P.M.: Inventory model under trade credit when time of payment is uncertain. Int. J. Math. Arch. 3(7), 2669–2679 (2012)

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Computer Applications, T. John College, Bannerghatta Road, Bangalore, 560083, India

    Mohini Kumari

  2. Mangalore University, Mangalagangotri, Mangalore, 574199, India

    T. P. M. Pakkala

Authors
  1. Mohini Kumari
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. T. P. M. Pakkala
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to T. P. M. Pakkala.

Appendix A

Appendix A

$$ \begin{array}{l}\frac{\mathrm{d}\mathrm{PV}}{\mathrm{d}\mathrm{T}}=\frac{\mathrm{d}}{\mathrm{d}\mathrm{T}}\left[\frac{\mathrm{A}}{\left(1-{\mathrm{e}}^{-\mathrm{r}\mathrm{T}}\right)}+\frac{\mathrm{hD}}{\uptheta \left(\uptheta +\mathrm{r}\right)}\frac{\left({\mathrm{e}}^{\uptheta \mathrm{T}}-{\mathrm{e}}^{-\mathrm{r}\mathrm{T}}\right)}{\left(1-{\mathrm{e}}^{-\mathrm{r}\mathrm{T}}\right)}-\frac{\mathrm{hD}}{\uptheta \mathrm{r}}+\frac{\mathrm{CD}}{\uptheta}\frac{\left({\mathrm{e}}^{\uptheta \mathrm{T}}-1\right)}{\left(1-{\mathrm{e}}^{-\mathrm{r}\mathrm{T}}\right)}\left[\mathrm{k}\right]\right]=0\kern0.5em \&\frac{{\mathrm{d}}^2\mathrm{P}V}{{\mathrm{d}\mathrm{T}}^2}>0\\ {}\frac{1}{{\left(1-{\mathrm{e}}^{-\mathrm{r}\mathrm{T}}\right)}^2}\left[\begin{array}{l}\left(1-{\mathrm{e}}^{-\mathrm{r}\mathrm{T}}\right)(0)-\mathrm{A}\left({\mathrm{r}\mathrm{e}}^{-\mathrm{r}\mathrm{T}}\right)+\frac{\mathrm{hD}}{\uptheta \left(\uptheta +\mathrm{r}\right)}\left\{\left(1-{\mathrm{e}}^{-\mathrm{r}\mathrm{T}}\right)\left(\uptheta\;{\mathrm{e}}^{\uptheta \mathrm{T}}+{\mathrm{r}\mathrm{e}}^{-\mathrm{r}\mathrm{T}}\right)-\left({\mathrm{e}}^{\uptheta \mathrm{T}}-{\mathrm{e}}^{-\mathrm{r}\mathrm{T}}\right)\left({\mathrm{r}\mathrm{e}}^{-\mathrm{r}\mathrm{T}}\right)\right\}\\ {}+\frac{\mathrm{CD}\mathrm{K}}{\uptheta}\left\{\left(1-{\mathrm{e}}^{-\mathrm{r}\mathrm{T}}\right)\uptheta\;{\mathrm{e}}^{\uptheta \mathrm{T}}-\left({\mathrm{e}}^{\uptheta \mathrm{T}}-1\Big){\mathrm{r}\mathrm{e}}^{-\mathrm{r}\mathrm{T}}\right)\right\}\end{array}\right]=0\\ {}-\mathrm{A}\left({\mathrm{r}\mathrm{e}}^{-\mathrm{r}\mathrm{T}}\right)+\frac{\mathrm{hD}}{\uptheta \left(\uptheta +\mathrm{r}\right)}\left[{\uptheta \mathrm{e}}^{\uptheta \mathrm{T}}-{\uptheta \mathrm{e}}^{\left(\uptheta\;\mathrm{T}-\mathrm{r}\mathrm{T}\right)}+{\mathrm{r}\mathrm{e}}^{-\mathrm{r}\mathrm{T}}-{\mathrm{r}\mathrm{e}}^{-2\mathrm{r}\mathrm{T}}-{\mathrm{r}\mathrm{e}}^{\left(\uptheta\;\mathrm{T}-\mathrm{r}\mathrm{T}\right)}+{\mathrm{r}\mathrm{e}}^{-2\mathrm{r}\mathrm{T}}\right]+\\ {}\kern19.25em \frac{\mathrm{CD}\mathrm{K}}{\uptheta}\left[{\uptheta \mathrm{e}}^{\uptheta \mathrm{T}}-{\uptheta \mathrm{e}}^{\left(\uptheta\;\mathrm{T}-\mathrm{r}\mathrm{T}\right)}-{\mathrm{r}\mathrm{e}}^{\left(\uptheta\;\mathrm{T}-\mathrm{r}\mathrm{T}\right)}-{\mathrm{r}\mathrm{e}}^{-\mathrm{r}\mathrm{T}}\right]=0\\ {}\mathrm{Dividing}\kern1em \mathrm{b}\mathrm{y}\kern1em {\mathrm{r}\mathrm{e}}^{-\mathrm{r}\mathrm{T}}\kern1em \mathrm{through}\kern1em \mathrm{out}\kern1em \mathrm{the}\kern1em \mathrm{e}\mathrm{quation}\kern1em \mathrm{we}\kern1em \mathrm{get},\\ {}-\mathrm{A}+\frac{\mathrm{hD}}{\uptheta \left(\uptheta +\mathrm{r}\right)}\left[\frac{\uptheta}{\mathrm{r}}{\mathrm{e}}^{\left(\uptheta\;\mathrm{T}+\mathrm{r}\mathrm{T}\right)}-\frac{\uptheta}{\mathrm{r}}{\mathrm{e}}^{\uptheta \mathrm{T}}+1-{\mathrm{e}}^{-\mathrm{r}\mathrm{T}}-{\mathrm{e}}^{\uptheta \mathrm{T}}+{\mathrm{e}}^{-\mathrm{r}\mathrm{T}}\right]+\frac{\mathrm{CD}\mathrm{K}}{\uptheta}\left[\frac{\uptheta}{\mathrm{r}}{\mathrm{e}}^{\left(\uptheta\;\mathrm{T}+\mathrm{r}\mathrm{T}\right)}-\frac{\uptheta}{\mathrm{r}}{\mathrm{e}}^{\uptheta \mathrm{T}}-{\mathrm{e}}^{\uptheta \mathrm{T}}+1\right]=0\\ {}-\mathrm{A}+\frac{\mathrm{hD}}{\uptheta \left(\uptheta +\mathrm{r}\right)}\left[\frac{\uptheta}{\mathrm{r}}{\mathrm{e}}^{\left(\uptheta\;\mathrm{T}+\mathrm{r}\mathrm{T}\right)}-\frac{\uptheta}{\mathrm{r}}{\mathrm{e}}^{\uptheta \mathrm{T}}+1-{\mathrm{e}}^{\uptheta \mathrm{T}}\right]+\frac{\mathrm{CD}\mathrm{K}}{\uptheta}\left[\frac{\uptheta}{\mathrm{r}}{\mathrm{e}}^{\left(\uptheta\;\mathrm{T}+\mathrm{r}\mathrm{T}\right)}-\frac{\uptheta}{\mathrm{r}}{\mathrm{e}}^{\uptheta \mathrm{T}}-{\mathrm{e}}^{\uptheta \mathrm{T}}+1\right]=0\\ {}-\mathrm{A}+\left[\frac{\mathrm{hD}}{\uptheta \left(\uptheta +\mathrm{r}\right)}+\frac{\mathrm{CD}\mathrm{K}}{\uptheta}\right]\kern0.5em \left[\frac{\uptheta}{\mathrm{r}}{\mathrm{e}}^{\left(\uptheta\;\mathrm{T}+\mathrm{r}\mathrm{T}\right)}-\frac{\uptheta}{\mathrm{r}}{\mathrm{e}}^{\uptheta \mathrm{T}}-{\mathrm{e}}^{\uptheta \mathrm{T}}+1\right]=0\end{array} $$
$$ \begin{array}{l}\mathrm{A}=\left[\frac{\mathrm{hD}}{\uptheta \left(\uptheta +\mathrm{r}\right)}+\frac{\mathrm{CDK}}{\uptheta}\right]\kern0.5em \left[{\uptheta \mathrm{e}}^{\left(\uptheta\;\mathrm{T}+\mathrm{r}\mathrm{T}\right)}-{\uptheta \mathrm{e}}^{\uptheta \mathrm{T}}-{\mathrm{re}}^{\uptheta \mathrm{T}}+\mathrm{r}\right]\\ {}\mathrm{A}=\left[\frac{\mathrm{hD}}{\uptheta \left(\uptheta +\mathrm{r}\right)}+\frac{\mathrm{CDK}}{\uptheta}\right]\kern0.5em \left[{\uptheta \mathrm{e}}^{\left(\uptheta +\mathrm{r}\right)\mathrm{T}}-\left(\uptheta +\mathrm{r}\right){\mathrm{e}}^{\uptheta \mathrm{T}}+\mathrm{r}\right]\\ {}\mathrm{r}\mathrm{A}=\left[\frac{\mathrm{hD}}{\uptheta \left(\uptheta +\mathrm{r}\right)}+\frac{\mathrm{CDK}}{\uptheta}\right]\left[{\uptheta \mathrm{e}}^{\left(\uptheta +\mathrm{r}\right)\mathrm{T}}-\left(\uptheta +\mathrm{r}\right){\mathrm{e}}^{\uptheta \mathrm{T}}+\mathrm{r}\right]\end{array} $$
(1)
$$ \begin{array}{l}rA={K}^{\hbox{'}}\left[\theta {e}^{\left(\theta +r\right)T}-\left(\theta +r\right){e}^{\theta T}+r\right]\kern1em ,\kern1em \mathrm{Where}\kern0.5em k\hbox{'}=\left[\frac{hD}{\theta \left(\theta +r\right)}+\frac{CDK}{\theta}\right]\\ {}\mathrm{Using}\ \mathrm{Taylor}\hbox{'}\mathrm{s}\ \mathrm{s}\mathrm{eries}\ \mathrm{approximation},\ \mathrm{we}\ \mathrm{have}, \kern1.25em {e}^{rT}\approx 1+rT+\frac{(rT)^2}{2}\\ {}\mathrm{From}(1),\kern0.5em \mathrm{we}\kern0.75em \mathrm{get},\ \\ {}rA=k\hbox{'}\left\{\theta \kern0.5em \left(1+\left(\theta +r\right)T+\frac{{\left(\theta +r\right)}^2{T}^2}{2}\right)-\left(\theta +r\right)\kern0.5em \left(1+\theta T+\frac{\theta^2{T}^2}{2}\right)+r\right\}\\ {}rA=K\hbox{'}\kern0.5em \left(\frac{\theta {r}^2{T}^2}{2}+\frac{r{\theta}^2{T}^2}{2}\right)\\ {}2A=k\hbox{'}\theta \kern0.5em {T}^2\left(r+\theta \right)\\ {}{T}^2=\frac{2A}{k\hbox{'}\theta \kern0.5em \left(r+\theta \right)}\\ {}{T}^{*}=\sqrt{\begin{array}{l}\frac{2A}{k\hbox{'}\theta \left(r+\theta \right)}\\ {}\end{array}}\\ {}\mathrm{Also}\frac{d^2PV}{d{T}^2}\mathrm{found}\ \mathrm{t}\mathrm{o}\ \mathrm{be}\ \mathrm{positive}\end{array} $$

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Kumari, M., Pakkala, T.P.M. Inventory policy for deteriorating items under trade credit when time of payment is uncertain. OPSEARCH 53, 178–196 (2016). https://doi.org/10.1007/s12597-015-0223-8

Download citation

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s12597-015-0223-8

Keywords

Search

Navigation