Skip to main content
SpringerLink
Log in

Phase Portraits of a Class of Cubic Systems with an Ellipse and a Straight Line as Invariant Algebraic Curves

  • Original Research
  • Published:
Differential Equations and Dynamical Systems Aims and scope Submit manuscript

Abstract

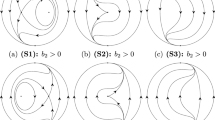
In this paper we classify the phase portraits in the Poincaré disc of a class of cubic polynomial differential systems having an invariant ellipse and an invariant straight line. We prove that such a class of cubic polynomial differential systems have exactly 43 topologically different phase portraits in the Poincaré disc. Also we obtain that the invariant ellipse in two of these phase portraits is a limit cycle.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16
Fig. 17
Fig. 18
Fig. 19
Fig. 20
Fig. 21
Fig. 22
Fig. 23
Fig. 24
Fig. 25
Fig. 26
Fig. 27
Fig. 28
Fig. 29
Fig. 30
Fig. 31
Fig. 32
Fig. 33

Similar content being viewed by others

References

  1. Alvarez, M.J., Ferragut, A., Jarque, X.: A survey on the blow up technique. Int. J. Bifurc. Chaos 21, 3103–3118 (2011)

    Article  MathSciNet  Google Scholar 

  2. Artés, J.C., Llibre, J.: Quadratic Hamiltonian vector fields. J. Differ. Equ. 107, 80–95 (1994)

    Article  MathSciNet  Google Scholar 

  3. Artés, J.C., Llibre, J., Schlomiuk, D., Vulpe, N.: Geometric configurations of singularities of planar polynomial differential systems. A global classification in the quadratic case. Birkhäuser, Basel (2021)

    Book  Google Scholar 

  4. Artés, J.C., Llibre, J., Valls, C.: Dynamics of the Higgins-Selkov and Selkov systems. Chaos, Solitons Fractals 114, 145–150 (2018)

    Article  MathSciNet  Google Scholar 

  5. Artés, J.C., Llibre, J., Vulpe, N.: Complete geometric invariant study of two classes of quadratic systems. Electron. J. Differ. Equ. 09, 1–35 (2012)

    MathSciNet  MATH  Google Scholar 

  6. Bautin, N.N.: On the number of limit cycles which appear with the variation of coefficients from an equilibrium position of focus or center type (Mat. Sbornik 30: 181-196). Amer. Math. Soc. Transl. 100(1954), 1–19 (1952)

    Google Scholar 

  7. Christopher, C.: Polynomial vector fields with prescribed algebraic limit cycle. Geom. Dedic. 88, 255–258 (2001)

    Article  MathSciNet  Google Scholar 

  8. Christopher, C., Llibre, J., Pantazi, C., Zhang, X.: Darboux integrability and invariant algebraic curves for planar polynomial systems. J. Phys. A 35, 2457–2476 (2002)

    Article  MathSciNet  Google Scholar 

  9. Dumortier, F.: Singularities of vector fields on the plane. J. Differ. Equ. 23, 53–106 (1977)

    Article  MathSciNet  Google Scholar 

  10. Dumortier, F., Llibre, J., Artés, J.C.: Qualitative theory of planar differential systems. Universitext. Springer-Verlag, New York (2006)

    MATH  Google Scholar 

  11. Gasull, A.: Polynomial systems with enough invariant algebraic curves. In: Proceedings of the special program at Nankai Institute of Mathematics, Tianjin, China, Nankai, series in pure applied Mathematical and Theoretical Physics, pp. 73–78. World Scientific, Singapore (1993)

    Google Scholar 

  12. Kalin, Y.F., Vulpe, N.I.: Affine-invariant conditions for the topological discrimination of quadratic Hamiltonian differential systems. Differ. Uravn. 34(3), 298–302 (1998)

    MathSciNet  Google Scholar 

  13. Kapteyn, W.: On the midpoints of integral curves of differential equations of the first degree. Nederl. Akad Wetensch. Verslag. Afd. Natuurk. Konikl. Nederland 19, 1446–1457 (1911). (Dutch)

    Google Scholar 

  14. Kapteyn, W.: New investigations on the midpoints of integrals of differential equations of the first degree. Nederl. Akad. Wetensch. Verslag Afd. Natuurk. 20, 1354–1365 (1912). (Dutch)

    Google Scholar 

  15. Llibre, J., Schlomiuk, D.: On the limit cycles bifurcating from an ellipse of a quadratic center. Discrete Contin. Dyn. Syst. Ser. A 35, 1091–1102 (2015)

    Article  MathSciNet  Google Scholar 

  16. Llibre, J., Valls, C.: Liouvillian and analytic integrability of the quadratic vector fields having an invariant ellipse. Acta Math. Sin. 30, 453–466 (2014)

    Article  MathSciNet  Google Scholar 

  17. Llibre, J., Yu, J.: Global phase portraits of quadratic systems with an ellipse and a straight line as invariant algebraic curves. Electron. J. Differ. Equ. 2015(314), 1–14 (2015)

    MathSciNet  MATH  Google Scholar 

  18. Markus, L.: Global structure of ordinary differential equations in the plane. Trans. Am. Math Soc. 76, 127–148 (1954)

    Article  MathSciNet  Google Scholar 

  19. Neumann, D.A.: Classification of continuous flows on 2-manifolds. Proc. Am. Math. Soc. 48, 73–81 (1975)

    Article  MathSciNet  Google Scholar 

  20. Oliveira, R.D.S., Rezende, A.C., Vulpe, N.: Family of quadratic differential systems with invariant hyperbolas: A complete classification in the space $^{12}$. Electron. J. Differ. Equ. 162, 50 (2016)

    MathSciNet  MATH  Google Scholar 

  21. Oliveira, R.D.S., Rezende, A.C., Schlomiuk, D., Vulpe, N.: Geometric and algebraic classification of quadratic differential systems with invariant hyperbolas. Electron. J. Differ. Equ. 295, 122 (2017)

    MathSciNet  MATH  Google Scholar 

  22. Oliveira, R.D.S., Rezende, A.C., Schlomiuk, D., Vulpe, N.: Characterization and bifurcation diagram of the family of quadratic differential systems with an invariant ellipse in terms of invariant polynomials. Rev. Mat. Complut. 2021, 1988–2807 (2021)

    Google Scholar 

  23. Peixoto, M.M.: Dynamical systems. Proccedings of a symposium held at the University of Bahia, pp. 389–420. Academic Press, New York (1973)

    Google Scholar 

  24. Reyn, J.W.: Phase portraits of planar quadratic systems. In: Mathematics and its applications, vol. 583, pp. 16–334. Springer, Berlin (2007)

    Google Scholar 

  25. Schlomiuk, D.: Algebraic particular integrals, integrability and the problem of the center. Trans. Am. Math. Soc. 338, 799–841 (1993)

    Article  MathSciNet  Google Scholar 

  26. Vulpe,N. I.: Affine-invariant conditions for the topological discrimination of quadratic systems with a center, (Russian) Differentsial’nye Uravneniya 19 (1983), no. 3, 371–379; translation in Differential Equations 19 (1983), 273–280

  27. Yang, L.: Recent advances on determining the number of real roots of parametric polynomials. J. Symb. Comput. 28, 225–242 (1999)

    Article  MathSciNet  Google Scholar 

  28. Ye, Y., et al.: Theory of limit cycles. In: Translation of mathematical monographs, vol. 66. American Mathematical Society, Providence (1984)

    Google Scholar 

  29. Zoladek, H.: Quadratic systems with center and their perturbations. J. Differ. Equ. 109, 223–273 (1994)

    Article  MathSciNet  Google Scholar 

Download references

Acknowledgements

We thank to the reviewers their comments which allow us to improve the results of this paper. The first author is partially supported by Iranian Ministry of Science, Research and Technology and Iran’s National Elites Foundation. The second autor is partially supported by the Ministerio de Ciencia, Innovación y Universidades, Agencia Estatal de Investigación grant PID2019-104658GB-I00, the Agència de Gestió d’Ajuts Universitaris i de Recerca grant 2017SGR1617, and the H2020 European Research Council grant MSCA-RISE-2017-777911.

Author information

Authors and Affiliations

  1. Department of Mathematical Sciences, Isfahan University of Technology, 84156-83111, Isfahan, Iran

    Ali Bakhshalizadeh

  2. Department de Matematiques, Universitat Autònoma de Barcelona, Bellaterra, 08193, Barcelona, Catalonia, Spain

    Jaume Llibre

Authors
  1. Ali Bakhshalizadeh
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Jaume Llibre
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Ali Bakhshalizadeh.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Bakhshalizadeh, A., Llibre, J. Phase Portraits of a Class of Cubic Systems with an Ellipse and a Straight Line as Invariant Algebraic Curves. Differ Equ Dyn Syst (2022). https://doi.org/10.1007/s12591-022-00597-9

Download citation

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s12591-022-00597-9

Keywords

Mathematics Subject Classification

Search

Navigation