Skip to main content
SpringerLink
Log in

On computing maximum allowable time delay of Lur’e systems with uncertain time-invariant delays

  • Regular Papers
  • Control Theory
  • Published:
International Journal of Control, Automation and Systems Aims and scope Submit manuscript

Abstract

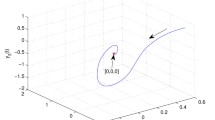
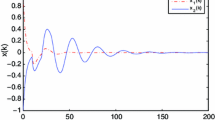
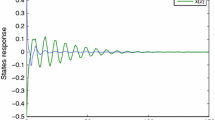
In this paper, we present an improved delay-dependent absolute stability criterion for Lur’e systems with time delays. The guarantee of absolute stability is provided by Lyapunov-Krasovskii theorem with the Lyapunov functional containing the integral of sector-bounded nonlinearities. The Lyapunov functional terms involving delay are partitioned to be associated with each equidistant fragment on the length of time delay. Employing the Jensen inequality and S-procedure, the sufficient condition is derived from time derivative of the Lyapunov functional. Then, the absolute stability criterion expressed in terms of linear matrix inequalities (LMIs) can be efficiently solved using available LMI solvers. The bisection method is used to determine the maximum allowable time delays to ensure the stability of Lur’e systems in the presence of uncertain time-invariant delays. In addition, the stability criterion is extended to Lur’e systems subject to norm-bounded uncertainties by using the matrix eliminating lemma. Numerical results from two benchmark problems show that the proposed criteria give significant improvement on the maximum allowable time delays.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. A. I. Lur’e and V. N. Postnikov, “On the theory of stability of control systems,” Applied Mathematics and Mechanics (in Russian), vol. 8, no. 3, pp. 246–248, 1944.

    MATH  Google Scholar 

  2. Q. C. Zhong, Robust Control of Time-delay Systems, Springer, 2006.

    MATH  Google Scholar 

  3. K. Gu, V. L. Kharitonov, and J. Chen, Stability of Time-delay Systems, Birkhauser, 2003.

    Book  MATH  Google Scholar 

  4. J. E. Normey-Rico and E. F. Camacho, Control of Dead-time Processes, Springer, 2007.

    Google Scholar 

  5. V. M. Popov and A. Halanay, “About stability of nonlinear controlled systems with delay,” Automation and Remote Control, vol. 23, no. 7, pp. 849–851, 1962.

    MathSciNet  Google Scholar 

  6. N. N. Krasovskii, Stability of Motion, (translation by J. Brenner), Stanford University Press, Stanford CA, USA, 1963.

    MATH  Google Scholar 

  7. A. Somolinos, “Stability of Lurie type functional equations,” Journal of Differential Equations, vol. 26, no. 2, pp. 191–199, 1977.

    Article  MATH  MathSciNet  Google Scholar 

  8. E. I. Verriest and W. Aggoune, “Stability of nonlinear differential delay systems,” Mathematics and Computers in Simulation, vol. 45, no. 3–4, pp. 257–267, 1998.

    Article  MATH  MathSciNet  Google Scholar 

  9. Z. Gan and W. Ge, “Lyapunov functional for multiple delay general Lurie systems with multiple non-linearities,” Journal of Mathematical Analysis and Applications, vol. 259, no. 2, pp. 596–608, 2001.

    Article  MATH  MathSciNet  Google Scholar 

  10. P. A. Bliman, “Lyapunov-Krasovskii functionals and frequency domain: delay-independent absolute stability criteria for delay systems,” International Journal of Robust and Nonlinear Control, vol. 11, no. 8, pp. 771–788, 2001.

    Article  MATH  MathSciNet  Google Scholar 

  11. Y. He and M. Wu, “Absolute stability for multiple delay general Lur’e control systems with multiple nonlinearities,” Journal of Computational and Applied Mathematics, vol. 159, no. 2, pp. 241–248, 2003.

    Article  MATH  MathSciNet  Google Scholar 

  12. E. Fridman, “New Lyapunov-Krasovskii functionals for stability of linear retarded and neutral type systems,” Systems & Control Letters, vol. 43, no. 9, pp. 309–319, 2001.

    Article  MATH  MathSciNet  Google Scholar 

  13. M. Wu, Y. He, and J. H. She, Stability Analysis and Robust Control of Time-delay Systems, Springer, 2010.

    Book  MATH  Google Scholar 

  14. P. Park, “A delay-dependent stability criterion for systems with uncertain time-invariant delays,” IEEE Trans. on Automatic Control, vol. 44, no. 4, pp. 876–877, April 1999.

    Article  MATH  Google Scholar 

  15. K. Gu and S. I. Niculescu, “Additional dynamics in transformed time-delay systems,” IEEE Trans. on Automatic Control, vol. 45, no. 3, pp. 572–575, March 2000.

    Article  MATH  MathSciNet  Google Scholar 

  16. K. Gu and S. I. Niculescu, “Further remarks on additional dynamics in various model transformations of linear delay systems,” IEEE Trans. on Automatic Control, vol. 46, no. 3, pp. 497–500, March 2001.

    Article  MATH  MathSciNet  Google Scholar 

  17. Y. S. Moon, P. Park, W. H. Kwon, and Y. S. Lee, “Delay-dependent robust stabilization of uncertain state-delayed systems,” International Journal of Control, vol. 74, no. 14, pp. 1447–1455, 2001.

    Article  MATH  MathSciNet  Google Scholar 

  18. L. Yu, Q. L. Han, S. Yu, and J. Gao, “Delaydependent conditions for robust absolute stability of uncertain time-delay systems,” Proc. of the 42nd IEEE Conference on Decision and Control, Maui, Hawaii, USA, pp. 6033–6037, 2003.

    Google Scholar 

  19. Y. He, M. Wu, J. H. She, and G. P. Liu, “Robust stability for delay Lur’e control systems with multiple nonlinearities,” Journal of Computational and Applied Mathematics, vol. 176, no. 2, pp. 371–380, 2005.

    Article  MATH  MathSciNet  Google Scholar 

  20. K. Gu, “A further refinement of discretized Lyapunov functional method for the stability of timedelay systems,” International Journal of Control, vol. 74, no. 10, pp. 967–976, 2001.

    Article  MATH  MathSciNet  Google Scholar 

  21. Q. L. Han, “Absolute stability of time-delay systems with sector-bounded nonlinearity,” Automatica, vol. 41, no. 12, pp. 2171–2176, 2005.

    Article  Google Scholar 

  22. D. Zhang and L. Yu, “Equivalence of some stability criteria for linear time-delay systems,” Applied Mathematics and Computation, vol. 202, no. 1, pp. 395–400, 2008.

    Article  MATH  MathSciNet  Google Scholar 

  23. S. Xu and G. Feng, “Improved robust absolute stability criteria for uncertain time-delay systems,” IET Control Theory & Applications, vol. 1, no. 6, pp. 1630–1637, 2007.

    Article  Google Scholar 

  24. K. Gu, “Discretized LMI set in the stability problem for linear uncertain time-delay systems,” International Journal of Control, vol. 68, no. 4, pp. 923–934, 1997.

    Article  MATH  MathSciNet  Google Scholar 

  25. F. Gouaisbaut and D. Peaucelle, “Delay-dependent robust stability of time delay systems,” Proc. of the 5th IFAC Symposium on Robust Control Design, Toulouse, France, pp. 453–458, 2006.

    Google Scholar 

  26. G. S. Park, H. L. Choi, and J. T. Lim, “On stability of linear time-delay systems with multiple delays,” International Journal of Systems Science, vol. 39, no. 8, pp. 839–852, 2008.

    Article  MATH  MathSciNet  Google Scholar 

  27. M. Wu, Z. Y. Feng, and Y. He, “Improved delaydependent absolute stability of Lur’e systems with time-delay,” International Journal of Control, Automation, and Systems, vol. 7, no. 6, pp. 1009–1014, 2009.

    Article  Google Scholar 

  28. Q. L. Han, “A delay decomposition approach to stability of linear neutral system,” Proc. of the 17th IFAC World Congress, Seoul, Korea, pp. 2607–2612, 2008.

    Google Scholar 

  29. F. Qiu, B. Cui, and Y. Ji, “Delay-dividing approach for absolute stability of Lurie control system with mixed delays,” Nonlinear Analysis: Real World Applications, vol. 11, no. 4, pp. 3110–3120, 2010.

    Article  MATH  MathSciNet  Google Scholar 

  30. C. Briat, “Convergence and equivalence results for the Jensen’s inequality-application to time-delay and sampled-data systems,” IEEE Trans. on Automatic Control, vol. 56, no. 7, pp. 1660–1665, July 2011.

    Article  MathSciNet  Google Scholar 

  31. L. Xie, “Output feedback H∞ control of systems with parameter uncertainty,” International Journal of Control, vol. 63, no. 4, pp. 741–750, 1996.

    Article  MATH  MathSciNet  Google Scholar 

  32. V. A. Yakubovich, “The S-procedure in non-linear control theory,” Vestnik, Leningrad University: Mathematics, vol. 4, no. 1, pp. 73–93, 1977 (in Russian, 1971).

    MathSciNet  Google Scholar 

  33. S. Boyd, L. El Ghaoui, E. Feron, and V. Balakrishnan, Linear Matrix Inequalities in System and Control Theory, vol. 15 of Studies in Applied Mathematics, SIAM, Philadelphia, PA, 1994.

    Google Scholar 

  34. Y. Nesterov and A. Nemirovski, Interior-point Polynomial Methods in Convex Programming, vol. 13 of Studies in Applied Mathematics, SIAM, Philadelphia, PA, 1994.

    Google Scholar 

  35. P. Gahinet and A. Nemirovski, “The projective method for solving linear matrix inequalities,” Mathematical Programming, vol. 77, no. 1, pp. 163–190, 1997.

    Article  MATH  MathSciNet  Google Scholar 

  36. F. Qiu and Q. Zhang, “Absolute stability analysis of Lurie control system with multiple delays: an integral-equality approach,” Nonlinear Analysis: Real World Applications, vol. 12, no. 3, pp. 1475–1484, 2011.

    Article  MATH  MathSciNet  Google Scholar 

  37. C. A. Desoer and M. Vidyasagar, Feedback Systems: Input-output Properties, Academic Press, New York, 1975.

    MATH  Google Scholar 

  38. P. Gahinet, A. Nemirovski, A. J. Laub, and M. Chilali, LMI Control Toolbox, Math Works, MA, 1995.

    Google Scholar 

  39. J. F. Gao, H. Y. Su, X. F. Ji, and J. Chu, “New delay-dependent absolute stability criteria for Lurie control systems,” Acta Automatica Sinica, vol. 34, no. 10, pp. 1275–1280, 2008.

    Article  MATH  MathSciNet  Google Scholar 

  40. A. Kazemy and M. Farrokhi, “Robust absolute stability analysis of multiple time-delay Lur’e systems with parametric uncertainties,” Asian Journal of Control, vol. 15, no. 1, pp. 203–213, 2013.

    Article  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Electrical Engineering, Faculty of Engineering, Chulalongkorn University, 254 Phayathai Road, Pathumwan, Bangkok, 10330, Thailand

    Thapana Nampradit & David Banjerdpongchai

Authors
  1. Thapana Nampradit
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. David Banjerdpongchai
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to David Banjerdpongchai.

Additional information

Recommended by Associate Editor Soohee Han under the direction of Editor Hyungbo Shim.

This work was supported by Thailand Research Fund through the Royal Golden Jubilee Ph.D. Program under Grant No. PHD/0148/2003.

Thapana Nampradit received his B.Eng. degree in Control Engineering from King Mongkut’s Institute of Technology Ladkrabang in 1998, and his M.Eng. degree in Electrical Engineering from Chulalongkorn University in 2002. He is currently a Ph.D. candidate at the Department of Electrical Engineering, Chulalongkorn University. His research interests include robust control, numerical optimization, and computer-aided control systems design.

David Banjerdpongchai received his B.Eng. degree (First class honors) from Chulalongkorn University, and his M.S. and Ph.D. degrees from Stanford University, all in Electrical Engineering, in 1990, 1993, and 1997, respectively. Since 1990, he has been with the department of Electrical Engineering, Faculty of Engineering, Chulalongkorn University. Currently, he is a professor of Electrical Engineering and head of department. He is a senior member of IEEE and has served as a chair of automatic control of ECTI Association since 2010. His research interests are energy management systems, analysis and synthesis of robust control systems, and convex optimization in robust control problems.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Nampradit, T., Banjerdpongchai, D. On computing maximum allowable time delay of Lur’e systems with uncertain time-invariant delays. Int. J. Control Autom. Syst. 12, 497–506 (2014). https://doi.org/10.1007/s12555-013-0433-x

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s12555-013-0433-x

Keywords

Search

Navigation