Abstract
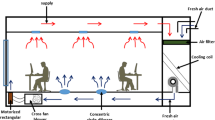
The air temperature distribution in a space with reduced diffuser flow rates and heat loads was studied using simulation. Computational fluid dynamics (CFD) was used to analyze the room air distribution from a side wall diffuser at the design flow rate, and the results were validated with experimental data. CFD was used to predict occupant discomfort under a range of reduced diffuser flow rates. It was found for diffuser flow rates above 30% of the design flow rate that the temperature influence from the jet was minimal. At these flow rates, there was nearly a uniform temperature distribution in the occupied zone. The predicted maximum value of percentage of dissatisfied occupants within the space began to increase for diffuser flow rates below 30% of the design flow rate. The percent dissatisfaction at 1 m room height was greater than 25% for the lowest diffuser flow rate tested (15% of the design flow rate) directly under the diffuser, which was the highest of the test cases, but was 5% or less throughout more than 90% of the room. In contrast, at the higher flow rates, the percent dissatisfied index was 5% or less in only 60%–80% of the room due to increased velocity. Evidence of dumping was already found at the traditional minimum flow rate setting of 30% of design, and so there would be little harm in reducing the minimum flow rate further. Reducing the flow rate below 30% of design just moved the location of the dumping closer to the diffuser. For very low diffuser flow rates (below 30% of the design flow rate), it is recommended that desks be placed away from the supply diffuser to avoid discomfort. Overall, the simulation results indicate that uniform temperatures are maintained in the room at flow rates as low as 15% of design except immediately under the diffuser. This suggests that the VAV minimum flow rates can be set below 30% of design flow as long as the diffuser is at least 1 m from an occupant’s position.
Similar content being viewed by others
References
ANSYS (2013). ANSYS Mechanical User’s Guide.
Arens E, Zhang H, Hoyt T, Kaam S, Goins J, Bauman F, Zhai Y, Webster T, West B, Paliaga G, Stein J, Seidl R, Tully B, Rimmer J, Toftum J (2012). Thermal and air quality acceptability in buildings that reduce energy by reducing minimum airflow from overhead diffusers. ASHRAE RP-1515.
Arens E, Xu T, Miura K, Hui Z, Fountain M, Bauman F (1998). A study of occupant cooling by personally controlled air movement. Energy and Buildings, 27: 45–59.
ASHRAE (2013). Thermal environmental conditions for human occupancy. ANSI/ASHRAE Standard 55-2013.
Cândido C, de Dear RJ, Lamberts R, Bittencourt L (2010). Air movement acceptability limits and thermal comfort in Brazil’s hot humid climate zone. Building and Environment, 45: 222–229.
Carlucci S, Pagliano L (2012). A review of indices for the long-term evaluation of the general thermal comfort conditions in buildings. Energy and Buildings, 53: 194–205.
Charles KE (2003). Fanger’s thermal comfort and draught models. National Research Council of Canada, IRC Research Report RR-162.
Chen Q (1995). Comparison of different k–e models for indoor airflow computations. Numerical Heat Transfer, Part B: Fundamentals, 28: 353–369.
Chen Q, Moser A (1991). Simulation of a multiple-nozzle diffuser. In: Proceedings of 12th AIVC Conference, Ottawa, Canada, pp. 1–14.
Cheng Y, Niu J, Gao N (2012). Thermal comfort models: A review and numerical investigation. Building and Environment, 47: 13–22.
Cheong KWD, Djunaedy E, Chua YL, Tham KW, Sekhar SC, Wong NH, Ullah MB (2003). Thermal comfort study of an air-conditioned lecture theatre in the tropics. Building and Environment, 38: 63–73.
De Dear RJ, Akimoto T, Arens E, Brager G, Candido C, Cheong KWD, Li B, Nishihara N, Sekhar SC, Tanabe S, Toftum J, Zhang H, Zhu Y (2013). Progress in thermal comfort research over the last twenty years. Indoor Air, 23: 442–461.
Djongyang N, Tchinda R, Njomo D (2010). Thermal comfort: A review paper. Renewable and Sustainable Energy Reviews, 14: 2626–2640.
Ewert M, Renz U, Vogl N, Zeller M (1991). Definition of the flow parameters at the room inlet devices-measurements and calculations. In: Proceedings of 12th AIVC Conference, Ottawa, Canada, pp. 231–237.
Fanger P, Melikov A, Hanzawa H, Ring J (1988). Air turbulence and sensation of draft. Energy and Buildings, 12: 21–39.
Fanger P, Christensen N (1986). Perception of draught in ventilated spaces. Ergonomics, 29: 215–235.
Fountain M, Bauman F, Arens E, Miura K, de Dear R (1994). Locally controlled air movement preferred in warm isothermal environments. ASHRAE Transactions, 100(2): 937–952.
Gosman A, Nielsen P, Restivo A, Whitelaw J (1980). The flow properties of rooms with small ventilation openings. Journal of Fluids Engineering, 102: 316–323.
Heikkinen J (1991a). Modeling of a supply air terminal for room airflow simulation. In: Proceedings of 12th AIVC Conference, Ottawa, Canada, pp. 213–230.
Heikkinen J (1991b). Measurements of test cases B2, B3, E2, E3 (isothermal and summer cooling cases). IEA Annex 20 Research item 1.16 and 1.17.
Huo Y, Zhang J, Shaw C, Haghighat F (1996). A new method to describe boundary conditions in CFD simulation. In: Proceedings of International Conference on Air Distribution in Rooms (ROOMVENT), Yokohama, Japan, pp. 233–240.
Kajiya R, Hiruta K, Sakai K, Ono H, Sudo T (2011). Thermal environment prediction using CFD with a virtual mannequin model and experiment with subject in a floor heating room. In: Proceedings of 12th International IBPSA Building Simulation Conference, Sydney, Australia, pp. 1670–1677.
Kubo H, Isoda N, Enomoto-Koshimizu H (1997). Cooling effects of preferred air velocity in muggy conditions. Building and Environment, 32: 211–218.
Kwong QJ, Adam NM, Sahari BB (2014). Thermal comfort assessment and potential for energy efficiency enhancement in modern tropical buildings: A review. Energy and Buildings, 68: 547–557.
Lemaire AD, Chen Q, Ewert M, Heikkinen J, Inard C, Moser A, Nielsen PV, Whittle G (1993). Room air and contaminant flow, evaluation of computational methods. Subtask-1 Summary Report.
Luo S, Heikkinen J, Roux B (2004). Simulation of airflow in the IEA annex 20 test room—Validation of a simplified model for the nozzle diffuser in isothermal test cases. Building and Environment, 39: 1403–1415.
Mendell MJ, Mirer AG (2009). Indoor thermal factors and symptoms in office workers: Findings from the US EPA BASE study. Indoor Air, 19: 291–302.
Nielsen PV (1992). The description of supply openings in numerical models for room air distribution. ASHRAE Transactions, 98(1): 963–971.
Nielsen PV (1989). Representation of boundary conditions at supply openings. IEA Annex 20 Research Item No. 1.11.
Noh K, Jang J, Oh M (2007). Thermal comfort and indoor air quality in the lecture room with 4-way cassette air-conditioner and mixing ventilation system. Building and Environment, 42: 689–698.
Riachi Y, Clodic D (2014). A numerical model for simulating thermal comfort prediction in public transportation buses. International Journal of Environmental Protection and Policy, 2: 1–8.
Rupp RF, Vásquez NG, Lamberts R (2015). A review of human thermal comfort in the built environment. Energy and Buildings, 105: 178–205.
Simone A, Olesen BW, Stoops JL, Watkins AW (2013). Thermal comfort in commercial kitchens (RP-1469): Procedure and physical measurements (part 1). HVAC&R Research, 19: 1001–1015.
Skovgaard M, Nielsen P (1991). Modeling complex inlet geometries in CFD applied to airflow in ventilated rooms. In: Proceedings of 12th AIVC Conference, Ottawa, Canada, pp. 183–200.
Srebric J, Chen Q (2002). Simplified numerical models for complex air supply diffusers. HVAC&R Research, 8: 277–294.
Srebric J, Chen Q (2001). A method of test to obtain diffuser data for CFD modeling of room airflow (RP-1009). ASHRAE Transactions, 107(2): 108–116.
Srebric J (2000). Simplified methodology for indoor environment design. PhD Dissertation, Massachusetts Institute of Technology, USA.
Stamou AI, Katsiris I, Schaelin A (2008). Evaluation of thermal comfort in Galatsi arena of the Olympics “Athens 2004” using a CFD model. Applied Thermal Engineering, 28: 1206–1215.
Svidt K (1994). Investigation of inlet boundary conditions for numerical prediction of airflow in livestock buildings. Aalborg University, Indoor Environmental Technology, No. 38. Vol. R9407.
Taleghani M, Tenpierik M, Kurvers S, van den Dobbelsteen A (2013). A review into thermal comfort in buildings. Renewable and Sustainable Energy Reviews, 26: 201–215.
Yang L, Yan H, Lam JC (2014). Thermal comfort and building energy consumption implications—A review. Applied Energy, 115: 164–173.
Zhang H, Arens E, Fard SA, Huizenga C, Paliaga G, Brager G, Zagreus L (2007). Air movement preferences observed in office buildings. International Journal of Biometeorology, 51: 349–360.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Gangisetti, K., Claridge, D.E., Srebric, J. et al. Influence of reduced VAV flow settings on indoor thermal comfort in an office space. Build. Simul. 9, 101–111 (2016). https://doi.org/10.1007/s12273-015-0254-3
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s12273-015-0254-3