Skip to main content
SpringerLink
Log in

Vibrational behavior of MDOF oscillators subjected to multiple contact constraints

  • Published:
Journal of Mechanical Science and Technology Aims and scope Submit manuscript

Abstract

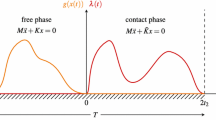
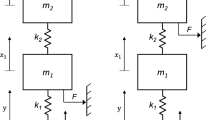
Vibrational behavior of harmonically excited MDOF oscillators subjected to multiple contact constraints is investigated in this paper using the combination of the Newmark integration scheme and the Linear complementarity problem (LCP) formulation. An oscillator with gap-activated non-smooth spring constraints exhibits various complex behavior such as sub-harmonic resonances, bifurcations and chaos, which are effectively predicted using the proposed method. Numerical results were obtained and presented for SDOF and 5-DOF systems with frequency and stiffness parameters varying in wide ranges to validate the Newmark-LCP method and to demonstrate its effectiveness in dealing with MDOF systems with multiple contact constraints.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. B. C. Wen, Y. N. Li, Y. M. Zhang and Z. W. Song, Vibration utilization engineering, Science Publisher, Beijing, China (2005).

    Google Scholar 

  2. S. W. Shaw, The dynamics of a harmonically excited system having rigid amplitude constraints, part 1: subharmonic motions and local bifurcations, Journal of Applied Mechanics, 52 (1985) 453–458.

    Article  MathSciNet  Google Scholar 

  3. J. S. Walker and T. Soule, Chaos in a simple impact oscillator: The bender bouncer, American Journal of Physics, 64 (4) (1996) 397–409.

    Article  Google Scholar 

  4. S. W. Shaw and P. J. Holmes, A periodically forced piecewise linear oscillator, Journal of Sound and Vibration, 90 (1983) 129–155.

    Article  MathSciNet  MATH  Google Scholar 

  5. G. S. Whiston, The vibro-impact response of a harmonically excited and preloaded one-dimensional linear oscillator, Journal of Sound and Vibration, 115 (1987) 303–319.

    Article  MathSciNet  MATH  Google Scholar 

  6. A. V. Dyskin, E. Pasternak and E. Pelinovsky, Periodic motions and resonances of impact oscillators, Journal of Sound and Vibration, 331 (12) (2012) 2856–2873.

    Article  Google Scholar 

  7. M. Wiercigroch, Modelling of dynamical systems with motion dependent discontinuities, Chaos Solutions and Fractals, 11 (2000) 2429–2442.

    Article  MathSciNet  MATH  Google Scholar 

  8. V. W. T. Sin and M. Wiercigroch, A symmetrically piecewise linear oscillator: Design and measurement, Proceedings of the Institution of Mechanical Engineers, Part C: Journal of Mechanical Engineering Science, 213 (3) (1999) 241–249.

    Google Scholar 

  9. P. Ing, E. Pavlovskaia, M. Wiercigroch and S. Banerjee, Experimental study of impact oscillator with one-sided elastic constraint, Philosophical Transactions of the Royal Society A: Mathematical, Physical and Engineering Sciences, 366 (2008) 679–704.

    Article  MATH  Google Scholar 

  10. Z. K. Peng, Z. Q. Lang, S. A. Billings and Y. Ku, Analysis of bilinear oscillators under harmonic loading using nonlinear output frequency response functions, International Journal of Mechanical Sciences, 49 (2007) 1213–1225.

    Article  Google Scholar 

  11. G. W. Luo, Period-doubling bifurcations and routes to chaos of the vibratory systems contacting stops, Physics Letters A, 323 (2004) 210–217.

    Article  MathSciNet  MATH  Google Scholar 

  12. G. W. Luo and Y. Zhang, Analyses of impact motions of harmonically excited systems having rigid amplitude constraints, International Journal of Impact Engineering, 34 (11) (2007) 1883–1905.

    Article  Google Scholar 

  13. S. H. Doole and S. J. Hogan, A piecewise linear suspension bridge model: nonlinear dynamics and orbit continuation, International Journal of Impact Engineering, 11 (1996) 19–47.

    MathSciNet  MATH  Google Scholar 

  14. S. D. Yu, An efficient computational method for vibration analysis of unsymmetric piecewise-linear dynamical systems with multiple degrees of freedom, Nonlinear Dynamics, 71 (3) (2013) 493–504.

    Article  MathSciNet  Google Scholar 

  15. M. Fadaee and S. D. Yu, Two-dimensional stick-slip motion of Coulomb friction oscillators, Proceedings of the Institution of Mechanical Engineers, Part C: Journal of Mechanical Engineering Science (2015).

    Google Scholar 

  16. A. B. Nordmark, Non-periodic motion caused by grazing incidence in impact oscillators, Journal of Sound and Vibration, 145 (2) (1991) 279–297.

    Article  Google Scholar 

  17. F. Peterka, Laws of impact motion of mechanical system with one degree of freedom, Part I-theoretical analysis of nmultiple (1/n)-impact motion, Acta Technica CSAV, 4 (1974) 462–473.

    MATH  Google Scholar 

  18. F. Peterka, T. Kotera and S. Cipera, Explanation of appearance and characteristics of intermittency chaos of the impact oscillator, Chaos, Solitons and Fractals, 19 (5) (2004) 1251–1259.

    Article  MATH  Google Scholar 

  19. S. S. Rao, Mechanical vibrations, 5th ed., Pearson Prentice Hall, Upper Saddle River, New Jersey, USA (2010).

Download references

Author information

Authors and Affiliations

  1. Department of Mechanical and Industrial Engineering, Ryerson University, Toronto, Ontario, M5B 2K3, Canada

    Mo Fadaee & Shudong Yu

Authors
  1. Mo Fadaee
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Shudong Yu
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Shudong Yu.

Additional information

Recommended by Associate Editor Junhong Park

Mo Fadaee is a Ph.D. candidate in Mechanical and Industrial Engineering Dept. at Ryerson University, Toronto, Canada. His Ph.D. thesis deals with the flow-induced vibration and frictional contact in CANDU fuel string. His research areas are nonlinear dynamics, chaos, contact and friction.

Shudong Yu obtained his Ph.D. in Mechanical Engineering from the University of Toronto in 1995. He is a Professor of Mechanical Engineering at Ryerson University, Toronto, Canada. His research interests include nuclear fuel design and modeling, non-linear vibrations, frictional contact, multi-body dynamics, and flow-induced vibration.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Fadaee, M., Yu, S. Vibrational behavior of MDOF oscillators subjected to multiple contact constraints. J Mech Sci Technol 31, 1551–1560 (2017). https://doi.org/10.1007/s12206-017-0302-2

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s12206-017-0302-2

Keywords

Search

Navigation