Skip to main content
SpringerLink
Log in

A saddle point characterization of efficient solutions for interval optimization problems

  • Original Research
  • Published:
Journal of Applied Mathematics and Computing Aims and scope Submit manuscript

Abstract

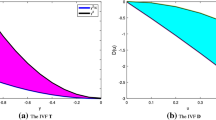
In this article, we attempt to characterize efficient solutions of constrained interval optimization problems. Towards this aim, at first, we study a scalarization characterization to capture efficient solutions. Then, with the help of saddle point of a newly introduced Lagrangian function, we investigate efficient solutions of an interval optimization problem. Several parts of the results are supported with numerical and pictorial illustration.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4

Similar content being viewed by others

References

  1. Bhurjee, A.K., Panda, G.: Efficient solution of interval optimization problems. Math. Methods Oper. Res. 76(3), 273–288 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  2. Chakraborty, D., Ghosh, D.: Analytical fuzzy plane geometry II. Fuzzy Sets Syst. 243, 84–10 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  3. Chalco-Cano, Y., Lodwick, W.A., Rufian-Lizana, A.: Optimality conditions of type KKT for optimization problem with interval-valued objective function via generalized derivative. Fuzzy Optim. Decis. Mak. 12(3), 305–322 (2013)

    Article  MathSciNet  Google Scholar 

  4. Chen, S.H., Wu, J., Chen, Y.D.: Interval optimization for uncertain structures. Finite Elem. Anal. Des. 40, 1379–1398 (2004)

    Article  Google Scholar 

  5. Cheng, J., Liu, Z., Wu, Z., Tang, M., Tan, J.: Direct optimization of uncertain structures based on degree of interval constraint violation. Comput. Struct. 164, 83–94 (2016)

    Article  Google Scholar 

  6. Chalco-Cano, Y., Rufian-Lizana, A., Roman-Flores, H., Jimenez-Gamero, M.D.: Calculus for interval-valued functions using generalized Hukuhara derivative and applications. Fuzzy Sets Syst. 219, 49–67 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  7. Frits, E.R., Rev, E., Lelkes, Z., Markot, M., Csendes, T.: Application of an interval optimization method for studying feasibility of batch extractive distillation. In: Proceedings of the International Workshop on Global Optimization, Almeria, pp. 103–108 (2005)

  8. Ghosh, D.: Newton method to obtain efficient solutions of the optimization problems with interval-valued objective functions. J. Appl. Math. Comput. 53, 709–731 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  9. Ghosh, D.: A Newton method for capturing efficient solutions of interval optimization problems. Opesearch 53, 648–665 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  10. Ghosh, D., Chakraborty, D.: Analytical fuzzy plane geometry I. Fuzzy Sets Syst. 209, 66–83 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  11. Ghosh, D., Chakraborty, D.: Analytical fuzzy plane geometry III. Fuzzy Sets Syst. 283, 83–107 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  12. Ghosh, D., Chakraborty, D.: A method for capturing the entire fuzzy non-dominated set of a fuzzy multi-criteria optimization problem. J. Intell. Fuzzy Syst. 26, 1223–1234 (2014)

    MATH  Google Scholar 

  13. Ghosh, D., Chakraborty, D.: A new Pareto set generating method for multi-criteria optimization problems. Oper. Res. Lett. 42, 514–521 (2014)

    Article  MathSciNet  Google Scholar 

  14. Ghosh, D., Chakraborty, D.: A method for capturing the entire fuzzy non-dominated set of a fuzzy multi-criteria optimization problem. Fuzzy Sets Syst. 272, 1–29 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  15. Ghosh, D., Chakraborty, D.: Quadratic interpolation technique to minimize univariable fuzzy functions. Int. J. Appl. Comput. Math. 3(2), 527–547 (2017)

    Article  MathSciNet  Google Scholar 

  16. Hong, F.-X., Li, D.-F.: Nonlinear programming method for interval-valued \(n\)-person cooperative games. Oper. Res. Int. J. 17(2), 479–497 (2017)

    Article  MathSciNet  Google Scholar 

  17. Kumar, P.: Inventory model with price-dependent demand rate and no shortages: an interval-valued linear fractional programming approach. Oper. Res. Appl. Int. J. 2(4), 1–14 (2015)

    Google Scholar 

  18. Oliveria, C., Antunes, C.H.: Multiple objective linear programming models with interval coefficients-an illustrated review. Eur. J. Oper. Res. 181, 1434–1463 (2007)

    Article  MATH  Google Scholar 

  19. Singh, D., Dar, B.A., Goyal, A.: KKT optimality conditions for interval-valued optimization problems. J. Nonlinear Anal. Optim. 5(2), 91–103 (2014)

    MathSciNet  Google Scholar 

  20. Wang, H., Zhang, R.: Optimality conditions and duality for arcwise connected interval optimization problems. Opsearch 52(4), 870–883 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  21. Wu, H.C.: The Karush–Kuhn–Tucker optimality conditions in an optimization problem with interval-valued objective function. Eur. J. Oper. Res. 176(1), 46–59 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  22. Wu, H.C.: Dulity theory for optimization problems with interval-valued objective fuction. J. Optim. Theory Appl. 144(3), 615–628 (2010)

    Article  MathSciNet  Google Scholar 

  23. Wu, H.C.: Duality theory in interval-valued linear programming problems. J. Optim. Theory Appl. 150, 298–316 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  24. Ying, Z.-Y., Xi, Y.-G., Zhang, Z.-J.: Test on reachability of a Robot to an object. In: The Proceedings of IEEE International Conference on Robotics and Automation, IEEE Xplore (2002)

  25. Zhang, J., Liu, S., Li, L., Feng, Q.: The KKT optimality conditions in a class of generalized convex optimization problems with an interval-valued objective function. Optim. Lett. 8(2), 607–631 (2012)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

Debdas Ghosh gratefully acknowledges the financial support of Early Career Research Award (ECR/2015/000467), Science and Engineering Research Board, Government of India. The authors are thankful to the anonymous reviewers’ suggestion to improve the perfection of the article.

Author information

Authors and Affiliations

  1. Department of Mathematics, Indian Institute of Technology Kharagpur, Kharagpur, India

    Debdulal Ghosh, Sushil Kumar Bhuiya & Lakshmi Kanta Patra

  2. Department of Mathematical Sciences, Indian Institute of Technology (BHU), Varanasi, Uttar Pradesh, 221005, India

    Debdas Ghosh

Authors
  1. Debdulal Ghosh
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Debdas Ghosh
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Sushil Kumar Bhuiya
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Lakshmi Kanta Patra
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Debdas Ghosh.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Ghosh, D., Ghosh, D., Bhuiya, S.K. et al. A saddle point characterization of efficient solutions for interval optimization problems. J. Appl. Math. Comput. 58, 193–217 (2018). https://doi.org/10.1007/s12190-017-1140-1

Download citation

  • Received:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s12190-017-1140-1

Keywords

Mathematics Subject Classification

Search

Navigation