Introduction

Metapopulation dynamics, in which local populations connected through dispersal persist through frequent extinction and colonization events, have been demonstrated in a wide variety of taxa including insects specializing on patchily distributed host plants (Hanski and Gilpin 1991), amphibians and other aquatic species inhabiting spatially discrete wetlands (Marsh and Trenham 2001), or species limited by availability of colonial breeding sites including bats (Hallam and McCracken 2011). Metapopulation dynamics can also result from habitat fragmentation and degradation (Opdam 1991; Hanski and Ovaskainen 2000). Human activities such as agriculture and urbanization can produce a network of fragmented, low-quality habitat patches across a landscape (Fahrig 2003). Reductions in breeding habitat and inter-patch dispersal can result in loss of genetic diversity and elevated local extinction risk (Wauters et al. 1994; Thomas 2000), threatening metapopulation persistence (Hanski 1991).

As conservation resources are often limited, cost-effective management of patchily distributed populations has benefited from insights provided by theory (Doak and Mills 1994). Consideration of classic (Levins 1969) and spatially realistic (Hanski 1994) metapopulation models suggests persistence can be enhanced by landscape-scale efforts to increase connectivity, such as by creating corridors between isolated patches, and to prioritize conservation of large, well-connected patches (Corlatti et al. 2009). At local scales, improving patch quality by enhancing food or breeding site availability or by removing predators and competitors can increase metapopulation persistence by enhancing local fecundity and survival and by disproportionally attracting immigrants, therefore reducing extinction risk (Pulliam 1988; Moilanen and Hanski 1998; Mortelliti et al. 2010). Improving patch quality is especially appealing as a management option when the number, size, and spatial configuration of patches are fixed, as in urbanized landscapes or networks of wetlands connected by flood events.

Patch improvement through the provisioning of spatially and temporally predictable food resources is a frequently used management strategy that has enhanced breeding success and survival in imperiled and range-restricted wildlife (Gonzalez et al. 2006; Angerbjörn et al. 2013), including a wide variety of species exhibiting metapopulation structure (examples are listed in Appendix A). Similarly, resource subsidies provided recreationally or unintentionally in urban and agricultural habitat, such as bird feeders and crop fields, can elevate local population densities (Robb et al. 2008; Galbraith et al. 2015). Yet this resource improvement can have negative consequences, including skewed sex ratios and increased predation (Clout et al. 2002; Cortés-Avizanda et al. 2009). Greater resource availability can also facilitate local pathogen transmission by aggregating hosts and amplifying pathogen replication and shedding (Gompper and Wright 2005; Vale et al. 2011). Resource provisioning has consequently been implicated in the emergence of pathogens such as Trichomonas gallinae and subsequent population declines (Robinson et al. 2010).

Management or unintentional actions that improve connectivity and patch quality could likewise influence the spread of pathogens through fragmented landscapes. A body of theory based on extending the Levins model (Levins 1969) suggests that increasing inter-patch colonization rates can increase risk of pathogen invasion (Hess 1996; Gog et al. 2002; McCallum and Dobson 2002). A more detailed model simulating viral dynamics in flying foxes suggested that increased resource availability in urban habitat results in decreased connectivity of bat colonies, reducing outbreak frequency across the landscape but in turn increasing the size of local outbreaks due to loss of herd immunity in isolated subpopulations (Plowright et al. 2011).

Although existing theory elucidates how connectivity influences pathogen spread in patchily distributed landscapes, the role of heterogeneity in habitat quality in promoting or limiting pathogen spread is poorly understood. This is particularly important for guiding conservation or recreational practices related to resource improvement and supplemental feeding in pathogen-susceptible or imperiled metapopulations. Here, we develop a simple metapopulation model to explore how patch occupancy, pathogen invasion, and infection prevalence are influenced by resource improvement at two spatial scales: local improvement to patch quality and the landscape-level deployment of patch improvement across the metapopulation. Contrary to expectation, we find a non-monotonic relationship between equilibrium occupancy and the number and quality of provisioned patches, such that habitat improvement instigated at all patches can result in lower occupancy than when a mixture of low- and high-quality patches is maintained. In addition to informing conservation and management strategies based in resource improvement, these findings suggest more generally that low-quality habitat patches can inhibit pathogen spread across landscapes if they act as sinks for infected subpopulations.

Model and Methods

Resource improvement and patch quality

To describe the dynamics of a metapopulation subject to improvement in patch quality, we extend the Levins model describing changes in the fraction of occupied patches resulting from colonization and extinction (Levins 1969; Hanski 1991) to include two patch types: low-quality (unprovisioned) and high-quality (provisioned) habitat, where N L and N H denote the respective proportions (not absolute numbers) of occupied patches. Effects of resource improvement are reflected in two model parameters. The parameter f represents the fraction of patches provisioned, while the parameter q represents the improvement in habitat quality, defined as the proportionate increase in expected occupancy time in provisioned versus unprovisioned patches (i.e., q = x L /x H , where x L and x H are the extinction rates of low- and high-quality patches). Since these changes focus on improving local habitat quality, for simplicity we assume the colonization rate (c) from any occupied patch to an empty patch is the same for provisioned and unprovisioned patches. The total colonization rate for low-quality patches is the product of the species-specific colonization rate (c), the fraction of empty low-quality patches (the difference between the total fraction of low-quality patches, 1 − f, and the fraction currently occupied, N L ), and the fraction of low- and high-quality patches currently occupied, N H  + N L ; a similar expression is readily derived for the colonization of high-quality patches. Therefore, the metapopulation dynamics can be described by the following differential equations:

$$ d{N}_L/dt=c\left(1-f-{N}_L\right)\left({N}_H+{N}_L\right)-{x}_L{N}_L $$
(1a)
$$ d{N}_H/dt=c\left(f-{N}_H\right)\left({N}_H+{N}_L\right)-\left({x}_L/q\right){N}_H $$
(1b)

Pathogen dynamics

Following (Hess 1996), we model pathogen dynamics by categorizing the fraction of occupied patches as susceptible (S) or infected I, with the subscripts L and H denoting low or high quality. For simplicity, we assume that infection status does not affect the colonization parameter c; that empty patches colonized by infected patches become infected, while colonized, disease-free patches become infected by infected patches with probability δ ≤ 1. We assume that once infected, patches remain infected until local extinction occurs. Infection increases the extinction rate of low- and high-quality patches by ν L and ν H respectively; we refer to these as the disease-induced extinction rates. We assume patch improvement reduces disease-induced extinction in the same way as it reduces disease-free extinction (ν H  = ν L /q); an alternative scenario where disease-induced extinction is independent of improvement (ν H  = ν L ) is considered in Appendix C. The dynamics are now described by:

$$ d{S}_L/dt=c\left(1-f-{S}_L-{I}_L\right)\left({S}_H+{S}_L\right)-{x}_L{S}_L-\updelta c{S}_L\left({I}_H+{I}_L\right) $$
(2a)
$$ d{I}_L/dt=c\left(1-f-{S}_L-{I}_L\right)\left({I}_H+{I}_L\right)+\updelta c{S}_L\left({I}_H+{I}_L\right)-\left({x}_L+{\upnu}_L\right){I}_L $$
(2b)
$$ d{S}_H/dt=c\left(f-{S}_H-{I}_H\right)\left({S}_H+{S}_L\right)-\left({x}_L/q\right){S}_H-\updelta c{S}_H\left({I}_H+{I}_L\right) $$
(2c)
$$ d{I}_H/dt=c\left(f-{S}_H-{I}_H\right)\left({I}_H+{I}_L\right)+\updelta c{S}_H\left({I}_H+{I}_L\right)-\left(\left({x}_L/q\right)+{\upnu}_H\right){I}_H $$
(2d)

A visualization of the model formulation can be seen in Fig. 1.

Fig. 1
figure 1

Conceptual framework for the quality-dependent metapopulation model. a Transitions between patch types follow a Hess-type epidemic model of unoccupied, susceptible, and infected patches, where model parameters are defined as in Table 1. b We consider two patch types, where unprovisioned patches are of low quality (square) and resource-improved patches are of high quality (circle) and are occupied for longer. Arrow length indicates the expected persistence time for each patch type, and the quality difference parameter (q) is defined as the ratio of persistence times in patches of high (circle) versus low (square) quality. c Heterogeneity in patch quality in the metapopulation is defined by the proportion of patches at which resource improvement occurs (f). d A snapshot of the metapopulation structure in which patch status, type, and the proportion improved are as defined in parts a–c

Full size image

Modeling approach

To assess the effects of local patch improvement and its landscape-level deployment on metapopulation and disease dynamics, we co-vary the increase in patch quality resulting from resource provisioning (q) and the fraction of patches provisioned (f) and calculate the equilibrium metapopulation occupancy (N* = S L  + I L  + S H  + I H ) and infection prevalence (fraction of occupied patches infected, P* = (I L  + I H )/N*). Where possible, we derive analytical expressions for metapopulation persistence and pathogen invasion (Appendix B); otherwise, the model is numerically solved using the deSolve package in R (Soetaert et al. 2010; R Core Team 2013). For initial conditions, we set the initial occupancy of low- and high-quality patches to their disease-free equilibrium for the particular provisioning scenario (specified by f and q; analytical expressions are given in Appendix B), and introduced a small fraction of infected patches; note that the equilibrium outcomes were not influenced by the specific choice of initial conditions. We illustrate our results for two scenarios of practical importance describing the nature of resource improvement and the state of the metapopopulation prior to improvement: (A) an imperiled metapopulation declining prior to management (c < x L ) that is rescued via resource supplementation and (B) a persistent metapopulation (c > x L ) subject to improved patch quality via recreational feeding or unintentional subsidies in human-modified habitats. In both scenarios, the metapopulation is challenged by a highly transmissible, virulent pathogen; parameter definitions and default values are given in Table 1.

Table 1 Parameterization of the metapopulation model, where parameters related to resource improvement are presented in boldface
Full size table

Results

Dynamics

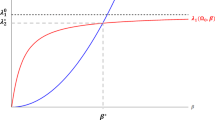
Numerical solution of the ordinary differential equations over a wide range of parameter values and initial conditions confirmed that dynamics converge on a point equilibrium after a relatively short transient period (typically 30–60 generations as measured by the expected persistence time of low-quality patches, 1/x L ). If occupancy in the unprovisioned metapopulation is too low to allow pathogen invasion, provisioning has the expected effect of increasing occupancy. However, once occupancy is high enough to permit pathogen invasion, equilibrium occupancy declines as the fraction of infected patches increases (example dynamics are shown in Fig. 2).

Fig. 2
figure 2

Example dynamics showing how total patch occupancy (bold line) and the fraction of infected patches (gray line) change through time, for the case of a declining metapopulation in which management is introduced at time t = 12. In the absence of the pathogen, equilibrium occupancy of 0.63 would be attained (dashed line). However, at time t = 23, occupancy becomes high enough to permit pathogen invasion and eventually become pandemic (i.e., all occupied patches are infected), resulting in a reduced occupancy of 0.47. Parameters were selected so that resource improvement results in a four-fold increase in the persistence time of provisioned over unprovisioned patches (q = 4) and that 80 % of patches in the metapopulation are improved (f = 0.8); other parameters describing host metapopulation and pathogen traits are given in Table 1

Full size image

Equilibrium analysis

Across all possible parameter ranges, the model has four equilibrium states: metapopulation extinction, disease-free persistence of the host, pathogen invasion and persistence at an endemic equilibrium (i.e., a constant fraction of patches are infected), and pandemic equilibrium (where all occupied patches are infected). Stability of these successive states is increasingly likely at higher degrees of local patch improvement (i.e., increasing values of q) and landscape deployment of provisioning (i.e., increasing values of f). Analytical expressions for these equilibria as functions of the model parameters are derived in Appendix B.

The threshold conditions for disease-free, endemic, and pandemic stability are described by approximately inverse relationships between the local and landscape provisioning parameters q and f (Table 2 and illustrated for Scenarios A and B in the left column of Fig. 3). Thus, we use f as our bifurcation parameter to understand how increasing provisioning influences host occupancy and pathogen prevalence, but note that qualitatively similar results would be obtained by fixing f and increasing q. We note the following general patterns between equilibrium occupancy and the fraction of patches provisioned.

Table 2 Model equilibria and conditions for their stability, expressed using the fraction of high-quality patches (f) as a bifurcation parameter
Full size table
Fig. 3
figure 3

Left: effect of local patch quality improvement (q) and the fraction of high-quality improved patches (f) on metapopulation occupancy and pathogen colonization. Shaded regions depict where the metapopulation is extinct (X, black), persists in a disease-free state (DF, white), and where the pathogen invades and becomes endemic (E, light gray) or pandemic (P, dark gray). Right: equilibrium occupancy and infection prevalence as a function of the fraction of high-quality patches (f) for moderate (q = 2) and large (q = 4) differences in patch quality. Row A illustrates results for the case of a metapopulation in decline prior to management through supplemental feeding (c = 0.9); row B illustrates results for the case of a persistent metapopulation where hosts are provisioned through resource subsidies (c = 1.4)

Full size image

  • When f df  > 0, provisioning is necessary for metapopulation persistence.

  • When f df  < f < f e , equilibrium occupancy is an increasing function of f. Therefore, provisioning is always beneficial if the pathogen is unable to invade when all patches are provisioned (i.e., when f e  > 1).

  • When f e  < f < f p , occupancy declines with increasing f. Therefore, metapopulation occupancy is maximized for mixtures of high- and low-quality patches whenever the pathogen can invade the fully provisioned metapopulation, but does not reach pandemic equilibrium (i.e., when f e  < 1 and f p  > 1).

  • When f > f p (i.e., all occupied patches are infected), occupancy again increases with f due to increased persistence time of infected provisioned patches relative to unprovisioned patches. If the pathogen is endemic in the unprovisioned metapopulation (f e  < 0), equilibrium occupancy is minimized at f = f p . If additionally the pathogen is endemic but not pandemic in a fully provisioned metapopulation (f p  > 1), provisioning is always detrimental to metapopulation occupancy.

Provisioning impacts on host occupancy and pathogen prevalence

The practical significance of these results is best illustrated with our two scenarios of (A) declining (c < x L ) versus (B) persistent (c > x L ) metapopulations prior to supplementation (i.e., when f = 0). For each scenario, we plot equilibrium occupancy and pathogen prevalence as a function of the fraction of patches provisioned (f) for two different levels of provisioning: moderate provisioning (q = 2, meaning that provisioned patches remain occupied for twice as long as unprovisioned patches in disease-free populations; Fig. 3 center panel) and high provisioning (q = 4, provisioned patches remain occupied four times as long as unprovisioned patches; Fig. 3 right panel). Although the specific values of q chosen are arbitrary, they illustrate qualitatively different relationships between occupancy and provisioning (unimodal versus non-monotonic increasing).

For a declining, managed metapopulation (scenario A) and subject to moderate provisioning, pathogen invasion is only possible when most patches are managed, and pandemic equilibrium is impossible even when all patches are provisioned (Fig. 3a, center). In this case, maintaining a mixture of high- and low-quality patches can result in higher occupancy than if all patches receive provisioning. Under high provisioning (q = 4, Fig. 3a, right), the local maximum in occupancy is attained when a smaller fraction of patches are treated. Here, maximum occupancy is achieved when all patches are provisioned, but all patches are infected. Therefore, maintaining some unmanaged, low-quality patches may be optimal both for increasing occupancy and minimizing pathogen spread.

For a persistent but subsidized metapopulation (scenario B) subject to moderate provisioning (Fig. 3b, center), provisioning always increases occupancy relative to the unprovisioned metapopulation. Yet the magnitude of this improvement declines over a large range of f, and pathogen invasion can occur when a relatively small fraction of patches is provisioned. However, under high provisioning (Fig. 3b, right), occupancy can decline below that in the unprovisioned metapopulation, and all patches become infected. Therefore, subsidizing persistent metapopulations tends to favor pathogen spread, and in some cases is also detrimental to host occupancy.

Sensitivity of results to pathogen traits

To better understand whether the non-monotonic relationships observed between provisioning and occupancy in our parameterization hold more generally, we performed extensive sensitivity analyses on pathogen parameters (Appendices C and D). Our model assumes that improvements to patch quality (increasing q) reduce both natural (x H ) and disease-induced (ν H ) extinction rates. Alternatively, if we assume that disease-induced extinction is unaffected by patch improvement (i.e., ν H  = ν L ), we obtain qualitatively similar results (Appendix C); however, for the same parameterization used in the main text, pathogen invasion occurs in a much smaller region of provisioning parameter space (when most patches receive high levels of provisioning). This is because the extinction rate of provisioned patches can never decrease below ν L , and so the positive effects of provisioning on infected patch persistence saturate.

The two cases of most practical interest in our provisioning scenarios are (1) when maintaining some low-quality patches results in higher occupancy than a fully provisioned metapopulation (i.e., when N*(f e ) > N*(1) for 0 < f e  < 1) and (2) when provisioning causes reductions in occupancy relative to the unprovisioned population (i.e., when N*(f p ) < N*(0) for f p  > 0). We varied pathogen transmissibility (probability of a colonized patch becoming infected, δ) and virulence (disease-induced extinction rate, ν L ) to see whether cases 1 and 2 occurred more generally (Appendix D; Fig. S3 and S4). Due to complex interactions between provisioning and pathogen parameters in the expressions for equilibrium occupancy, no clear mechanistic patterns emerged; however, we observed global maxima and minima in occupancy in provisioned, heterogeneous populations over a range of pathogen traits, suggesting that too much provisioning can be detrimental to metapopulations exposed to diverse pathogens.

Discussion

Variation in habitat quality has long been recognized as an important regulator of population dynamics (Holt 1985; Pulliam 1988). In source–sink dynamics, sink habitats (in which local population growth rates are negative) can play an important role in metapopulation persistence if they increase connectivity between source patches or encourage local adaptation (Dias 1996). Here, we outline conditions under which metapopulations containing some lower quality patches can attain higher occupancy than those with uniformly high-quality patches in the presence of virulent pathogens. A necessary, but not sufficient, condition for heterogeneity in patch quality to be beneficial is that low-quality patches occupied by infected host subpopulations have high extinction rates and thus act as sinks for the pathogen.

Our modeling approach has analogs in the theory of multi-host pathogen interactions, in which high- and low-quality patches are equivalent to competent reservoir and less-competent spillover host species (LoGiudice et al. 2003; Keesing et al. 2006). Comparable to the dilution effect hypothesis describing how host community composition shapes pathogen dynamics, our model suggests heterogeneity in patch quality, particularly in the frequency of low-quality patches with reduced occupancy times of hosts and pathogens, can mediate landscape-level infection prevalence. A key difference that results from treating patches rather than individual hosts as the base unit of infection is that all occupied patches can become infected if the proportion of high-quality patches is sufficiently large (whereas typically only a fraction of individual hosts in a population are infected). Existence of this pandemic equilibrium has been demonstrated as a consequence of high connectivity in homogeneous metapopulations (Hess 1996; McCallum and Dobson 2002), but this state has not been investigated in the context of habitat heterogeneity.

Resource improvement for conservation, recreation, or as a consequence of urbanization can increase local population growth and thus create source patches. However, provisioning may allow local epidemics of virulent pathogens to persist for longer (for example, due to larger host population sizes; Becker and Hall (2014)), promoting landscape-scale pathogen spread and reduction in overall occupancy. Thus, if the fraction of high-quality patches is too high, provisioned patches may in fact function as ecological traps (Schlaepfer et al. 2002).

Our analyses have practical importance for the conservation of imperiled metapopulations. If conservation management primarily relies on local habitat improvement, maintaining mixtures of unmanaged and managed patches can achieve two desirable goals: maximizing patch occupancy while minimizing risk of pathogen invasion. This could be particularly important for small subpopulations with low genetic variation, which are frequently highly susceptible to mass mortality following pathogen outbreaks (Haydon et al. 2002). In contrast, for non-threatened metapopulations subject to resource subsidies in urban or agricultural landscapes, subsidized habitat patches can lead to global declines in occupancy and to pandemic spread of infection, even when these patches are scarce relative to unsubsidized patches. Although pathogens are unlikely to cause catastrophic population declines in this case, high infection prevalence across the landscape can have negative consequences, including increased risk of spillover to wild, domestic, or human hosts or increased morbidity and mortality. For example, widespread bird feeding in the continental USA was likely a key driver in the emergence and spatial spread of mycoplasmal conjunctivitis in house finches (Altizer et al. 2004). Severe infections can cause disfiguring lesions and individual mortality, which threaten population declines and are likewise distressing to citizens who feed birds (Hochachka and Dhondt 2000).

Our model makes several simplifying assumptions that may not hold in real systems. We focused on effects of resource improvement on patch extinction rates, and following previous models (Hess 1996), we assumed that the colonization rate of all empty patches was equal irrespective of patch quality and the infection status of the donor patch. If more infected individuals (or propagules) disperse from high-quality patches, and lower quality patches produce fewer infected dispersers or propagules, we predict this would exacerbate the advantageous effects of patch heterogeneity on equilibrium occupancy by reducing disease spread relative to metapopulations with uniformly high patch quality. Our assumption of uniform colonization rates is most appropriate for highly mobile species such as birds and species dispersed passively by air or water currents or human activity (e.g., seeds or pelagic larvae). However, large inter-patch distances and geographical barriers will cause heterogeneity in movement patterns for less-mobile species. Although beyond the scope of this study, the effects of landscape heterogeneity and dispersal limitation could be investigated using spatially realistic metapopulation models (Ovaskainen and Hanski 2001). For example, our modeling approach could be extended to include patch isolation and to modify the attractiveness of each patch to depend on quality (resource availability) as well as patch size.

Our model only investigated susceptible–infected dynamics (i.e., infected patches remain infected until extinction), while infected subpopulations may be able clear infection prior to extinction (susceptible–infected–susceptible dynamics). Allowing recovery from infection makes pathogen invasion more difficult in homogeneous metapopulations by reducing the infectious period of patches (McCallum and Dobson 2002). If lower quality patches support fewer hosts, local pathogen extinction may occur well before host extinction, which would again support our hypothesis that low-quality patches can minimize pathogen impacts on occupancy. The role of patch heterogeneity in determining landscape infection prevalence may be reduced if the pathogen has an alternative, more abundant host (Gog et al. 2002) or a persistent environmental reservoir (Park 2012). Thus, our results apply primarily to specialist pathogens transmitted by direct contacts between hosts, by biting arthropod vectors, or by short-lived environmental stages (e.g., those transmitted through fecal–oral pathways). Finally, since our model tracks only patch occupancy and infection status, it cannot directly account for resource-mediated effects on individual hosts (e.g., body condition, immunity, and pathogen virulence), host population size, and subsequent infection processes. Mechanistically linking within-patch processes to between-patch spread in provisioned landscapes is worthy of further theoretical exploration, but will necessitate explicit modeling of within-patch host–pathogen dynamics (Becker and Hall 2014).

Resource improvement is increasingly recognized to facilitate pathogen transmission (Plowright et al. 2011; Becker et al. 2015), and changes to the spatial distribution and quality of resources influence population dynamics and behavior in ways that can exacerbate disease spread (Jones et al. 2014; Satterfield et al. 2015). We have developed a simple model to demonstrate how unmanaged resource subsidies for wildlife as well as conservation based in resource improvement can disproportionally contribute to the spatial dynamics of infectious disease and potentially lead to declines in occupancy of habitat patches across the landscape. To develop predictive models for the landscape-level consequences of local resource provisioning on host–pathogen dynamics, further empirical work at the landscape scale is urgently required. Estimation of local parameters relating to the intensity of provisioning (e.g., the amount and frequency of food supplements) and patch infection status (e.g., the presence, prevalence, or peak abundance of infectious hosts) will be especially important to quantify relative differences in persistence time and colonization rates between landscapes with varying configurations of low- and high-quality patches (Moilanen 2002; Barbraud et al. 2003). In particular, studies quantifying how the spatial deployment of resource subsidies influences movement between patches and pathogen spread will be vital for parameterizing predictive models and in turn guiding spatially coordinated conservation and management practices related to resource improvement and supplemental feeding.