Skip to main content
SpringerLink
Log in

Effect of couple stresses on static and dynamic characteristics of MHD wide tapered land slider bearing

  • Published:
Sādhanā Aims and scope Submit manuscript

Abstract

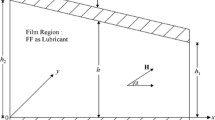
This paper presents an analytical study on the effect of couple stress on static and dynamic characteristics of wide tapered land slider bearing in the presence of applied magnetic field. A non-dimensional modified Reynolds equation is derived for the bearing under consideration. Closed-form expressions for the steady fluid film pressure, steady load carrying capacity and dynamic characteristics, viz., dynamic stiffness and dynamic damping coefficients are obtained. Numerical computations of the results revealed that magnetohydrodynamics tapered land slider bearing lubricated with couple stress fluid provides higher steady load carrying capacity, dynamic stiffness coefficient and dynamic damping coefficient as compared with the corresponding Newtonian case. It is observed that the presence of applied magnetic field characterized by the Hartmann number in the couple stress lubrication of tapered land slider bearing provides the improved static and dynamic characteristics as compared with the non-magnetic case.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Figure 1
Figure 2
Figure 3
Figure 4
Figure 5
Figure 6
Figure 7
Figure 8

Similar content being viewed by others

Abbreviations

\( B_{0} \) :

applied magnetic field (Wb/m2)

\( \bar{C}_{d} \) :

non-dimensional dynamic damping coefficient

\( d \) :

difference between the inlet and outlet film thickness (m)

\( F \) :

frictional force (N)

\( \bar{F} \) :

non-dimensional frictional force, \( \bar{F} = \frac{{Fh_{ms}^{2} }}{{\mu UL^{2} B_{0} }} \)

\( h(x,t) \) :

film thickness (m)

\( \bar{h} \) :

non-dimensional film thickness, \( \bar{h}(\bar{x},\,\bar{t}) = h(x,\,t)/h_{ms} \)

\( h_{m} (t) \) :

minimum squeezing film thickness (m)

\( \bar{h}_{m} (\bar{t}) \) :

non-dimensional minimum squeezing film thickness \( \bar{h}_{m} (\bar{t}) = h_{m} (t)/h_{ms} \)

\( h_{ms} \) :

steady-state reference minimum film thickness at outlet (m)

\( h_{1} \) :

inlet film thickness (m)

L :

length of the bearing (m)

\( l \) :

couple stress parameter (m)

\( \bar{l} \) :

non-dimensional couple stress parameter, \( \bar{l} = 2l/h_{ms} \)

\( M \) :

Hartmann number, \( M = B_{0} h_{ms} \left( {\sigma /\mu } \right)^{1/2} \)

\( p \) :

film pressure (Pa)

\( p_{s} \) :

steady film pressure (Pa)

\( \bar{p} \) :

non-dimensional film pressure, \( \bar{p} = ph_{ms}^{2} /\mu UL \)

\( \bar{p}_{s} \) :

non-dimensional steady film pressure

\( \bar{S}_{d} \) :

non-dimensional dynamic damping coefficient

\( t,\,\bar{t} \) :

time, \( \bar{t} = Ut /L \) (s)

U:

sliding velocity of lower part (m/s)

\( \bar{V} \) :

non-dimensional squeezing velocity, \( \bar{V} = d\bar{h}_{m} /d\bar{t} \)

u, w :

velocity components in x and z directions

\( W_{s} \) :

steady load carrying capacity (N)

\( \bar{W}_{s} \) :

non-dimensional steady load carrying capacity,

x, z :

Cartesian coordinates

\( \bar{x} \) :

non-dimensional coordinate \( \bar{x} = x /L \)

\( \delta \) :

profile parameter \( \delta = d /h_{ms} \)

\( \eta \) :

material constant responsible for couple stress parameter (Ns)

\( \mu \) :

lubricant viscosity (Pa s)

\( \sigma \) :

conductivity of the lubricant (mho/m)

References

  1. Stokes V K 1966 Couple stresses in fluids. Phys. Fluids 9: 1709–1715

    Article  Google Scholar 

  2. Sinha P and Singh C 1981 The effect of additives in the lubricant of a composite bearing with an inclined stepped surface. Wear 66: 17–26

    Article  Google Scholar 

  3. Ramanaiah G and Sarkar P 1979 Slider bearings lubricated by fluids with couple stress. Wear 52: 27–36

    Article  Google Scholar 

  4. Naduvinamani N B and Siddangouda A 2009 Squeeze film lubrication between circular stepped plates of couple stress fluids. J. Braz. Soc. Mech. Sci. Eng. 31: 21–26

    Article  Google Scholar 

  5. Apparao S, Naduvinamani N B and Patil M D 2013 Lubrication characteristics of porous inclined stepped composite bearings with couple stress fluids. Tribol. Online 8: 234–241

    Article  Google Scholar 

  6. Biradar T V 2013 Squeeze film lubrication between porous parallel stepped plates with couple stress fluids. Tribol. Online 8: 278–284

    Article  Google Scholar 

  7. Naduvinamani N B, Apparao S, Gundayya H A and Biradar S N 2015 Effect of pressure dependent viscosity on couple stress squeeze film lubrication between rough parallel plates. Tribol. Online 10: 76–83

    Article  Google Scholar 

  8. Hughes W F 1963 The magnetohydrodynamic inclined slider bearing with a transverse magnetic field. Wear 6: 315–324

    Article  Google Scholar 

  9. Malik M and Singh D V 1980 Analysis of finite magnetohydrodynamic journal bearings. Wear 62: 273–280

    Article  Google Scholar 

  10. Dudzinsky S J, Young F J and Hughes W F 1968 On the load carrying capacity of MHD journal bearing. J. Lubr. Technol. 90: 139–144

    Article  Google Scholar 

  11. Das N C 1998 A study of optimum load-bearing capacity for slider bearings lubricated with couple stress fluids in magnetic field. Tribol. Int. 31: 393–400

    Article  Google Scholar 

  12. Naduvinamani N B and Rajashekhar M 2011 MHD couple stress squeeze film characteristics between sphere and plane surface. Tribol. Mater. Surf. Interfaces 5: 94–99

    Article  Google Scholar 

  13. Lin J R, Chu L M, Hung C and Wang P Y 2013 Derivation of two dimensional couple stress hydromagnetic squeeze film Reynolds equation and application to wide parallel rectangular plates. Meccanica 48: 253–258.

    Article  Google Scholar 

  14. Fathima S T, Naduvinamani N B, Hanumagowda B N and Santoshkumar J 2015 Modified Reynolds equation for different types of finite plates with the combined effect of MHD and couple stresses. Tribol. Trans. 58: 660–667

    Article  Google Scholar 

  15. Taylor C M and Dowson D 1974 Turbulent lubrication theory – application to design. ASME J. Lubr. Technol. 96: 36–46

    Article  Google Scholar 

  16. Lin J R, Hung C R, Hsu C H and Lai C 2009 Dynamic stiffness and damping characteristics of one-dimensional magnetohydrodynamic inclined-plane slider bearings. Proc. Inst. Mech. Eng. J. J. Eng. Tribol. 223: 211–219

    Article  Google Scholar 

  17. Lin J R, Lu R F, Ho M H and Lin M C 2006 Dynamic characteristics of wide tapered land slider bearings. J. Chin. Inst. Eng. 29: 543–548

    Article  Google Scholar 

  18. Lin J R 2010 MHD steady and dynamic characteristics of wide tapered land slider bearings. Tribol. Int. 43: 2378–2383

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, Gulbarga University, Kalaburagi, 585106, India

    N B Naduvinamani & S Shridevi

  2. Department of Mathematics, Appa Institute of Engineering and Technology, Kalaburagi, 585103, India

    A Siddangouda

  3. Department of Science, Government Polytechnic, Shorapur, 585224, India

    Siddharama Patil

Authors
  1. N B Naduvinamani
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. A Siddangouda
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Siddharama Patil
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. S Shridevi
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to N B Naduvinamani.

Appendix A

Appendix A

$$ f_{11} = \frac{{\beta^{2} }}{{\left( {\alpha^{2} - \beta^{2} } \right)}}\left\{ {\frac{{\sinh \left( {\frac{\alpha h}{l}} \right) - \sinh \left( {\frac{\alpha z}{l}} \right) + \sinh \left( {\frac{{\alpha \left( {h - z} \right)}}{l}} \right)}}{{\sinh \left( {\frac{\alpha h}{l}} \right)}}} \right\} $$
(A1a)
$$ f_{12} = \frac{{\alpha^{2} }}{{\left( {\alpha^{2} - \beta^{2} } \right)}}\left\{ {\frac{{\sinh \left( {\frac{\beta h}{l}} \right) - \sinh \left( {\frac{\beta z}{l}} \right) + \sinh \frac{{\beta \left( {h - z} \right)}}{l}}}{{\sinh \left( {\frac{\beta h}{l}} \right)}}} \right\} $$
(A1b)
$$ f_{13} = \frac{{\beta^{2} }}{{\frac{{\beta^{2} }}{\alpha }\tanh \left( {\frac{\alpha h}{2l}} \right) - \frac{{\alpha^{2} }}{\beta }\tanh \left( {\frac{\beta h}{2l}} \right)}}\left\{ {\frac{{\sinh \left( {\frac{\alpha h}{l}} \right) - \sinh \left( {\frac{\alpha z}{l}} \right) - \sinh \frac{{\alpha \left( {h - z} \right)}}{l}}}{{\sinh \left( {\frac{\alpha h}{l}} \right)}}} \right\} $$
(A1c)
$$ f_{14} = \frac{{\alpha^{2} }}{{\frac{{\beta^{2} }}{\alpha }\tanh \left( {\frac{\alpha h}{2l}} \right) - \frac{{\alpha^{2} }}{\beta }\tanh \left( {\frac{\beta h}{2l}} \right)}}\left\{ {\frac{{\sinh \left( {\frac{\beta h}{l}} \right) - \sinh \left( {\frac{\beta z}{l}} \right) - \sinh \frac{{\beta \left( {h - z} \right)}}{l}}}{{\sinh \left( {\frac{\beta h}{l}} \right)}}} \right\} $$
(A1d)
$$ f_{21} = \frac{{{\sin}h\left( {\frac{z - h}{\sqrt 2 l}} \right) + {\sin}h\left( {\frac{z}{\sqrt 2 l}} \right) - {\sin}h\left( {\frac{h}{\sqrt 2 l}} \right)}}{{{\sin}h\left( {\frac{h}{\sqrt 2 l}} \right)}} $$
(A1e)
$$ f_{22} = \frac{{z{\cos}h\left( {\frac{z - h}{\sqrt 2 l}} \right) + y{\cos}h\left( {\frac{z}{\sqrt 2 l}} \right) - h\coth \left( {\frac{h}{2\sqrt 2 l}} \right)\sinh \left( {\frac{z}{\sqrt 2 l}} \right)}}{{2\sqrt 2 l\,{\sin}h\left( {\frac{h}{\sqrt 2 l}} \right)}} $$
(A1f)
$$ f_{23} = \frac{{z{\sin}h\left( {\frac{z - h}{\sqrt 2 l}} \right) + z\sinh \left( {\frac{z}{\sqrt 2 l}} \right) - h\sinh \left( {\frac{z}{\sqrt 2 l}} \right)}}{{\left( {6l\,{\sin}h\left( {\frac{h}{\sqrt 2 l}} \right) - \sqrt 2 h} \right)}} $$
(A1g)
$$ f_{24} = \frac{{2{\cos}{h}\left( {\frac{z - h}{\sqrt 2 l}} \right) + 2{\cos}{h}\left( {\frac{z}{\sqrt 2 l}} \right) - 2{\cos}{h}\left( {\frac{h}{\sqrt 2 l}} \right) - 2}}{{\left( {3\sqrt 2 {\sin}{h}\left( {\frac{h}{\sqrt 2 l}} \right) - \frac{h}{l}} \right)}} $$
(A1h)
$$ f_{31} = \frac{{{{{\cosh}}} \alpha_{1} z{\cos}\beta_{1} \left( {z - h} \right) - {\cos} \beta_{1} z{\cos}h\alpha_{1} \left( {z - h} \right)}}{{\left( {{\cos}h\alpha_{1} h - {\cos}\beta_{1} h} \right)}} $$
(A1i)
$$ f_{32} = \frac{{\cot\varphi \left\{ {{{{\sinh}}} \alpha_{1} z{\sin}\beta_{1} \left( {z - h} \right) - {\sin} \beta_{1} z{\sin}h\alpha_{1} \left( {z - h} \right)} \right\} + \left( {{\cos}h\alpha_{1} h - {\cos}\beta_{1} h} \right)}}{{\left( {{\cos}h\alpha_{1} h - {\cos}\beta_{1} h} \right)}} $$
(A1j)
$$ f_{33} = \frac{M}{{h_{ms} }}\left\{ {\frac{{\cot\varphi \left\{ {{{\sin}h}\alpha_{1} z\sin\beta_{1} \left( {z - h} \right) + \sin\beta_{1} z{\sin}h\alpha_{1} \left( {z - h} \right)} \right\} + \left( {\cos\beta_{1} h + {\cos}h\alpha_{1} h} \right)}}{{\left( {\beta_{1} - \alpha_{1} \cot\varphi } \right)\sin\beta_{1} h + \left( {\alpha_{1} + \beta_{1} \cot\varphi } \right){\sin}h\alpha_{1} h}}} \right\} $$
(A1k)
$$ f_{34} = \frac{M}{{h_{ms} }}\left\{ {\frac{{{\cos} \beta_{1} z{\cos}h\alpha_{1} \left( {z - h} \right) + {\cosh} \alpha_{1} z\cos\beta_{1} \left( {z - h} \right)}}{{\left( {\beta_{1} - \alpha_{1} \cot\varphi } \right)\sin\beta_{1} h + \left( {\alpha_{1} + \beta_{1} \cot\varphi } \right){\sin}h\alpha_{1} h}}} \right\} $$
(A1l)
$$ \xi_{A} \left( {\bar{x},\,\bar{h}_{m} } \right) = \int\limits_{{\bar{x} = 0}}^{{\bar{x}}} {\frac{{\bar{h}_{1} (\bar{x},t)}}{{\bar{g}\left( {\bar{h},\,\bar{l},\,M} \right)}}} d\bar{x} $$
(A2a)
$$ \xi_{B} \left( {\bar{x},\,\bar{h}_{m} } \right) = \int\limits_{{\bar{x} = 0}}^{{\bar{x}}} {\frac{{\bar{x}}}{{\bar{g}\left( {\bar{h},\,\bar{l},\,M} \right)}}} d\bar{x} $$
(A2b)
$$ \xi_{C} \left( {\bar{x},\,\bar{h}_{m} } \right) = \int\limits_{{\bar{x} = 0}}^{{\bar{x}}} {\frac{1}{{\bar{g}\left( {\bar{h},\,\bar{l},\,M} \right)}}} d\bar{x} $$
(A2c)
$$ \xi_{D} \left( {\bar{x},\,\bar{h}_{m} } \right) = \int\limits_{{\bar{x} = 1}}^{{\bar{x}}} {\frac{{\bar{x}}}{{\bar{g}\left( {\bar{h},\,\bar{l},\,M} \right)}}} d\bar{x} $$
(A2d)
$$ \xi_{E} \left( {\bar{x},\,\bar{h}_{m} } \right) = \int\limits_{{\bar{x} = 1}}^{{\bar{x}}} {\frac{1}{{\bar{g}\left( {\bar{h},\,\bar{l},\,M} \right)}}} d\bar{x} $$
(A2e)
$$ \xi_{AK} \left( {\bar{h}_{m} } \right) = \int\limits_{{\bar{x} = 0}}^{K} {\frac{{\bar{h}_{1} (\bar{x},t)}}{{\bar{g}\left( {\bar{h},\,\bar{l},\,M} \right)}}} d\bar{x} $$
(A2f)
$$ \xi_{BK} \left( {\bar{h}_{m} } \right) = \int\limits_{{\bar{x} = 0}}^{K} {\frac{{\bar{x}}}{{\bar{g}\left( {\bar{h},\,\bar{l},\,M} \right)}}} d\bar{x} $$
(A2g)
$$ \xi_{CK} \left( {\bar{h}_{m} } \right) = \int\limits_{{\bar{x} = 0}}^{K} {\frac{1}{{\bar{g}\left( {\bar{h},\,\bar{l},\,M} \right)}}} d\bar{x} $$
(A2h)
$$ \xi_{DK} \left( {\bar{h}_{m} } \right) = \int\limits_{{\bar{x} = 1}}^{K} {\frac{{\bar{x}}}{{\bar{g}\left( {\bar{h},\,\bar{l},\,M} \right)}}} d\bar{x} $$
(A2i)
$$ \xi_{EK} \left( {\bar{h}_{m} } \right) = \int\limits_{{\bar{x} = 1}}^{K} {\frac{1}{{\bar{g}\left( {\bar{h},\,\bar{l},\,M} \right)}}} d\bar{x} $$
(A2j)
$$ \chi_{AK} \left( {\bar{h}_{m} } \right) = \int\limits_{{\bar{x} = 0}}^{K} {\int\limits_{{\bar{x} = 0}}^{{\bar{x}}} {\frac{{\bar{h}_{1} (\bar{x},t)}}{{\bar{g}\left( {\bar{h},\,\bar{l},\,M} \right)}}d\bar{x}\,} } d\bar{x} $$
(A3a)
$$ \chi_{BK} \left( {\bar{h}_{m} } \right) = \int\limits_{{\bar{x} = 0}}^{K} {\int\limits_{{\bar{x} = 0}}^{{\bar{x}}} {\frac{{\bar{x}}}{{\bar{g}\left( {\bar{h},\,\bar{l},\,M} \right)}}d\bar{x}\,} } d\bar{x} $$
(A3b)
$$ \chi_{CK} \left( {\bar{h}_{m} } \right) = \int\limits_{{\bar{x} = 0}}^{K} {\int\limits_{{\bar{x} = 0}}^{{\bar{x}}} {\frac{1}{{\bar{g}\left( {\bar{h},\,\bar{l},\,M} \right)}}d\bar{x}\,} } d\bar{x} $$
(A3c)
$$ \chi_{DK} \left( {\bar{h}_{m} } \right) = \int\limits_{{\bar{x} = K}}^{1} {\int\limits_{{\bar{x} = 1}}^{{\bar{x}}} {\frac{{\bar{x}}}{{\bar{g}\left( {\bar{h},\,\bar{l},\,M} \right)}}d\bar{x}\,} } d\bar{x} $$
(A3d)
$$ \chi_{EK} \left( {\bar{h}_{m} } \right) = \int\limits_{{\bar{x} = K}}^{1} {\int\limits_{{\bar{x} = 1}}^{{\bar{x}}} {\frac{1}{{\bar{g}\left( {\bar{h},\,\bar{l},\,M} \right)}}d\bar{x}\,} } d\bar{x} $$
(A3e)
$$ \frac{{\partial \chi_{AK} }}{{\partial \bar{h}_{m} }} = - \int\limits_{{\bar{x} = 0}}^{K} {\int\limits_{{\bar{x} = 0}}^{{\bar{x}}} {\frac{{\bar{h}_{1} \left( {\bar{x}} \right)}}{{\left\{ {\bar{g}\left( {\bar{h},\,\bar{l},\,M} \right)} \right\}^{2} }}\frac{{\partial \bar{g}}}{{\partial \bar{h}_{m} }}} }\,d\bar{x}\,d\bar{x} $$
(A4a)
$$ \frac{{\partial \chi_{CK} }}{{\partial \bar{h}_{m} }} = - \int\limits_{{\bar{x} = 0}}^{K} {\int\limits_{{\bar{x} = 0}}^{{\bar{x}}} {\frac{1}{{\left\{ {\bar{g}\left( {\bar{h},\,\bar{l},\,M} \right)} \right\}^{2} }}\frac{{\partial \bar{g}}}{{\partial \bar{h}_{m} }}} }\,d\bar{x}\,d\bar{x} $$
(A4b)
$$ \frac{{\partial \chi_{EK} }}{{\partial \bar{h}_{m} }} = - \int\limits_{{\bar{x} = K}}^{1} {\int\limits_{{\bar{x} = 1}}^{{\bar{x}}} {\frac{1}{{\left\{ {\bar{g}\left( {\bar{h},\,\bar{l},\,M} \right)} \right\}^{2} }}\frac{{\partial \bar{g}}}{{\partial \bar{h}_{m} }}} }\,d\bar{x}\,d\bar{x} $$
(A4c)
$$ \left( {\frac{{\partial C_{1} }}{{\partial \bar{h}_{m} }}} \right)_{s} = - 6\left[ {\frac{{\left\{ {\xi_{CK} - \xi_{EK} } \right\}\frac{{\partial \xi_{AK} }}{{\partial \bar{h}_{m} }} - \xi_{AK} \left( {\frac{{\partial \xi_{CK} }}{{\partial \bar{h}_{m} }} - \frac{{\partial \xi_{EK} }}{{\partial \bar{h}_{m} }}} \right)}}{{\left\{ {\xi_{CK} - \xi_{EK} } \right\}^{2} }}} \right] $$
(A4d)
$$ \left( {\frac{{\partial C_{1} }}{{\partial \bar{V}}}} \right)_{s} = 12\left[ {\frac{{\xi_{DK} - \xi_{BK} }}{{\xi_{CK} - \xi_{EK} }}} \right] $$
(A4e)

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Naduvinamani, N.B., Siddangouda, A., Patil, S. et al. Effect of couple stresses on static and dynamic characteristics of MHD wide tapered land slider bearing. Sādhanā 43, 162 (2018). https://doi.org/10.1007/s12046-018-0940-9

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s12046-018-0940-9

Keywords

Search

Navigation