Skip to main content

Advertisement

SpringerLink
Log in

Global stability-based design optimization of truss structures using multiple objectives

  • Published:
Sadhana Aims and scope Submit manuscript

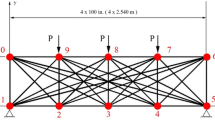
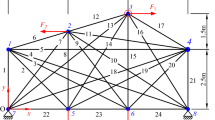
Abstract

This paper discusses the effect of global stability on the optimal size and shape of truss structures taking into account of a nonlinear critical load, truss weight and serviceability at the same time. The nonlinear critical load is computed by arc-length method. In order to increase the accuracy of the estimation of critical load (ignoring material nonlinearity), an eigenvalue analysis is implemented into the arc-length method. Furthermore, a pure pareto-ranking based multi-objective optimization model is employed for the design optimization of the truss structure with multiple objectives. The computational performance of the optimization model is increased by implementing an island model into its evolutionary search mechanism. The proposed design optimization approach is applied for both size and shape optimization of real world trusses including 101, 224 and 444 bars and successful in generating feasible designations in a large and complex design space. It is observed that the computational performance of pareto-ranking based island model is better than the pure pareto-ranking based model. Therefore, pareto-ranking based island model is recommended to optimize the design of truss structure possessing geometric nonlinearity.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Figure 1
Figure 2
Figure 3
Figure 4
Figure 5
Figure 6
Figure 7
Figure 8
Figure 9
Figure 10
Figure 11
Figure 12
Fig. 13
Figure 14
Figure 15
Figure 16
Figure 17
Figure 18
Figure 19

Similar content being viewed by others

References

  • Cantu-Paz E 1999 Migration policies and takeover times in parallel Genetic Algorithms. Int. Conf. on Genetic and Evolutionary Computation 775–779

  • Cardoso J B and Arora J S 1988 Variational method for design sensitivity analysis in nonlinear structural mechanics. AIAA J. 26: 5–22

    Article  MathSciNet  Google Scholar 

  • Choi K K and Santos J L T 1987 Design sensitivity analysis of nonlinear structural systems, Part I: theory. Int. J. Numer. Meth. Eng. 24: 2039–2055

    Article  MATH  Google Scholar 

  • Crisfield M A 1997 Nonlinear finite element analysis of solids and structures, Vol. 2 Advanced topics. UK: John Wiley & Sons

    MATH  Google Scholar 

  • Eby D, Averill R, Goodman E and Punch W 1999 The optimization of flywheels using an injection island genetic algorithm. Evol. Design Computers 167–190

  • Gien H 2007 Geometrically nonlinear static analysis of 3D trusses using the arc-length method. 13th Int. Conf. on Comp. Methods and Experimental Meas. Prague, Czech Republic

  • Goldberg D E and Richardson J 1987 Genetic algorithms with sharing for multimodal function optimization. Genetic algorithms and their applications, 2nd Int. Conf. on Genetic Algor. and Their Appl. Massachusetts, USA, 41–49

  • Hasancebi O and Erbatur F 2002 Layout optimization of trusses using simulated annealing. Advance Eng. Soft. 33: 681–696

    Article  MATH  Google Scholar 

  • Kamat M P, Khot N S and Venkayya V B 1984 Optimization of shallow trusses against limit point instability. AIAA J. 22: 403–408

    Article  MATH  Google Scholar 

  • Khot N S and Kamat M P 1985 Minimum weight design of truss structures with geometric nonlinear behavior. AIAA J. 23: 139–144

    Article  MATH  Google Scholar 

  • Khot N S, Venkayya V B and Berke L 1976 Optimum structural design with stability constraints. Int. J. Num. Meth. Eng. 10: 1097–1114

    Article  MATH  Google Scholar 

  • Kouhia R and Mikkola M 1999 Some aspects of efficient path-following. Comput. and Struct. 72: 509–524

    Article  MATH  Google Scholar 

  • Krenk S 1995 An orthogonal residual procedure for nonlinear finite element equations. Int. J. Numer. Meth. Eng. 38(5): 823–839

    Article  MathSciNet  MATH  Google Scholar 

  • Lamberti L and Pappalettere C 2004 Improved sequential linear programming formulation for structural weight minimization. Comput. Methods Appl. Mech. Eng. 193: 3493–3521

    Article  MATH  Google Scholar 

  • Levy R 1994a Optimization for buckling with exact geometries. Comput. and Struct. 53: 1139–1144

    Article  MATH  Google Scholar 

  • Levy R 1994b Optimal design of trusses for overall stability. Comput. and Struct. 53(5): 1133–1138

    Article  MATH  Google Scholar 

  • Levy R and Perng H S 1988 Optimization for nonlinear stability. Comput. and Struct. 30: 529–535

    Article  Google Scholar 

  • Levy R, Su M and Kocvara M 2004 On the modeling and solving of the truss design problem with global stability constraints. Struc. Multidisc. Opt. 26: 367–378

    Article  Google Scholar 

  • Lin C C and Liu I W 1989 Optimal design based on optimality criterion for frame structures including buckling constraints. Comput. and Struct. 31(4): 535–544

    Article  Google Scholar 

  • Magnusson A 2006 Treatment of bifurcation points with asymptotic expansion. Comput. and Struct. 77: 475–484

    Article  MathSciNet  Google Scholar 

  • Malott B, Averill R C, Goodman E D, Ding Y and Punch W F 1996 Use of genetic algorithms for optimal design of laminated composite sandwich panels with bending-twisting coupling. 37th Int. Conf. of Struc. Dyn and Mat. AIAA/ASME/ASCE/AHC/ASC. Utah, USA

  • Memon B A and Su X 2004 Arc-length technique for nonlinear finite element analysis. J. Zhejiang Univ. Science 5(5): 618–628

    Article  MATH  Google Scholar 

  • Ohsaki M 2001 Sensitivity analysis and optimization corresponding to a degenerate critical point. Int. J. Solids Struct. 38: 4955–4967

    Article  MATH  Google Scholar 

  • Ohsaki M 2005 Design sensitivity analysis and optimization for nonlinear buckling of finite-dimensional elastic conservative structures. Comp. Meth. Appl. Mech. Eng. 194: 3331–3358

    Article  MATH  Google Scholar 

  • Ohsaki M and Ikeda K 2006 Imperfection sensitivity analysis of hill-top branching with many symmetric bifurcation points. Int. J. Solids Struct. 43(16): 4704–4719

    Article  MATH  Google Scholar 

  • Ortiz T A and Walls R H 2003 Wtest: test for homogeneity of variances. http://www.mathworks.com/matlabcentral/fileexchange/

  • Plaut R H, Ruangsilasingha P and Kamat M P 1984 Optimization of an asymmetric two-bar truss against instability. J. Struct. Mech. 12(4): 465–470

    Article  Google Scholar 

  • Polheim H 1998 Genetic and evolutionary algorithm toolbox for use with MATLAB. Technical Report. Technical University Ilmnau

  • Rezaiee-Rojand M and Vejdari-Nogreiyan H R 2006 Computation of multiple bifurcation points. Int. J. Computer-aided Eng. Soft. 23(5): 552–565

    Article  Google Scholar 

  • Ritto-Correa M and Camotim D 2008 On the Arc-length and other quadratic control methods: Established, less known and new implementation procedures. Comput. and Struct. 86: 1353–1368

    Article  Google Scholar 

  • Santos J L T and Choi K K 1988 Sizing design sensitivity analysis of nonlinear structural systems, Part II. Int. J. Numer. Meth. Eng. 26: 2039–2055

    Article  Google Scholar 

  • Schlierkamp-Voosen D and Mühlenbein H 1996 Adaptation of population sizes by competing subpopulations. Int. Conf. on Evolutionary Computation IEEE 330–335

  • Sedaghati R and Tabarrok B 2000 Optimum design of truss structures undergoing large deflections subject to a system stability constraint. Int. J. Numer. Meth. Eng. 48(3): 421–434

    Article  MATH  Google Scholar 

  • Talaslioglu T 2010 Multiobjective design optimization of grillage systems by scatter search methodology. Int. J. Civil Struc. Eng. 1(3): 477–496

    Google Scholar 

  • Veldhuizen D V and Lamont G B 1998 Multi-objective evolutionary algorithm research: a history and analysis. Technical Report TR-98-03. Ohio, USA: Department of Electrical and Computer Engineering, Air Force Institute of Technology

  • Wrigger P, Wagner W and Miehe C 1988 Quadratically convergent procedure for the calculation of stability points in finite elements. Comp. Meth. Appl. Mech. 70: 329–347

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Civil Engineering, Korkut Ata University, Osmaniye, 8000, Turkey

    TUGRUL TALASLIOGLU

Authors
  1. TUGRUL TALASLIOGLU
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to TUGRUL TALASLIOGLU.

Appendices

Appendix A. Computation of nodal coordinates.

Node number

Matlab expressions used to compute nodal coordinates of arc structure with 101 bar

X Coordinate

Y Coordinate

1

−(R1*sin(pi*(45-alpha1)/180))

(R1*cos(pi*(45-alpha1)/180))

2

−((R1+a1)*sin(pi*(45-alpha1)/180))

((R1+a1)*cos(pi*(45-alpha1)/180))

3

−(R2*sin(pi*(45-alpha2)/180))

(R2*cos(pi*(45-alpha2)/180))

4

−((R2+a2)*sin(pi*(45-alpha2)/180))

((R2+a2)*cos(pi*(45-alpha2)/180))

5

−(R3*sin(pi*(45-alpha3)/180))

(R3*cos(pi*(45-alpha3)/180))

6

−((R3+a3)*sin(pi*(45-alpha3)/180))

((R3+a3)*cos(pi*(45-alpha3)/180))

7

−(R4*sin(pi*(45-alpha4)/180))

(R4*cos(pi*(45-alpha4)/180))

8

−((R4+a4)*sin(pi*(45-alpha4)/180))

((R4+a4)*cos(pi*(45-alpha4)/180))

9

−(R5*sin(pi*(45-alpha5)/180))

(R5*cos(pi*(45-alpha5)/180))

10

−((R5+a5)*sin(pi*(45-alpha5)/180))

((R5+a5)*cos(pi*(45-alpha5)/180))

11

−(R6*sin(pi*(45-alpha6)/180))

(R6*cos(pi*(45-alpha6)/180))

12

−((R6+a6)*sin(pi*(45-alpha6)/180))

((R6+a6)*cos(pi*(45-alpha6)/180))

13

−(R7*sin(pi*(45-alpha7)/180))

(R7*cos(pi*(45-alpha7)/180))

14

−((R7+a7)*sin(pi*(45-alpha7)/180))

((R7+a7)*cos(pi*(45-alpha7)/180))

15

−(R8*sin(pi*(45-alpha8)/180))

(R8*cos(pi*(45-alpha8)/180))

16

−((R8+a8)*sin(pi*(45-alpha8)/180))

((R8+a8)*cos(pi*(45-alpha8)/180))

17

−(R9*sin(pi*(45-alpha9)/180))

(R9*cos(pi*(45-alpha9)/180))

18

−((R9+a9)*sin(pi*(45-alpha9)/180))

((R9+a9)*cos(pi*(45-alpha9)/180))

19

−(R10*sin(pi*(45-alpha10)/180))

(R10*cos(pi*(45-alpha10)/180))

20

−((R10+a10)*sin(pi*(45-alpha10)/180))

((R10+a10)*cos(pi*(45-alpha10)/180))

21

0

R11

22

0

(R11+a11)

Nomenclature

p ext :

External joint load

P :

Load increment used for incremental stage

p int :

Internal force

R :

Residual force

δ1 :

Displacement increment computed in the end of iteration process (beginning point of incremental stage or first end of arc-length)

δ2:

Displacement increment computed by external load (in incremental stage)

δ3:

Sub-displacement computed in incremental stage but updated in iterative stage

δ4:

Sub-displacement computed using residual force r (in iterative stage)

δ5:

Sub-displacement increment for iterative stage

β :

Scaling factor

β1:

Sub-scaling factor used to update β computed in iterative stage

ε :

Desired convergence degree

inc_max :

Maximum number of increments

it_max :

Maximum number of iterations

K :

System stiffness matrix

det[K] :

Determinant of stiffness matrix

L :

Limit Load

W :

Weight of truss structure

δ :

Deflection

x,y,z :

Coordinates of nodes

P :

Material density

Nda :

Number of different cross-sectional areas

Nn :

Number of nodes

Ntm :

Number of truss member

Rights and permissions

Reprints and permissions

About this article

Cite this article

TALASLIOGLU, T. Global stability-based design optimization of truss structures using multiple objectives. Sadhana 38, 37–68 (2013). https://doi.org/10.1007/s12046-013-0111-y

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s12046-013-0111-y

Keywords

Search

Navigation