Untangling two systems of noncrossing curves

Abstract

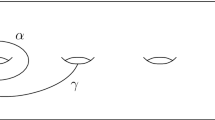
We consider two systems (α ₁, …, α _m ) and (β ₁, …,β _n ) of simple curves drawn on a compact two-dimensional surface M with boundary.

Each α _i and each β _j is either an arc meeting the boundary of M at its two endpoints, or a closed curve. The α _i are pairwise disjoint except for possibly sharing endpoints, and similarly for the β _j . We want to “untangle” the β _j from the ai by a self-homeomorphism of M; more precisely, we seek a homeomorphism φ:M→M fixing the boundary of M pointwise such that the total number of crossings of the ai with the φ(β _j ) is as small as possible. This problem is motivated by an application in the algorithmic theory of embeddings and 3-manifolds.

We prove that if M is planar, i.e., a sphere with h ≥ 0 boundary components (“holes”), then O(mn) crossings can be achieved (independently of h), which is asymptotically tight, as an easy lower bound shows.

In general, for an arbitrary (orientable or nonorientable) surface M with h holes and of (orientable or nonorientable) genus g ≥ 0, we obtain an O((m + n)⁴) upper bound, again independent of h and g.

The proofs rely, among other things, on a result concerning simultaneous planar drawings of graphs by Erten and Kobourov.