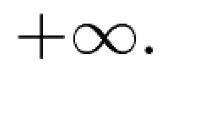Skip to main content
SpringerLink
Log in

Geometric properties of infinite graphs and the Hardy–Littlewood maximal operator

  • Published:
Journal d'Analyse Mathématique Aims and scope

Abstract

We study different geometric properties on infinite graphs, related to the weak-type boundedness of the Hardy–Littlewood maximal averaging operator. In particular, we analyze the connections between the doubling condition, having finite dilation and overlapping indices, uniformly bounded degree, the equidistant comparison property and the weak-type boundedness of the centered Hardy–Littlewood maximal operator. Several non-trivial examples of infinite graphs are given to illustrate the differences among these properties.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. D. Aalto and J. Kinnunen, The discrete maximal operator in metric spaces, J. Anal. Math. 111 (2010), 369–390.

    Article  MathSciNet  MATH  Google Scholar 

  2. M. Akcoglu, J. Baxter, A. Bellow and R. L. Jones, On restricted weak type (1,1); the discrete case, Israel J. Math. 124 (2001), 285–297.

    Article  MathSciNet  MATH  Google Scholar 

  3. J. M. Aldaz, Local comparability of measures, averaging and maximal averaging operators, Potential Anal. 49 (2018), 309–330.

    Article  MathSciNet  MATH  Google Scholar 

  4. N. Alon and J. H. Spencer, The Probabilistic Method, Wiley-Interscience Series in Discrete Mathematics and Optimization, John Wiley & Sons, Inc., Hoboken, NJ, 2008.

    Book  MATH  Google Scholar 

  5. B. Bollobás, Modern Graph Theory, Graduate Texts in Mathematics, Vol. 184, Springer-Verlag, New York, 1998.

  6. A. Bondy and U. S. R. Murty, Graph Theory, Graduate Texts in Mathematics, Vol. 244, Springer- Verlag, New York, 2008.

  7. J. Bourgain, On the maximal ergodic theorem for certain subsets of the integers, Israel J. Math. 61 (1988), 39–72.

    Article  MathSciNet  MATH  Google Scholar 

  8. A. Carbonaro, G. Mauceri and S. Meda, H1 and BMO for certain locally doubling metric measure spaces, Ann. Sc. Norm. Super. Pisa Cl. Sci. (5) 8 (2009), 543–582.

    Google Scholar 

  9. E. Carneiro and K. Hughes, On the endpoint regularity of discrete maximal operators, Math. Res. Lett. 19 (2012), 1245–1262.

    Article  MathSciNet  MATH  Google Scholar 

  10. M. Cowling, S. Meda and A. G. Setti, A weak type (1,1) estimate for a maximal operator on a group of isometries of homogeneous trees, Colloq. Math. 118 (2010), 223–232.

    Article  MathSciNet  MATH  Google Scholar 

  11. M. Gromov, Asymptotic invariants of infinite groups, in Geometric Group Theory, Vol. 2 (Sussex, 1991), Cambridge University Press, Cambridge, 1993, pp. 1–295.

    Google Scholar 

  12. J. Heinonen, Lectures on Analysis on Metric Spaces, Springer-Verlag, New York, 2001.

    Book  MATH  Google Scholar 

  13. A. Ionescu, An endpoint estimate for the discrete spherical maximal function, Proc. Amer. Math. Soc. 132 (2004), 1411–1417.

    Article  MathSciNet  MATH  Google Scholar 

  14. A. Magyar, E. M. Stein and S. Wainger, Discrete analogues in harmonic analysis: spherical averages, Ann. of Math. (2) 155 (2002), 189–208.

    Article  MathSciNet  MATH  Google Scholar 

  15. M. T. Menárguez and F. Soria, Weak type (1,1) inequalities of maximal convolution operators, Rend. Circ. Mat. Palermo (2) 41 (1992), 342–352.

    Article  MathSciNet  MATH  Google Scholar 

  16. K. H. Moon, On restricted weak type (1,1), Proc. Amer. Math. Soc. 42 (1974), 148–152.

    MathSciNet  MATH  Google Scholar 

  17. J. Nagata, Note on dimension theory for metric spaces, Fund. Math. 45 (1958), 143–181.

    Article  MathSciNet  MATH  Google Scholar 

  18. A. Naor and T. Tao, Random martingales and localization of maximal inequalities, J. Funct. Anal. 259 (2010), 731–779.

    Article  MathSciNet  MATH  Google Scholar 

  19. F. Nazarov, S. Treil, and A. Volberg, Weak type estimates and Cotlar inequalities for Calderón–Zygmund operators on nonhomogeneous spaces, Internat. Math. Res. Notices 9 (1998), 463–487.

    Article  MATH  Google Scholar 

  20. R. Rochberg and M. Taibleson, Factorization of the Green’s operator and weak type estimates for a random walk on a tree, Publ. Mat. 35 (1991), 187–207.

    Article  MathSciNet  MATH  Google Scholar 

  21. Y. Sawano, Sharp estimates of the modified Hardy–Littlewood maximal operator on the nonhomogeneous space via covering lemmas, Hokkaido Math. J. 34 (2005), 435–458.

    Article  MathSciNet  MATH  Google Scholar 

  22. J. Soria and P. Tradacete, Best constants for the Hardy–Littlewood maximal operator on finite graphs, J. Math. Anal. Appl. 436 (2016), 661–682.

    Article  MathSciNet  MATH  Google Scholar 

  23. E. M. Stein, Harmonic Analysis: Real-Variable Methods, Orthogonality, and Oscillatory Integrals, Princeton Mathematical Series, Vol. 43, Princeton University Press, Princeton, NJ, 1993.

    MATH  Google Scholar 

  24. E. M. Stein and S. Wainger, Discrete analogues in harmonic analysis. II. Fractional integration, J. Anal. Math. 80 (2000), 335–355.

    Article  MathSciNet  MATH  Google Scholar 

  25. K. Stempak, Modified Hardy–Littlewood maximal operators on nondoubling metric measure spaces, Ann. Acad. Sci. Fenn. Math. 40 (2015), 443–448.

    Article  MathSciNet  MATH  Google Scholar 

  26. T. Tao, Expansion in Finite Simple Groups of Lie Type, Graduate Studies in Mathematics, Vol. 164, American Mathematical Society, Providence, RI, 2015.

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics and Computer Science, University of Barcelona, Gran via 585, E-08007, Barcelona, Spain

    Javier Soria

  2. Instituto de Ciencias Matematicas (CSIC-UAM-UC3M-UCM), Consejo Superior de Investigaciones Cientificas, Nicolas Cabrera, 13–15, Campus de Cantoblanco UAM, 28049, Madrid, Spain

    Pedro Tradacete

Authors
  1. Javier Soria
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Pedro Tradacete
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Pedro Tradacete.

Additional information

The first author has been partially supported by the Spanish Government grant MTM2016-75196-P (MINECO / FEDER, UE) and the Catalan Autonomous Government grant 2017SGR358.

The second author has been partially supported by the Spanish Government grants MTM2016-75196-P, MTM2016-76808-P, Grupo UCM 910346 and the “Severo Ochoa Programme for Centres of Excellence in R&D” (SEV-2015-0554).

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Soria, J., Tradacete, P. Geometric properties of infinite graphs and the Hardy–Littlewood maximal operator. JAMA 137, 913–937 (2019). https://doi.org/10.1007/s11854-019-0019-5

Download citation

  • Received:

  • Revised:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11854-019-0019-5

Search

Navigation