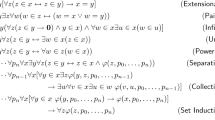Abstract
Category theory is often treated as an algebraic foundation for mathematics, and the widely known algebraization of ZF set theory in terms of this discipline is referenced as “categorical set theory” or “set theory for category theory”. The method of algebraization used in this theory has not been formulated in terms of universal algebra so far. In current paper, a universal algebraic method, i.e. one formulated in terms of universal algebra, is presented and used for algebraization of a ground mereological system described as “quantitative” (QMS) and of the standard set theories built upon it. The QMS is algebraized by using abelian generalized groups (AGGs) generalizing both abelian groups and Boolean algebras. To algebraize set theory, the AGG are enriched with operators to produce extensional algebras (EA) of Tarskian “Boolean algebras with operators” type. EAs explicate the intuition of set theory’ universes (of discourse). The axiomatic set theory presented in this paper, named extension theory, previously went through an intuitive phase of development during its use by the author in computer science projects, where it served as the basis for development of a foundational data science framework and an extensional model of natural languages also outlined here.



Similar content being viewed by others
References
Drugus, I.: A wholebrain approach to the web. In: IEEE proceeding of web intelligence and intelligent agent technology conference (2007), Silicon Valley, pp. 68–71
Drugus, I.: Universics: a common formalization framework for brain informatics and semantic web. Web Intell. Intell Agents 55-78 (2010)
Drugus, I.: PML: a punctuation symbolism for semantic markup. In: 11th international conference Linguistic resources and tools for processing the Romanian language, ConsILR-2015, 26-27 Nov. 2015, Iasi, Romania., pp. 79-92 (2015)
Drugus, I.: Towards an algebraic set theory for the formalization of data structures. In: proceedings of conference on mathematical foundations of informatics. July 2-6, 2018, Chisinau, Moldova, pp. 73-89
Drugus, I.: Generalized Boolean algebras as single composition systems for measure theory. In: proceedings of the 4th conference of mathematical society of Moldova (CMSM-2017), June 28 – July 2, 2017, Chisinau, Moldova, pp. 75–78 (2017)
Drugus, I.: Metalingua: a language to mediate communication with semantic web in natural languages. In: Thaung, K.S. (ed.) Advanced information technology in education, AISC 126, pp. 109–115. Springer, Berlin (2012)
Drugus, I., Skobelev, V.: Imbrication algebras - algebraic structures of nesting order. Comput. Sci. J. Moldova 26(3), 233–249 (2018)
Drugus, I., Skobelev, V.: Some Developments of aggregate theory.In: proceedings of conference on mathematical foundations of informatics. July 3-6, Iasi, Romania, pp. 73-89, 2019
Joyal, A., Moerdijk, I.: Algebraic set theory. Cambridge University Press, Cambridge (1995)
Awodey, S.: A brief introduction to algebraic set theory. Bull. Symbol. Logic 14(3), 281–298 (2008)
MacLane, S., Moerdijk, I.: Sheaves in Geometry and Logic, a First Introduction to Topos Theory. Springer, Berlin (1994)
Adámek, J., Rosický, J., Vitale, E.M.: Algebraic Theories, a Categorical Introduction to General Algebra. Cambridge University Press, Cambridge (2008)
Veblen, O.: A system of axioms for geometry. Trans. Amer. Math. Soc. 5, 343–384 (1904)
Burris, S., Sankappanavar, H.P.: A course in universal algebra (Graduate Texts in Mathematics, vol. 78), 1st ed., New-York, Heidelberg, Berlin: Springer-Verlag, 276 p. ISBN: 0-387-90578-2 (1981)
Abbott, J.C.: Semi-Boolean algebras. Matematicki Vesnik 4(19), 177–198 (1967)
Stone, M.H.: Postulates for Boolean algebras and generalized Boolean algebras. Am. J. Math. 57(4), 703–732 (1935)
Horn, A., Tarski, A.: Measures in Boolean algebras. Trans. Amer. Math. Soc. 64, 467–497 (1948)
Tarski, A., Givant, S.: A Formalization of Set Theory without Variables. American Mathematical Society, RI (1987)
Bernays, P.: A system of axiomatic set theory-part I. J. Symbol. Logic Assoc. Symbol. Logic 2(1), 65–77 (1937)
Kirby, L.: Finitary set theory. Notre Dame J. Formal Logic 50(3), (2009)
Boolos, G.: Logic. Harvard University Press, Cambridge (1998)
Szmielew, W., A. Tarski, A.: Mutual interpretability of some essentially undecidable theories, In: proceedings of international congress of mathematicians (Cambridge, Massachussetts.,1950), vol. 1., American Mathematical Society, Providence, R. I., p.734 (1952)
Burgess, J.: Fixing Frege. Princeton University Press, Princeton (2005)
Vaught, R.L.: On a theorem of Cobham concerning undecidable theories. In: Nagel, E., Suppes, P., Tarski, A. (eds.) Logic, methodology and philosophy of science, pp. 14–25. Elsevier, London (1960)
Lewis, D.: Parts of Classes. Blackwell, Oxford (1991)
Jónsson, B., Tarski, A.: On two properties of free algebras. Mathematica Scandinavica 9, 95–101 (1961)
Bourbaki, N.: Elements of Mathematics: theory of sets. Hermann, Paris (1968)
Nikitchenko, M.S., Shkilniak, S.S.: Algebras of quasiary relations, theoretical and applied aspects of program systems development. In: TAAPSD’2014: 11th international conference, pp. 174–181. Ukraine, Kiev (2014)
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
About this article
Cite this article
Drugus, I. A Universal Algebraic Set Theory Built on Mereology with Applications. Log. Univers. 16, 253–283 (2022). https://doi.org/10.1007/s11787-022-00295-8
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11787-022-00295-8