Skip to main content
SpringerLink
Log in

Scale and time effects on mathematical models for transport in the environment

  • Published:
Applied Mathematics-A Journal of Chinese Universities Aims and scope Submit manuscript

Abstract

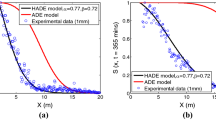
The purpose of this paper is to analyse mathematical models used in environmental modelling. Following a brief survey of the development in modelling scale-and time-dependent dispersion processes in the environment, this paper compares three similarity solutions, one of which is a solution of the generalized Feller equation (GF) with fractal parameters, and the other two for the newly-developed generalized Fokker-Planck equation (GFP). The three solutions are derived with parameters having physical significance. Data from field experiments are used to verify the solutions. The analyses indicate that the solutions of both GF and GFP represent the physically meaningful natural processes, and simulate the realistic shapes of tracer breakthrough curves.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Barry D A, Sposito G. Analytical solution of a convection-dispersion model with time-dependent transport coefficients, Water Resour Res, 1989, 25: 2407–2416.

    Google Scholar 

  2. Barry D A. Time dependence of solute dispersion in aquifers. In: R Fell, T Phillips and C Gerrard eds, Geomechanical Management of Waste and Contamination, Rotterdam: Balkema, 1993, 329–334.

    Google Scholar 

  3. Batchelor G K. Diffusion in a field of homogeneous turbulence, II, The relative motion of particles, Proc Cambridge Phil Soc, 1952, 48: 345–362.

    Article  MATH  MathSciNet  Google Scholar 

  4. Bear J. On the tensor form of dispersion, J Geophys Res, 1961, 66: 1185–1197.

    MathSciNet  Google Scholar 

  5. Bear J, Verruijt A. Modelling groundwater flow and pollution, Dordrecht: Reidel Publishing Company, 1987.

    Google Scholar 

  6. Carslaw H S, Jaeger J C. Conduction of Heat in Solids, 2nd ed, Clarendon: Oxford University Press, 1959.

    Google Scholar 

  7. Crank J A. Theoretical investigation of the influence of molecular relaxation and internal stress on diffusion in polymers, J Polymer Sci, 1953, 11(2): 151–168.

    Article  Google Scholar 

  8. Day P R. Dispersion of a moving salt water boundary, Trans Amer Geophys Un, 1956, 37:595–601.

    Google Scholar 

  9. de Josselin de Jong G. Longitudinal and transverse diffusion in granular deposits, Trans Amer Geophys Un, 1958, 39: 67–74.

    Google Scholar 

  10. de Marsily G. Quantitative hydrogeology: Groundwater Hydrology for Engineers, California, San Diego: Academic Press, 1986.

    Google Scholar 

  11. Dieulin A, Matheron G, de Marsily G, et al. Time dependence of an “equivalent dispersion coefficient” for transport in porous media, In: A Verruijt, F B J Barends eds, Proc Euromech, 143, Rotterdam: Balkema, 1981, 199–202.

    Google Scholar 

  12. Frisch H L, Wang T T, Kwei T K. Diffusion in glassy polymers, J Polymer Sci, Part A-2, 1969, 7: 879–887.

    Article  Google Scholar 

  13. Frisch U. Turbulence: The Legacy of A N Kolmogorov, Cambridge: Cambridge University Press, 1995.

    MATH  Google Scholar 

  14. Gelhar L W, Mantoglou A, Welty C, et al. A review of field scale physical solute transport processes in saturated and unsaturated porous media, Rep EA-4190, Calif, Palo Alto: Electro Power Res Inst, 1985.

    Google Scholar 

  15. Gelhar L W, Welty C, Rehfeldt K R. A critical review of data on field-scale dispersion in aquifers, Water Resour Res, 1992, 28(7): 1955–1974.

    Article  Google Scholar 

  16. Graf W H. Hydraulics of Sediment Transport, Colorado, Littleton: Water Resour Publ, 1984, 513.

    Google Scholar 

  17. Hentschel H G E, Procaccia I. Relative diffusion in turbulent media: The fractal dimension of clouds, Phys Rev A, 1984, 29: 1461–1470.

    Article  MathSciNet  Google Scholar 

  18. Klafter J, Bluman A, Shlesinger M F. Stochastic pathway to anomalous diffusion, Phys Rev A, 1987, 35, 3081–3085.

    Article  MathSciNet  Google Scholar 

  19. Kolmogorov A N. The local structure of turbulence in incompressible viscous fluid for very large Reynolds number, SSSR Dokl Akad Nauk, 1941a, 30: 9–13.

    Google Scholar 

  20. Kolmogorov A N. On degeneration (decay) of isotropic turbulence in an incompressible viscous liquid, SSSR Dokl Akad Nauk, 1941b, 31: 538–540.

    Google Scholar 

  21. Kolmogorov A N. Dissipation of energy in locally isotropic turbulence, SSSR Dokl Akad Nauk, 1941c, 32: 16–18.

    Google Scholar 

  22. Lallemand-Barrs A, Peaudecerf P. Recherch des relations entre les valeurs mesures de la dispersivit macroscopique d’un millieu aquifre, ses autres caractristiques, et les conditions de mesure, Bull Bur Rech Geol Min Fr, Sect, 1978, 3(4): 277–284.

    Google Scholar 

  23. Lehnigk, S H. The generalized Feller equation and related topics, England, Harlow, Essex: Longman, 1993.

    MATH  Google Scholar 

  24. Leibenzon L S. Gas movement in a porous medium (in Russian), Neft i Slants Khoz, 1929, 10:497–519.

    Google Scholar 

  25. Lovejoy S. Area-perimeter relation for rain and cloud areas, Science, 1982, 216: 185–187.

    Article  Google Scholar 

  26. Muralidhar R, Ramkrishna D. Diffusion in pore fractals: A review of linear response models, Transp Porous Media, 1993, 13: 79–95.

    Article  Google Scholar 

  27. Muskat M. The flow of homogeneous fluids through porous media, NY: McGraw-Hill, 1937.

    MATH  Google Scholar 

  28. Nikolaevskii V N, Konvektivnaya diffusiya v poristykh sredakh Prikl Mat Mekh, 1959, 23: 1042–1050.

    MathSciNet  Google Scholar 

  29. O’shaughnessy B, Procaccia I. Analytical solutions for diffusion on fractal objects, Phys Rev Lett, 1985, 54: 455–458.

    Article  Google Scholar 

  30. Pang L, Hunt B. Solutions and verification of a scale dependent dispersion model, J Contam Hydrol, 2001, 53: 21–29.

    Article  Google Scholar 

  31. Philip J R. Theory of infiltration, Adv Hydrosci,1969, 5: 215–296.

    Google Scholar 

  32. Philip J R. Issues in flow and transport in heterogeneous porous media, Transp Porous Media, 1986, 1: 319–338.

    Article  Google Scholar 

  33. Pickens J F, Grisak G E. Modeling of scale-dependent dispersion in hydrogeologic systems, Water Resour Res, 1981, 17: 1701–1711.

    Google Scholar 

  34. Richardson L F. Atmospheric diffusion shown on a distance-neighbour graph, Proc Roy Soc London Ser A, 1926, 110: 709–737.

    Article  Google Scholar 

  35. Risken H. The Fokker-Planck equation, 2nd ed, Berlin: Springer-Verlag, 1996.

    MATH  Google Scholar 

  36. Saffman P G. A theory of dispersion in a porous medium, J Fluid Mech, 1959, 6: 321–349.

    Article  MathSciNet  Google Scholar 

  37. Scheidegger A E. Statistical hydrodynamics in porous media, J Appl Phys, 1954, 25: 994–1001.

    Article  MATH  MathSciNet  Google Scholar 

  38. Scheidegger A E. General theory of dispersion in porous media, J Geophys Res,1961, 66: 3273–3278.

    Article  Google Scholar 

  39. Scheidegger A E. Statistical hydrodynamics in porous media, Adv Hydrosci,1964, 1: 161–181.

    Google Scholar 

  40. Slichter C S. Field measurement of the rate of movement of underground waters, USGS Water Supply Paper, 1905, 140.

  41. Stephenson J. Some non-linear diffusion equations and fractal diffusion, Physica A, 1995, 222:234–247.

    Article  Google Scholar 

  42. Su N H. Development of the Fokker-Planck equation and its solutions for modeling transport of conservative and reactive solutes in physically heterogeneous media, Water Resour Res, 1995, 31(12): 3025–3032.

    Article  Google Scholar 

  43. Su N H. Extension of the Feller Fokker Planck equation to two and three dimensions for modeling solute transport in fractal porous media, Water Resour Res, 1997, 33(5): 1195–1197.

    Article  Google Scholar 

  44. Su N H, Sander G C, Liu F, et al. Similarity solutions for solute transport in fractal porous media with a time-and scale-dependent dispersivity, Applied Math Model, 2005, 29(9): 852–870.

    Article  MATH  Google Scholar 

  45. Taylor G I. Diffusion by continuous movements, Proc London Math Soc, 1921, 20: 196–211.

    Article  Google Scholar 

  46. Taylor G I. Dispersion of soluble matter in solvent flowing slowly through a tube, Proc Royal Soc London A, 1953, 219: 186–203.

    Article  Google Scholar 

  47. Tyler S W, Wheatcraft S W. Reply to J R Philip’s comment on “An explanation of scaledependent dispersivity in heterogeneous aquifers using concepts of fractal geometry” Water Resour Res, 1992, 28(5): 1487–1490.

    Article  Google Scholar 

  48. Wang T T, Kwei T K, Frisch H L. Diffusion in glassy polymers, III, J Polymer Sci, Part A-2, 1969, 7: 2019–2028.

    Article  Google Scholar 

  49. Wheatcraft S W, Tyler S W. An explanation of scale-dependent dispersivity in heterogeneous aquifers using concepts of fractal geometry, Water Resour Res, 1988, 24(4): 566–578.

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. School of Mathematics and Computational Science, Xiangtan University, Hunan, 411105, China

    Su Ninghu

  2. School of Earth and Environmental Sciences, James Cook University, Cairns, Queensland, 4870, Australia

    Su Ninghu

  3. Department of Natural Resources and Water, Mareeba, Queensland, 4880, Australia

    Su Ninghu

Authors
  1. Su Ninghu
    View author publications

    You can also search for this author in PubMed Google Scholar

Additional information

Supported by the NNSF of China (30570426), Fok Ying Tung Education Foundation (101004) and the Youth Foundation of Educational Department of Hunan Province in China (05B007).

Rights and permissions

Reprints and permissions

About this article

Cite this article

Su, N. Scale and time effects on mathematical models for transport in the environment. Appl. Math. Chin. Univ. 22, 267–276 (2007). https://doi.org/10.1007/s11766-007-0303-5

Download citation

  • Received:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11766-007-0303-5

MR Subject Classification

Keywords

Search

Navigation