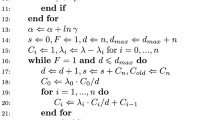Abstract
Several concepts of generalized orderings and generalized ageing classes have been considered in the literature (see Fagiuoli and Pellerey, 1993, Nav Res Logist 40: 829–842). They have been used in reliability, economics and actuarial sciences. These generalized notions provide a knowledge of the intrinsic structure of the ageing and ordering properties of random variables and have become an important tool in applied probability.
In this paper, we provide new results about generalized orderings of excess lifetimes at different times of a renewal process when the underlying distribution belongs to some generalized ageing class. We also derive some interpretations and give some discussions of these results.
Similar content being viewed by others
References
Barlow R, Proschan F (1975) Statistical theory of reliability and life testing: Probability models. Holt, Rinehart and Winston, New York
Belzunce F, Ortega EM, Ruiz JM (1999) The Laplace order and ordering of residual lives. Stat Probab Lett 42:145–156
Belzunce F, Ortega EM, Ruiz JM (2001) A note on stochastic comparisons of excess lifetimes of renewal processes. J Appl Probab 38:747–753
Cao J, Wang Y (1991) The NBUC and NWUC classes of life distributions. J Appl Probab 28:473–479
Chen Y (1994) Classes of life distributions and renewal counting processes. J Appl Probab 31:1110–1115
Denuit M, Lefèvre C, Shaked M (1998) The s-convex orders among real random variables, with applications. Math Inequalities Appl 1:585–613
Deshpande JV, Kochar SC, Singh H (1986) Aspects of positive ageing. J Appl Probab 23:748–758
Fagiuoli E, Pellerey F (1993) New partial orderings and applications. Nav Res Logist 40:829–842
Fagiuoli E, Pellerey F (1994) Preservation of certain classes of life distributions under Poisson shock models. J Appl Probab 31:458–465
Hu T, Kundu A, Nanda AK (2001) On generalized orderings and ageing properties with their implications. In: Hayakawa Y, Irony T, Xie M (eds) System and Bayesian reliability. Series on quality, reliability and engineering statistics, vol 5. World Scientific, Singapore, pp 199–228
Hu T, Ma M, Nanda AK (2004a) Characterizations of generalized ageing classes by the excess lifetime. Southeast Asian Bull Math 28:279–285
Hu T, Nanda AK, Xie H, Zhu Z (2004b) Properties of some stochastic orders: a unified study. Nav Res Logist 51:193–216
Kaas R, van Heerwaarden AE, Goovaerts MJ (1994) Ordering of actuarial risks. Caire education series. Brussels
Karlin S (1968) Total positivity. Standford University Press, Standford
Li X, Kochar SC (2001) Some new results involving the NBU(2) class of life distributions. J Appl Probab 38:242–246
Li X, Li Z, Jing B (2000) Some results about NBUC class of life distributions. Stat Probab Lett 46:229–237
Mukherjee SP, Chatterjee A (1992) Stochastic dominance of higher orders and its implications. Commun Stat Theory Methods 21:1977–1986
Nanda AK (1997) On improvement and deterioration of a repairable system. IAPQR Trans 22:107–113
Nanda AK, Jain K, Singh H (1996a) On closure of some partial orderings under mixtures. J Appl Probab 33:698–706
Nanda AK, Jain K, Singh H (1996b) Properties of moments for s-order equilibrium distributions. J Appl Probab 33:1108–1111
Ross S (1996) Stochastic processes, 2nd edn. Wiley, New York
Shaked M, Shanthikumar JG (1994) Stochastic orders and their applications. Academic, San Diego
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Belzunce, F., Nanda, A.K., Ortega, EM. et al. Generalized orderings of excess lifetimes of renewal processes. TEST 17, 297–310 (2008). https://doi.org/10.1007/s11749-006-0033-7
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11749-006-0033-7