Skip to main content
SpringerLink
Log in

Simulation of Precipitation Kinetics with Non-Spherical Particles

  • Published:
Journal of Phase Equilibria and Diffusion Aims and scope Submit manuscript

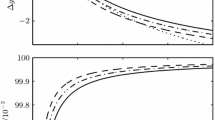
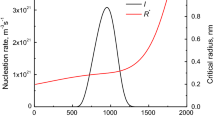
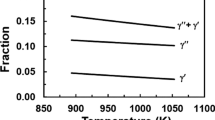
Abstract

A kinetic model that is modified based on Langer and Schwartz theory has been developed to treat precipitation kinetics of non-spherical precipitates. Three types of particle morphology, cuboid, needle and plate, have been treated. Explicitly accounting for coherent elastic strain energy and interfacial energy anisotropy, the model enables the prediction of equilibrium particle morphology. The shape effect on growth kinetics has also been investigated assuming quasi steady-state and shape preserving conditions. Validations against a couple of practical examples have shown reasonable agreements, though care must be taken due to uncertainties from model limitations and discrepancies in experimental data.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7

Similar content being viewed by others

References

  1. CALPHAD. www.calphad.org. Accessed 2018

  2. L. Kaufman and H. Bernstein, Computer Calculation of Phase Diagrams, Academic Press, New York, 1970

    Google Scholar 

  3. N. Saunders and A.P. Miodownik, CALPHAD (Calculation of Phase Diagrams): A Comperhensive Guide, Elsevier Science Ltd., New York, 1998

    Google Scholar 

  4. J.S. Langer and A.J. Schwartz, Kinetics of Nucleation in Near-Critical Fluids, Phys. Rev. A, 1980, 21(3), p 948-958

    Article  ADS  MathSciNet  Google Scholar 

  5. I.M. Lifshitz and V.V. Slyozov, The Kinetics of Precipitation from Supersaturated Solid Solutions, J. Phys. Chem. Solids, 1961, 15, p 35

    Article  Google Scholar 

  6. C. Wagner, Theorie Der Alterung Von Niederschlägen Durch Umlösen (Ostwald-Reifung), Z. Elektrochem., 1961, 65, p 581

    Google Scholar 

  7. TC-PRISMA. http://www.thermocalc.com/products-services/software/precipitation-module-(tc-prisma). Accessed 2018

  8. F.S. Ham, Theory of Diffusion-Limited Precipitation, J. Phys. Chem. Solids, 1958, 6(4), p 335-351

    Article  ADS  MathSciNet  Google Scholar 

  9. F.S. Ham, Shape-Preserving Solutions of the Time-Dependent Diffusion Equation, Q. Appl. Math., 1959, 17(2), p 137-145

    Article  MathSciNet  Google Scholar 

  10. J.D. Boyd and R.B. Nicholson, The Coarsening Behaviour of θ” and θ’ Precipitates in Two Al-Cu Alloys, Acta Metall., 1971, 19(12), p 1379-1391

    Article  Google Scholar 

  11. Q. Du, B. Holmedal, J. Friis, and C.D. Marioara, Precipitation of Non-Spherical Particles in Aluminum Alloys Part II: Numerical Simulation and Experimental Characterization During Aging Treatment of an Al-Mg-Si Alloy, Metall. Mater. Trans. A, 2016, 47(1), p 589-599

    Article  Google Scholar 

  12. B. Holmedal, E. Osmundsen, and Q. Du, Precipitation of Non-Spherical Particles in Aluminum Alloys Part I: generalization of the Kampmann-Wagner Numerical Model, Metall. Mater. Trans. A, 2016, 47(1), p 581-588

    Article  Google Scholar 

  13. Precipitation Module (TC-PRISMA) User Guide. http://www.thermocalc.com/media/40970/precipitation-module-tc-prisma-user-guide.pdf. Accessed 2018

  14. R. Kampmann and R. Wagner, Kinetics of Precipitation in Metastable Binary Alloys—Theory and Application to Cu-1.9 at% Ti and Ni-14 at% Al, Decomposition of Alloys: The Early Stages, ed. by P. Haasen, V. Gerold, R. Wagner, and M.F. Ashby, Pergamon, 1984, pp. 91-103

  15. D. Kashchiev, Nucleation, 1st ed., Butterworth-Heinemann, Oxford, 2000

    Google Scholar 

  16. K.C. Russel, Nucleation in Solids: The Induction and Steady State Effects, Adv. Colloid Interface Sci., 1980, 13(3–4), p 205-318

    Article  Google Scholar 

  17. H.I. Aaronson and J.K. Lee, The Kinetic Equations of Solid- > Solid Nucleation Theory and Comparisions with Experimental Observations, Lectures on the Theory of Phase Transformations, 2nd edn., ed. by H.I. Aaronson, The Minerals, Metals & Materials Society, Warrendale, PA, 1999, p 165-230

  18. C.A. Wert and C. Zener, Interference of Growing Spherical Precipitate Particles, J. Appl. Phys., 1950, 21, p 5-8

    Article  ADS  Google Scholar 

  19. W.C. Johnson, Influence of Elastic Stress on Phase Transformations, Lectures on the Theory of Phase Transformations, 2nd edn., ed. by H.B. Aaron, The Minerals, Metals & Materials Society, Warrendale, PA, USA, 1999, p 35-134

  20. S. Onaka, N. Kobayashi, T. Fujii, and M. Kato, Energy Analysis with a Superspherical Shape Approximation on the Spherical to Cubical Shape Transitions of Coherent Precipitates in Cubic Materials, Mater. Sci. Eng. A, 2003, 347(1), p 42-49

    Article  Google Scholar 

  21. A.G. Khachaturyan, Theory of Structural Transformations in Solids, Wiley, New York, 1983

    Google Scholar 

  22. J.D. Eshelby, The Determination of the Elastic Field of an Ellipsoidal Inclusion, and Relative Problems, Proc. R. Soc. A Math. Phys. Eng. Sci., 1957, 241(1226), p 376-396

    Article  ADS  MathSciNet  Google Scholar 

  23. J.D. Eshelby, The Elastic Field Outside an Ellipsoidal Inclusion, Proc. R. Soc. A Math. Phys. Eng. Sci., 1959, 252(1271), p 561-569

    Article  ADS  MathSciNet  Google Scholar 

  24. C. Weinberger, W. Cai, and D. Barnett, ME340B Lecture Notes—Elasticity of Microscopic Structures, Stanford Univeristy, 2005. http://micro.stanford.edu/~caiwei/me340b/content/me340b-notes_v01.pdf

  25. C.A. Johnson, Generalization of the Gibbs–Thomson Equation, Surf. Sci., 1965, 3(5), p 429-444

    Article  ADS  Google Scholar 

  26. G.A. Korn and T.M. Korn, Mathematical Handbook for Scientists and Engineers, McGraw-Hill, New York, 1961

    MATH  Google Scholar 

  27. Y. Tang, Q. Chen, and A. Engström, Kinetic Simulations of Diffusion-Controlled Phase Transformations in Cu-Based Alloys, Diffus. Found., 2018, 15, p 1-22

    Article  Google Scholar 

  28. W.A. Soffa and D.E. Laughlin, High-Strength Age Hardening Copper-Titanium Alloys: Redivivus, Prog. Mater Sci., 2004, 49(3), p 347-366

    Article  Google Scholar 

  29. R. Kampmann, H. Eckerlebe, and R. Wagner, Precipitation Kinetics in Metastable Solid Solutions—Theoretical Considerations and Application to Cu-Ti Alloys, MRS Proc., 1985, 57, p 525

    Article  Google Scholar 

  30. C. Borchers, Catastrophic Nucleation During Decomposition of Cu-0.9at.% Ti, Philos. Mag. A, 1999, 79(3), p 537-547

    Article  ADS  Google Scholar 

  31. J.K. Lee, D.M. Barnett, and H.I. Aaronson, The Elastic Strain Energy of Coherent Ellipsoidal Precipitates in Anisotropic Crystalline Solids, Metall. Trans. A, 1977, 8(6), p 963-970

    Article  Google Scholar 

  32. L.V. Alvensleben and R. Wagner, FIM-Atom Probe Studies of Early Stage Decomposition in Cu-Ti Alloys, Decomposition of Alloys: The Early Stages, Pergamon, 1984, pp. 143–148

    Chapter  Google Scholar 

  33. M. Sundararaman, P. Mukhopadhyay, and S. Banerjee, Some Aspects of the Precipitation of Metastable Intermetallic Phases in Inconel 718, Metall. Trans. A, 1992, 23(7), p 2015-2028

    Article  Google Scholar 

  34. A.K. Koul, P. Au, N. Bellinger, R. Thamburaj, W. Wallace, and J.P. Immarigeon, Development of a Damage Tolerant Microstructure for Inconel 718 Turbine Disc Material, Superalloys 1988, ed. by S. Reichman, D.N. Duhl, G. Maurer, S. Antolovich, C. Lund, Seven Springs, PA, TMS Society, 1988, pp. 3–12

  35. A. Devaux, L. Nazé, R. Molins, A. Pineau, A. Organista, J.Y. Guédou, J.F. Uginet, and P. Héritier, Gamma Double Prime Precipitation Kinetic in Alloy 718, Mater. Sci. Eng. A, 2008, 486(1), p 117-122

    Article  Google Scholar 

  36. Y.-F. Han, P. Deb, and M.C. Chaturvedi, Coarsening Behavior of γ‘‘- and γ‘- Particles in Inconel Alloy 718, Metal Sci., 1982, 16(12), p 555-561

    Article  Google Scholar 

  37. R. Cozar and A. Pineau, Influence of Coherency Strains on Precipitate Shape in a Fe-Ni-Ta Alloy, Scr. Metall., 1973, 7(8), p 851-854

    Article  Google Scholar 

  38. T.M. Smith, C.K. Sudbrack, P. Bonacuse, and R. Rogers, Microstructural Characterization and Modeling of SLM Superalloy 718. https://ntrs.nasa.gov/search.jsp?R=20180000817. Accessed 2017

Download references

Acknowledgment

The first author (KW) would like to thank Dr. Qiang Du of SINTEF Materials and Chemistry, Trondheim, Norway for the valuable discussions. The theoretical guidance from QuestTek LLC through the close collaboration is also greatly appreciated. In celebrating his 80th birthday, the authors (KW and QC) are deeply grateful to Prof. Zhanpeng Jin, a lifetime mentor, for his continuing guidance and support throughout our career.

Author information

Authors and Affiliations

  1. Thermo-Calc Software Inc., 4160 Washington Rd Ste 230, Pittsburgh, PA, 15317, USA

    Kaisheng Wu & Paul Mason

  2. Thermo-Calc Software AB, Råsundavägen 18, 169 67, Solna, Sweden

    Qing Chen

Authors
  1. Kaisheng Wu
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Qing Chen
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Paul Mason
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Kaisheng Wu.

Additional information

This invited article is part of a special issue of the Journal of Phase Equilibria and Diffusion in honor of Prof. Zhanpeng Jin’s 80th birthday. The special issue was organized by Prof. Ji-Cheng (JC) Zhao, The Ohio State University; Dr. Qing Chen, Thermo-Calc Software AB; and Prof. Yong Du, Central South University.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Wu, K., Chen, Q. & Mason, P. Simulation of Precipitation Kinetics with Non-Spherical Particles. J. Phase Equilib. Diffus. 39, 571–583 (2018). https://doi.org/10.1007/s11669-018-0644-1

Download citation

  • Received:

  • Revised:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11669-018-0644-1

Keywords

Search

Navigation