Skip to main content
SpringerLink
Log in

Noise Models in Numerical Analysis of Stochastic Creep Damage Growth and Residual Life Assessment

  • Techical Article---Peer Reviewed
  • Published:
Journal of Failure Analysis and Prevention Aims and scope Submit manuscript

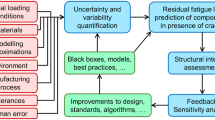
Abstract

Experimental observations of creep life in structural systems exhibit significant scatter. As a result, probabilistic methods that incorporate the associated uncertainties in residual life assessment methodologies have been developed. Most studies in the literature adopt Gaussian models for the noise. However, Monte Carlo simulations with such models lead to possibilities of physically unrealistic negative damage growth increments in certain sample damage growth realizations. This study investigates the use of alternative models for noise in damage growth analyses and compares these predictions with those obtained when Gaussian models are used. A continuum damage mechanics based approach is used for obtaining the thermal creep damage growth in a nuclear power plant component. Numerical results are presented to highlight the salient features arising from this study.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7

Similar content being viewed by others

References

  1. E. Van der Giessen, V. Tvergaard, Effect of random variations in microstructure on the development of final creep failure in polycrystalline aggregates. Modell. Simul. Mater. Sci. Eng. 2, 721–738 (1994)

    Article  Google Scholar 

  2. E. Van der Giessen, V. Tvergaard, Development of final creep failures in polycrystalline aggregates. Acta Met. Mat. 42(3), 959–973 (1994)

    Article  Google Scholar 

  3. H.T. Yao, F.Z. Xuan, Z. Wang, S.T. Tu, A review of creep analysis and design under multi-axial stress states. Nucl. Eng. Des. 237, 1969–1986 (2007)

    Article  Google Scholar 

  4. C.W. Woo, D.L. Li, Statistical analysis of material damage with changing internal structure. Eng. Fract. Mech. 45(2), 245–254 (1993)

    Article  Google Scholar 

  5. C.W. Woo, D.L. Li, A general stochastic dynamic model of continuum damage mechanics. Int. J. Solids Struct. 29, 2921–2932 (1992)

    Article  Google Scholar 

  6. B. Bhattacharya, B. Ellingwood, A CDM analysis of stochastic ductile damage growth and reliability. Probab. Eng. Mech. 14, 45–54 (1999)

    Article  Google Scholar 

  7. B. Bhattacharya, B. Ellingwood, Continuum damage mechanics based model of stochastic damage growth. ASCE J. Eng. Mech. 124(9), 1000–1009 (1998)

    Article  Google Scholar 

  8. J.T. Boyle, J. Spence, Stress Analysis for Creep (Butterworths Co., London, 1983)

    Google Scholar 

  9. T. Kitamura, L.J. Ghosn, R. Ohtani, Stochastic modeling of crack initiation and short crack growth under creep and creep fatigue conditions. ASME J. Appl. Mech. 59, S35–S42 (1992)

    Article  Google Scholar 

  10. A.G. Miller, Crack propagation due to random thermal fluctuations: effect of temporal incoherence. Int. J. Press. Vessel Pip. 8, 15–24 (1980)

    Article  Google Scholar 

  11. W.N. Huang, R.A. Valentin, The effect of random temperature fluctuations on creep and creep rupture of cylindrical tubes. Nucl. Eng. Des. 28, 289–298 (1974)

    Article  Google Scholar 

  12. F.A. Cozzarelli, W.N. Huang, Effect of random material parameters on nonlinear steady creep solutions. Int. J. Solids Struct. 7, 1477–1494 (1971)

    Article  Google Scholar 

  13. D.G. Harlow, T.J. Delph, A probabilistic model for creep-fatigue failure. ASME J. Press. Vessel Technol. 119, 45–51 (1997)

    Article  Google Scholar 

  14. C. Zhou, S. Tu, A stochastic computation model for the creep damage of furnace tube. Int. J. Press. Vessel Pip. 78, 617–625 (2001)

    Article  Google Scholar 

  15. K.M. Nikbin, M. Yatomi, K. Wasmer, G.A. Webster, Probabilistic analysis of creep crack initiation and growth in pipe components. Int. J. Press. Vessel Pip. 80, 585–595 (2003)

    Article  Google Scholar 

  16. H. Mao, S. Mahadevan, Reliability analysis of creep-fatigue failure. Int. J. Fatigue 22, 789–797 (2000)

    Article  Google Scholar 

  17. P.E. Kloden, E. Platen, Numerical Solutions of Stochastic Differential Equations (Springer, New York, 1992)

    Book  Google Scholar 

  18. C. Kim, E.K. Lee, P. Hanggi, P. Talkner. Numerical method for solving stochastic differential equations with Poisson white shot noise. Phys. Rev. E. 76 (2007)

Download references

Acknowledgement

The work was supported from the project sponsored by the Board of Research in Nuclear Sciences, Department of Atomic Energy, Government of India.

Author information

Authors and Affiliations

  1. Department of Applied Mechanics, Indian Institute of Technology Madras, Chennai, 600 036, India

    Yash Vyas, Y. Appalanaidu & Sayan Gupta

Authors
  1. Yash Vyas
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Y. Appalanaidu
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Sayan Gupta
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Sayan Gupta.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Vyas, Y., Appalanaidu, Y. & Gupta, S. Noise Models in Numerical Analysis of Stochastic Creep Damage Growth and Residual Life Assessment. J Fail. Anal. and Preven. 14, 259–264 (2014). https://doi.org/10.1007/s11668-014-9792-8

Download citation

  • Received:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11668-014-9792-8

Keywords

Search

Navigation