Modeling the Spread of Zika Virus in a Stage-Structured Population: Effect of Sexual Transmission

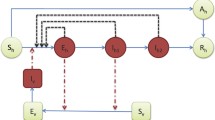
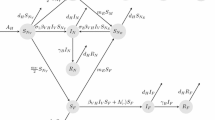
Abstract

The recent Zika virus outbreak has been spreading rapidly all over the world, expanding its traditionally geographical affected regions, making it a global public health hazard and endangering millions of people. One unique property of the Zika virus compared to most vector-borne diseases is the fact that the virus is transmitted both by mosquitoes and by direct sexual contact. In the present manuscript, we formulate and analyze five mathematical compartmental models of Zika transmission. We model both transmission routes (i.e., vector-borne and sexual transmission). In order to make the model more realistic, heterogeneity in the sexual transmission is modeled in several ways. We fitted the five different models to data, inferred the parameters and selected the most appropriated model, which describes the Zika outbreak in Columbia. For all the models, we estimate the reproduction numbers, namely direct (sexual) transmission, vector transmission and the basic reproduction number $(R_0)$. The analysis revealed that the sexual transmission contribution to $R_0$ is highest [15.36% (95% CI 12.83–17.4)] for the model which stratifies each gender to high-risk and low-risk individuals in their sexual behavior. For this model, the estimated $R_0$ is 1.89 (95% CI 1.21–2.13), the direct transmission reproduction number is 0.42 (95% CI 0.29–0.64), and the vector transmission reproduction number is 1.51 (95% CI 1.23–1.87). The sensitivity analysis demonstrated that the value of $R_0$ depends on three controllable parameters: the biting rate, the sexual transmission rate and the average ratio of mosquito to human.

Appendices

Appendix A: Mathematical Analysis for the Model (2)

1.1 Positivity and Boundedness of Solutions for the Model (2)

Lemma

The closed and bounded set

$$\begin{aligned} \varOmega= & {} \Bigg \{\left( S_\mathrm{m}, S_\mathrm{f}, I_\mathrm{m}, I_\mathrm{np}, I_\mathrm{p}, R, S_\mathrm{v}, I_\mathrm{v}\right) \in \mathbb {R}^8_+ : S_\mathrm{m}+S_\mathrm{f}+I_\mathrm{m}+I_\mathrm{np}+I_\mathrm{p}+R+S_\mathrm{v}\\&+\,I_\mathrm{v} \le N_\mathrm{h}(0); S_\mathrm{v} + I_\mathrm{v} \le N_\mathrm{v}(0)\Bigg \}, \end{aligned}$$

is positively invariant and attracting with respect to the Model (2).

Proof

The system (2) can be written as

$$\begin{aligned} \frac{\hbox {d}X}{\hbox {d}t} = AX + B \end{aligned}$$

where $X = \left( S_\mathrm{m}, S_\mathrm{f}, I_\mathrm{m}, I_\mathrm{np}, I_\mathrm{p}, R, S_\mathrm{v}, I_\mathrm{v}\right) ^T$. The matrix A is given by

$$\begin{aligned} A = \displaystyle \left[ \begin{array}{cccccccc} -\left( \mu _\mathrm{h}+\lambda _\mathrm{m}\right) &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 \\ 0 &{} -\left( \mu _\mathrm{h}+\lambda _\mathrm{f}\right) &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 \\ \lambda _\mathrm{m} &{} 0 &{} -\left( \mu _\mathrm{h}+\gamma \right) &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 \\ 0 &{} (1-p)\lambda _\mathrm{f} &{} 0 &{} -\left( \mu _\mathrm{h}+\gamma \right) &{} 0 &{} 0 &{} 0 &{} 0 \\ 0 &{} p\lambda _\mathrm{f} &{} 0 &{} 0 &{} -\left( \mu _\mathrm{h}+\gamma \right) &{} 0 &{} 0 &{} 0 \\ 0 &{} 0 &{} \gamma &{} \gamma &{} \gamma &{} -\mu _\mathrm{h} &{} 0 &{} 0 \\ 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} -\left( \mu _\mathrm{v}+\lambda _v\right) &{} 0 \\ 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} \lambda _v &{} -\mu _\mathrm{v} \end{array} \right] \end{aligned}$$

where $\lambda _\mathrm{m} = \frac{\beta _1\theta _1I_\mathrm{v}}{N_\mathrm{h}} + \frac{\beta _2\left( I_\mathrm{np}+I_\mathrm{p}\right) }{\rho N_\mathrm{h}}$, $\lambda _\mathrm{f} = \frac{\beta _1\theta _1I_\mathrm{v}}{N_\mathrm{h}} + \frac{\beta _2I_\mathrm{m}}{\rho N_\mathrm{h}}$ and $\lambda _v = \frac{\beta _1\theta _2\left( I_\mathrm{m}+I_\mathrm{np}+I_\mathrm{p}\right) }{N_\mathrm{h}}$.

The vector $B = \left( \frac{\mu _\mathrm{h}N_\mathrm{h}}{2}, \frac{\mu _\mathrm{h}N_\mathrm{h}}{2}, 0, 0, 0, 0, \mu _\mathrm{v}N_\mathrm{v}, 0\right) ^T$ is positive. Note that A(X) has all off diagonal entries nonnegative, i.e., A(X) is a Metzler matrix, for all $X\in \mathbb {R}^8_+$. Since $B\ge 0$ system (2) is positively invariant in $\mathbb {R}^8_+$ (Abate et al. 2009). Therefore, any trajectory of the system (2) starting from an initial state in $\mathbb {R}^8_+$ remains trapped forever in $\mathbb {R}^8_+$.

Adding the first six equations of the Model (2), we get

$$\begin{aligned} \frac{\hbox {d}N_\mathrm{h}}{\hbox {d}t} = 0. \end{aligned}$$

Therefore, as $t\rightarrow \infty$, $0\le N_\mathrm{h}(t) \le N_\mathrm{h}(0)$. Similarly, by adding last two equations of the Model (2), we get as $t\rightarrow \infty$, $0\le N_\mathrm{v}(t) \le N_\mathrm{v}(0)$.

Therefore, all mathematically and biologically feasible solutions of the model system (2) enter the region $\varOmega$, i.e., $\varOmega$ is attracting. Hence, it is now sufficient to study the dynamical properties of the Model (2) in $\varOmega$. $\square$

1.2 Disease-Free Equilibrium Stability

The Model (2) has the unique disease-free equilibrium $E_0 =\left( \frac{N_\mathrm{h}}{2}, \frac{N_\mathrm{h}}{2}, 0, 0, 0, 0,\right. \left. N_\mathrm{v}, 0\right)$. Now by Theorem 2 in Van den Driessche and Watmough (2002), the disease-free equilibrium $E_0$ is locally asymptotically stable if $R_0 < 1$, but unstable if $R_0 > 1$. The global stability of the disease-free equilibrium is given in the following theorem.

Theorem

In the region,

$$\begin{aligned} \varOmega _1= & {} \Bigg \{\left( S_\mathrm{m}, S_\mathrm{f}, I_\mathrm{m}, I_\mathrm{np}, I_\mathrm{p}, R, S_\mathrm{v}, I_\mathrm{v}\right) \in \mathbb {R}^8_+ : S_\mathrm{m}+S_\mathrm{f}+I_\mathrm{m}+I_\mathrm{np}+I_\mathrm{p}\\&+\,R+S_\mathrm{v}+I_\mathrm{v} \le N_\mathrm{h}(0); S_\mathrm{m},S_\mathrm{f} \le \rho N_\mathrm{h}(0); S_\mathrm{v} + I_\mathrm{v} \le N_\mathrm{v}(0); S_\mathrm{v} \le N_\mathrm{h}(0)\Bigg \}, \end{aligned}$$

the disease-free equilibrium $E_0 = \left( \frac{N_\mathrm{h}}{2}, \frac{N_\mathrm{h}}{2}, 0, 0, 0, 0, N_\mathrm{v}, 0\right)$ is globally asymptotically stable equilibrium of (2) provided that $R_0 < 1$.

Proof

The system (2) can be written as

$$\begin{aligned} \frac{\hbox {d}X}{\hbox {d}t}= & {} F(X,Z) \\ \frac{\hbox {d}Z}{\hbox {d}t}= & {} G(X,Z),\quad ~G(X,0) = 0, \end{aligned}$$

where $X = \left( S_\mathrm{m}, S_\mathrm{f}, R, S_\mathrm{v}\right) \in \mathbb {R}^4$ (the number of uninfected individuals compartments), $Z = \left( I_\mathrm{m}, I_\mathrm{np}, I_\mathrm{p}, I_\mathrm{v}\right) \in \mathbb {R}^4$ (the number of infected individuals compartments), and $E_0 = \left( \frac{N_\mathrm{h}}{2}, \frac{N_\mathrm{h}}{2}, 0, 0, 0, 0, N_\mathrm{v}, 0\right)$ is the disease-free equilibrium of the system (2).

The following two conditions must be met to guarantee global asymptotic stability:

• 1. For $\frac{\hbox {d}X}{\hbox {d}t} = F(X,0)$, $X^*$ is globally asymptotically stable,

• 2. $G(X,Z) = AZ - {\widehat{G}}(X,Z)$, ${\widehat{G}}(X,Z) \ge 0$ for $(X, Z) \in \varOmega$,

where $A = D_z G(X^*,0)$ is an Metzler matrix and $\varOmega$ is the region where the model makes biological sense. If the system (2) satisfies the above two conditions, then by the theorem given in Castillo-Chavez et al. (2002), the disease-free equilibrium $E_0$ is globally asymptotically stable when $R_0 < 1$. Now,

$$\begin{aligned} F(X,0)= & {} \displaystyle \left[ \begin{array}{cccc} \mu _\mathrm{h}\left( \frac{N_\mathrm{h}}{2}-S_\mathrm{m}\right) \\ \mu _\mathrm{h}\left( \frac{N_\mathrm{h}}{2}-S_\mathrm{f}\right) \\ -\mu _\mathrm{h}R\\ \mu _\mathrm{v}N_\mathrm{v} - \mu _\mathrm{v}S_\mathrm{v}\\ \end{array} \right] ,\\ A= & {} \displaystyle \left[ \begin{array}{cccc} -\left( \mu _\mathrm{h}+\gamma \right) &{} \beta _2 &{} \beta _2 &{} \beta _1\theta _1 \\ \left( 1-p\right) \beta _2 &{}\quad -\left( \mu _\mathrm{h}+\gamma \right) &{} \quad 0 &{} \quad (1-p)\beta _1\theta _1 \\ p\beta _2 &{}\quad 0 &{} \quad -\left( \mu _\mathrm{h}+\gamma \right) &{}\quad p\beta _1\theta _1 \\ \beta _1\theta _2 &{} \quad \beta _1\theta _2 &{}\quad \beta _1\theta _2 &{}\quad -\mu _\mathrm{v} \\ \end{array} \right] \end{aligned}$$

and

$$\begin{aligned} {\widehat{G}}(X,Z) = \displaystyle \left[ \begin{array}{cccc} \beta _2I_\mathrm{np}\left( 1-\frac{S_\mathrm{m}}{\rho N_\mathrm{h}}\right) + \beta _2I_\mathrm{p}\left( 1-\frac{S_\mathrm{m}}{\rho N_\mathrm{h}}\right) + \beta _1\theta _1I_\mathrm{v}\left( 1-\frac{S_\mathrm{m}}{N_\mathrm{h}}\right) \\ (1-p)\beta _2I_\mathrm{m}\left( 1-\frac{S_\mathrm{f}}{\rho N_\mathrm{h}}\right) + (1-p)\beta _1\theta _1I_\mathrm{v}\left( 1-\frac{S_\mathrm{f}}{N_\mathrm{h}}\right) \\ p\beta _2I_\mathrm{m}\left( 1-\frac{S_\mathrm{f}}{\rho N_\mathrm{h}}\right) + p\beta _1\theta _1I_\mathrm{v}\left( 1-\frac{S_\mathrm{f}}{N_\mathrm{h}}\right) \\ \beta _1\theta _2I_\mathrm{m}\left( 1-\frac{S_\mathrm{v}}{N_\mathrm{h}}\right) + \beta _1\theta _2I_\mathrm{np}\left( 1-\frac{S_\mathrm{v}}{N_\mathrm{h}}\right) + \beta _1\theta _2I_\mathrm{p}\left( 1-\frac{S_\mathrm{v}}{N_\mathrm{h}}\right) \end{array} \right] . \end{aligned}$$

It is clear that ${\widehat{G}}(X,Z)\ge 0$ in the region $\varOmega _1$. Therefore, it is also clear that $X^* = \left( \frac{N_\mathrm{h}}{2}, \frac{N_\mathrm{h}}{2}, 0, N_\mathrm{v}\right)$ is a globally asymptotically stable equilibrium of the system $\frac{\hbox {d}X}{\hbox {d}t} = F(X,0)$. Thus, the above theorem holds. $\square$

1.3 Existence of Endemic Equilibria

Suppose $E_* = \left( S_\mathrm{m}^*, S_\mathrm{f}^*, I_\mathrm{m}^*, I_\mathrm{np}^*, I_\mathrm{p}^*, R^*, S_\mathrm{v}^*, I_\mathrm{v}^*\right)$ is an endemic equilibrium of the Model (2), where

$$\begin{aligned} S_\mathrm{m}^*= & {} x \\ S_\mathrm{f}^*= & {} x \\ I_\mathrm{m}^*= & {} \frac{N_\mathrm{h}^2\rho \left[ \beta _1\theta _2\mu _\mathrm{h}+\mu _\mathrm{v}\left( \gamma +\mu _\mathrm{h}\right) \right] - \left[ 2\beta _1\theta _2\rho \left( \mu _\mathrm{h}N_\mathrm{h}+\beta _1\theta _1N_\mathrm{v}\right) +\beta _2\mu _\mathrm{v}N_\mathrm{h}\right] x}{2\beta _1\beta _2\theta _2x} \\ I_\mathrm{np}^*= & {} \frac{\mu _\mathrm{h}(1-p)\left( N_\mathrm{h}-2x\right) }{2\left( \gamma +\mu _\mathrm{h}\right) } \\ I_\mathrm{p}^*= & {} \frac{p\mu _\mathrm{h}\left( N_\mathrm{h}-2x\right) }{2\left( \gamma +\mu _\mathrm{h}\right) } \\ R^*= & {} \frac{N_\mathrm{h}^2\rho \left[ \beta _1\theta _2\mu _\mathrm{h}+\mu _\mathrm{v}\left( \gamma +\mu _\mathrm{h}\right) \right] - \left[ 2\beta _1\theta _2\rho \left( \mu _\mathrm{h}N_\mathrm{h}+\beta _1\theta _1N_\mathrm{v}\right) +\beta _2\mu _\mathrm{v}N_\mathrm{h}\right] x}{\beta _1\beta _2\theta _2\mu _\mathrm{h} x} \\ S_\mathrm{v}^*= & {} \frac{\mu _\mathrm{v}N_\mathrm{h}\left[ \rho N_\mathrm{h}\left( \gamma +\mu _\mathrm{h}\right) -\beta _2x\right] }{\beta _1^2\theta _1\theta _2\rho x} \\ I_\mathrm{v}^*= & {} \frac{\left( \beta _2\mu _\mathrm{v}N_\mathrm{h}+2\beta _1^2\theta _1\theta _2N_\mathrm{v}\rho \right) x - \mu _\mathrm{v}N_\mathrm{h}^2\rho \left( \gamma +\mu _\mathrm{h}\right) }{2\theta _1\theta _2\beta _1^2\rho x}. \end{aligned}$$

Here, x is the positive roots of the following quadratic equation

$$\begin{aligned} x^2 + Bx + C = 0, \end{aligned}$$

where

$$\begin{aligned} B= & {} -\frac{\left( \gamma +\mu _\mathrm{h}\right) \left[ 2\beta _1\theta _2\rho (\mu _\mathrm{h}N_\mathrm{h}+\beta _1\theta _1N_\mathrm{v})+\beta _2N_\mathrm{h}\mu _\mathrm{v}\right] +\beta _1\beta _2\theta _2\mu _\mathrm{h}N_\mathrm{h}}{2\beta _1\beta _2\theta _2\mu _\mathrm{h}} \\ C= & {} \frac{N_\mathrm{h}^2\rho \left( \gamma +\mu _\mathrm{h}\right) \left[ \beta _1\theta _2\mu _\mathrm{h}+\mu _\mathrm{v}\left( \gamma +\mu _\mathrm{h}\right) \right] }{2\beta _1\beta _2\theta _2\mu _\mathrm{h}} \end{aligned}$$

Endemic equilibria exists if

$$\begin{aligned}&\frac{\mu _\mathrm{v}N_\mathrm{h}^2\rho \left( \gamma +\mu _\mathrm{h}\right) }{\beta _2\mu _\mathrm{v}N_\mathrm{h}+2\beta _1^2\theta _1\theta _2N_\mathrm{v}\rho }< x \\&\quad < \min \Big \{\frac{N_\mathrm{h}}{2}, \frac{\rho N_\mathrm{h}\left( \gamma +\mu _\mathrm{h}\right) }{\beta _2}, \frac{N_\mathrm{h}^2\rho \left( \beta _1\theta _2\mu _\mathrm{h}+\mu _\mathrm{v}\gamma +\mu _\mathrm{v}\mu _\mathrm{h}\right) }{\beta _2\mu _\mathrm{v}N_\mathrm{h}+2\beta _1\theta _2\rho \left( \mu _\mathrm{h}N_\mathrm{h}+\beta _1\theta _1N_\mathrm{v}\right) }\Big \}. \end{aligned}$$

• Case I: If $\varDelta = B^2 - 4C < 0$, no interior equilibrium exists.

• Case II: If $\varDelta = B^2 - 4C = 0$, unique interior equilibrium may exists.

• Case III: If $\varDelta = B^2 - 4C > 0$, two interior equilibrium may exist.

Appendix B: Some Inference Plots

See Figs. 6, 7, and 8.

Fig. 6

Posterior distribution of different variables and parameters of the Model (1)

Fig. 7

Traces of the model variable and parameter values as obtained by the MCMC sampling for 500, 00 iteration numbers, for the Model (1)

Fig. 8

Monotone relationship of sensitivity parameters with respect to the total number of infected pregnant women cases per week, for the Model (1), which is the prerequisite before computing the PRCC