Skip to main content
SpringerLink
Log in

Mathematical Model of Cardiovascular and Metabolic Responses to Umbilical Cord Occlusions in Fetal Sheep

  • Original Article
  • Published:
Bulletin of Mathematical Biology Aims and scope Submit manuscript

Abstract

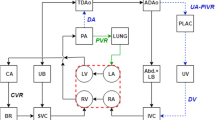
Fetal acidemia during labor is associated with an increased risk of brain injury and lasting neurological deficits. This is in part due to the repetitive occlusions of the umbilical cord (UCO) induced by uterine contractions. Whereas fetal heart rate (FHR) monitoring is widely used clinically, it fails to detect fetal acidemia. Hence, new approaches are needed for early detection of fetal acidemia during labor. We built a mathematical model of the UCO effects on FHR, mean arterial blood pressure (MABP), oxygenation and metabolism. Mimicking fetal experiments, our in silico model reproduces salient features of experimentally observed fetal cardiovascular and metabolic behavior including FHR overshoot, gradual MABP decrease and mixed metabolic and respiratory acidemia during UCO. Combined with statistical analysis, our model provides valuable insight into the labor-like fetal distress and guidance for refining FHR monitoring algorithms to improve detection of fetal acidemia and cardiovascular decompensation.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8

Similar content being viewed by others

Abbreviations

FHR:

Fetal heart rate

MABP:

Mean arterial blood pressure

UCO:

Umbilical cord occlusions

BD:

Base deficit

RMSSD:

Root-mean-square of the successive differences

\(a_h\) :

Coefficient related to ventricular elastance during relaxation (mmHg/ml\(^2\))

\(\alpha _b\) :

Maximum binding capacity of hemoglobin (ml \(\mathrm {O_2}\)/g Hb)

b :

Parameter related to ventricular volume for zero diastolic pressure (ml)

\(\beta _d\) :

Scaling factor on dissolved oxygen (1/mmHg)

\(\beta _h(H)\) :

Time for the onset of ventricular relaxation (s)

\(\eta \) :

Parameter controlling the steepness of the relation for heart pressure

\(\theta \) :

Median value of time for peak pressure in heart model (1/s)

\(\phi \) :

Median value of heart pressure in heart model (1/s)

\(\psi \) :

Scaling factor in mean arterial pressure (1/s)

\(\mathrm {[CO_2]}_i\) :

Carbon dioxide concentration in compartment \(i=\mathrm{nc,c,um}\) (ml \(\mathrm {CO_2}\)/ml blood)

\(\mathrm {[CO_2]}_{a}\) :

Feeding Carbon dioxide concentration in fetus (ml \(\mathrm {CO_2}\)/ml blood)

\(c_\mathrm{f}, c_\mathrm{m}\) :

Heart contractility for fetus/mother (mmHg/ml)

\(c_{1,\mathrm m}, c_{1,\mathrm f}\) :

Scaling constant in the relation between Oxygen content and its partial pressure for mother and fetus (\(\hbox {mmHg}^3\))

\(c_{2,\mathrm{m}}, c_{2,\mathrm{f}}\) :

Scaling constant in the relation between Oxygen content and its partial pressure for mother and fetus (\(\hbox {mmHg}^2\))

\(C_i\) :

Compliance in compartment \(i=\mathrm{nc,c,um,ut}\) (ml/mmHg)

\(d_\mathrm{f}, d_\mathrm{m}\) :

Parameter related to the volume-dependent and volume-independent components of the developed pressure (mmHg)

\(\delta G_{T}\) :

Scaling factor for sympathetic gain (\(\hbox {s}^2\))

D :

Mass transfer coefficient in Oxygen model (\(\hbox {ml }\mathrm {O_2}\hbox {/s/mmHg}\))

\(D_{\mathrm{CO}_2}\) :

Mass transfer coefficient in Carbon dioxide model (\(\hbox {ml }\mathrm {CO_2} \hbox {/s/mmHg}\))

\(\Delta C_v\) :

Effector in venous compliance (ml/mmHg)

\(\Delta c\) :

Effector in heart contractility (mmHg/ml)

\(f_{\mathrm{br}}, f_{\mathrm{cr}}\) :

Afferent firing rate via stimulation of baro/chemoreceptor (1/s)

\(f_{i,o}\) :

Offset values that create a threshold for vagal or sympathetic response \(i=\mathrm{va},s\beta ,s\alpha \) (1/s)

\(f_{i,\mathrm{min}}, f_{i,\mathrm{max}}\) :

Control constants in sigmoid functions \(i=s,\hbox {va},\hbox {hy},\hbox {br},\hbox {cr}\) (1/s)

\(f_{i,n}\) :

A normal value for corresponding afferent or efferent firing rate \(i=\hbox {br}, \hbox {cr}, \hbox {va},s\beta ,s\alpha \) (1/s)

\(f_\mathrm{s,0}, f_{\mathrm{s},\infty }\) :

Constants in sympathetic firing rate function (1/s)

\(f_{\mathrm{s}\beta }, f_{\mathrm{s}\alpha }\) :

Efferent \(\alpha \)- and \(\beta \)- sympathetic firing rate (1/s)

\(f_{\mathrm{s},1}\) :

Control parameter in sympathetic firing rate function (1/s)

\(f_\mathrm{va}\) :

Efferent vagal firing rate (1/s)

\(G_{i}\) :

Gain constants \(i=T_\mathrm{va}, T_s\) (\(\hbox { s}^2\))

\(G_c, G_\mathrm{va}\) :

Constants in effectors (mmHg s/ml)

\(\mathrm {[GL]}_a\) :

Arterial glucose concentration (mM)

\(\mathrm {[GL]}_i\) :

Glucose concentration in compartment \(i=\hbox {nc}, \hbox {c}, \hbox {um}\) (mM)

g(t):

Activation function in heart model

H (FHR):

Heart rate (1/s)

\(\mathrm {[H^+]}_a\) :

Arterial proton concentration (nM)

\(\mathrm {[H^+]}_i\) :

Proton concentration in compartment \(i=\hbox {nc}, \hbox {c}, \hbox {um}\) (nM)

\(Hb_\mathrm{f}, Hb_\mathrm{m}\) :

Hemoglobin concentration in fetus or mother (g Hb/ml blood)

\(K_{f}\) :

Constant in Oxygen metabolic model (ml blood/s)

\(K_1\) :

Kinetic constant (mmol/s)

\(K_i\) :

Kinetic constant \(i=2,3,4,5,6\) (ml/s)

\(K_7\) :

Kinetic constant (\(10^{-6}\,\hbox {ml/ s}\))

\(K_{8}, K_9\) :

Kinetic constant (nmol/s)

\(K_{10}\) :

Kinetic constant (\(10^{6}\,\hbox {ml/s}\))

\(K_{\mathrm {CO_2}}\) :

Scaling constants (ml \(\mathrm {CO_2}\)/ml blood/mmHg)

\(k_{\mathrm {CO_2}}\) :

Scaling constants ml (\(\mathrm {CO_2}/\hbox {ml blood}\))

\(k_{c}\) :

Control constant

\(k_\mathrm{hy}, k_{\mathrm{pr},s}\) :

Control constant (mmHg)

\(k_{i}\) :

Constant \(i=R_\mathrm{nc},\hbox {br}, \hbox {cr}, \hbox {s}, \hbox {va}\) (1/s)

\(\mathrm {[LA]}_{a}\) :

Arterial lactate concentration (mM)

\(\mathrm {[LA]}_{i}\) :

Lactate concentration in compartment \(i=\hbox {nc}, \hbox {c}, \hbox {um}\) (mM)

\(M_i\) :

Production rate of \(\mathrm {[CO_2]_i}\) with \(i=\hbox {nc}, \hbox {c}, \hbox {um}\) (ml/s)

\(M_\mathrm{pH}\) :

Production rate due to \(\mathrm {[H^+]}\) accumulation (ml/s)

\(N_p\) :

Normalization factor (s)

\(\nu \) :

Parameter related to steepness of the time for peak pressure in heart model

m, n :

Parameters related to the onset time of ventricular relaxation in heart model

\(\mathrm {[O_{2}]}_\mathrm{th}\) :

Threshold value to control Oxygen metabolic uptake (\(\hbox {ml }\mathrm {O_2}/ \hbox {ml blood}\))

\(\mathrm {[O_{2}]}_{a}, \mathrm {[O_{2}]}_{m,a}\) :

Feeding Oxygen concentration in fetus and mother (\(\hbox {ml }\mathrm {O_2}/\hbox {ml blood}\))

\(\mathrm {[O_2]}_{a,n}\) :

A normal value to control the sigmoid function in systemic resistance (\(\hbox {ml }\mathrm {O_2}/\hbox {ml blood}\))

\(\mathrm {[O_2]_i}\) :

Oxygen concentration in compartment \(i=\hbox {nc}, \hbox {c}, \hbox {um}, \hbox {ivs}\) (ml \(\mathrm {O_2}/\hbox {ml blood}\))

\(\mathrm {O_{\mathrm{met},i}}\) :

Metabolic Oxygen uptake in compartment \(i=\hbox {nc, c}\) (ml \(\mathrm {O_2}/\hbox {s}\))

\(p_\mathrm{min},p_\mathrm{max}\) :

Control constants in heart model (mmHg)

\(p_i\) :

Blood pressure in compartment \(i=\hbox { nc, c, um, h, ut, ivs}\) (mmHg)

\(\bar{p}_a\) (MABP):

Mean arterial blood pressure (mmHg)

\(\mathrm {P}_{\mathrm {O_2},i}\) :

Oxygen partial pressure in compartment \(i=\hbox {nc, c, um, ivs}\) (mmHg)

\(\mathrm {P}_{\mathrm {CO_2},i}\) :

Carbon dioxide partial pressure in compartment \(i=\hbox {nc, c, um}\) (mmHg)

\(\mathrm {P}_{\mathrm {CO_2},\hbox {nc, n}}\) :

A normal value for Carbon dioxide partial pressure in systemic compartment (mmHg)

\(\mathrm {P}_{\mathrm {O_{2}},c,0}\) :

Threshold value for cerebral Oxygen partial pressure to control the sigmoid function in firing rate function \(f_\mathrm{hy}\) (mmHg)

\(\mathrm {[PY]_a}\) :

Arterial pyruvate concentration (mM)

\(\mathrm {[PY]_i}\) :

Pyruvate concentration in compartment \(i=\hbox {nc, c, um}\) (mM)

\(q_{i,a}\) :

Arterial flow rate into compartment \(i=\hbox {nc, c, um}\) (ml/s)

\(q_{i,v}\) :

Venous flow rate out of compartment \(i=\hbox {nc, c, um}\) (ml/s)

\(q_\mathrm{in}, q_\mathrm{out}\) :

Flow rate in/out of corresponding compartment by summing over flow rate from local branches (ml/s)

\(R_i\) :

Resistance in compartment \(i=\mathrm{nc, c, um, ut, ivs, m}\) (mmHg s/ml)

\(R_{c,a,0}, R_{cn,0}\) :

Baseline values for cerebral and systemic resistance (mmHg s/ml)

\(R_{c, \mathrm{min}}, R_{c, \mathrm{max}}\) :

Constants in cerebral autoregulation model (mmHg s/ml)

\(R_{\mathrm{nc, min}}, R_{\mathrm{nc, max}}\) :

Constants in systemic resistance model (mmHg s/ml)

\(\Delta R_\mathrm{nc}\) :

Effector in systemic resistance (mmHg s/ml)

\(S_{ij}\) :

Relative sensitivity matrix

\(S_{\mathrm {[O_2]},d}\) :

Oxygen diffusion (ml \(\mathrm {O_2}/\hbox {s}\))

\(S_{\mathrm {P[O_2]}}\) :

Scaling function to relate Oxygen content and its partial pressure

\(s_\mathrm{mv}, s_\mathrm{av}\) :

Indicator function of mitral/aortic valve

\(t_\mathrm{p}\) :

Peak time (s)

\(t_\mathrm{min},t_\mathrm{max}\) :

Control constants in heart model (s)

\(T_0\) :

Base line value for heart period (s)

\(T_{v,0}, T_{\mathrm{s},0}\) :

Contributions from vagal and sympathetic activities on the base heart period (s)

\(\tau _i\) :

Control constant \(i=\hbox {gs},\hbox {gv}\) (1/s)

\(\tau _0\) :

Offset constant in heart period (s)

\(\tau _\mathrm{pr,br},\tau _\mathrm{pr,cr}\) :

Control constant (mmHg)

\(\tau _\mathrm{br}, \tau _\mathrm{cr}, \tau _s, \tau _{Rc}\) :

Time constant (s)

\(\tau _{T,s}, \tau _{T,v}\) :

Time constant (s)

\(\Delta T_s,\Delta T_\mathrm{va}\) :

Contribution to heart period from sympathetic/vagal activity (s)

\(V_i\) :

Volume in compartment \(i=\hbox {nc, c, um, h, ut}\) (ml)

\(V_\mathrm{total}\) :

Total volume of all compartments in the circulation system (ml)

\(W_{\mathrm{br},i}, W_{\mathrm{cr},i}\) :

Weight factor \(i=s\alpha , s\beta \)

References

  • Agrawal S, Doucette F, Gratton R, Richardson B, Gagnon R (2003) Intrapartum computerized fetal heart rate parameters and metabolic acidosis at birth. Obstet Gynecol 102:731

    Article  Google Scholar 

  • Bennet L, Westgate J, Liu Y, Wassink G, Gunn A (2005) Fetal acidosis and hypotension during repeated umbilical cord occlusions are associated with enhanced chemoreflex responses in near-term fetal sheep. J Appl Physiol 99:1477

    Article  Google Scholar 

  • Bishai J, Blood A, Hunter C, Longo L, Power G (2003) Fetal lamb cerebral blood flow (CBF) and oxygen tensions during hypoxia: a comparison of laser Doppler and microsphere measurements of CBF. J Physiol 456:869

    Article  Google Scholar 

  • Breit M (2004) Sensitivity analysis of biological pathways, Master’s thesis, University for Health Sciences, Medical Informatics and Technology, Germany

  • Cabrera M, Saidel G, Kalhan S (1998) Role of O\(_2\) in regulation of lactate dynamics during hypoxia: mathematical model and analysis. Ann Biomed Eng 26:1

    Article  Google Scholar 

  • Cloutier M, Bolger F, Lowry J, Wellstead P (2009) An integrative dynamic model of brain energy metabolism using in vivo neurochemical measurements. J Comput Neurosci 3:391

    Article  MathSciNet  Google Scholar 

  • Couto P, Meurs W, Bernardes J, de Sa JM, Goodwin J (2002) Mathematical model for educational simulation of the oxygen delivery to the fetus. Control Eng Pract 10:59

    Article  Google Scholar 

  • D’Angelo C, Papelier Y (2005) Mathematical modeling of the cardiovascular system and skeletal muscle interaction during excecise. ESAIM Proc 14:72

    MathSciNet  MATH  Google Scholar 

  • de Haan H, Gunn A, Gluckman P (1997) Fetal heart rate changes do not reflect cardiovascular deterioration during brief repeated umbilical cord occlusions in near-term fetal lambs. Am J Obstet Gynecol 176:8

    Article  Google Scholar 

  • Durosier L, Green G, Batkin I, Seely A, Ross M, Richardson B, Frasch M (2014) Sampling rate of heart rate variability impacts the ability to detect acidemia in ovine fetuses near-term. Front Pediatr 2:PMC4017161

    Article  Google Scholar 

  • Ellwein L, Tran H, Zapata C, Novak V, Olufsen M (2008) Sensitivity analysis and model assessment: mathematical models for arterial blood flow and blood pressure. Cardiovasc Eng 8:94

    Article  Google Scholar 

  • Frasch M, Muller T, Hoyer D, Weiss C, Schubert H, Schwab M (2009a) Nonlinear properties of vagal and sympathetic modulations of heart rate variability in ovine fetus near term. Am J Physiol Regul Integr Comp Physiol 296:R702

    Article  Google Scholar 

  • Frasch M, Mansano R, Gagnon R, Richardson B, Ross M (2009b) Measures of acidosis with repetitive umbilical cord occlusions leading to fetal asphyxia in the near-term ovine fetus. AJOG 200(200):e1

    Google Scholar 

  • Frasch M, Muller T, Hoyer D, Weiss C, Schubert H, Schwab M (2009c) Heart rate variability analysis allows early asphyxia detection in ovine fetus. Reprod Sci 16:509

    Article  Google Scholar 

  • Frasch M, Keen A, Gagnon R, Ross M, Richardson B (2011) Monitoring fetal electrocortical activity during labour for predicting worsening acidemia: a prospective study in the ovine fetus near term. PLoS One 6:e22100

    Article  Google Scholar 

  • Frasch M, Frank B, Last M, Muller T (2012) Time scales of autonomic information flow in near-term fetal sheep. Front Physiol 3:378

    Article  Google Scholar 

  • Gold N, Frasch M, Herry C, Richardson B, Seely A, Wang X (2015) Online change-point detection for non stationary biological time series with Gaussian processes. In preparation

  • Gui R, Schüthe C, Bernhard S (2015) Mathematical modeling and sensitivity analysis of arterial anastomosis in arm arteries. ZIB Report

  • Hunter C, Blood A, Power G (2003) Cerebral metabolism during cord occlusion and hypoxia in the fetal sheep: a novel method of continuous measurement based on heat production. J Physiol 552:241

    Article  Google Scholar 

  • Jensen A, Garnier Y, Berger R (1999) Dynamics of fetal circulatory responses to hypoxia and asphyxia. Eur J Obstet Gynecol Reprod Biol 84:155

    Article  Google Scholar 

  • Khoo M, Kronauer R, Strohl K, Slutsky A (1982) Factors inducing periodic breathing in humans: a general model. J Appl Physiol 53:644

    Google Scholar 

  • Khoo M, Gottschalk A, Pack A (1991) Sleep induced periodic breathing and apnea: a theoretical study. J Appl Physiol 70:2014

    Google Scholar 

  • Koos B, Chau A, Qgunyemi D (1995) Adenosine mediates metabolic and cardiovascular responses to hypoxia in fetal sheep. J Physiol 488:761

    Article  Google Scholar 

  • Kubota T, Alexander J, Itaya R, Todaka K, Sugimachi M, Sunagawa K, Nose Y (1992a) Dynamic effects of carotid sinus baroreflex on ventriculoarterial coupling studied in anesthetized dogs. Circ Res 70:1044

    Article  Google Scholar 

  • Kubota T, Chishaki H, Yoshida T, Sunagawa K, Takeshita A, Nose Y (1992b) How to encode arterial pressure into carotid sinus nerve to invoke natural baroreflex. Am J Physiol 263:H307

    Google Scholar 

  • Levy M, Zieske H (1969) Autonomic control of cardiac pacemaker activity and atrioventricular transmission. J Appl Physiol 27:465

    Google Scholar 

  • Liang F, Liu H (2006) Simulation of hemodynamic responses to the Valsalva Maneuver: an integrative computational model of the cardiovascular system and the autonomic nervious system. J Physiol Sci 56:45

    Article  Google Scholar 

  • Liang F, Takagi S, Himeno R (2009) Multi-scale modeling of the human cardiovascular system with applications to aortic valvular and arterial stenoses. Med Biol Eng Comput 47:743

    Article  Google Scholar 

  • Liston R, Sawchuck D, Young D (2007) Fetal health surveillance: antepartum and intrapartum consensus guideline. J Obstet Gynecol Can 29:53

    Google Scholar 

  • Low J, Panagiotopoulos C, Derrick E (1995) Newborn complications after intrapartum asphyxia with metabolic acidosis in the preterm fetus. Am J Obstet Gynecol 172:805

    Article  Google Scholar 

  • Magosso E, Ursino M (2001) A mathematical model of CO\(_2\) effect on cardiovascular regulation. Am J Physiol Heart Circ Physiol 281:H2036

    Google Scholar 

  • Mallard E, Williams C, Johnston B, Gunning M, Davis S et al (1995) Repeated episodes of umbilical cord occlusion in fetal sheep lead to preferential damage to the striatum and sensitize the heart to further insults. Pediatr Res 37:707

    Article  Google Scholar 

  • Olufsen M, Tran H, Ottesen J (2004) Modeling cerebral blood flow control during posture change from sitting to standing. Cardiovasc Eng Int J 4:47

    Article  Google Scholar 

  • Olufsen M, Ottesen J, Tran H, Ellwein L, Lipsitz L, Novak V (2005) Blood pressure and blood flow variation during postural change from sitting to standing: model development and validation. J Appl Physiol 99:1523

    Article  Google Scholar 

  • Orlowski P, Chappell M, Park C, Grau V, Payne S (2011) Modeling of pH dynamics in brain cells after stroke. Interface Focus 1:408

    Article  Google Scholar 

  • Quilligan E, Vasicka A, Aznar R, Lipsitz P, Moore T, Bloor BM (1960) Partial pressure of oxygen in the intervillous space and the umbilical vessels. Am J Obstet Gynecol 79:1048

    Google Scholar 

  • Ramsay J, Hooker G, Campbell D, Cao J (2007) Parameter estimation for differential equations: a generalized smoothing approach. J R Stat Soc B 69:741

    Article  MathSciNet  Google Scholar 

  • Ross M, Jessie M, Amaya K, Matushewski B, Durosier L, Martin M, Richardson B (2013) Correlation of arterial fetal base deficit and lactate changes with severity of variable heart rate decelerations in the near-term ovine fetus. Am J Obstet Gynecol 208:e1

    Article  Google Scholar 

  • Shi Y, Patricia L, Rodney H (2011) Review of zero-D and 1-D models of blood flow in the cardiovascular system. Biomed Eng Online 10:1

    Article  Google Scholar 

  • Thakor A, Giussani D (2008) Effects of acute acidemia on the fetal cardiovascular defense to acute hypoxemia. Am J Physiol Regul Integr Comp Physiol 296:R90

    Article  Google Scholar 

  • Ursino M (1998) Interaction between carotid baroregulation and the pulsating heart: a mathematical model. Am J Physiol 275:H1733

    Google Scholar 

  • Ursino M, Magosso E (2000) Acute cardiovascular response to isocapnic hypoxia. I. A mathematical model. Am J Physiol Heart Circ Physiol 279:H149

    Google Scholar 

  • Ursino M, Magosso E (2003) Role of short-term cardiovascular regulation in heart period variability. a modeling study. Am J Physiol Heart Circ Physiol 284:H1479

    Article  Google Scholar 

  • van der Hout-van der Jagt M, Oei S, Bovendeerd P (2012) A mathematical model for simulation of early decelerations in cardiotocogram during labor. Med Eng Phys 34:579

    Article  Google Scholar 

  • van der Hout-van der Jagt M, Oei S, Bovendeerd P (2013) Simulation of reflex late decelerations in labor with a mathematical model. Early Hum Dev 89:7

    Article  Google Scholar 

  • Wang X, Durosier L, Ross M, Richardson B, Frasch M (2014) Online detection of fetal acidemia during labour by testing synchronization of EEG and heart rate: a prospective study in fetal sheep. PLoS One 9:PMC4182309

    Google Scholar 

  • Westgate J, Bennet L, Gunn A (1999) Fetal heart rate variability changes during brief repeated umbilical cord occlusion in near term fetal sheep. J Obstet Gynecol 106:664

    Google Scholar 

  • Westgate J, Bennet L, de Haan H, Gunn A (2001) Fetal heart rate overshoot during repeated umbilical cord occlusion in sheep. Obstet Gynecol 97:454

    Article  Google Scholar 

  • Westgate J, Wibbens B, Bennet L, Wassink G, Parer J, Gunn A (2007) The intrapartum deceleration in center stage: a physiologic approach to the interpretation of fetal heart rate changes in labor. Am J Obstet Gynecol 197(236):e1

    Google Scholar 

  • Yan E, Baburamant A, Walker A, Walker D (2009) Changes in cerebral blood flow, cerebral metabolites, and breathing movements in the sheep fetus following asphyxia produced by occlusion of the umbilical cord. Am J Physiol Regul Integr Comp Physiol 297:R60

    Article  Google Scholar 

Download references

Acknowledgments

The authors are grateful to Dr. Josh Chang for some useful discussions. This work is partially supported by MITACS, NeuroDevNet, NSERC, CIHR and the Fields Institute.

Author information

Authors and Affiliations

  1. Department of Mathematics and Statistics, York University, Toronto, ON, M3J 1P3, Canada

    Qiming Wang, Nathan Gold, Huaxiong Huang & Xiaogang Wang

  2. Department of Obstetrics and Gynecology, Faculty of Medicine, CHU Sainte-Justine Research Center, Montréal, QC, H3T 1C5, Canada

    Martin G. Frasch

  3. Department of Neurosciences, Faculty of Medicine, CHU Sainte-Justine Research Center, Montréal, QC, H3T 1C5, Canada

    Martin G. Frasch

  4. Centre de Recherche en Reproduction Animale (CRRA), Université de Montréal, 3200 rue Sicotte, Saint-Hyacinthe, QC, J2S 7C6, Canada

    Martin G. Frasch

  5. UPMC, Laboratoire Jacques-Louis Lions, CNRS, UMR 7598, INRIA, EPI REO, Sorbonne University, 75252, Paris, France

    Marc Thiriet

Authors
  1. Qiming Wang
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Nathan Gold
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Martin G. Frasch
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Huaxiong Huang
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Marc Thiriet
    View author publications

    You can also search for this author in PubMed Google Scholar

  6. Xiaogang Wang
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Huaxiong Huang.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Wang, Q., Gold, N., Frasch, M.G. et al. Mathematical Model of Cardiovascular and Metabolic Responses to Umbilical Cord Occlusions in Fetal Sheep. Bull Math Biol 77, 2264–2293 (2015). https://doi.org/10.1007/s11538-015-0122-4

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11538-015-0122-4

Keywords

Search

Navigation