A Model of Oscillatory Protein Dynamics in Bacteria

Abstract

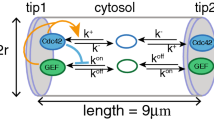
Spatial oscillations of proteins in bacteria have recently attracted much attention. The cellular mechanism underlying these oscillations can be studied at molecular as well as at more macroscopic levels. We construct a minimal mathematical model with two proteins that is able to produce self-sustained regular pole-to-pole oscillations without having to take into account molecular details of the proteins and their interactions. The dynamics of the model is based solely on diffusion across the cell body and protein reactions at the poles, and is independent of stimuli coming from the environment. We solve the associated system of reaction–diffusion equations and perform a parameter scan to demonstrate robustness of the model for two possible sets of the reaction functions.

Appendix

This section describes the numerical algorithm used in the simulations and the parameter scan.

Algorithm

The ultimate goal is to check for robustness of the model, in other words, to determine the range of the values of the parameters where the dynamical systems with reaction functions (35) and (36) display qualitatively the desired behavior (oscillations of the protein concentrations at a fixed position in space). To automatize computation, the parameters are input from an array p. A parameter search involves scanning the admissible values of the array p and selecting those leading to oscillatory solutions. In our simulations the array has 3 entries.

Corollary 5 gives us a criterion for existence of an unstable equilibrium (9), which means that the chosen equilibrium c in (50) for the approximating ODE system given by (37), (46)–(45) is unstable, also. It is therefore enough to check whether the solution of the discrete problem is periodic whenever the matrix V given by (13) has an eigenvalue with positive real part. Due to the nonlinear terms the orbits starting from a perturbation of c may bend and not tend to ∞. Because of mass conservation (48) and the positivity of the variables, guaranteed by the form of on- and off-rates, whenever the eigenvalues of V are positive, the orbit starting near c may either circle around the equilibrium or finish at a different stable equilibrium c′. However, if a limit cycle around c exists, the orbit starting from a perturbation of c may never join it, but continue moving around it (Verhulst 1990). A periodic solution of the ODE system is thus asymptotically stable, with the orbit approaching the limit cycle arbitrarily close.

The period of oscillation of the solution is approximated numerically using a Poincaré section. The Poincaré section Ω is a (n−1)-dimensional manifold transversal to the limit cycle. To determine the length of the cycle we search for the time points t where an orbit starting from the given initial value passes through Ω, always entering from the same direction (Seydel 2010; Verhulst 1990). Since in the laboratory experiment, the motion of the bacterium is observed on a specific time scale (say, 45 min to 1 h), it is enough to check that the numerically computed solution sol oscillates within a certain time interval [0,T _max] for a fixed T _max. To find the period of oscillation, we need at least one period occurring in [0,T _max], since periods longer than the period of observation are not registered in the experiment. Then we take the average over the computed periods. We have chosen an upper limit of T _max=500 time units.

To minimize errors arising from transient irregular oscillations at the beginning of the orbit, we begin our search from the endpoint at T _max, that is, we inspect backward the numerically computed solution sol(j)↦sol(T _max−j).

We search for the range of the values of p leading to oscillations, using the following pseudocode:

• P1. set p.

• P2. compute eigenvalues of V.

• P3. if a positive eigenvalue exists, goto P4, else goto P1.

• P4. solve ODE.

• P5. if the ODE solution sol is periodic, goto P6 else goto P1.

• P6. check for period of solution (optional).

Since the Jacobian of the ODE system (37), (46)–(45) linearized at c has eigenvalues whose absolute value grows with increasing the grid size n, the problem is stiff.

A linearly-implicit solver scheme must be used to achieve numerical precision. In particular, we have used a Krylov-ROW method implemented in ROWMAP, a solver developed for solving large stiff initial value problems in (Weiner et al. 1997). Its advantage lies in the “matrix-free” method, which does not require explicit computation of the full Jacobian, and solves the equations by a multiple Arnoldi process. Furthermore, the time-step used is variable because it is controlled by local error estimates for increased efficiency. Because the ultimate goal is to perform a parameter scan, these features are an advantageous way to speed up the computations. The current version of ROWMAP can be found at http://numerik.mathematik.uni-halle.de/forschung/software/.

Checking for Ghost Solutions

To verify the robustness of our model, we have to exclude ghost solutions arising from discretization errors. In our model, these could appear whenever the Jacobian of the discrete ODE system (37), (46)–(45) has an eigenvalue with positive real part, but the matrix A ⁻¹ V from Corollary 5 does not have an eigenvalue with positive real part.

It has been numerically verified that for reaction rates (35) and (36) inside the computed parameter ranges the eigenvalues λ with $\operatorname{Re}\lambda>0$ of the Jacobian of the right-hand side of the discrete ODE system (37), (46)–(45) never correspond to eigenvalues ρ of A ⁻¹ V with $\operatorname{Re}\rho<0$. Thus, “ghost” oscillations arising from discretization errors do not appear. In fact, for the Jacobian matrix, $\operatorname{Re}\lambda$ crosses into the positive only after $\operatorname{Re}\rho$ when one parameter a _i is varied and the other two are fixed; see, for example, Fig. 9.

Fig. 9

Plot of $\operatorname{Re}\rho$ and smallest non zero eigenvalue λ of the Jacobian vs. a ₃ for different grid size n and Choice A with a ₁=0.4,a ₂=0.8