Skip to main content
SpringerLink
Log in

Numerical Investigation of a Branch-Shaped Filter Based on Metal-Insulator-Metal Waveguide

  • Published:
Plasmonics Aims and scope Submit manuscript

Abstract

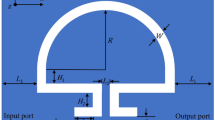
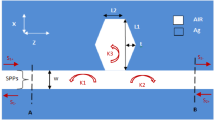
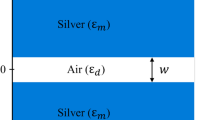
Using the finite difference time-domain method, we present a comprehensive numerical investigation of a branch-shaped filter based on the metal-insulator-metal (MIM) waveguide. The results show that several passbands and stopbands appear in the transmission spectra, which are resulted by the phase differences between the surface plasmon polaritons (SPPs) propagating along the straight waveguide and the SPPs resonating in the circuit formed by the branch and the straight waveguide. The effects of the structural parameters of the branch-shaped filters on their transmission properties are also studied. These results not only present an alternative plasmonic filter for the MIM waveguides but also help us to understand the transmission properties of the circuit-shaped structures.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6

Similar content being viewed by others

References

  1. Raether H (1988) Surface plasmons on smooth and rough surfaces and on gratings. Springer, Berlin

    Google Scholar 

  2. Gramotnev DK, Bozhevolnyi SI (2010) Plasmonics beyond the diffraction limit. Nat Photonics 4:83–91

    Article  CAS  Google Scholar 

  3. Rosenzveig T, Hermannsson PG, Leosson K (2010) Modelling of polarization-dependent loss in plasmonic nanowire waveguides. Plasmonics 5:75–77

    Article  CAS  Google Scholar 

  4. Fang Y, Li Z, Huang Y, Zhang S, Nordlander P, Halas NJ, Xu H (2010) Branched silver nanowires as controllable plasmon routers. Nano Lett 10:1950–1954

    Article  CAS  Google Scholar 

  5. Bozhevolnyi SI, Volkov VS, Devaux E, Laluet JY, Ebbesen TW (2006) Channel plasmon subwavelength waveguide components including interferometers and ring resonators. Nature 440:508–511

    Article  CAS  Google Scholar 

  6. Fang Z, Qi H, Wang C, Zhu X (2010) Hybrid plasmonic waveguide based on tapered dielectric nanoribbon: excitation and focusing. Plasmonics 5:207–212

    Article  CAS  Google Scholar 

  7. Wang Y (2003) Wavelength selection with coupled surface plasmon waves. Appl Phys Lett 82:4385–4387

    Article  CAS  Google Scholar 

  8. Lei DY, Ong HC (2007) Enhanced forward emission from ZnO via surface plasmons. Appl Phys Lett 91:211107–211109

    Article  Google Scholar 

  9. Veronis G, Fan S (2005) Bends and splitters in metal-dielectric-metal subwavelength plasmonic waveguides. Appl Phys Lett 87:131102–131104

    Article  Google Scholar 

  10. Hao H, Shi H, Wang C, Du C, Luo X, Deng Q, Lv Y, Lin X, Yao H (2005) Surface plasmon polariton propagation and combination in Y-shaped metallic channels. Opt Express 13:10795–10800

    Article  Google Scholar 

  11. Nikolajsen T, Leosson K, Bozhevolnyi SI (2004) Surface plasmon polariton based modulators and switches operating at telecom wavelengths. Appl Phys Lett 85:5833–5835

    Article  CAS  Google Scholar 

  12. Wang B, Wang GP (2004) Surface plasmon polariton propagation in nanoscale metal gap waveguides. Opt Lett 29:1992–1994

    Article  Google Scholar 

  13. Wang B, Wang GP (2005) Plasmon Bragg reflectors and nanocavities on flat metallic surface. Appl Phys Lett 87:013107–013109

    Article  Google Scholar 

  14. Lin WH, Wang GP (2007) Metal heterowaveguide superlattices for a plasmonic analogue to electron Bloch oscillations. Appl Phys Lett 91:143121–143123

    Article  Google Scholar 

  15. Hossieni A, Massoud Y (2006) A low-loss metal-insulator-metal plasmonic bragg reflector. Opt Express 14:11318–11323

    Article  Google Scholar 

  16. Park J, Kim H, Lee B (2008) High order plasmonic Bragg reflection in the metal-insulator-metal waveguide Bragg grating. Opt Express 16:413–425

    Article  Google Scholar 

  17. Hosseini A, Massoud Y (2007) Nanoscale surface plasmon based resonator using rectangular geometry. Appl Phys Lett 90:181102–181104

    Article  Google Scholar 

  18. Lin X-S, Huang X-G (2008) Tooth-shaped plasmonic waveguide filters with nanometeric sizes. Opt Lett 33:2874–2876

    Article  Google Scholar 

  19. Wang T-B, Wen X-W, Yin C-P, Wang H-Z (2009) The transmission characteristics of surface plasmon polaritons in ring resonator. Opt Express 17:24096–24101

    Article  CAS  Google Scholar 

  20. Yun B, Hu G, Cui Y (2010) Theoretical analysis of a nanoscale plasmonic filter based on a rectangular metal-insulator-metal waveguide. J Phys D: Appl Phys 43:385102–385109

    Article  Google Scholar 

  21. Kekatpure RD, Hryciw AC, Barnard ES, Brongersma ML (2009) Solving dielectric and plasmonic waveguide dispersion relations on a pocket calculator. Opt Express 17:24112–24129

    Article  Google Scholar 

  22. Gai H, Wang J, Tian Q (2007) Modified Debye model parameters of metals applicable for broadband calculations. Appl Opt 46:2229–2233

    Article  CAS  Google Scholar 

  23. Burke JJ, Stegeman GI, Tamir T (1986) Surface-polariton-like waves guided by thin, lossy metal films. Phys Rev B 33:5186–5201

    Article  CAS  Google Scholar 

Download references

Acknowledgements

This work was supported by National Natural Foundation of China (Grant Nos. 11004160, 10904009, and 10974157), the Natural Science Foundation of CQ CSTC (Grant Nos. CSTC2010BB4005 and CSTC2010BA6002), the Fundamental Research Funds for the Central Universities (Grant Nos. XDJK2009C078 and XDJK2009A001), and the Southwest University Research Foundation (Grant No. SWU109024).

Author information

Authors and Affiliations

  1. School of Physical Science and Technology, Southwest University, Chongqing, 400715, People’s Republic of China

    Zhongyue Zhang, Yanan Zhao, Dong Lu & Zuhong Xiong

  2. School of Physical Electronics, University of Electronic Science and Technology of China, Chengdu, 610054, People’s Republic of China

    Jiandong Wang

Authors
  1. Zhongyue Zhang
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Jiandong Wang
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Yanan Zhao
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Dong Lu
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Zuhong Xiong
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Zhongyue Zhang.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Zhang, Z., Wang, J., Zhao, Y. et al. Numerical Investigation of a Branch-Shaped Filter Based on Metal-Insulator-Metal Waveguide. Plasmonics 6, 773–778 (2011). https://doi.org/10.1007/s11468-011-9263-y

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11468-011-9263-y

Keywords

Search

Navigation