Abstract
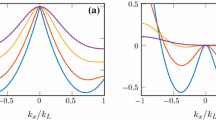
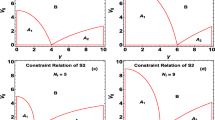
The experimental and theoretical research of spin–orbit-coupled ultracold atomic gases has advanced and expanded rapidly in recent years. Here, we review some of the progress that either was pioneered by our own work, has helped to lay the foundation, or has developed new and relevant techniques. After examining the experimental accessibility of all relevant spin–orbit coupling parameters, we discuss the fundamental properties and general applications of spin–orbit-coupled Bose–Einstein condensates (BECs) over a wide range of physical situations. For the harmonically trapped case, we show that the ground state phase transition is a Dicke-type process and that spin–orbit-coupled BECs provide a unique platform to simulate and study the Dicke model and Dicke phase transitions. For a homogeneous BEC, we discuss the collective excitations, which have been observed experimentally using Bragg spectroscopy. They feature a roton-like minimum, the softening of which provides a potential mechanism to understand the ground state phase transition. On the other hand, if the collective dynamics are excited by a sudden quenching of the spin–orbit coupling parameters, we show that the resulting collective dynamics can be related to the famous Zitterbewegung in the relativistic realm. Finally, we discuss the case of a BEC loaded into a periodic optical potential. Here, the spin–orbit coupling generates isolated flat bands within the lowest Bloch bands whereas the nonlinearity of the system leads to dynamical instabilities of these Bloch waves. The experimental verification of this instability illustrates the lack of Galilean invariance in the system.
Article PDF
Similar content being viewed by others
Avoid common mistakes on your manuscript.
References
A. L. Fetter, Rotating trapped Bose–Einstein condensates, Rev. Mod. Phys. 81(2), 647 (2009)
Y. J. Lin, R. L. Compton, K. Jiménez-García, J. V. Porto, and I. B. Spielman, Synthetic magnetic fields for ultracold neutral atoms, Nature 462(7273), 628 (2009)
M. Aidelsburger, M. Atala, M. Lohse, J. T. Barreiro, B. Paredes, and I. Bloch, Realization of the Hofstadter Hamiltonian with ultracold atoms in optical lattices, Phys. Rev. Lett. 111(18), 185301 (2013)
H. Miyake, G. A. Siviloglou, C. J. Kennedy, W. C. Burton, and W. Ketterle, Realizing the Harper Hamiltonian with laser-assisted tunneling in optical lattices, Phys. Rev. Lett. 111(18), 185302 (2013)
M. Aidelsburger, M. Atala, S. Nascimbène, S. Trotzky, Y.A. Chen, and I. Bloch, Experimental realization of strong effective magnetic fields in an optical lattice, Phys. Rev. Lett. 107(25), 255301 (2011)
I. Žutic, J. Fabian, and S. Das Sarma, Spintronics: Fundamentals and applications, Rev. Mod. Phys. 76(2), 323 (2004)
M. Z. Hasan and C. L. Kane, Colloquium: Topological insulators, Rev. Mod. Phys. 82(4), 3045 (2010)
T. Jungwirth, J. Wunderlich, and K. Olejník, Spin Hall effect devices, Nat. Mater. 11(5), 382 (2012)
Y. J. Lin, K. Jiménez-García, and I. B. Spielman, Spin–orbit-coupled Bose–Einstein condensates, Nature 471(7336), 83 (2011)
J. Ruseckas, G. Juzeliunas, P. Öhberg, and M. Fleischhauer, Non-Abelian gauge potentials for ultracold atoms with degenerate dark states, Phys. Rev. Lett. 95(1), 010404 (2005)
S. L. Zhu, H. Fu, C. J. Wu, S. C. Zhang, and L. M. Duan, Spin Hall effects for cold atoms in a light-induced gauge potential, Phys. Rev. Lett. 97(24), 240401 (2006)
X. J. Liu, X. Liu, L. C. Kwek, and C. H. Oh, Optically induced spin-Hall effect in atoms, Phys. Rev. Lett. 98(2), 026602 (2007)
T. D. Stanescu, B. Anderson, and V. Galitski, Spin–orbit coupled Bose–Einstein condensates, Phys. Rev. A 78(2), 023616 (2008)
J. Larson, J. P. Martikainen, A. Collin, and E. Sjöqvist, Spin–orbit-coupled Bose–Einstein condensate in a tilted optical lattice, Phys. Rev. A 82(4), 043620 (2010)
M. Merkl, A. Jacob, F. E. Zimmer, P. Öhberg, and L. Santos, Chiral confinement in quasirelativistic Bose–Einstein condensates, Phys. Rev. Lett. 104(7), 073603 (2010)
R. A. Williams, L. J. LeBlanc, K. Jiménez-García, M. C. Beeler, A. R. Perry, W. D. Phillips, and I. B. Spielman, Synthetic partial waves in ultracold atomic collisions, Science 335(6066), 314 (2012)
L. J. LeBlanc, M. C. Beeler, K. Jiménez-García, A. R. Perry, S. Sugawa, R. A. Williams, and I. B. Spielman, Direct observation of Zitterbewegung in a Bose–Einstein condensate, New J. Phys. 15(7), 073011 (2013)
M. C. Beeler, R. A. Williams, K. Jiménez-García, L. J. LeBlanc, A. R. Perry, and I. B. Spielman, The spin Hall effect in a quantum gas, Nature 498(7453), 201 (2013)
K. Jiménez-García, L. J. LeBlanc, R. A. Williams, M. C. Beeler, C. Qu, M. Gong, C. Zhang, and I. B. Spielman, Tunable spin–orbit coupling via strong driving in ultracold-atom systems, Phys. Rev. Lett. 114(12), 125301 (2015)
L. W. Cheuk, A. T. Sommer, Z. Hadzibabic, T. Yefsah, W. S. Bakr, and M. W. Zwierlein, Spin-injection spectroscopy of a spin–orbit coupled Fermi gas, Phys. Rev. Lett. 109(9), 095302 (2012)
C. L. Qu, C. Hamner, M. Gong, C. W. Zhang, and P. Engels, Observation of Zitterbewegung in a spin–orbit coupled Bose–Einstein condensate, Phys. Rev. A 88, 021604(R) (2013)
C. Hamner, C. Qu, Y. Zhang, J. J. Chang, M. Gong, C. Zhang, and P. Engels, Dicke-type phase transition in a spin–orbit-coupled Bose–Einstein condensate, Nat. Commun. 5, 4023 (2014)
M. A. Khamehchi, Y. Zhang, C. Hamner, T. Busch, and P. Engels, Measurement of collective excitations in a spin–orbit-coupled Bose–Einstein condensate, Phys. Rev. A 90(6), 063624 (2014)
C. Hamner, Y. Zhang, M. A. Khamehchi, M. J. Davis, and P. Engels, Spin–orbit-coupled Bose–Einstein condensates in a one-dimensional optical lattice, Phys. Rev. Lett. 114(7), 070401 (2015)
A. J. Olson, S. J. Wang, R. J. Niffenegger, C. H. Li, C. H. Greene, and Y. P. Chen, Tunable Landau–Zener transitions in a spin–orbit-coupled Bose–Einstein condensate, Phys. Rev. A 90(1), 013616 (2014)
A. J. Olson, Chuan-Hsun Li, David B. Blasing, R. J. Niffenegger, and Yong P. Chen, Engineering an atominterferometer with modulated light-induced 3π spin–orbit coupling, arXiv: 1502.04722 (2015)
J. Y. Zhang, S. C. Ji, Z. Chen, L. Zhang, Z. D. Du, B. Yan, G. S. Pan, B. Zhao, Y. J. Deng, H. Zhai, S. Chen, and J. W. Pan, Collective dipole oscillations of a spin–orbit coupled Bose–Einstein condensate, Phys. Rev. Lett. 109(11), 115301 (2012)
S. C. Ji, J. Y. Zhang, L. Zhang, Z. D. Du, W. Zheng, Y. J. Deng, H. Zhai, S. Chen, and J. W. Pan, Experimental determination of the finite-temperature phase diagram of a spin–orbit coupled Bose gas, Nat. Phys. 10(4), 314 (2014)
S. C. Ji, L. Zhang, X. T. Xu, Z. Wu, Y. Deng, S. Chen, and J. W. Pan, Softening of roton and phonon modes in a Bose-Einstein condensate with spin–orbit coupling, Phys. Rev. Lett. 114(10), 105301 (2015)
Z. Wu, L. Zhang, W. Sun, X. T. Xu, B. Z. Wang, S. C. Ji, Y. Deng, S. Chen, X. J. Liu, and J. W. Pan, Realization of two-dimensional spin–orbit coupling for Bose–Einstein condensates, arXiv: 1511.08170 (2015)
Z. K. Fu, P. J. Wang, S. J. Chai, L. H. Huang, and J. Zhang, Bose–Einstein condensate in a light-induced vector gauge potential using 1064-nm optical-dipole-trap lasers, Phys. Rev. A 84, 043609 (2011)
P. Wang, Z. Q. Yu, Z. Fu, J. Miao, L. Huang, S. Chai, H. Zhai, and J. Zhang, Spin–orbit coupled degenerate Fermi gases, Phys. Rev. Lett. 109(9), 095301 (2012)
Z. Fu, L. Huang, Z. Meng, P. Wang, X. J. Liu, H. Pu, H. Hu, and J. Zhang, Radio-frequency spectroscopy of a strongly interacting spin–orbit-coupled Fermi gas, Phys. Rev. A 87(5), 053619 (2013)
Z. Fu, L. Huang, Z. Meng, P. Wang, L. Zhang, S. Zhang, H. Zhai, P. Zhang, and J. Zhang, Production of Feshbach molecules induced by spin–orbit coupling in Fermi gases, Nat. Phys. 10(2), 110 (2013)
L. Huang, Z. Meng, P. Wang, P. Peng, S.L. Zhang, L. Chen, D. Li, Q. Zhou, and J. Zhang, Experimental realization of a two-dimensional synthetic spin–orbit coupling in ultracold Fermi gases, Nat. Phys. (2016), arXiv: 1506.02861
Z. Meng, L. Huang, P. Peng, D. Li, L. Chen, Y. Xu, C. Zhang, P. Wang, and J. Zhang, Experimental observation of topological band gap opening in ultracold Fermi gases with two-dimensional spin–orbit coupling, arXiv: 1511.08492 (2015)
X. Luo, L. Wu, J. Chen, Q. Guan, K. Gao, Z.F. Xu, L. You, and R. Wang, Tunable spin–orbit coupling synthesized with a modulating gradient magnetic field, Sci. Rep. 6, 18983 (2016), arXiv: 1502.07091
Y. Xu and C. Zhang, Topological Fulde–Ferrell superfluids of a spin–orbit coupled Fermi gas, Int. J. Mod. Phys. B 29(01), 1530001 (2015)
Y. Li, G. I. Martone, and S. Stringari, Bose-Einstein condensation with spin–orbit coupling, Annual Review of Cold Atoms and Molecules 3, 201 (2015)
C. Wang, C. Gao, C. M. Jian, and H. Zhai, Spin–orbit coupled spinor Bose–Einstein condensates, Phys. Rev. Lett. 105(16), 160403 (2010)
T. L. Ho and S. Zhang, Bose–Einstein condensates with spin–orbit interaction, Phys. Rev. Lett. 107(15), 150403 (2011)
C. J. Wu, I. Mondragon-Shem, and X. F. Zhou, Unconventional Bose–Einstein condensations from spin–orbit coupling, Chin. Phys. Lett. 28(9), 097102 (2011)
Y. Li, L. P. Pitaevskii, and S. Stringari, Quantum tricriticality and phase transitions in spin–orbit coupled Bose–Einstein condensates, Phys. Rev. Lett. 108(22), 225301 (2012)
Q. Zhu, C. Zhang, and B. Wu, Exotic superfluidity in spin–orbit coupled Bose–Einstein condensates, Europhys. Lett. 100(5), 50003 (2012)
L. Wen, Q. Sun, H. Q. Wang, A. C. Ji, and W. M. Liu, Ground state of spin-1 Bose–Einstein condensates with spin–orbit coupling in a Zeeman field, Phys. Rev. A 86, 043602 (2012)
X. L. Cui and Q. Zhou, Enhancement of condensate depletion due to spin–orbit coupling, Phys. Rev. A 87, 031604(R) (2013)
Y. Li, G. I. Martone, L. P. Pitaevskii, and S. Stringari, Superstripes and the excitation spectrum of a spin–orbit-coupled Bose–Einstein condensate, Phys. Rev. Lett. 110(23), 235302 (2013)
G. I. Martone, Y. Li, and S. Stringari, Approach for making visible and stable stripes in a spin–orbit-coupled Bose–Einstein superfluid, Phys. Rev. A 90, 041604(R) (2014)
Z. Lan and P. Öhberg, Raman-dressed spin-1 spin–orbitcoupled quantum gas, Phys. Rev. A 89, 023630 (2014)
S. Sinha, R. Nath, and L. Santos, Trapped twodimensional condensates with synthetic spin–orbit coupling, Phys. Rev. Lett. 107(27), 270401 (2011)
H. Hu, B. Ramachandhran, H. Pu, and X. J. Liu, Spin–orbit coupled weakly interacting Bose–Einstein condensates in harmonic traps, Phys. Rev. Lett. 108(1), 010402 (2012)
Y. Zhang, L. Mao, and C. Zhang, Mean-field dynamics of spin–orbit coupled Bose–Einstein condensates, Phys. Rev. Lett. 108(3), 035302 (2012)
S. Gautam and S. K. Adhikari, Phase separation in a spin–orbit-coupled Bose–Einstein condensate, Phys. Rev. A 90(4), 043619 (2014)
O. V. Marchukov, A. G. Volosniev, D. V. Fedorov, A. S. Jensen, and N. T. Zinner, Statistical properties of spectra in harmonically trapped spin–orbit coupled systems, J. Phys. At. Mol. Opt. Phys. 47(19), 195303 (2014)
W. S. Cole, S. Zhang, A. Paramekanti, and N. Trivedi, Bose–Hubbard models with synthetic spin–orbit coupling: Mott insulators, spin textures, and superfluidity, Phys. Rev. Lett. 109(8), 085302 (2012)
Z. Cai, X. Zhou, and C. Wu, Magnetic phases of bosons with synthetic spin–orbit coupling in optical lattices, Phys. Rev. A 85, 061605(R) (2012)
M. J. Edmonds, J. Otterbach, R. G. Unanyan, M. Fleischhauer, M. Titov, and P. Öhberg, From Anderson to anomalous localization in cold atomic gases with effective spin–orbit coupling, New J. Phys. 14(7), 073056 (2012)
G. B. Zhu, Q. Sun, Y. Y. Zhang, K. S. Chan, W. M. Liu, and A. C. Ji, Spin-based effects and transport properties of a spin–orbit-coupled hexagonal optical lattice, Phys. Rev. A 88(2), 023608 (2013)
L. Zhou, H. Pu, and W. Zhang, Anderson localization of cold atomic gases with effective spin–orbit interaction in a quasiperiodic optical lattice, Phys. Rev. A 87(2), 023625 (2013)
Y. Qian, M. Gong, V. W. Scarola, and C. Zhang, Spin–orbit driven transitions between Mott insulators and finite momentum superfluids of bosons in optical lattices, arXiv: 1312.4011 (2013)
Y. V. Kartashov, V. V. Konotop, and F. K. Abdullaev, Gap solitons in a spin–orbit-coupled Bose–Einstein condensate, Phys. Rev. Lett. 111(6), 060402 (2013)
H. Sakaguchi and B. Li, Vortex lattice solutions to the Gross–Pitaevskii equation with spin–orbit coupling in optical lattices, Phys. Rev. A 87(1), 015602 (2013)
V. E. Lobanov, Y. V. Kartashov, and V. V. Konotop, Fundamental, multipole, and half-vortex gap solitons in spin–orbit coupled Bose–Einstein condensates, Phys. Rev. Lett. 112(18), 180403 (2014)
S. Zhang, W. S. Cole, A. Paramekanti, and N. Trivedi, Spin–orbit coupling in optical lattices, Annual Review of Cold Atoms and Molecules 3, 135 (2015)
D. Toniolo and J. Linder, Superfluidity breakdown and multiple roton gaps in spin–orbit-coupled Bose–Einstein condensates in an optical lattice, Phys. Rev. A 89, 061605(R) (2014)
J. Zhao, S. Hu, J. Chang, P. Zhang, and X. Wang, Ferromagnetism in a two-component Bose–Hubbard model with synthetic spin–orbit coupling, Phys. Rev. A 89(4), 043611 (2014)
Z. Xu, W. S. Cole, and S. Zhang, Mott-superfluid transition for spin–orbit-coupled bosons in one-dimensional optical lattices, Phys. Rev. A 89, 051604(R) (2014)
Y. Cheng, G. Tang, and S. K. Adhikari, Localization of a spin–orbit-coupled Bose–Einstein condensate in a bichromatic optical lattice, Phys. Rev. A 89(6), 063602 (2014)
M. Piraud, Z. Cai, I. P. McCulloch, and U. Schollwöck, Quantum magnetism of bosons with synthetic gauge fields in one-dimensional optical lattices: A density-matrix renormalization-group study, Phys. Rev. A 89(6), 063618 (2014)
W. Han, G. Juzeliunas, W. Zhang, and W. M. Liu, Supersolid with nontrivial topological spin textures in spin–orbit-coupled Bose gases, Phys. Rev. A 91(1), 013607 (2015)
W. Li, L. Chen, Z. Chen, Y. Hu, Z. Zhang, and Z. Liang, Probing the flat band of optically trapped spin–orbitalcoupled Bose gases using Bragg spectroscopy, Phys. Rev. A 91(2), 023629 (2015)
Y. Zhang, Y. Xu, and T. Busch, Gap solitons in spin–orbit-coupled Bose–Einstein condensates in optical lattices, Phys. Rev. A 91(4), 043629 (2015)
D. W. Zhang, L. B. Fu, Z. D. Wang, and S. L. Zhu, Josephson dynamics of a spin–orbit-coupled Bose–Einstein condensate in a double-well potential, Phys. Rev. A 85(4), 043609 (2012)
M. A. Garcia-March, G. Mazzarella, L. Dell’Anna, B. Juliá-Díaz, L. Salasnich, and A. Polls, Josephson physics of spin–orbit-coupled elongated Bose–Einstein condensates, Phys. Rev. A 89(6), 063607 (2014)
R. Citro and A. Naddeo, Spin–orbit coupled Bose–Einstein condensates in a double well, Eur. Phys. J. Spec. Top. 224(3), 503 (2015)
X. Q. Xu and J. H. Han, Spin–orbit coupled Bose–Einstein condensate under rotation, Phys. Rev. Lett. 107(20), 200401 (2011)
J. Radic T. A. Sedrakyan, I. B. Spielman, and V. Galitski, Vortices in spin–orbit-coupled Bose–Einstein condensates, Phys. Rev. A 84(6), 063604 (2011)
X. F. Zhou, J. Zhou, and C. Wu, Vortex structures of rotating spin–orbit-coupled Bose–Einstein condensates, Phys. Rev. A 84(6), 063624 (2011)
B. Ramachandhran, B. Opanchuk, X.J. Liu, H. Pu, P. D. Drummond, and H. Hu, Half-quantum vortex state in a spin–orbit-coupled Bose–Einstein condensate, Phys. Rev. A 85(2), 023606 (2012)
Y. X. Du, H. Yan, D. W. Zhang, C. J. Shan, and S. L. Zhu, Proposal for a rotation-sensing interferometer with spin–orbit-coupled atoms, Phys. Rev. A 85(4), 043619 (2012)
C.-F. Liu, H. Fan, Y.-C. Zhang, D.-S. Wang, and W.-M. Liu, Circular-hyperbolic skyrmion in rotating pseudo-spin-1/2 Bose–Einstein condensates with spin–orbit coupling, Phys. Rev. A 86, 053616 (2012)
L. Dong, L. Zhou, B. Wu, B. Ramachandhran, and H. Pu, Cavity-assisted dynamical spin–orbit coupling in cold atoms, Phys. Rev. A 89, 011602(R) (2014)
F. Mivehvar and D. L. Feder, Synthetic spin–orbit interactions and magnetic fields in ring-cavity QED, Phys. Rev. A 89(1), 013803 (2014)
Y. Deng, J. Cheng, H. Jing, and S. Yi, Bose–Einstein condensates with cavity-mediated spin–orbit coupling, Phys. Rev. Lett. 112(14), 143007 (2014)
B. Padhi and S. Ghosh, Spin–orbit-coupled Bose–Einstein condensates in a cavity: Route to magnetic phases through cavity transmission, Phys. Rev. A 90(2), 023627 (2014)
F. Mivehvar and D. L. Feder, Enhanced stripe phases in spin–orbit-coupled Bose–Einstein condensates in ring cavities, Phys. Rev. A 92(2), 023611 (2015)
Y. Deng, J. Cheng, H. Jing, C. P. Sun, and S. Yi, Spin–orbit-coupled dipolar Bose–Einstein condensates, Phys. Rev. Lett. 108(12), 125301 (2012)
R. M. Wilson, B. M. Anderson, and C. W. Clark, Meron ground state of Rashba spin–orbit-coupled dipolar bosons, Phys. Rev. Lett. 111(18), 185303 (2013)
S. Gopalakrishnan, I. Martin, and E. A. Demler, Quantum quasicrystals of spin–orbit-coupled dipolar bosons, Phys. Rev. Lett. 111(18), 185304 (2013)
H. T. Ng, Topological phases in spin–orbit-coupled dipolar lattice bosons, Phys. Rev. A 90(5), 053625 (2014)
Y. Yousefi, E. Ö. Karabulut, F. Malet, J. Cremon, and S. M. Reimann, Wigner-localized states in spin–orbitcoupled bosonic ultracold atoms with dipolar interaction, Eur. Phys. J. Spec. Top. 224(3), 545 (2015)
Y. Xu, Y. Zhang, and C. Zhang, Bright solitons in a twodimensional spin–orbit-coupled dipolar Bose–Einstein condensate, Phys. Rev. A 92(1), 013633 (2015)
M. Gong, S. Tewari, and C. Zhang, BCS–BEC crossover and topological phase transition in 3D spin–orbit coupled degenerate Fermi gases, Phys. Rev. Lett. 107, 195303 (2011)
H. Hu, L. Jiang, X. J. Liu, and H. Pu, Probing anisotropic superfluidity in atomic Fermi gases with Rashba spin–orbit coupling, Phys. Rev. Lett. 107(19), 195304 (2011)
Z. Q. Yu and H. Zhai, Spin–orbit coupled Fermi gases across a Feshbach resonance, Phys. Rev. Lett. 107(19), 195305 (2011)
J. Dalibard, F. Gerbier, G. Juzeliunas, and P. Öhberg, Colloquium: Artificial gauge potentials for neutral atoms, Rev. Mod. Phys. 83(4), 1523 (2011)
V. Galitski and I. B. Spielman, Spin–orbit coupling in quantum gases, Nature 494(7435), 49 (2013)
X. Zhou, Y. Li, Z. Cai, and C. Wu, Unconventional states of bosons with the synthetic spin–orbit coupling, J. Phys. At. Mol. Opt. Phys. 46(13), 134001 (2013)
N. Goldman, G. Juzeliunas, P. Öhberg, and I. B. Spielman, Light-induced gauge fields for ultracold atoms, Rep. Prog. Phys. 77(12), 126401 (2014)
H. Zhai, Degenerate quantum gases with spin–orbit coupling: A review, Rep. Prog. Phys. 78(2), 026001 (2015)
P. J. Wang and J. Zhang, Spin-orbit coupling in Bose–Einstein condensate and degenerate Fermi gases, Front. Phys. 9(5), 598 (2014)
J. Zhang, H. Hu, X. J. Liu, and H. Pu, Fermi gases with synthetic spin–orbit coupling, Annual Review of Cold Atoms and Molecules 2, 81 (2014)
Y. Zhang, G. Chen, and C. Zhang, Tunable spin–orbit coupling and quantum phase transition in a trapped Bose–Einstein condensate, Sci. Rep. 3, 1937 (2013)
Y. Zhang and C. Zhang, Bose–Einstein condensates in spin–orbit-coupled optical lattices: Flat bands and superfluidity, Phys. Rev. A 87(2), 023611 (2013)
R. H. Dicke, Coherence in spontaneous radiation processes, Phys. Rev. 93(1), 99 (1954)
C. Emary, and T. Brandes, Chaos and the quantum phase transition in the Dicke model, Phys. Rev. E 67(6), 066203 (2003)
M. Gross and S. Haroche, Superradiance: An essay on the theory of collective spontaneous emission, Phys. Rep. 93(5), 301 (1982)
K. Baumann, C. Guerlin, F. Brennecke, and T. Esslinger, Dicke quantum phase transition with a superfluid gas in an optical cavity, Nature 464(7293), 1301 (2010)
Y. Li, G. Martone, and S. Stringari, Sum rules, dipole oscillation and spin polarizability of a spin–orbit coupled quantum gas, Europhys. Lett. 99(5), 56008 (2012)
S. Stringari, Collective excitations of a trapped Bosecondensed gas, Phys. Rev. Lett. 77(12), 2360 (1996)
D. Guéry-Odelin and S. Stringari, Scissors mode and superfluidity of a trapped Bose–Einstein condensed gas, Phys. Rev. Lett. 83(22), 4452 (1999)
O. M. Maragò, S. A. Hopkins, J. Arlt, E. Hodby, G. Hechenblaikner, and C. J. Foot, Observation of the Scissors mode and evidence for superfluidity of a trapped Bose–Einstein condensed gas, Phys. Rev. Lett. 84(10), 2056 (2000)
J. Lian, L. Yu, J. Q. Liang, G. Chen, and S. Jia, Orbitinduced spin squeezing in a spin–orbit coupled Bose–Einstein condensate, Sci. Rep. 3, 3166 (2013)
Y. Huang and Z. D. Hu, Spin and field squeezing in a spin–orbit coupled Bose–Einstein condensate, Sci. Rep. 5, 8006 (2015)
W. Zheng, Z. Q. Yu, X. Cui, and H. Zhai, Properties of Bose gases with the Raman-induced spin–orbit coupling, J. Phys. At. Mol. Opt. Phys. 46(13), 134007 (2013)
J. Higbie and D. M. Stamper-Kurn, Periodically dressed Bose–Einstein condensate: A superfluid with an anisotropic and variable critical velocity, Phys. Rev. Lett. 88(9), 090401 (2002)
G. I. Martone, Y. Li, L. P. Pitaevskii, and S. Stringari, Anisotropic dynamics of a spin–orbit-coupled Bose–Einstein condensate, Phys. Rev. A 86(6), 063621 (2012)
L. D. Landau, The theory of superfluidity of Helium II, J. Phys. (USSR) 5, 71 (1941)
H. Palevsky, K. Otnes, and K. E. Larsson, Excitation of rotons in Helium II by cold neutrons, Phys. Rev. 112(1), 11 (1958)
J. L. Yarnell, G. P. Arnold, P. J. Bendt, and E. C. Kerr, Excitations in liquid Helium: Neutron scattering measurements, Phys. Rev. 113(6), 1379 (1959)
D. G. Henshaw and A. D. B. Woods, Modes of atomic motions in liquid helium by inelastic scattering of neutrons, Phys. Rev. 121(5), 1266 (1961)
L. Santos, G. V. Shlyapnikov, and M. Lewenstein, Roton–Maxon spectrum and stability of trapped dipolar Bose–Einstein condensates, Phys. Rev. Lett. 90(25), 250403 (2003)
D. H. J. O’Dell, S. Giovanazzi, and G. Kurizki, Rotons in gaseous Bose–Einstein condensates irradiated by a laser, Phys. Rev. Lett. 90(11), 110402 (2003)
P. B. Blakie, D. Baillie, and R. N. Bisset, Roton spectroscopy in a harmonically trapped dipolar Bose–Einstein condensate, Phys. Rev. A 86(2), 021604 (2012)
M. Jona-Lasinio, K. Lakomy, and L. Santos, Time-offlight roton spectroscopy in dipolar Bose–Einstein condensates, Phys. Rev. A 88(2), 025603 (2013)
Y. Pomeau and S. Rica, Model of superflow with rotons, Phys. Rev. Lett. 71(2), 247 (1993)
Y. Pomeau and S. Rica, Dynamics of a model of supersolid, Phys. Rev. Lett. 72(15), 2426 (1994)
R. Ozeri, N. Katz, J. Steinhauer, and N. Davidson, Colloquium: Bulk Bogoliubov excitations in a Bose–Einstein condensate, Rev. Mod. Phys. 77(1), 187 (2005)
J. Stenger, S. Inouye, A. P. Chikkatur, D. M. Stamper-Kurn, D. E. Pritchard, and W. Ketterle, Bragg spectroscopy of a Bose–Einstein condensate, Phys. Rev. Lett. 82(23), 4569 (1999)
D. M. Stamper-Kurn, A. P. Chikkatur, A. Görlitz, S. Inouye, S. Gupta, D. E. Pritchard, and W. Ketterle, Excitation of phonons in a Bose–Einstein condensate by light scattering, Phys. Rev. Lett. 83(15), 2876 (1999)
J. Steinhauer, R. Ozeri, N. Katz, and N. Davidson, Excitation spectrum of a Bose–Einstein condensate, Phys. Rev. Lett. 88(12), 120407 (2002)
J. Steinhauer, N. Katz, R. Ozeri, N. Davidson, C. Tozzo, and F. Dalfovo, Bragg spectroscopy of the Multibranch–Bogoliubov spectrum of elongated Bose–Einstein condensates, Phys. Rev. Lett. 90(6), 060404 (2003)
S. B. Papp, J. M. Pino, R. J. Wild, S. Ronen, C. E. Wieman, D. S. Jin, and E. A. Cornell, Bragg spectroscopy of a strongly interacting Rb85 Bose–Einstein condensate, Phys. Rev. Lett. 101, 135301 (2008)
X. Du, S. Wan, E. Yesilada, C. Ryu, D. J. Heinzen, Z. Liang, and B. Wu, Bragg spectroscopy of a superfluid Bose–Hubbard gas, New J. Phys. 12(8), 083025 (2010)
N. Fabbri, D. Clément, L. Fallani, C. Fort, M. Modugno, K. M. R. van der Stam, and M. Inguscio, Excitations of Bose–Einstein condensates in a one-dimensional periodic potential, Phys. Rev. A 79(4), 043623 (2009)
D. Clément, N. Fabbri, L. Fallani, C. Fort, and M. Inguscio, Exploring correlated 1D Bose gases from the superfluid to the Mott-insulator state by inelastic light scattering, Phys. Rev. Lett. 102, 155301 (2009)
P. T. Ernst, S. Götze, J. S. Krauser, K. Pyka, D. S. Lühmann, D. Pfannkuche, and K. Sengstock, Probing superfluids in optical lattices by momentum-resolved Bragg spectroscopy, Nat. Phys. 6(1), 56 (2010)
G. Bismut, B. Laburthe-Tolra, E. Maréchal, P. Pedri, O. Gorceix, and L. Vernac, Anisotropic excitation spectrum of a dipolar quantum Bose gas, Phys. Rev. Lett. 109(15), 155302 (2012)
L. C. Ha, L. W. Clark, C. V. Parker, B. M. Anderson, and C. Chin, Roton-Maxon excitation spectrum of Bose condensates in a shaken optical lattice, Phys. Rev. Lett. 114(5), 055301 (2015)
Z. Chen and H. Zhai, Collective-mode dynamics in a spin–orbit coupled Bose–Einstein condensate, Phys. Rev. A 86, 041604(R) (2012)
V. Achilleos, D. J. Frantzeskakis, and P. G. Kevrekidis, Beating dark–dark solitons and Zitterbewegung in spin–orbit-coupled Bose–Einstein condensates, Phys. Rev. A 89(3), 033636 (2014)
Sh. Mardonov, M. Palmero, M. Modugno, E. Ya. Sherman, and J. G. Muga, Interference of spin–orbitcoupled Bose–Einstein condensates, Europhys. Lett. 106(6), 60004 (2014)
Y. Li, C. Qu, Y. Zhang, and C. Zhang, Dynamical spindensity waves in a spin–orbit-coupled Bose–Einstein condensate, Phys. Rev. A 92(1), 013635 (2015)
Sh. Mardonov, E. Ya. Sherman, J. G. Muga, H. W. Wang, Y. Ban, and X. Chen, Collapse of spin–orbitcoupled Bose–Einstein condensates, Phys. Rev. A 91(4), 043604 (2015)
S. Cao, C. J. Shan, D. W. Zhang, X. Qin, and J. Xu, Dynamical generation of dark solitons in spin–orbitcoupled Bose–Einstein condensates, J. Opt. Soc. Am. B 32(2), 201 (2015)
E. Schrödinger, Über die kräftefreie Bewegung in der relativistischen Quantenmechanik, Sitzungsber. Preuss. Akad. Wiss. Phys.-Math. Kl. 24, 418 (1930)
W. Zawadzki and T. M. Rusin, Zitterbewegung (trembling motion) of electrons in semiconductors: A review, J. Phys.: Condens. Matter 23(14), 143201 (2011)
R. Gerritsma, G. Kirchmair, F. Zähringer, E. Solano, R. Blatt, and C. F. Roos, Quantum simulation of the Dirac equation, Nature 463(7277), 68 (2010)
F. Dreisow, M. Heinrich, R. Keil, A. Tünnermann, S. Nolte, S. Longhi, and A. Szameit, Classical simulation of relativistic Zitterbewegung in photonic lattices, Phys. Rev. Lett. 105, 143902 (2010)
J. Schliemann, D. Loss, and R. M. Westervelt, Zitterbewegung of electronic wave packets in III-V zincblende semiconductor quantum wells, Phys. Rev. Lett. 94, 206801 (2005)
J. Vaishnav and C. Clark, Observing Zitterbewegung with ultracold atoms, Phys. Rev. Lett. 100(15), 153002 (2008)
Y. J. Lin, R. L. Compton, K. Jiménez-García, W. D. Phillips, J. V. Porto, and I. B. Spielman, A synthetic electric force acting on neutral atoms, Nat. Phys. 7(7), 531 (2011)
Y.-C. Zhang, S.-W. Song, C.-F. Liu, and W.-M. Liu, Zitterbewegung effect in spin–orbit-coupled spin-1 ultracold atoms, Phys. Rev. A 87, 023612 (2013)
O. Morsch and M. Oberthaler, Dynamics of Bose–Einstein condensates in optical lattices, Rev. Mod. Phys. 78, 179 (2006)
C. L. Kane and E. J. Mele, Quantum spin Hall effect in graphene, Phys. Rev. Lett. 95(22), 226801 (2005)
Q. Zhu and B. Wu, Superfluidity of Bose–Einstein condensates in ultracold atomic gases, Chin. Phys. B 24(5), 050507 (2015)
F. Lin, C. Zhang, and V. W. Scarola, Emergent kinetics and fractionalized charge in 1D spin–orbit coupled Flatband optical lattices, Phys. Rev. Lett. 112, 110404 (2014)
Biao Wu and Qian Niu, Landau and dynamical instabilities of the superflow of Bose–Einstein condensates in optical lattices, Phys. Rev. A 64, 061603(R) (2001)
A. Smerzi, A. Trombettoni, P. G. Kevrekidis, and A. R. Bishop, Dynamical superfluid–insulator transition in a chain of weakly coupled Bose–Einstein condensates, Phys. Rev. Lett. 89(17), 170402 (2002)
M. Machholm, C. J. Pethick, and H. Smith, Band structure, elementary excitations, and stability of a Bose–Einstein condensate in a periodic potential, Phys. Rev. A 67(5), 053613 (2003)
M. Modugno, C. Tozzo, and F. Dalfovo, Role of transverse excitations in the instability of Bose–Einstein condensates moving in optical lattices, Phys. Rev. A 70(4), 043625 (2004)
A. J. Ferris, M. J. Davis, R. W. Geursen, P. B. Blakie, and A. C. Wilson, Dynamical instabilities of Bose–Einstein condensates at the band edge in one dimensional optical lattices, Phys. Rev. A 77, 012712 (2008)
S. Hooley and K. A. Benedict, Dynamical instabilities in a two-component Bose–Einstein condensate in a one dimensional optical lattice, Phys. Rev. A 75, 033621 (2007)
J. Ruostekoski and Z. Dutton, Dynamical and energetic instabilities in multicomponent Bose–Einstein condensates in optical lattices, Phys. Rev. A 76(6), 063607 (2007)
G. Barontini and M. Modugno, Instabilities of a matter wave in a matter grating, Phys. Rev. A 80(6), 063613 (2009)
S. Burger, F. S. Cataliotti, C. Fort, F. Minardi, M. Inguscio, M. L. Chiofalo, and M. P. Tosi, Superfluid and dissipative dynamics of a Bose–Einstein condensate in a periodic optical potential, Phys. Rev. Lett. 86(20), 4447 (2001)
L. Fallani, L. De Sarlo, J. E. Lye, M. Modugno, R. Saers, C. Fort, and M. Inguscio, Observation of dynamical instability for a Bose–Einstein condensate in a moving 1D optical lattice, Phys. Rev. Lett. 93, 140406 (2004)
J. Mun, P. Medley, G. K. Campbell, L. G. Marcassa, D. E. Pritchard, and W. Ketterle, Phase diagram for a Bose–Einstein condensate moving in an optical lattice, Phys. Rev. Lett. 99(15), 150604 (2007)
T. Ozawa, L. P. Pitaevskii, and S. Stringari, Supercurrent and dynamical instability of spin–orbit-coupled ultracold Bose gases, Phys. Rev. A 87(6), 063610 (2013)
P. Cladé, S. Guellati-Khélifa, F. Nez, and F. Biraben, Large momentum beam splitter using Bloch oscillations, Phys. Rev. Lett. 102(24), 240402 (2009)
Z. Chen and Z. Liang, Ground-state phase diagram of a spin–orbit-coupled bosonic superfluid in an optical lattice, Phys. Rev. A 93(1), 013601 (2016)
G. Juzeliunas, J. Ruseckas, and J. Dalibard, Generalized Rashba–Dresselhaus spin–orbit coupling for cold atoms, Phys. Rev. A 81(5), 053403 (2010)
D. L. Campbell, G. Juzeliunas, and I. B. Spielman, Realistic Rashba and Dresselhaus spin-orbit coupling for neutral atoms, Phys. Rev. A 84(2), 025602 (2011)
B. M. Anderson, G. Juzeliunas, V. M. Galitski, and I. B. Spielman, Synthetic 3D spin–orbit coupling, Phys. Rev. Lett. 108, 235301 (2012)
Z. F. Xu, L. You, and M. Ueda, Atomic spin–orbit coupling synthesized with magnetic-field-gradient pulses, Phys. Rev. A 87(6), 063634 (2013)
B. M. Anderson, I. B. Spielman, and G. Juzeliunas, Magnetically generated spin–orbit coupling for ultracold atoms, Phys. Rev. Lett. 111, 125301 (2013)
G. Juzeliunas, J. Ruseckas, M. Lindberg, L. Santos, and P. Öhberg, Quasirelativistic behavior of cold atoms in light fields, Phys. Rev. A 77, 011802(R) (2008)
Chuanwei Zhang, Spin–orbit coupling and perpendicular Zeeman field for fermionic cold atoms: Observation of the intrinsic anomalous Hall effect, Phys. Rev. A 82, 021607(R) (2010)
Author information
Authors and Affiliations
Corresponding authors
Rights and permissions
Open Access This article is distributed under the terms of the Creative Commons Attribution 4.0 International License (https://creativecommons.org/licenses/by/4.0), which permits use, duplication, adaptation, distribution, and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made.
About this article
Cite this article
Zhang, Y., Mossman, M.E., Busch, T. et al. Properties of spin–orbit-coupled Bose–Einstein condensates. Front. Phys. 11, 118103 (2016). https://doi.org/10.1007/s11467-016-0560-y
Received:
Accepted:
Published:
DOI: https://doi.org/10.1007/s11467-016-0560-y