Abstract
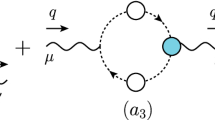
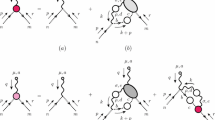
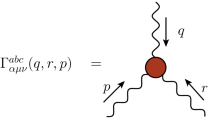
We present a pedagogical overview of the nonperturbative mechanism that endows gluons with a dynamical mass. This analysis is performed based on pure Yang–Mills theories in the Landau gauge, within the theoretical framework that emerges from the combination of the pinch technique with the background field method. In particular, we concentrate on the Schwinger–Dyson equation satisfied by the gluon propagator and examine the necessary conditions for obtaining finite solutions within the infrared region. The role of seagull diagrams receives particular attention, as do the identities that enforce the cancellation of all potential quadratic divergences.We stress the necessity of introducing nonperturbative massless poles in the fully dressed vertices of the theory in order to trigger the Schwinger mechanism, and explain in detail the instrumental role of these poles in maintaining the Becchi–Rouet–Stora–Tyutin symmetry at every step of the mass-generating procedure. The dynamical equation governing the evolution of the gluon mass is derived, and its solutions are determined numerically following implementation of a set of simplifying assumptions. The obtained mass function is positive definite, and exhibits a power law running that is consistent with general arguments based on the operator product expansion in the ultraviolet region. A possible connection between confinement and the presence of an inflection point in the gluon propagator is briefly discussed.
Article PDF
Similar content being viewed by others
Avoid common mistakes on your manuscript.
References
J. M. Cornwall, Dynamical mass generation in continuum QCD, Phys. Rev. D 26, 1453 (1982)
A. C. Aguilar, A. A. Natale, and P. S. Rodrigues da Silva, Relating a gluon mass scale to an infrared fixed point in pure gauge QCD, Phys. Rev. Lett. 90, 152001 (2003), arXiv: hep-ph/0212105
A. C. Aguilar, A. Mihara, and A. A. Natale, Phenomenological tests for the freezing of the QCD running coupling constant, Int. J. Mod. Phys. A 19, 249 (2004)
D. Binosi, Lei Chang, J. Papavassiliou, and C. D. Roberts, Bridging a gap between continuum-QCD and ab initio predictions of hadron observables, Phys. Lett. B 742, 183 (2015), arXiv: 1412.4782 [nucl-th]
I. C. Cloet and C. D. Roberts, Explanation and prediction of observables using continuum strong QCD, Prog. Part. Nucl. Phys. 77, 1 (2014), arXiv: 1310.2651 [nucl-th]
C. D. Roberts, Hadron physics and QCD: Just the basic facts, in: 37th BrazilianWorkshop on Nuclear Physics Maresias, S˜ao Paulo, Brazil, September 8–12, 2014 (2015), arXiv: 1501.06581 [nucl-th]
R. Jackiw and K. Johnson, Dynamical model of spontaneously broken gauge symmetries, Phys. Rev. D 8, 2386 (1973)
R. Jackiw, Dynamical symmetry breaking, in: Erice, Proceedings, Laws of Hadronic Matter, New York, 1975, 225–251 and MIT Cambridge - COO-3069-190 (73, REC. AUG 74), 1973, p. 23
J. M. Cornwall and R. E. Norton, Spontaneous symmetry breaking without scalar mesons, Phys. Rev. D 8, 3338 (1973)
E. Eichten and F. Feinberg, Dynamical symmetry breaking of nonAbelian gauge symmetries, Phys. Rev. D 10, 3254 (1974)
E. C. Poggio, E. Tomboulis, and S. H. H. Tye, Dynamical symmetry breaking in non-Abelian field theories, Phys. Rev. D 11, 2839 (1975)
C. W. Bernard, Adjoint Wilson lines and the effective gluon mass, Nucl. Phys. B 219, 341 (1983)
J. F. Donoghue, The gluon “mass” in the bag model, Phys. Rev. D 29, 2559 (1984)
I. L. Bogolubsky, E. M. Ilgenfritz, M. Muller-Preussker, and A. Sternbeck, Lattice gluodynamics computation of Landau gauge Green’s functions in the deep infrared, Phys. Lett. B 676, 69 (2009), arXiv: 0901.0736 [hep-lat]
I. L. Bogolubsky, E. M. Ilgenfritz, M. Muller-Preussker, and A. Sternbeck, The Landau gauge gluon and ghost propagators in 4D SU(3) gluodynamics in large lattice volumes, arXiv: 0710.1968 [hep-lat]
P. O. Bowman, et al., Scaling behavior and positivity violation of the gluon propagator in full QCD, Phys. Rev. D 76, 094505 (2007), arXiv: hep-lat/0703022
O. Oliveira and P. J. Silva, The Lattice infrared Landau gauge gluon propagator: The infinite volume limit, PoS LAT 2009, 226 (2009), arXiv: 0910.2897 [hep-lat]
A. Cucchieri and T. Mendes, What’s up with IR gluon and ghost propagators in Landau gauge? A puzzling answer from huge lattices, PoS LAT 2007, 297 (2007), arXiv: 0710.0412 [hep-lat]
A. Cucchieri and T. Mendes, Constraints on the IR behavior of the gluon propagator in Yang–Mills theories, Phys. Rev. Lett. 100, 241601 (2008), arXiv: 0712.3517 [hep-lat]
A. Cucchieri and T. Mendes, Landau-gauge propagators in Yang–Mills theories at beta = 0: Massive solution versus conformal scaling, Phys. Rev. D 81, 016005 (2010), arXiv: 0904.4033 [hep-lat]
A. Cucchieri and T. Mendes, Numerical test of the Gribov–Zwanziger scenario in Landau gauge, PoS QCD-TNT 09, 026 (2009), arXiv: 1001.2584 [hep-lat]
A. C. Aguilar, D. Binosi, and J. Papavassiliou, Gluon and ghost propagators in the Landau gauge: Deriving lattice results from Schwinger–Dyson equations, Phys. Rev. D 78, 025010 (2008), arXiv: 0802.1870 [hep-ph]
A. P. Szczepaniak and E. S. Swanson, Coulomb gauge QCD, confinement, and the constituent representation, Phys. Rev. D 65, 025012 (2002), arXiv: hep-ph/0107078 [hep-ph]
P. Maris and C. D. Roberts, Dyson–Schwinger equations: A Tool for hadron physics, Int. J. Mod. Phys. E12, 297 (2003), arXiv: nucl-th/0301049 [nucl-th]
A. P. Szczepaniak, Confinement and gluon propagator in Coulomb gauge QCD, Phys. Rev. D 69, 074031 (2004), arXiv: hep-ph/0306030 [hep-ph]
A. C. Aguilar and A. A. Natale, A dynamical gluon mass solution in a coupled system of the Schwinger–Dyson equations, J. High Energy Phys. 08, 057 (2004), arXiv: hepph/0408254
K.-I. Kondo, Gauge-invariant gluon mass, infrared Abelian dominance and stability of magnetic vacuum, Phys. Rev. D 74, 125003 (2006), arXiv: hep-th/0609166
J. Braun, H. Gies, and J. M. Pawlowski, Quark confinement from color confinement, Phys. Lett. B 684, 262 (2010), arXiv: 0708.2413 [hep-th]
D. Epple, H. Reinhardt, W. Schleifenbaum, and A. P. Szczepaniak, Subcritical solution of the Yang–Mills Schroedinger equation in the Coulomb gauge, Phys. Rev. D 77, 085007 (2008), arXiv: 0712.3694 [hep-th]
Ph. Boucaud, et al., On the IR behaviour of the Landaugauge ghost propagator, J. High Energy Phys. 06, 099 (2008), arXiv: 0803.2161 [hep-ph]
D. Dudal, J. A. Gracey, S. Paolo Sorella, N. Vandersickel, and H. Verschelde, A refinement of the Gribov–Zwanziger approach in the Landau gauge: Infrared propagators in harmony with the lattice results, Phys. Rev. D 78, 065047 (2008), arXiv: 0806.4348 [hepth]
C. S. Fischer, A. Maas, and J. M. Pawlowski, On the infrared behavior of Landau gauge Yang–Mills theory, Annals Phys. 324, 2408 (2009), arXiv: 0810.1987 [hep-ph]
A. P. Szczepaniak and Hrayr H. Matevosyan, A model for QCD ground state with magnetic disorder, Phys. Rev. D 81, 094007 (2010), arXiv: 1003.1901 [hep-ph]
P. Watson and H. Reinhardt, The Coulomb gauge ghost Dyson–Schwinger equation, Phys. Rev. D 82, 125010 (2010), arXiv: 1007.2583 [hep-th]
J. Rodriguez-Quintero, On the massive gluon propagator, the PT-BFM scheme and the lowmomentum behaviour of decoupling and scaling DSE solutions, J. High Energy Phys. 1101, 105 (2011), arXiv: 1005.4598 [hep-ph]
D. R. Campagnari, and H. Reinhardt, Non-Gaussian wave functionals in Coulomb gauge Yang–Mills theory, Phys. Rev. D 82, 105021 (2010), arXiv: 1009.4599 [hep-th]
M. Tissier and N. Wschebor, Infrared propagators of Yang–Mills theory from perturbation theory, Phys. Rev. D 82, 101701 (2010), arXiv: 1004.1607 [hep-ph]
M. R. Pennington and D. J. Wilson, Are the dressed gluon and ghost propagators in the Landau gauge presently determined in the confinement regime of QCD? Phys. Rev. D 84, 119901 (2011), arXiv: 1109.2117 [hep-ph]
P. Watson and H. Reinhardt, Leading order infrared quantum chromodynamics in Coulomb gauge, Phys. Rev. D 85, 025014 (2012), arXiv: 1111.6078 [hep-ph]
K.-I. Kondo, A low-energy effective Yang–Mills theory for quark and gluon confinement, Phys. Rev. D 84, 061702 (2011), arXiv: 1103.3829 [hep-th]
F. Siringo, Gluon propagator in Feynman gauge by the method of stationary variance, Phys. Rev. D 90, 094021 (2014), arXiv: 1408.5313 [hep-ph]
J. S. Schwinger, Gauge invariance and mass, Phys. Rev. 125, 397 (1962)
J. S. Schwinger, Gauge invariance and mass (2), Phys. Rev. 128, 2425 (1962)
Lectures given by J. P. at the Workshop Dyson–Schwinger Equations in Modern Mathematics and Physics, Trento, September 22–26, 2014
C. D. Roberts and A. G. Williams, Dyson–Schwinger equations and their application to hadronic physics, Prog. Part. Nucl. Phys. 33, 477 (1994), arXiv: hep-ph/9403224
J. M. Cornwall and J. Papavassiliou, Gauge invariant three gluon vertex in QCD, Phys. Rev. D 40, 3474 (1989)
D. Binosi and J. Papavassiliou, The pinch technique to all orders, Phys. Rev. D 66, 111901(R) (2002), arXiv: hepph/0208189
D. Binosi and J. Papavassiliou, Pinch technique selfenergies and vertices to all orders in perturbation theory, J. Phys. G 30, 203 (2004), arXiv: hep-ph/0301096 [hep-ph]
D. Binosi and J. Papavassiliou, Pinch technique: Theory and applications, Phys. Rep. 479, 1 (2009), arXiv: 0909.2536 [hep-ph]
L. F. Abbott, The background field method beyond one loop, Nucl. Phys. B 185, 189 (1981)
L. F. Abbott, Introduction to the background field method, Acta Phys. Polon. B 13, 33 (1982)
A. C. Aguilar and J. Papavassiliou, Gluon mass generation in the PT-BFM scheme, J. High Energy Phys. 12, 012 (2006), arXiv: hep-ph/0610040
D. Binosi and J. Papavassiliou, Gauge-invariant truncation scheme for the Schwinger–Dyson equations of QCD, Phys. Rev. D 77, 061702 (2008), arXiv: 0712.2707 [hep-ph]
D. Binosi and J. Papavassiliou, New Schwinger–Dyson equations for non-Abelian gauge theories, J. High Energy Phys. 0811, 063 (2008), arXiv: 0805.3994 [hep-ph]
A. C. Aguilar and J. Papavassiliou, Gluon mass generation without seagull divergences, Phys. Rev. D 81, 034003 (2010), arXiv: 0910.4142 [hep-ph]
A. C. Aguilar, D. Binosi, and J. Papavassiliou, The dynamical equation of the effective gluon mass, Phys. Rev. D 84, 085026 (2011), arXiv: 1107.3968 [hep-ph]
D. Binosi, D. Iba˜nez, and J. Papavassiliou, The all-order equation of the effective gluon mass, Phys. Rev. D 86, 085033 (2012), arXiv: 1208.1451 [hep-ph]
N. Nakanishi, Covariant quantization of the electromagnetic field in the landau gauge, Prog. Theor. Phys. 35, 1111 (1966)
B. Lautrup, Canonical quantum electrodynamics in covariant gauges, Mat. Fys. Medd. Dan. Vid. Selsk. 35, 1 (1966)
C. Becchi, A. Rouet, and R. Stora, Renormalization of the Abelian Higgs–Kibble Model, Commun. Math. Phys. 42, 127 (1975)
I. V. Tyutin, Gauge invariance in field theory and statistical physics in operator formalism, LEBEDEV-75-39
K. Fujikawa, B. W. Lee, and A. I. Sanda, Generalized renormalizable gauge formulation of spontaneously broken gauge theories, Phys. Rev. D 6, 2923 (1972)
D. Binosi and A. Quadri, AntiBRST symmetry and background field method, Phys. Rev. D 88, 085036 (2013), arXiv: 1309.1021 [hep-th]
D. Binosi and J. Papavassiliou, Pinch technique and the Batalin–Vilkovisky formalism, Phys. Rev. D 66, 025024 (2002), arXiv: hep-ph/0204128 [hep-ph]
P. A. Grassi, Tobias Hurth, and Matthias Steinhauser, Practical algebraic renormalization, Ann. Phys. 288, 197 (2001), arXiv: hep-ph/9907426
J. S. Ball and T.-W. Chiu, Analytic properties of the vertex function in gauge theories (2), Phys. Rev. D 22, 2550 (1980)
M. Pelaez, M. Tissier, and N. Wschebor, Three-point correlation functions in Yang–Mills theory, Phys. Rev. D 88, 125003 (2013), arXiv: 1310.2594 [hep-th]
A. C. Aguilar, D. Binosi, D. Iba˜nez, and J. Papavassiliou, Effects of divergent ghost loops on the Green’s functions of QCD, Phys. Rev. D 89, 085008 (2014), arXiv: 1312.1212 [hep-ph]
G. Eichmann, R. Williams, R. Alkofer, and M. Vujinovic, The threegluon vertex in Landau gauge, Phys. Rev. D 89, 105014 (2014), arXiv: 1402.1365 [hep-ph]
A. Blum, M. Q. Huber, M. Mitter, and L. von Smekal, Gluonic three-point correlations in pure Landau gauge QCD, Phys. Rev. D 89, 061703 (2014), arXiv: 1401.0713 [hep-ph]
P. A. Grassi, T. Hurth, and A. Quadri, On the Landau background gauge fixing and the IR properties of YM Green functions, Phys. Rev. D 70, 105014 (2004), arXiv: hepth/0405104
A. C. Aguilar, D. Binosi, J. Papavassiliou, and J. Rodriguez-Quintero, Non-perturbative comparison of QCD effective charges, Phys. Rev. D 80, 085018 (2009), arXiv: 0906.2633 [hep-ph]
A. C. Aguilar, D. Binosi, and J. Papavassiliou, Indirect determination of the Kugo–Ojima function from lattice data, J. High Energy Phys. 0911, 066 (2009), arXiv: 0907.0153 [hep-ph]
A. C. Aguilar, D. Binosi, and J. Papavassiliou, QCD effective charges from lattice data, J. High Energy Phys. 1007, 002 (2010), arXiv: 1004.1105 [hep-ph]
D. Binosi and J. Papavassiliou, Gauge invariant Ansatz for a special three-gluon vertex, J. High Energy Phys. 1103, 121 (2011), arXiv: 1102.5662 [hep-ph]
K. G. Wilson, Quantum field theory models in less than four-dimensions, Phys. Rev. D 7, 2911 (1973)
J. C. Collins, Renormalization: An Introduction to Renormalization, the Renormalization Group, and the Operator Product Expansion, 1984
A. C. Aguilar, D. Ibanez, V. Mathieu, and J. Papavassiliou, Massless bound-state excitations and the Schwinger mechanism in QCD, Phys. Rev. D 85, 014018 (2012), arXiv: 1110.2633 [hep-ph]
D. Iba˜nez and J. Papavassiliou, Gluon mass generation in the massless bound-state formalism, Phys. Rev. D 87, 034008 (2013), arXiv: 1211.5314 [hep-ph]
A. C. Aguilar, D. Binosi, and J. Papavassiliou, Renormalization group analysis of the gluon mass equation, Phys. Rev. D 89, 085032 (2014), arXiv: 1401.3631 [hep-ph]
D. Binosi, D. Iba˜nez, and J. Papavassiliou, Nonperturbative study of the four gluon vertex, J. High Energy Phys. 1409, 059 (2014), arXiv: 1407.3677 [hep-ph]
A. K. Cyrol, Markus Q. Huber, and Lorenz von Smekal, A Dyson–Schwinger study of the four-gluon vertex, Eur. Phys. J. C 75, 102 (2015), arXiv: 1408.5409 [hep-ph]
J. M. Cornwall and Wei-Shu Hou, Extension of the gauge technique to broken symmetry and finite temperature, Phys. Rev. D 34, 585 (1986)
M. Lavelle, Gauge invariant effective gluon mass from the operator product expansion, Phys. Rev. D 44, 26 (1991)
A. C. Aguilar and J. Papavassiliou, Power-law running of the effective gluon mass, Eur. Phys. J. A 35, 189 (2008), arXiv: 0708.4320 [hep-ph]
M. J. Lavelle and M. Schaden, Propagators and condensates in QCD, Phys. Lett. B 208, 297 (1988)
E. Bagan and T. G. Steele, QCD condensates and the Slavnov–Taylor identities, Phys. Lett. B 219, 497 (1989)
S. J. Brodsky, C. D. Roberts, R. Shrock, and P. C. Tandy, Essence of the vacuum quark condensate, Phys. Rev. C 82, 022201 (2010), arXiv: 1005.4610 [nucl-th]
L. Del Debbio, M. Faber, J. Greensite, and S. Olejnik, Center dominance and Z(2) vortices in SU(2) lattice gauge theory, Phys. Rev. D 55, 2298 (1997), arXiv: hep-lat/9610005 [hep-lat]
K. Langfeld, H. Reinhardt, and J. Gattnar, Gluon propagators and quark confinement, Nucl. Phys. B 621, 131 (2002), arXiv: hep-ph/0107141 [hep-ph]
See, for example, J. Greensite, The confinement problem in lattice gauge theory, Prog. Theor. Phys. Suppl. 1 (2003), and references therein
J. Gattnar, K. Langfeld, and H. Reinhardt, Signals of con nement in Green functions of SU(2) Yang–Mills theory, Phys. Rev. Lett. 93, 061601 (2004), arXiv: hep-lat/0403011
J. Greensite, H. Matevosyan, S. Olejnik, M. Quandt, H. Reinhardt, et al., Testing Proposals for the Yang–Mills Vacuum Wavefunctional by Measurement of the Vacuum, Phys. Rev. D 83, 114509 (2011), arXiv: 1102.3941 [hep-lat]
A. Ayala, A. Bashir, D. Binosi, M. Cristoforetti, and J. Rodriguez-Quintero, Quark avour effects on gluon and ghost propagators, Phys. Rev. D 86, 074512 (2012), arXiv: 1208.0795 [hep-ph]
A. C. Aguilar, D. Binosi, and J. Papavassiliou, Unquenching the gluon propagator with Schwinger–Dyson equations, Phys. Rev. D 86, 014032 (2012), arXiv: 1204.3868 [hep-ph]
A. C. Aguilar, D. Binosi, and J. Papavassiliou, Gluon mass generation in the presence of dynamical quarks, Phys. Rev. D 88, 074010 (2013), arXiv: 1304.5936 [hep-ph]
P. Bicudo, D. Binosi, N. Cardoso, O. Oliveira, and P. J. Silva, The lattice gluon propagator in renormalizable ξ gauges, arXiv: 1505.05897 [hep-lat]
A. C. Aguilar, D. Binosi, and J. Papavassiliou, Yang–Mills two-point functions in linear covariant gauges, Phys. Rev. D 91, 085014 (2015), arXiv: 1501.07150 [hep-ph]
M. Q. Huber, Gluon and ghost propagators in linear covariant gauges, Phys. Rev. D 91, 085018 (2015), arXiv: 1502.04057 [hep-ph]
F. Siringo, Second order gluon polarization for SU(N) theory in linear covariant gauge, arXiv: 1507.00122 [hep-ph]
M. A. L. Capri, D. Dudal, D. Fiorentini, M. S. Guimaraes, I. F. Justo, A. D. Pereira, B. W. Mintz, L. F. Palhares, R. F. Sobreiro, and S. P. Sorella, Exact nilpotent nonperturbative BRST symmetry for the Gribov–Zwanziger action in the linear covariant gauge, Phys. Rev. D 92, 045039 (2015), arXiv: 1506.06995 [hep-th]
S. J. Brodsky and R. Shrock, Maximum wavelength of confined quarks and gluons and properties of quantum chromodynamics, Phys. Lett. B 666, 95 (2008), arXiv: 0806.1535 [hep-th]
Author information
Authors and Affiliations
Corresponding author
Additional information
This article is published with open access at www.springer.com/11467 and journal.hep.com.cn/fop
Rights and permissions
Open Access This article is distributed under the terms of the Creative Commons Attribution 4.0 International License (https://creativecommons.org/licenses/by/4.0), which permits use, duplication, adaptation, distribution, and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made.
About this article
Cite this article
Aguilar, A.C., Binosi, D. & Papavassiliou, J. The gluon mass generation mechanism: A concise primer. Front. Phys. 11, 111203 (2016). https://doi.org/10.1007/s11467-015-0517-6
Received:
Accepted:
Published:
DOI: https://doi.org/10.1007/s11467-015-0517-6