Abstract
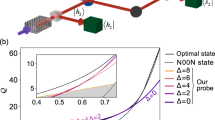
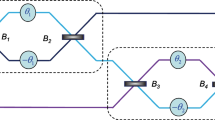
With squeezed states or entangled states being the source, quantum metrology, imaging and sensing can break the standard quantum limit (SQL), even reach the Heisenberg limit (HL), which is difficult to achieve by traditional methods. However, the photon loss or phase fluctuation caused by the atmospheric attenuation and turbulence cannot be ignored in the actual application. Atmospheric transmittance and phase fluctuation are related to the detection distance, and the phase sensitivity becomes worse as the distance increases. As the functions of distance, the photon loss and phase fluctuation are uniformly expressed according to the introduction of atmospheric attenuation coefficient, turbulence structure constant and receive aperture size in this paper. The density matrixes and phase sensitivities of N00N states and M&M′ states in the atmospheric environment are proposed in terms of distance variables. Then the quantitative computation of super-sensitive distance is carried out. SQL-contour is proposed to describe the super-sensitive ability of the quantum interferometer for the affection from both photon loss and phase fluctuation. The simulation results show that, in atmospheric environment the super-sensitive distance can reach hundreds of meters. M&M′ states with less total photon number are more likely to reflect the advantage of super-sensitivity. SQL-contour can provide references for interferometric source choosing.
创新点
以马赫曾德干涉仪(MZI)为基础, 以探测距离为基本变量, 给出了在光子损耗及相位波动共同影响下的N00N态与M&M’态的密度矩阵及相位探测精度, 并计算了不同条件下的超灵敏作用距离。同时提出以SQL等高线表征上述两种因素共同影响下量子干涉仪超灵敏探测性能的方法。仿真结果表明, 在典型大气环境下, 光量子干涉的超灵敏作用距离能够达到百米量级且总光子数较低的M&M’态在大气环境中最易体现出其超灵敏探测能力.
Similar content being viewed by others
References
Lanzagorta M. Quantum Radar. 1st ed. San Rafael: Morgan & Claypool Publishers, 2011. 1–5
Lloyd G V, Maccone S. Quantum-enhanced measurements: beating the standard quantum limit. Science, 2004, 306: 1330–1336
Sacchi M F. Entanglement can enhance the distinguishability of entanglement-breaking channels. Phys Rev Ser A, 2005, 72: 14305
Smith J F. Quantum entangled radar theory and a correction method for the effects of the atmosphere on entanglement. In: Proceedings of SPIE Defense, Security, and Sensing, International Society for Optics and Photonics, Orlando, 2009. 7342: 457
Smith J F. Quantum interferometer and radar theory based on N00N, M and M or linear combinations of entangled states. In: Proceedings of SPIE Defense, Security, and Sensing, International Society for Optics and Photonics, Orlando, 2010. 7702: 131–142
Gilbert G, Hamrick M, Weinstein Y. Use of maximally entangled N-photon states for practical quantum interferometry. J Opt Soc America B, 2008, 28: 1336–1340
Rubin M A, Kaushik S. Loss-induced limits to phase measurement precision with maximally entangled states. Phys Rev A, 2007, 75: 497–500
Parks A D, Spence S E, Troupe J E, et al. Tripartite loss model for Mach-Zehnder interferometers with application to phase sensitivity. Rev Sci Instrum, 2005, 76: 043103
Huver S D, Wildfeuer C F, Dowling J P. Entangled fock states for robust quantum optical metrology, imaging, and sensing. Phys Rev A, 2008, 78: 063828
Jiang K, Brignac C J, Weng Y, et al. Strategies for choosing path-entangled number states for optimal robust quantum optical metrology in the presence of loss. Phys Rev A, 2012, 86: 013826
Bardhan B R, Jiang K, Dowling J P. Effects of phase fluctuations on phase sensitivity and visibility of path-entangled photon Fock states. Phys Rev A, 2013, 88: 195–201
Bardhan B R, Dowling J P. Effects of phase fluctuations on the sensitivity of NOON state in a noisy environment. In: Proceedings of the Rochester Conferences on Coherence and Quantum Optics and the Quantum Information and Measurement meeting. New York: Optical Society of America, 2013. M6.19
Fuwa K, Valle B L. The pertinence of the Beer-Lambert law. Anal Chem, 1963, 35: 942–946
Andrews L C, Phillips R L. Laser Beam Propagation Through Random Media. 2nd ed. Bellingham: SPIE Press, 2005. 168–169
Afek I, Silberberg Y. High-NOON states by mixing quantum and classical light. Science, 2010, 328: 879–881
Ruizhong R. Light Propagation in the Turbulent Atmosphere. 2nd ed. Hefei: Anhui Science & Technology Publishing House, 2005. 66–68
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Hu, Y., Xu, S. Super-sensitive detection of quantum interferometer in atmospheric environment. Sci. China Inf. Sci. 60, 032502 (2017). https://doi.org/10.1007/s11432-015-0878-8
Received:
Accepted:
Published:
DOI: https://doi.org/10.1007/s11432-015-0878-8
Keywords
- quantum interferometer
- standard quantum limit
- entangled states
- phase sensitivity
- atmospheric transmission