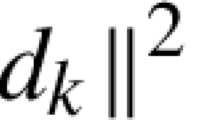Skip to main content
SpringerLink
Log in

An accurate a posteriori error estimator for the Steklov eigenvalue problem and its applications

  • Articles
  • Published:
Science China Mathematics Aims and scope Submit manuscript

Abstract

In this paper, a type of accurate a posteriori error estimator is proposed for the Steklov eigenvalue problem based on the complementary approach, which provides an asymptotic exact estimate for the approximate eigenpair. Besides, we design a type of cascadic adaptive finite element method for the Steklov eigenvalue problem based on the proposed a posteriori error estimator. In this new cascadic adaptive scheme, instead of solving the Steklov eigenvalue problem in each adaptive space directly, we only need to do some smoothing steps for linearized boundary value problems on a series of adaptive spaces and solve some Steklov eigenvalue problems on a low dimensional space. Furthermore, the proposed a posteriori error estimator provides the way to refine mesh and control the number of smoothing steps for the cascadic adaptive method. Some numerical examples are presented to validate the efficiency of the algorithm in this paper.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Adams R-A. Sobolev Spaces. New York: Academic Press, 1975

    MATH  Google Scholar 

  2. Ahn H. Vibration of a pendulum consisting of a bob suspended from a wire. Quart Appl Math, 1981, 39: 109–117

    MathSciNet  MATH  Google Scholar 

  3. Andreev A, Todorov T. Isoparametric finite-element approximation of a Steklov eigenvalue problem. IMA J Numer Anal, 2004, 24: 309–322

    MathSciNet  MATH  Google Scholar 

  4. Armentano M, Padra C. A posteriori error estimates for the Steklov eigenvalue problem. Appl Numer Math, 2008, 58: 593–601

    MathSciNet  MATH  Google Scholar 

  5. Arnold D, Falk S, Winther R. Preconditioning in H (div) and applications. Math Comp, 1997, 66: 957–984

    MathSciNet  MATH  Google Scholar 

  6. Babuška I, Osborn J. Finite element-Galerkin approximation of the eigenvalues and eigenvectors of selfadjoint problems. Math Comp, 1989, 52: 275–297

    MathSciNet  MATH  Google Scholar 

  7. Babuška I, Osborn J. Eigenvalue problems. In: Handbook of Numerical Analysis, vol. II. Finite Element Methods (Part 1). Amsterdam: North-Holland, 1991, 641–787

    Google Scholar 

  8. Babuška I, Rheinboldt W. Error estimates for adaptive finite element computations. SIAM J Numer Anal, 1978, 15: 736–754

    MathSciNet  MATH  Google Scholar 

  9. Babuška I, Rheinboldt W. A-posteriori error estimates for the finite element method. Int J Numer Methods Eng, 1978, 12: 1597–1615

    MATH  Google Scholar 

  10. Bermudez A, Rodriguez R, Santamarina D. A finite element solution of an added mass formulation for coupled fluid-solid vibrations. Numer Math, 2000, 887: 201–227

    MathSciNet  MATH  Google Scholar 

  11. Bi H, Li H, Yang Y. An adaptive algorithm based on the shifted inverse iteration for the Steklov eigenvalue problem. Appl Numer Math, 2016, 105: 64–81

    MathSciNet  MATH  Google Scholar 

  12. Bi H, Yang Y. A two-grid method of the non-conforming Crouzeix-Raviart element for the Steklov eigenvalue problem. Appl Math Comput, 2011, 217: 9669–9678

    MathSciNet  MATH  Google Scholar 

  13. Bornemann F, Deuhard P. The cascadic multigrid method for elliptic problems. Numer Math, 1996, 75: 135–152

    MathSciNet  MATH  Google Scholar 

  14. Bornemann F, Erdmann B, Kornhuber R. A posteriori error estimates for elliptic problems in two and three space dimensions. SIAM J Numer Anal, 1996, 33: 1188–1204

    MathSciNet  MATH  Google Scholar 

  15. Bramble J-H, Osborn, J-E. Approximation of Steklov eigenvalues of non-selfadjoint second order elliptic operators. In: The Mathematical Foundations of the Finite Element Method with Applications to Partial Differential Equations. New York: Academic Press, 1972, 387–408

    Google Scholar 

  16. Bramble J-H, Zhang X. The analysis of multigrid methods. Handb Numer Anal, 2000, 7: 173–415

    MathSciNet  MATH  Google Scholar 

  17. Brenner S, Scott L. The Mathematical Theory of Finite Element Methods. New York: Springer-Verlag, 1994

    MATH  Google Scholar 

  18. Brezzi F, Fortin M. Mixed and Hybrid Finite Element Methods. New York: Springer-Verlag, 1991

    MATH  Google Scholar 

  19. Cascon J, Kreuzer C, Nochetto R, et al. Quasi-optimal convergence rate for an adaptive finite element method. SIAM J Numer Anal, 2008, 46: 2524–2550

    MathSciNet  MATH  Google Scholar 

  20. Ciarlet P-G. The Finite Element Method for Elliptic Problems. Amsterdam: North-Holland, 1978

    MATH  Google Scholar 

  21. Conca C, Planchard J, Vanninathan M. Fluids and Periodic Structures. Chichester: John Wiley & Sons, 1995

    MATH  Google Scholar 

  22. Dai X, Xu J, Zhou A. Convergence and optimal complexity of adaptive finite element eigenvalue computations. Numer Math, 2008, 110: 313–355

    MathSciNet  MATH  Google Scholar 

  23. Deuflhard P, Leinen P, Yserentant H. Concepts of an adaptive hierarchical finite element code. IMPACT Comput Sci Engrg, 1989, 1: 3–35

    MATH  Google Scholar 

  24. Dórfler W. A convergent adaptive algorithm for Poisson’s equation. SIAM J Numer Anal, 1996, 33: 1106–1124

    MathSciNet  MATH  Google Scholar 

  25. Garau E-M, Morin P. Convergence and quasi-optimality of adaptive FEM for Steklov eigenvalue problems. IMA J Numer Anal, 2011, 31: 914–946

    MathSciNet  MATH  Google Scholar 

  26. Grisvard P. Singularities in Boundary Value Problems. Berlin: Springer-Verlag, 1985

    MATH  Google Scholar 

  27. Hackbusch W. Multi-grid Methods and Applications. Berlin: Springer-Verlag, 1985

    MATH  Google Scholar 

  28. Han H, Guan Z, He B. Boundary element approximation of Steklov eigenvalue problem. J Chinese Univ Appl Math Ser A, 1994, 9: 231–238

    MathSciNet  Google Scholar 

  29. Han X, Li Y, Xie H. A multilevel correction method for Steklov eigenvalue problem by nonconforming finite element methods. Numer Math Theory Methods Appl, 2015, 8: 383–405

    MathSciNet  MATH  Google Scholar 

  30. Han X, Xie H, Xu F. A cascadic multigrid method for eigenvalue problem. J Comput Math, 2017, 322: 747–759

    MathSciNet  MATH  Google Scholar 

  31. Heuveline V, Rannacher R. A posteriori error control for finite element approximations of ellipic eigenvalue problems. Adv Comput Math, 2001, 15: 107–138

    MathSciNet  MATH  Google Scholar 

  32. Hong Q, Xie H, Yue M, et al. Fully computable error bounds for eigenvalue problem. Int J Numer Anal Model, 2018, 15: 2560–276

    MathSciNet  MATH  Google Scholar 

  33. Huang J, Lü T. The mechanical quadrature methods and their extrapolation for solving BIE of Steklov eigenvalue problems. J Comput Math, 2004, 22: 719–726

    MathSciNet  MATH  Google Scholar 

  34. Lin Q, Xie H. A multilevel correction type of adaptive finite element method for Steklov eigenvalue problems. In: Proceedings of the International Conference Applications of Mathematics. Prague: Institute of Mathematics, Academy of Sciences of the Czech Republic, 2012, 134–143

    Google Scholar 

  35. Mekchay K, Nochetto R. Convergence of adaptive finite element methods for general second order linear elliptic PDEs. SIAM J Numer Anal, 2005, 43: 1803–1827

    MathSciNet  MATH  Google Scholar 

  36. Morin P, Nochetto R, Siebert K. Convergence of adaptive finite element methods. SIAM Rev, 2002, 44: 631–658

    MathSciNet  MATH  Google Scholar 

  37. Neittaanmaüki P, Repin S. Reliable Methods for Computer Simulation, Error Control and A Posteriori Estimates. Studies in Mathematics and its Applications, vol. 33. Amsterdam: Elsevier, 2004

    Google Scholar 

  38. Rognes M, Kirby R, Logg A. Efficient assembly of H (div) and H (curl) conforming finite elements. SIAM J Sci Comput, 2009, 31: 4130–4151

    MathSciNet  MATH  Google Scholar 

  39. Shaidurov V. Some estimates of the rate of convergence for the cascadic conjugate-gradient method. Comput Math Appl, 1996, 31: 161–171

    MathSciNet  MATH  Google Scholar 

  40. Shaidurov V, Tobiska L. The convergence of the cascadic conjugate-gradient method applied to elliptic problems in domains with re-entrant corners. Math Comp, 2000, 69: 501–520

    MathSciNet  MATH  Google Scholar 

  41. Stevension R. Optimality of a standard adaptive finite element method. Found Comput Math, 2007, 7: 245–269

    MathSciNet  Google Scholar 

  42. Tang W, Guan Z, Han H. Boundary element approximation of Steklov eigenvalue problem for Helmholtz equation. J Comput Math, 1998, 2: 165–178

    MathSciNet  MATH  Google Scholar 

  43. Vejchodský T. Complementarity based a posteriori error estimates and their properties. Math Comput Simulation, 2012, 82: 2033–2046

    MathSciNet  MATH  Google Scholar 

  44. Wang L, Xu X. The Basic Mathematical Theory of Finite Element Methods (in Chinese). Beijing: Science Press, 2004

    Google Scholar 

  45. Xie H. A type of multilevel method for the Steklov eigenvalue problem. IMA J Numer Anal, 2014, 34: 592–608

    MathSciNet  MATH  Google Scholar 

  46. Xu J. Iterative methods by space decomposition and subspace correction. SIAM Rev, 1992, 34: 581–613

    MathSciNet  MATH  Google Scholar 

  47. Xu J, Chen L, Nechetto R. Optimal Multilevel Methods for H (grad), H (curl), and H (div) Systems on Graded and Unstructured Grids. Multiscale, Nonlinear and Adaptive Approximation. Berlin: Springer, 2009

    Google Scholar 

  48. Xu J, Zhou A. A two-grid discretization scheme for eigenvalue problems. Math Comp, 2001, 70: 17–25

    MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

This work was supported by National Natural Science Foundation of China (Grant Nos. 11801021 and 11571027), Foundation for Fundamental Research of Beijing University of Technology (Grant No. 006000546318504) and International Research Cooperation Seed Fund of Beijing University of Technology (Grant No. 2018B32).

Author information

Authors and Affiliations

  1. Beijing Institute for Scientific and Engineering Computing, College of Applied Sciences, Beijing University of Technology, Beijing, 100124, China

    Fei Xu & Qiumei Huang

Authors
  1. Fei Xu
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Qiumei Huang
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Qiumei Huang.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Xu, F., Huang, Q. An accurate a posteriori error estimator for the Steklov eigenvalue problem and its applications. Sci. China Math. 64, 623–638 (2021). https://doi.org/10.1007/s11425-018-9525-2

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11425-018-9525-2

Keywords

MSC(2010)

Search

Navigation