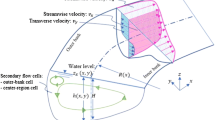
Abstract
Exposure time is an important characteristic for hydrodynamics that has simultaneous impacts on the biochemical processes in tidal systems. To eliminate man-made errors, decrease computational effort, and increase simulation efficiency, exposure time was evaluated under different hydrodynamic conditions for a bay to investigate the impact of the external domain on the accuracy of the computational results for exposure time. The exposure time was explicitly defined and computed using a hydrodynamic model and tracer experiments for a set of ten external domain sizes (EDS), five external domain lengths (EDL), and three special hydrodynamic conditions. The results indicated that the external domain had a significant influence on the exposure time, and the intensity of this influence was related to hydrodynamic conditions. The sensitivity of the exposure time to the external domain increased with increasing tidal range, while freshwater inflows decreased this sensitivity. However, the variation trends for exposure time with different EDS and EDL were independent of the hydrodynamic conditions. Considering the computational efficiency (maximum), the calculated error (minimum) of the exposure time, and the impact of the boundary conditions (minimum), the recommended EDS and EDL range from 9 to 13 times the initial domain size and 1.30 to 1.45 times the length in the bay, respectively. The research regarding exposure time and external domains not only helps to eliminate the errors caused by man-made factors and reduce the computational effort but also provides a reference for understanding the interrelationship between coastal waters, reciprocating flow, and the water environment.








Similar content being viewed by others
References
Allen CM (1982) Numerical simulation of contaminant dispersion in estuary flows. Proc R Soc A 381(1780):179–194. https://doi.org/10.1098/rspa.1982.0064
Andutta FP, Ridd PV, Deleersnijder E, Prandle D (2014) Contaminant exchange rates in estuaries—new formulae accounting for advection and dispersion. Prog Oceanogr 120:139–153. https://doi.org/10.1016/j.pocean.2013.08.009
Andutta FP, Helfer F, de Miranda LB, Deleersnijder E, Thomas C, Lemckert C (2016) An assessment of transport timescales and return coefficient in adjacent tropical estuaries. Cont Shelf Res 124:49–62. https://doi.org/10.1016/j.csr.2016.05.006
Ascione Kenov I, Garcia AC, Neves R (2012) Residence time of water in the Mondego estuary (Portugal). Estuar Coast Shelf Sci 106:13–22. https://doi.org/10.1016/j.ecss.2012.04.008
Bacher C, Filgueira R, Guyondet T (2016) Probabilistic approach of water residence time and connectivity using Markov chains with application to tidal embayments. J Mar Syst 153:25–41. https://doi.org/10.1016/j.jmarsys.2015.09.002
Baleo JN, Humeau P, Le Cloirec P (2001) Numerical and experimental hydrodynamic studies of a lagoon pilot. Water Res 35(9):2268–2276. https://doi.org/10.1016/S0043-1354(00)00502-9
Bolin B, Rodhe H (1973) A note on the concepts of age and residence time in natural reservoirs. Tellus 25(1):58–62
Camacho RA, Martin JL (2013) Hydrodynamic modeling of first-order transport timescales in the St. Louis Bay estuary, Mississippi. J Environ Eng ASCE 139(3):317–331. https://doi.org/10.1061/(ASCE)EE.1943-7870.0000647
Chen WB, Liu WC, Hsu MH (2015) Modeling assessment of a saltwater intrusion and a transport time scale response to sea-level rise in a tidal estuary. Environ Fluid Mech 15(3):491–514. https://doi.org/10.1007/s10652-014-9367-y
Cornaton F, Perrochet P (2006) Groundwater age, life expectancy and transit time distributions in advective–dispersive systems: 1. Generalized reservoir theory. Adv Water Resour 29(9):1267–1291. https://doi.org/10.1016/j.advwatres.2005.10.009
Cucco A, Umgiesser G, Ferrarin C, Perilli A, Canu DM, Solidoro C (2009) Eulerian and lagrangian transport time scales of a tidal active coastal basin. Ecol Model 220(7):913–922. https://doi.org/10.1016/j.ecolmodel.2009.01.008
de Brauwere A, de Brye B, Blaise S, Deleersnijder E (2011) Residence time, exposure time and connectivity in the Scheldt estuary. J Mar Syst 84(3–4):85–95. https://doi.org/10.1016/j.jmarsys.2010.10.001
de Brye B, de Brauwere A, Gourgue O, Delhez EJM, Deleersnijder E (2012) Water renewal timescales in the Scheldt estuary. J Mar Syst 94:74–86. https://doi.org/10.1016/j.jmarsys.2012.03.002
Deleersnijder E, Beckers J-M, Delhez EJM (2006) On the behaviour of the residence time at the bottom of the mixed layer. Environ Fluid Mech 6(6):541–547. https://doi.org/10.1007/s10652-006-9003-6
Delhez EJM (2006) Transient residence and exposure times. Ocean Sci 2(1):1–9. https://doi.org/10.5194/os-2-1-2006
Delhez EJM (2013) On the concept of exposure time. Cont Shelf Res 71:27–36. https://doi.org/10.1016/j.csr.2013.09.026
Delhez EJM, Deleersnijder E (2010) Residence time and exposure time of sinking phytoplankton in the euphotic layer. J Theor Biol 262(3):505–516. https://doi.org/10.1016/j.jtbi.2009.10.004
Delhez ÉJM, Heemink AW, Deleersnijder É (2004) Residence time in a semi-enclosed domain from the solution of an adjoint problem. Estuar Coast Shelf Sci 61(4):691–702. https://doi.org/10.1016/j.ecss.2004.07.013
Delhez JM, de Brye B, de Brauwere A, Deleersnijder E (2014) Residence time vs influence time. J Mar Syst 132:185–195. https://doi.org/10.1016/j.jmarsys.2013.12.005
Etemad-Shahidi A, Pirnia M, Moshfeghi H, Lemckert C (2014) Investigation of hydraulics transport time scales within the Arvand River estuary, Iran. Hydrol Process 28(25):6006–6015. https://doi.org/10.1002/hyp.10095
Gao X, Chen Y, Zhang C (2013) Water renewal timescales in an ecological reconstructed lagoon in China. J Hydroinf 15(3):991–1001. https://doi.org/10.2166/hydro.2013.136
Gao X, Xu L, Zhang C (2015) Modelling the effect of water diversion projects on renewal capacity in an urban artificial lake in China. J Hydroinf 17(6):990–1002. https://doi.org/10.2166/hydro.2015.004
Gao X, Xu L, Zhang C (2016) Estimating renewal timescales with residence time and connectivity in an urban man-made lake in China. Environ Sci Pollut Res 23(14):13973–13983. https://doi.org/10.1007/s11356-016-6569-5
Gourgue O, Deleersnijder E, White L (2007) Toward a generic method for studying water renewal, with application to the epilimnion of Lake Tanganyika. Estuar Coast Shelf Sci 74(4):628–640. https://doi.org/10.1016/j.ecss.2007.05.009
Hamrick JM (1992) A three-dimensional environmental fluid dynamics computer code: theoretical and computational aspect. Special report in applied marine science and ocean engineering. No. 317. The College of William and Mary, VIMS, 63 pp
Hart JA, Phlips EJ, Badylak S, Dix N, Petrinec K, Mathews AL, Green W, Srifa A (2015) Phytoplankton biomass and composition in a well-flushed, sub-tropical estuary: the contrasting effects of hydrology, nutrient loads and allochthonous influences. Mar Environ Res 112(Pt A):9–20. https://doi.org/10.1016/j.marenvres.2015.08.010
Heemink AW (1990) Stochastic modelling of dispersion in shallow water. Stoch Env Res Risk A 4(2):161–174. https://doi.org/10.1007/BF01543289
Lancelot C, Billen G (1984) Activity of heterotrophic bacteria and its coupling to primary production during the spring phytoplankton bloom in the southern bight of the North Sea. Limnol Oceanogr 29(4):721–730. https://doi.org/10.4319/lo.1984.29.4.0721
Liu S, Butler D, Memon FA, Makropoulos C, Avery L, Jefferson B (2010) Impacts of residence dime during storage on potential of water saving for grey water recycling system. Water Res 44(1):267–277. https://doi.org/10.1016/j.watres.2009.09.023
Liu Z, Wang H, Guo X, Wang Q, Gao H (2012) The age of Yellow River water in the Bohai Sea. J Geophys Res Oceans 117(C11):317–323. https://doi.org/10.1029/2012JC008263
Lucas LV (2010) Implications of estuarine transport for water quality. In: Valle-Levin-son A (ed) Contemporary issues in estuarine physic. Cambridge University Press, UK, pp 273–307
Lucas LV, Thompson JK, Brown LR (2009) Why are diverse relationships observed between phytoplankton biomass and transport time? Limnol Oceanogr 54(1):381–390. https://doi.org/10.4319/lo.2009.54.1.0381
Luther KH, Haitjema HM (1998) Numerical experiments on the residence time distributions of heterogeneous groundwatersheds. J Hydrol 207(1–2):1–17. https://doi.org/10.1016/S0022-1694(98)00112-7
Majewsky M, Galle T, Bayerle M, Goel R, Fischer K, Vanrolleghem PA (2011) Xenobiotic removal efficiencies in wastewater treatment plants: residence time distributions as a guiding principle for sampling strategies. Water Res 45(18):6152–6162. https://doi.org/10.1016/j.watres.2011.09.005
Meyers SD, Luther ME (2008) A numerical simulation of residual circulation in Tampa Bay. Part II: Lagrangian residence time. Estuar Coasts 31(5):815–827. https://doi.org/10.1007/s12237-008-9085-0
Monsen NE, Cloern JE, Lucas LV, Monismith SG (2002) A comment on the use of flushing time, residence time, and age as transport time scales. Limnol Oceanogr 47(5):1545–1553. https://doi.org/10.4319/lo.2002.47.5.1545 http://www.jstor.org/stable/3068975
Nauman EB (1981) Residence time distributions in systems governed by the dispersion equation. Chem Eng Sci 36(6):957–966. https://doi.org/10.1016/0009-2509(81)80080-2
Odebrecht C, Abreu PC, Carstensen J (2015) Retention time generates short-term phytoplankton blooms in a shallow microtidal subtropical estuary. Estuar Coast Shelf Sci 162:35–44. https://doi.org/10.1016/j.ecss.2015.03.004
Oliveira A, Baptista AM (1997) Diagnostic modeling of residence times in estuaries. Water Resour Res 33(8):1935–1946. https://doi.org/10.1029/97WR00653
Pilotti M, Simoncelli S, Valerio G (2014) A simple approach to the evaluation of the actual water renewal time of natural stratified lakes. Water Resour Res 50(4):2830–2849. https://doi.org/10.1002/2013WR014471
Quinlan EL, Phlips EJ (2007) Phytoplankton assemblages across the marine to low-salinity transition zone in a blackwater dominated estuary. J Plankton Res 29(5):401–416. https://doi.org/10.1093/plankt/fbm024
Ren Y, Lin B, Sun J, Pan S (2014) Predicting water age distribution in the Pearl River estuary using a three-dimensional model. J Mar Syst 139:276–287. https://doi.org/10.1016/j.jmarsys.2014.07.005
Reynolds CS (2006) Ecology of phytoplankton. Cambridge University Press, New York. https://doi.org/10.1017/CBO9780511542145
Shen J, Haas L (2004) Calculating age and residence time in the tidal York River using three-dimensional model experiments. Estuar Coast Shelf Sci 61(3):449–461. https://doi.org/10.1016/j.ecss.2004.06.010
Shen J, Lin J (2006) Modeling study of the influences of tide and stratification on age of water in the tidal James River. Estuar Coast Shelf Sci 68(1–2):101–112. https://doi.org/10.1016/j.ecss.2006.01.014
Spivakovskaya D, Heemink AW, Deleersnijder E (2007) Lagrangian modelling of multi-dimensional advection-diffusion with space-varying diffusivities: theory and idealized test cases. Ocean Dyn 57(3):189–203. https://doi.org/10.1007/s10236-007-0102-9
Sun J, Lin B, Li K, Jiang G (2014) A modelling study of residence time and exposure time in the Pearl River estuary, China. J Hydro Environ Res 8(3):281–291. https://doi.org/10.1016/j.jher.2013.06.003
Umgiesser G, Ferrarin C, Cucco A, De Pascalis F, Bellafiore D, Ghezzo M, Bajo M (2014) Comparative hydrodynamics of 10 Mediterranean lagoons by means of numerical modeling. J Geophys Res Oceans 119(4):2212–2226. https://doi.org/10.1002/2013JC009512
Viero DP, Defina A (2016) Water age, exposure time, and local flushing time in semi-enclosed, tidal basins with negligible freshwater inflow. J Mar Syst 156:16–29. https://doi.org/10.1016/j.jmarsys.2015.11.006
Wan Y, Qiu C, Doering P, Ashton M, Sun D, Coley T (2013) Modeling residence time with a three-dimensional hydrodynamic model: linkage with chlorophyll a in a subtropical estuary. Ecol Model 268:93–102. https://doi.org/10.1016/j.ecolmodel.2013.08.008
Wang CF, Hsu MH, Kuo AY (2004) Residence time of the Danshuei River estuary, Taiwan. Estuar Coast Shelf Sci 60(3):381–393. https://doi.org/10.1016/j.ecss.2004.01.013
Wang Y, Shen JA, He Q (2010) A numerical model study of the transport timescale and change of estuarine circulation due to waterway constructions in the Changjiang estuary, China. J Mar Syst 82(3):154–170. https://doi.org/10.1016/j.jmarsys.2010.04.012
Xavier M, Emma RN, Jean-Pascal T, Olivier P, Aymeric J, Christophe M (2007) Water residence time: a regulatory factor of the DOM to POM transfer efficiency. Limnol Oceanogr 52(2):808–819. https://doi.org/10.4319/lo.2007.52.2.0808
Acknowledgments
This research was supported by the Science Fund for Creative Research Groups of the National Natural Science Foundation of China (No. 51621092), the National Natural Science Foundation of China (No. 51679160), and the Tianjin key research and development program (No. 16YFXTSF00310). Special thanks are addressed to the High Performance Computing Center of Tianjin University, China.
Author information
Authors and Affiliations
Corresponding author
Additional information
Responsible editor: Marcus Schulz
Electronic supplementary material
ESM 1
(DOCX 3195 kb)
Rights and permissions
About this article
Cite this article
Gao, X., Zhao, G., Zhang, C. et al. Modeling the exposure time in a tidal system: the impacts of external domain, tidal range, and inflows. Environ Sci Pollut Res 25, 11128–11142 (2018). https://doi.org/10.1007/s11356-018-1426-3
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11356-018-1426-3