Closed-Form Outage Probability Expressions for Multihop Cognitive Radio Network with Best Path Selection Schemes in RF Energy Harvesting Environment

Abstract

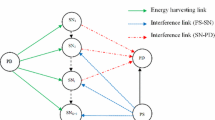
In this paper, a comparative study of parallel best path selection (PBPS) and immediate best path selection IBPS in terms of outage probability and throughput has been done in a multi hop cognitive radio network. The closed form expressions for the outage probability of secondary network in PBPS protocol as well as IBPS protocol in multihop scenario have been derived. We also find the impact of the number of parallel paths in multihop scenario on the outage probability and throughput of secondary network. The performance of PBPS scheme is compared with IBPS scheme. An optimum value of initial harvesting time for secondary nodes $(\tau )$ to achieve maximum throughput is also found for both the path selection schemes.

Appendix

1.1 A.1 Appendix I: Proof of proposition 1 in (14)

Proof

Considering the interference contributed by PT, the cdf of SINR become analytically untraceable. To avoid the mathematical complexity we do not consider interference. From the (7), we can write

$$\begin{aligned} \begin{aligned} {F^{PBPS}_{{\gamma _{({l^{**}},m + 1)}}}}({\gamma _{th}}){\mathrm{{ }}}&= {P_r}\left\{ {{\gamma _{({l^{**}},m + 1)}}< {\gamma _{th}}} \right\} \\&= {P_r}\left\{ {\frac{{min(AZ,\frac{{{I_P}}}{Y})X}}{{{N_o}}}< {\gamma _{th}}} \right\} \\&=\Pr \left\{ {\min \left( {AZ,\frac{{{I_P}}}{Y}} \right) X< {\gamma _{th}}{N_0}} \right\} \\&= \underbrace{\Pr \left\{ {X< \frac{{{\gamma _{th}}{N_0}}}{{Az}},\;Y< \frac{{{I_P}}}{{Az}}} \right\} }_{{P^{PBPS}_1}} + \underbrace{\Pr \left\{ {X < \frac{{{\gamma _{th}}{N_0}y}}{{{I_P}}},\;Z \ge \;\frac{{{I_P}}}{{Ay}}} \right\} }_{{P^{PBPS}_2}} \end{aligned} \end{aligned}$$

(19)

In $P^{PBPS}_1$ of (19), X and Y are independent over a given Z and taking expected value of $P_1$ over the distribution Z, we have

$$\begin{aligned} \begin{aligned} {P^{PBPS}_1}&= \int _0^\infty {{F_X}\left( {\frac{{{\gamma _{th}}{N_0}}}{{Az}}} \right) } \;{F_Y}\left( {\frac{{{I_P}}}{{Az}}} \right) {f_Z}\left( z \right) dz\\&= \int \limits _0^\infty {\left( {1 - {e^{ - \frac{{{\gamma _{th}}{N_0}}}{{{\lambda _x}Az}}}}} \right) \left( {1 - {e^{ - \frac{{{I_P}}}{{{\lambda _y}Az}}}}} \right) \frac{1}{{{\lambda _z}}}{e^{ - \frac{z}{{{\lambda _z}}}}}} dz\\&= \frac{1}{{{\lambda _z}}}\int \limits _0^\infty {{e^{ - \frac{z}{{{\lambda _z}}}}}} dz \\&\quad - \frac{1}{{{\lambda _z}}}\int \limits _0^\infty {{e^{ - \left( {\frac{{{\gamma _{th}}{N_0}}}{{{\lambda _x}Az}} + \frac{z}{{{\lambda _z}}}} \right) }}} dz - \frac{1}{{{\lambda _z}}}\int \limits _0^\infty {{e^{ - \left( {\frac{{{I_P}}}{{{\lambda _y}Az}} + \frac{z}{{{\lambda _z}}}} \right) }}} dz + \frac{1}{{{\lambda _z}}}\int \limits _0^\infty {{e^{ - \left( {\frac{{{\gamma _{th}}{N_0}}}{{{\lambda _x}Az}} + \frac{{{I_P}}}{{{\lambda _y}Az}} + \frac{z}{{{\lambda _z}}}} \right) }}} dz\\&\begin{array}{l} = 1 - \frac{1}{{{\lambda _z}}}\sqrt{\frac{{4{\gamma _{th}}{N_0}{\lambda _z}}}{{{\lambda _x}A}}} {K_1}\left( {\sqrt{\frac{{4{\gamma _{th}}{N_0}}}{{{\lambda _x}{\lambda _z}A}}} } \right) - \frac{1}{{{\lambda _z}}}\sqrt{\frac{{4{I_P}{\lambda _z}}}{{{\lambda _y}A}}} {K_1}\left( {\sqrt{\frac{{4{I_P}}}{{{\lambda _y}{\lambda _z}A}}} } \right) \\ + \frac{1}{{{\lambda _z}}}\sqrt{4\left( {\frac{{{\gamma _{th}}{N_0}}}{{{\lambda _x}A}} + \frac{{{I_P}}}{{{\lambda _y}A}}} \right) {\lambda _z}} {K_1}\left( {\sqrt{4\left( {\frac{{{\gamma _{th}}{N_0}}}{{{\lambda _x}A}} + \frac{{{I_P}}}{{{\lambda _y}A}}} \right) \frac{1}{{{\lambda _z}}}} } \right) \end{array} \end{aligned} \end{aligned}$$

(20)

where $\;\int \nolimits _0^\infty {{e^{ - \;\frac{\beta }{{4x}} - \gamma x}}dx} = \sqrt{\frac{\beta }{\gamma }} {K_1}\left( {\sqrt{\beta \gamma } } \right)$ is used [22, §3.324.1] and $K_1(.)$ is the first order modified Bessel function of the second kind.

In $P^{PBPS}_2$ of (19), X and Z are independent over a given Y and taking expected value of $P_2$ over the distribution Y, we have

$$\begin{aligned} \begin{aligned} {P^{PBPS}_2}&= \int \limits _0^\infty {{F_X}\left( {\frac{{{\gamma _{th}}{N_0}y}}{{{I_P}}}} \right) \left\{ {1 - {F_Z}\left( {\frac{{{I_P}}}{{Ay}}} \right) } \right\} {f_Y}\left( y \right) dy}\\&= \int \limits _0^\infty {\left( {1 - {e^{ - \frac{{{\gamma _{th}}{N_0}y}}{{{\lambda _x}{I_P}}}}}} \right) {e^{ - \frac{{{I_P}}}{{{\lambda _z}Ay}}}}\frac{1}{{{\lambda _y}}}{e^{ - \frac{y}{{{\lambda _y}}}}}dy}\\&= \frac{1}{{{\lambda _y}}}\int \limits _0^\infty {{e^{ - \left( {\frac{{{I_P}}}{{{\lambda _z}Ay}} + \frac{y}{{{\lambda _y}}}} \right) }}dy} - \frac{1}{{{\lambda _y}}}\int \limits _0^\infty {{e^{ - \left( {\frac{{{I_P}}}{{{\lambda _z}Ay}} + \frac{y}{{{\lambda _y}}} + \frac{{{\gamma _{th}}{N_0}y}}{{{\lambda _x}{I_P}}}} \right) }}dy}\\&= \frac{1}{{{\lambda _y}}}\sqrt{\frac{{4{I_P}{\lambda _y}}}{{{\lambda _z}A}}} {K_1}\left( {\sqrt{\frac{{4{I_P}}}{{{\lambda _y}{\lambda _z}A}}} } \right) \\&\quad - \frac{1}{{{\lambda _y}}}\sqrt{\frac{{4{I_P}}}{{{\lambda _z}A\left( {\frac{{{\gamma _{th}}{N_0}}}{{{\lambda _x}{I_P}}} + \frac{1}{{{\lambda _y}}}} \right) }}} {K_1}\left( {\sqrt{\frac{{4{I_P}}}{{{\lambda _z}A}}\left( {\frac{{{\gamma _{th}}{N_0}}}{{{\lambda _x}{I_P}}} + \frac{1}{{{\lambda _y}}}} \right) } } \right) \end{aligned} \end{aligned}$$

(21)

Similar as (20), $\;\int \limits _0^\infty {{e^{ - \;\frac{\beta }{{4x}} - \gamma x}}dx} = \sqrt{\frac{\beta }{\gamma }} {K_1}\left( {\sqrt{\beta \gamma } } \right)$ is used [22, §3.324.1]. Plugging (20) and (21) in (19), we obtain the ${F^{PBPS}_{{\gamma _{({l^{**}},m + 1)}}}}({\gamma _{th}}){\mathrm{{ }}}$ expression in (14). $\square$

1.2 A.2 Appendix II: Proof of proposition 2 in (18)

Proof

Considering the interference coming from PT, the cdf of SINR becomes analytically untraceable. To reduce the mathematical complexity, we neglect the interference from PT. From the (7), we can write

$$\begin{aligned}&{F^{IBPS}_{{\gamma _{({l^{**}},m + 1)}}}}({\gamma _{th}}){\mathrm{{ }}} = {P_r}\left\{ {{\gamma _{({l^{**}},m + 1)}}< {\gamma _{th}}} \right\} \nonumber \\&= {P_r}\left\{ {\frac{{min(AZ,\frac{{{I_P}}}{Y})X}}{{{N_o}}}< {\gamma _{th}}} \right\} \nonumber \\&=\Pr \left\{ {\min \left( {AZ,\frac{{{I_P}}}{Y}} \right) X< {\gamma _{th}}{N_0}} \right\} \nonumber \\&= \underbrace{\Pr \left\{ {X< \frac{{{\gamma _{th}}{N_0}}}{{Az}},\;Y< \frac{{{I_P}}}{{Az}}} \right\} }_{{P^{IBPS}_1}} + \underbrace{\Pr \left\{ {X < \frac{{{\gamma _{th}}{N_0}y}}{{{I_P}}},\;Z \ge \;\frac{{{I_P}}}{{Ay}}} \right\} }_{{P^{IBPS}_2}} \end{aligned}$$

(22)

$$\begin{aligned}&{P^{IBPS}_1} = \int \limits _0^\infty {{F_X}\left( {\frac{{{\gamma _{th}}{N_0}}}{{Az}}} \right) } {F_Y}\left( {\frac{{{I_P}}}{{Az}}} \right) {f_Z}\left( z \right) dz \nonumber \\&= \int \limits _0^\infty {{{\left( {1 - {e^{ - \frac{{{\gamma _{th}}{N_0}}}{{{\lambda _w}Az}}}}} \right) }^L}} \left( {1 - {e^{ - \frac{{{I_P}}}{{{\lambda _y}Az}}}}} \right) \frac{1}{{{\lambda _z}}}{e^{ - \frac{z}{{{\lambda _z}}}}}dz \nonumber \\&= \int \limits _0^\infty {{{\left( {1 - {e^{ - \frac{{{\gamma _{th}}{N_0}}}{{{\lambda _w}Az}}}}} \right) }^L}} \frac{1}{{{\lambda _z}}}{e^{ - \frac{z}{{{\lambda _z}}}}}dz - \int \limits _0^\infty {{{\left( {1 - {e^{ - \frac{{{\gamma _{th}}{N_0}}}{{{\lambda _w}Az}}}}} \right) }^L}} \frac{1}{{{\lambda _z}}}{e^{ - \left( {\frac{z}{{{\lambda _z}}} + \frac{{{I_P}}}{{{\lambda _y}Az}}} \right) }}dz\nonumber \\&= \int \limits _0^\infty {\sum \limits _{l = 0}^L {{{\left( { - 1} \right) }^l}{}^L{C_l}} } {e^{ - l\frac{{{\gamma _{th}}{N_0}}}{{{\lambda _w}Az}}}}\frac{1}{{{\lambda _z}}}{e^{ - \frac{z}{{{\lambda _z}}}}}dz \nonumber \\&\quad - \int \limits _0^\infty {\sum \limits _{l = 0}^L {{{\left( { - 1} \right) }^l}{}^L{C_l}} } {e^{ - l\frac{{{\gamma _{th}}{N_0}}}{{{\lambda _w}Az}}}}\frac{1}{{{\lambda _z}}}{e^{ - \left( {\frac{z}{{{\lambda _z}}} + \frac{{{I_P}}}{{{\lambda _y}Az}}} \right) }}dz\nonumber \\&= \sum \limits _{l = 0}^L {{{\left( { - 1} \right) }^l}{}^L{C_l}} \frac{1}{{{\lambda _z}}}\sqrt{\frac{{4l{\gamma _{th}}{N_0}{\lambda _z}}}{{{\lambda _w}A}}} {K_1}\left( {\sqrt{\frac{{4l{\gamma _{th}}{N_0}}}{{{\lambda _z}{\lambda _w}A}}} } \right) \nonumber \\&- \sum \limits _{l = 0}^L {{{\left( { - 1} \right) }^l}{}^L{C_l}} \frac{1}{{{\lambda _z}}}\sqrt{\left( {\frac{{4l{\gamma _{th}}{N_0}}}{{{\lambda _w}A}} + \frac{{4{I_P}}}{{{\lambda _y}A}}} \right) {\lambda _z}} {K_1}\left( {\sqrt{\left( {\frac{{4l{\gamma _{th}}{N_0}}}{{{\lambda _w}A}} + \frac{{4{I_P}}}{{{\lambda _y}A}}} \right) \frac{1}{{{\lambda _z}}}} } \right) \end{aligned}$$

(23)

$$\begin{aligned}&{P^{IBPS}_2} = {F_X}\left( {\frac{{{\gamma _{th}}{N_0}y}}{{{I_P}}}} \right) \left( {1 - {F_Z}\left( {\frac{{{I_P}}}{{Ay}}} \right) } \right) {f_Y}\left( y \right) dy\nonumber \\&= \int \limits _0^\infty {{{\left( {1 - {e^{ - \frac{{{\gamma _{th}}{N_0}y}}{{{\lambda _w}{I_P}}}}}} \right) }^L}{e^{ - \frac{{{I_P}}}{{{\lambda _z}Ay}}}}} \frac{1}{{{\lambda _y}}}{e^{ - \frac{y}{{{\lambda _y}}}}}dy\nonumber \\&= \int \limits _0^\infty {\sum \limits _{l = 0}^L {{{\left( { - 1} \right) }^l}{}^L{C_l}} {e^{ - l\frac{{{\gamma _{th}}{N_0}y}}{{{\lambda _w}{I_P}}}}}} \frac{1}{{{\lambda _y}}}{e^{ - \left( {\frac{y}{{{\lambda _y}}} + \frac{{{I_P}}}{{{\lambda _z}Ay}}} \right) }}dy\nonumber \\&= \sum \limits _{l = 0}^L {{{\left( { - 1} \right) }^l}{}^L{C_l}} \frac{1}{{{\lambda _y}}}\sqrt{\frac{{4{I_P}}}{{{\lambda _z}A\left( {l\frac{{{\gamma _{th}}{N_0}}}{{{\lambda _w}{I_P}}} + \frac{1}{{{\lambda _y}}}} \right) }}} {K_1}\left( {\sqrt{\frac{{4{I_P}}}{{{\lambda _z}A}}\left( {l\frac{{{\gamma _{th}}{N_0}}}{{{\lambda _w}{I_P}}} + \frac{1}{{{\lambda _y}}}} \right) } } \right) \end{aligned}$$

(24)

$\square$

Plugging (23) and (24) in (22), we obtain the ${F^{IBPS}_{{\gamma _{({l^{**}},m + 1)}}}}({\gamma _{th}}){\mathrm{{ }}}$ expression in (18).