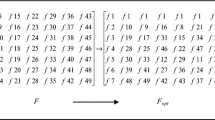
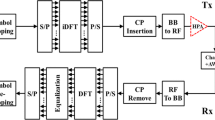
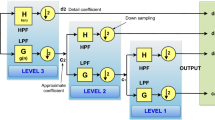
Abstract
A hybrid technique, which is a combination of the Fast Fourier Transform (FFT) and Sliding Window (SW) technique, is proposed for the development of new structure for Discrete Multiwavelet Critical-Sampling Transform based Orthogonal Frequency Division Multiplexing (DMWCST-OFDM) system under multipath fading channels with the Doppler frequency effect. FFT is utilized to increase the orthogonality of subcarriers against the multipath frequency-selective fading channels. SW technique reduces the fluctuation of signal amplitude, thereby reducing the Doppler frequency effect. This hybrid technique offers a good tradeoff between performance and complexity, where this technique needs more computations. However, it performs better than the standard DMWCST-OFDM system. The performance of the proposed system, called SW-DMWCST-OFDM, was compared to that of the standard DMWCST-OFDM, SW-FFT-OFDM, and FFT-OFDM systems under three channels, namely, additive white Gaussian noise, flat fading, and frequency-selective fading. The simulation results show that the proposed model performs better than the three other models in all types of channels.













Similar content being viewed by others
References
Chen, S., & Zhu, C. (2004). ICI and ISI analysis and mitigation for OFDM systems with insufficient cyclic prefix in time-varying channels. IEEE Transactions on Consumer Electronics, 50(1), 78–83.
Jayakody, D. N., Iheme, L. O., & Ince, E. A. (2010). Coded QPSK-OFDM for data transmission over fading channels. In Proceedings of conference on information and automation for sustainability (pp. 276–282).
Banelli, P. (2003). Theoretical analysis and performance of OFDM signals in nonlinear fading channels. IEEE Transaction on Wireless Communications, 2(2), 284–293.
Oltean, M. (2007). Wavelet OFDM performance in flat fading channels. Scientific Bulletin of University Politehnica Timisoara, ETC Series, 52, 167–172.
Li, Y. G., & Stuber, G. L. (2006). Orthogonal frequency division multiplexing for wireless communications. Berlin: Springer.
Roque, D., & Siclet, C. (2013). Performances of weighted cyclic prefix OFDM with low-complexity equalization. IEEE Communications Letters, 17(3), 439–442.
Lindsey, A. R. (1997). Wavelet packet modulation for orthogonally multiplexed communication. IEEE Transactions on Signal Processing, 45(5), 1336–1339.
Negash, B. G., & Nikookar, H. (2001). Wavelet based OFDM for wireless channels. Proceedings of Conference on Vehicular Technology, 1, 688–691.
Zhang, H., Yuan, D., Jiang, M., & Wu, D. (2004). Research of DFT-OFDM and DWT-OFDM on different transmission scenarios. In Proceedings of the 2nd international conference on information technology for application (ICITA 2004).
Abdullah, K., & Hussain, Z. M. (2007). Performance of Fourier-based and wavelet-based OFDM for DVB-T systems. In Proceedings of conference on telecommunication networks and applications (pp. 475–479).
Strela, V., Heller, P. N., Strang, G., Topiwala, P., & Heil, C. (1999). The application of multiwavelet filter banks to image processing. IEEE Transactions on Image Processing, 8(4), 548–563.
Milovanovic, D., Marincic, A., Petrovic, G., & Barbaric, Z. (1999). Comparative study of scalar and multiwavelet filters in transform-based compression of IRLS images. In Proceedings of IEEE international conference on telecommunications in modern satellite, cable and broadcasting services (pp. 169–172).
Martin, M. B., & Bell, A. E. (2001). New image compression techniques using multiwavelets and multiwavelet packets. IEEE Transactions on Image Processing, 10(4), 500–510.
Dawood, S. A., Malek, F., Anuar, M. S., & Hadi, S. Q. (2015). Discrete multiwavelet critical-sampling transform-based OFDM system over Rayleigh fading channels. Mathematical Problems in Engineering,. doi:10.1155/2015/676217.
Bahai, A. S., Saltzberg, B. R., & Ergen, M. (2004). Multi-carrier digital communications: Theory and applications of OFDM (2nd ed.). New York: Springer.
Jansons, J., Ipatovs, A., & Petersons, E. (2009). Estimation of Doppler shift for IEEE 802.11g standard. In Baltic conference advanced topics in telecommunication, University of Rostock (pp. 73–82).
Al-Naffouri, T. Y., Zahidul Islam, K. M., Al-Dhahir, N., & Lu, S. (2010). A model reduction approach for OFDM channel estimation under high mobility conditions. IEEE Transactions on Signal Processing, 58(4), 2181–2193.
Salih, S. M. (2010). Novel sliding window technique of OFDM modem for the physical layer of IEEE 802.11a standard. Journal of Telecommunications, 3(1), 67–71.
Salih, S. M., & Mahmoud, W. A. (2011). Modified OFDM model based on sub-sliding window technique. Computer and Information Science, 4(1), 100–109.
Kumar, S., & Sharma, S. (2013). Performance evaluation physical layer of IEEE 802.11 standards under Fourier transform and wavelet transform over Rician fading channel. In Proceedings of the 2nd international conference on information management in the knowledge economy (IMKE) (pp. 69–74).
Zaier, A., & Bouallègue, R. (2011). Channel estimation study for block-pilot insertion in OFDM systems under slowly time varying conditions. International Journal of Computer Networks and Communications, 3(6), 39–54.
Forouzan, B., Coombs, C. A., & Fegan, S. C. (1998). Introduction to data communications and networking. New York: WCB/McGraw-Hill.
Wu, W., Srinivasan, V. V. G., Hsu, C., Kim, Y., Lee, C., & Rappaport, T. (2003). Wireless communication project (EE381K-11) technical report optimal channel estimation for capacity maximization in OFDM systems. Department of Electrical and Computer Engineering, University of Texas, Austin, TX, USA.
Coleri, S., Ergen, M., Puri, A., & Bahai, A. (2002). Channel estimation techniques based on pilot arrangement in OFDM systems. IEEE Transactions on Broadcasting, 48(3), 223–229.
Mestdagh, D., & Spruyt, P. (1996). A method to reduce the probability of clipping in DMT-based transceivers. IEEE Transactions on Communication, 44(10), 1234–1238.
Wulich, D., & Goldfeld, L. (1999). Reduction of peak factor in orthogonal multicarrier modulation by amplitude limiting and coding. IEEE Transactions on Communication, 47(1), 18–21.
Wang, Y., Chen, W., & Tellambura, C. (2010). PAPR reduction method based on parametric minimum cross entropy for OFDM signals. IEEE Communications Letters, 14, 563–565.
Leftah, H. A., & Boussakta, S. (2014). Novel OFDM based on C-transform for improving multipath transmission. IEEE Transactions on Signal Processing, 62(23), 6158–6170.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Dawood, S.A., Malek, F., Anuar, M.S. et al. Performance Enhancement of Discrete Multiwavelet Critical-Sampling Transform-Based OFDM System Using Hybrid Technique Under Different Channel Models. Wireless Pers Commun 91, 419–438 (2016). https://doi.org/10.1007/s11277-016-3468-5
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11277-016-3468-5