Secrecy and throughput performance of an energy harvesting hybrid cognitive radio network with spectrum sensing

Abstract

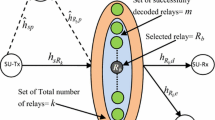
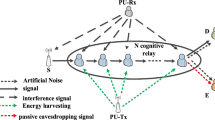
In this paper, we evaluate the secrecy outage performance and throughput of a hybrid cognitive radio network, where a secondary user (SU) accesses the primary spectrum either in underlay or overlay mode based on spectrum sensing decision. In underlay, the transmit power of the SU as well as the relay is limited by the maximum acceptable interference at primary user (PU) receiver as required by an PU outage constraint, a quality of service for PU. The secondary network employs a decode and forward relay which harvests energy from the radio frequency signal of SU following a time switching relaying protocol. We develop analytical expressions for secrecy outage considering the impact of sensing decision and sensing time. Impact of sensing time, imperfect channel state information of interfering link, energy harvesting time, acceptable interference threshold and PU outage constraint on the secrecy outage probability, as well as throughput of SU are investigated. Further, an interplay between throughput performance and secrecy outage of the network is highlighted.

Appendices

Appendix 1. Derivation of CDF of $\gamma _{sr}^u$

$$\begin{aligned} \gamma _{sr}^u &= \frac{{\eta {P_{th}}\,{g_{sr}}}}{{{\sigma ^2}\;{{{\hat{g}}}_{s{p_2}}}}}\nonumber \\ {F_{\gamma _{sr}^u}}(x)& = prob\left( {\frac{{\eta {P_{th}}\,{g_{sr}}}}{{{\sigma ^2}\;{{{\hat{g}}}_{s{p_2}}}}} \le x} \right) \nonumber \\ &= P\left( {\frac{{{g_{sr}}}}{{{{{\hat{g}}}_{s{p_2}}}}} \le \frac{{x{\sigma ^2}}}{{\eta {P_{th}}}}} \right) \quad \nonumber \\& = {F_{\frac{{{g_{sr}}}}{{{{{\hat{g}}}_{s{p_2}}}}}}}\left( {\frac{{x{\sigma ^2}}}{{\eta {P_{th}}}}} \right) \nonumber \\ {F_{\gamma _{sr}^u}}(x) &= 1 - \frac{{{\lambda _{sr}}\eta {P_{th}}}}{{{\lambda _{sr}}\eta {P_{th}} + x{\sigma ^2}{\lambda _{s{p_2}}}}} \end{aligned}$$

(50)

Similarly, we can derive the CDF of ${F_{\gamma _{se}^u}}(x)$ as

$$\begin{aligned} {F_{\gamma _{se}^u}}(x) = 1 - \frac{{{\lambda _{se}}\eta {P_{th}}}}{{{\lambda _{se}}\eta {P_{th}} + x{\sigma ^2}{\lambda _{s{p_2}}}}} \end{aligned}$$

(51)

Appendix 2. Derivation of CDF of $\gamma _{rd}^u$

$$\begin{aligned} \gamma _{_{rd}}^u &= \min \left( {\underbrace{\frac{{{a_1}{} {g_{sr}}\,{g_{rd}}}}{{{{{\hat{g}}}_{s{p_2}}}}}}_{{X_1}},\underbrace{\frac{{\eta {P_{th}}\,{g_{rd}}}}{{{\sigma ^2}{{{\hat{g}}}_{rp}}}}}_{{X_2}}} \right) \nonumber \\ {F_{\gamma _{rd}^u}}(x) &= \left( {\min ({X_1},{X_2}) \le x} \right) \nonumber \\& = 1 - prob(\min ({X_1},{X_2}) \ge x)\nonumber \\ &= 1 - \left\{ {P({X_1} \ge {x_1}).P({X_2} \ge {x_2})} \right\} \nonumber \\& = 1 - \left[ {\left\{ {1 - {F_{{X_1}}}({x_1})} \right\} \left\{ {1 - {F_{{X_2}}}({x_2})} \right\} } \right] \end{aligned}$$

(52)

Now the CDFs of ${F_{{X_1}}}({x_1})$ and ${F_{{X_2}}}({x_2})$ can be derived as,

$$\begin{aligned} {F_{{X_1}}}({x_1}) &= prob\left( {\frac{{{a_1}{} {g_{sr}}\,{g_{rd}}}}{{{{{\hat{g}}}_{s{p_2}}}}} \le {x_1}} \right) \nonumber \\ &= P\left( {{g_{sr}} \le \frac{{{x_1}}}{{{a_1}}}\underbrace{\frac{{{{{\hat{g}}}_{s{p_2}}}}}{{{g_{rd}}}}}_Y} \right) \quad \nonumber \\&= P\left( {{g_{sr}} \le \frac{{{x_1}Y}}{{{a_1}{} \,}}} \right) \nonumber \\ &= \int \limits _0^\infty {{F_{{g_{sr}}}}\left( {\frac{{{x_1}y}}{{{a_1}{} \,}}} \right) .{f_{\frac{{{{{\hat{g}}}_{s{p_2}}}}}{{{g_{rd}}}}}}(y)\,dy} \nonumber \\ &= \int \limits _0^\infty {(1 - {e^{\frac{{{x_1}y}}{{{a_1}{} {\lambda _{sr}}\,}}}})} .\frac{{{\lambda _{rd}}{\lambda _{s{p_2}}}}}{{{{({\lambda _{s{p_2}}} + y{\lambda _{rd}})}^2}}}dy\nonumber \\ {F_{{X_1}}}({x_1}) &= 1 - \frac{{{\lambda _{s{p_2}}}{x_1}}}{{{a_1}{\lambda _{rd}}{\lambda _{sr}}}}{e^{\frac{{{\lambda _{s{p_2}}}{x_1}}}{{{a_1}{\lambda _{rd}}{\lambda _{sr}}}}}}Ei\left( { - \frac{{{\lambda _{s{p_2}}}{x_1}}}{{{a_1}{\lambda _{rd}}{\lambda _{sr}}}}} \right) \nonumber \\&- \frac{{{\lambda _{rd}}}}{{{\lambda _{s{p_2}}}}} \end{aligned}$$

(53)

where Ei(.) is the exponential integral [25], 3.353.3]

$$\begin{aligned} {F_{{X_2}}}({x_2}) &= prob\left( {\frac{{\eta {P_{th}}\,{g_{rd}}}}{{{\sigma ^2}{{{\hat{g}}}_{rp}}}} \le {x_2}} \right) \nonumber \\&= P\left( {\frac{{{g_{rd}}}}{{{{{\hat{g}}}_{rp}}}} \le \frac{{{x_2}{\sigma ^2}}}{{\eta {P_{th}}}}} \right) \quad \nonumber \\ &= {F_{\frac{{{g_{rd}}}}{{{{{\hat{g}}}_{rp}}}}}}\left( {\frac{{{x_2}{\sigma ^2}}}{{\eta {P_{th}}}}} \right) \nonumber \\ {F_{{X_2}}}({x_2})& = 1 - \frac{{{\lambda _{rd}}\eta {P_{th}}}}{{{\lambda _{rd}}\eta {P_{th}} + {x_2}{\sigma ^2}{\lambda _{rp}}}} \end{aligned}$$

(54)

Substituting the CDFs ${F_{{X_1}}}({x_1})$ and ${F_{{X_2}}}({x_2})$ from Eqs. (53) and 54 respectively into Eq. (52)

$$\begin{aligned} {F_{\gamma _{rd}^u}}(x) &= 1 - \frac{{{\lambda _{rd}}\eta {P_{th}}}}{{{\lambda _{rd}}\eta {P_{th}} + x{\sigma ^2}{\lambda _{rp}}}}\frac{{{\lambda _{s{p_2}}}x}}{{{a_1}{\lambda _{rd}}{\lambda _{sr}}}}{e^{\frac{{{\lambda _{s{p_2}}}x}}{{{a_1}{\lambda _{rd}}{\lambda _{sr}}}}}}Ei\nonumber \\&\quad \times\,\left( { - \frac{{{\lambda _{s{p_2}}}x}}{{{a_1}{\lambda _{rd}}{\lambda _{sr}}}}} \right) \nonumber \\&\quad-\, \frac{{{\lambda _{rd}}\eta {P_{th}}}}{{{\lambda _{rd}}\eta {P_{th}} + x{\sigma ^2}{\lambda _{rp}}}}\frac{{{\lambda _{rd}}}}{{{\lambda _{s{p_2}}}}} \end{aligned}$$

(55)

Appendix 3. Derivation of CDF of $\gamma _{m}^u$

$$\begin{aligned} \gamma _m^u &= \min \;(\gamma _{sr}^u,\gamma _{rd}^u)\nonumber \\ {F_{\gamma _m^u}}(x) &= P\left( {\min \;(\gamma _{sr}^u,\gamma _{rd}^u) \le x} \right) \nonumber \\& = 1 - \left[ {P(\gamma _{sr}^u \ge x).P(\gamma _{rd}^u \ge x)} \right] \nonumber \\ &= 1 - \left[ {\left\{ {1 - {F_{\gamma _{sr}^u}}(x)} \right\} \left\{ {1 - {F_{\gamma _{rd}^u}}(x)} \right\} } \right] \end{aligned}$$

(56)

By substituting the CDFs of ${F_{\gamma _{sr}^u}}(x)$ and ${F_{\gamma _{rd}^u}}(x)$ from Eqs. (50) and (55) respectively in the above Eq. (56) we can get

$$\begin{aligned}& {F_{\gamma _m^u}}(x)\; = 1 - \left[ \left\{ \frac{{{\lambda _{sr}}\eta {P_{th}}}}{{{\lambda _{sr}}\eta {P_{th}} + x{\sigma ^2}{\lambda _{s{p_2}}}}} \right\} \nonumber \right. \\&\quad \left. \left\{ \begin{array}{l} \left( {\frac{{{\lambda _{rd}}\eta {P_{th}}}}{{{\lambda _{rd}}\eta {P_{th}} + x{\sigma ^2}{\lambda _{rp}}}}\frac{{{\lambda _{s{p_2}}}x}}{{{a_1}{\lambda _{rd}}{\lambda _{sr}}}}{e^{\frac{{{\lambda _{s{p_2}}}{} x}}{{{a_1}{\lambda _{rd}}{\lambda _{sr}}}}}}Ei\left( { - \frac{{{\lambda _{s{p_2}}}x}}{{{a_1}{\lambda _{rd}}{\lambda _{sr}}}}} \right) } \right) \\ + \left( {\frac{{{\lambda _{rd}}\eta {P_{th}}}}{{{\lambda _{rd}}\eta {P_{th}} + x{\sigma ^2}{\lambda _{rp}}}}\frac{{{\lambda _{rd}}}}{{{\lambda _{s{p_2}}}}}} \right) \end{array} \right\} \right] \end{aligned}$$

(57)

Appendix 4. Derivation of CDF of $\gamma _{rd}^o$

$$\begin{aligned} \gamma _{rd}^o &= \frac{{2\delta \alpha {P_{m\,}}}}{{(1 - \alpha )\,{\sigma ^2}}}{g_{sr}}\,{g_{rd}}\nonumber \\ {F_{\gamma _{rd}^o}}(x) &= P\left( {{g_{sr}}\, \le \frac{x}{{{a_2}{g_{rd}}}}} \right) \nonumber \\&= \int \limits _0^\infty {{F_{{g_{sr}}}}\left( {\frac{x}{{{a_2}{g_{rd}}}}} \right) .{f_{{g_{rd}}}}(.)\,d{g_{rd}}} \nonumber \\ &= 1 - \frac{1}{{{\lambda _{rd}}}}\int \limits _0^\infty {\exp \left( { - \frac{\beta }{{4{g_{rd}}}} - \gamma {g_{rd}}} \right) } \,d{g_{rd}}\nonumber \\ {F_{\gamma _{rd}^o}}(x) &= 1 - \sqrt{\frac{{4x}}{{{a_2}{\lambda _{rd}}{\lambda _{sr}}}}} {k_1}\left( {\sqrt{\frac{{4x}}{{{a_2}{\lambda _{rd}}{\lambda _{sr}}}}} } \right) \end{aligned}$$

(58)

where ${a_2} = \frac{{2\delta \alpha {P_{m\,}}}}{{(1 - \alpha )\,{\sigma ^2}}}$ , $\beta = \frac{{4x}}{{{a_2}{\lambda _{sr}}}}$, $\gamma = \frac{1}{{{\lambda _{rd}}}}$ and $K_1()$ is the first-order modified Bessel function of the second kind.By using the formula $\int \limits _0^\infty {\exp \left( { - \frac{\beta }{{4x}} - \gamma x} \right) } \,dx = \sqrt{\frac{\beta }{\gamma }} {K_1}(\sqrt{\beta \gamma } )$,[25],3.324.1] we have calculated the above Eq. (58).

Appendix 5. Derivation of CDF of $\gamma _{m}^o$

$$\begin{aligned} \gamma _m^o &= \min \;(\gamma _{sr}^o,\gamma _{rd}^o)\nonumber \\ {F_{\gamma _m^o}}(x) &= P\left( {\min \;(\gamma _{sr}^o,\gamma _{rd}^o) \le x} \right) \nonumber \\&= 1 - \left[ {\left\{ {1 - {F_{\gamma _{sr}^o}}(x)} \right\} \left\{ {1 - {F_{\gamma _{rd}^o}}(x)} \right\} } \right] \end{aligned}$$

(59)

By substituting the CDFs of ${F_{\gamma _{sr}^o}}(x)$ and ${F_{\gamma _{rd}^o}}(x)$ from Eqs. (36) and 58 respectively in the above Eq. (59) we can get

$$\begin{aligned}&{F_{\gamma _m^o}}(x) = 1 \nonumber \\&\quad - \left\{ {\exp \left( {\frac{{x{\sigma ^2}}}{{{P_m}{\lambda _{sr}}}}} \right) \sqrt{\frac{{4x}}{{{a_2}{\lambda _{rd}}{\lambda _{sr}}}}} \,{k_1}\left( {\sqrt{\frac{{4x}}{{{a_2}{\lambda _{rd}}{\lambda _{sr}}}}} } \right) } \right\} \end{aligned}$$

(60)