Gappy, glutty, glappy

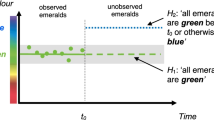
Abstract

According to the Determinable Based Account (DBA) of metaphysical indeterminacy (MI), there is MI when there is an indeterminate state of affairs, roughly a state of affairs in which a constituent object x has a determinable property but fails to have a unique determinate of that determinable. There are different ways in which x might have a determinable but no unique determinate: x has no determinate—gappy MI, or x has more than one determinate—glutty MI. Talk of determinables and determinates is usually constructed as relative to levels of determination. In this paper I first (1) provide a formal construction for determinables and determinates that pays crucial attention to intermediate levels of determination, and then (2) explore the consequences for the DBA of introducing such intermediate levels. In particular, I argue that intermediate levels of determination highlight crucial differences between gappy and glutty cases of MI, and allow one to introduce a third way of indeterminacy, glappy MI.

1 Introduction

According to the Determinable Based Account (DBA) of metaphysical indeterminacy (MI), there is MI when there is an indeterminate state of affairs, roughly a state of affairs in which a constituent object x has a determinable property but fails to have a unique determinate of that determinable. I will abuse terminology and say that x is MI. There are different ways in which x might have a determinable but no unique determinate: x has no determinate—gappy MI, or x has more than one determinate—glutty MI.¹ Talk of determinables and determinates is usually constructed as relative to levels of determination—more on this later on. In this paper I first (i) provide a formal construction for determinables and determinates that pays crucial attention to intermediate levels of determination,² and then (ii) explore the consequences for the DBA of introducing such intermediate levels. Let me be clear from the start. It is not the aim of the paper to argue either in favor or against the DBA.³ Rather, it is to investigate and discuss some of its (alleged) consequences and commitments, especially in the light of the existence of intermediate levels of determination.

2 Determinables, determinates, and determination

As a first pass,⁴

[D]eterminables and determinates are in the first instance type-level properties that stand in a distinctive specification relation: the “determinable determinate” relation (for short, “determination”). For example, color is a determinable having red, blue, and other specific shades of color as determinates; shape is a determinable having rectangular, oval, and other specific (including many irregular) shapes as determinates; mass is a determinable having specific mass values as determinates (Wilson 2017b: Introduction).

The determination relation is usually characterized by a list of principles.⁵ Wilson (2017b) lists different such principles. Two of them will be central to our discussion. Wilson calls them Requisite Determination, and Unique Determination. I adopt and adapt her formulation:⁶

• Requisite determination: If x has a determinable $d_1$ at time t, then, for every level L of determination of $d_1$: x has some L-level determinate $d_2$ of $d_1$ at t.⁷

• Unique determination: If x has a determinable $d_1$ at time t, then x has a unique determinate $d_2$ at any given level of determination at t.

Implicit in Wilson’s formulation is the thought that determinables admit of different levels of determination, so that the characterization of a property as determinable and determinate is relative to levels. Simons (2013) offers arguments in favor of the crucial metaphysical role of intermediate levels of determination.⁸

I will not consider any such argument here. I am interested in exploring the consequences of introducing intermediate levels, especially when it comes to the DBA.

In what follows I am going to set forth a formal framework that serves two related, yet distinct purposes: (i) First I show that the “the space of determinable and determinate properties” of a given family—more on this later on—can be generated by the determination relation alone (Sect. 2.1); (ii) Second, I provide a formal rendition of different principles of determination (Sect. 2.2). Let me spend a few words on (i) and (ii), starting from (i). The assumptions on the determination relation I use in Sect. 2.1 do not involve any of the controversial principles of determination I discuss in Sect. 2.2. Therefore, the construction in Sect. 2.1 should be of interest to anyone that is interested in determinables, determinates, and their applications. It provides a “proof of concept”, so to speak, that determination alone can be used to generate the determinable space of a given family of properties. I take this to be a significant result on its own. As for (ii), it is not my intention to provide a fully-fledged formal theory of determination. I will use formal renditions rather opportunistically: they mainly serve the purpose to highlight some consequences and commitments of the DBA—though this might not be their only purpose. In light of this, I will be opportunistic in my choices as well. I will unashamedly use set-theory and higher-order quantification.

2.1 Getting the determinable structure out of determination

In this subsection I show how to construct the determinable space of a given family of properties out of determination alone. Thus, I will take determination D to be my only primitive. I assume that D is a strict order that holds between properties at different levels of determination $L^i$—as it will be clear shortly, the superscripts count the steps from the top-level $L^{\top }$. That is to say that $D(d^ i_n, d^j_m) \rightarrow L^i \ne L^j$ holds—in effect, this last assumption is redundant in that it can be derived once the formal construction is laid out. I am going to assume that there is a top level $L^{\top }$ and a bottom level $L^{\bot }$. Furthermore, I am going to assume that there is only one $d^{\top } \in L^{\top }$, whereas there are multiple $d^{\bot }_i \in L^{\bot }$.⁹ The unique $d^{\top } \in L^{\top }$ represents what in the literature is called a maximally unspecific determinable, i.e. a determinable property that is not itself a determinate of any other determinable. By contrast, the various $d^{\bot }_i \in L^{\bot }$ represent maximally specific determinates, i.e. determinate properties that are themselves not the determinable of any other determinate. We say that $d^i_n$ is a determinable of $d^j_m$ iff $D(d^j_m, d^i_n)$. Conversely, we say that $d^i_n$ is a determinate of $d^j_m$ iff $d^j_m$ is a determinable of $d^i_n$, that is iff $D(d^i_n, d^j_m)$.¹⁰ Finally, I will assume that every determinate has a unique determinable at each level of determination.

The construction I am about to provide works for discrete finite structures—that is, structures that contain a finite number of levels of determination. By contrast, each level might contain either a finite or an infinite amount of properties.¹¹ Given that such structures are enough to make the main points in the paper I will restrict my attention to them. Let us focus on a single “family of properties P”. This expression is supposed to refer to all of the determinables and determinates of the “the same kind”. To illustrate, one paradigmatic family of properties, the color properties, will be referred to as:

$$\begin{aligned} P=\{Color, Red, Blue,..., Scarlet, Maroon,...., Sapphire, Perwinkle, ....\} \end{aligned}$$

The structure of said properties is what I called—using an admittedly vague expression—the determinable space of a given family of properties, in this case, color properties. First define the notion of “Immediate Determination”, that is, define “$d^i_n$ is an immediate determinate of $d^j_m$”—$ID(d^i_n, d^j_m)$:

$$\begin{aligned} ID(d^i_n, d^j_m) =_{df} D(d^i_n, d^j_m) \wedge \lnot \exists d (D(d^i_n, d) \wedge D(d, d^j_m)) \end{aligned}$$

(1)

That is to say that $d^i_n$ is an immediate determinate of $d^j_m$ iff it $d^i_n$ is a determinate of $d^j_m$ and there is nothing D-related between them, namely a d such that d is a determinable of $d^i_n$ but a determinate of $d^j_m$. The target notion we want to define is “being n-steps away from the top level”, $S^{n\top } (d^n_i)$. First, define $S^{1\top }$ “being 1 step away from the top level”—e.g. Red, or (...), or Blue in the example above, the maximally unspecific determinable being Color—see Fig. 2:

$$\begin{aligned} S^{1\top } (d^1_i) =_{df} ID(d^1_i, d^{\top }) \end{aligned}$$

(2)

Definition (2) captures the fact that there are no properties between $d^{\top }$ and any property that is 1 step away from the top level. Equivalently, a property that is 1 step away from the top level is an immediate determinate of the top level. For simplicity I shall call such properties $S^1$ properties—the same applies to every n. We can then define the target notion by induction:

$$\begin{aligned} S^{n\top } (d^n_i) =_{df} \exists d^{n-1}_j (ID(d^n_i, d^{n-1}_j) \wedge S^{n-1\top }(d^{n-1}_j)) \end{aligned}$$

(3)

Informally, a $S^n$ property is an immediate determinate of an $S^{n-1}$ property, a property that is n steps away from the top level $L^{\top }$.¹² Look now at the construction in (1)–(3). Upon inspection one sees that when ID holds, it holds between a property at $n-1$ steps from the top level and one at n steps away from the top level, in this order. In general, given the transitivity of (I)D, if D holds between $d^i$ and $d^j$ in this order, $d^i$ is further away from the top level than $d^j$.

Now we can define two relations, “being more steps away from the top level” $>_S$ (or being further away from, or being more distant from) , and “being as many steps away from the top level” $=_S$ (or being at the same distance from):

$$\begin{aligned} d^i_n>_S d^j_m= & {} _{df} S^{i\top }(d^i_n) \wedge S^{j\top }(d^j_m) \wedge i > j \end{aligned}$$

(4)

$$\begin{aligned} d^i_n= & {} _S d^j_m =_{df} S^{i\top }(d^i_n) \wedge S^{j\top }(d^j_m) \wedge i = j \end{aligned}$$

(5)

Informally, according to (4) a property $d^i_j$ is further away from the top level than a property $d^j_m$ iff it takes more steps to get to $d^i_n$ than to get to $d^j_m$. Similarly for (5). Clearly $=_S$ is an equivalence relation. I will identify levels of determination $L^i$ with its equivalence classes. Intuitively, they are just sets that contains properties at the same distance from the top level. Thus, $L^i$ contains all and only the $S^i$ properties. Note that every property $d^i$ is a certain number—namely i—of steps away from the top level, so that every $d^i$ belongs to only one level $L^i$. This ensures, importantly, that levels $L^i$-s are pairwise disjoint. It should be clear now that I used superscripts on properties and levels so as to have: $d^i \in L^i$. This choice is justified by the construction above. Hence, I will simply omit the levels to which properties belong to simplify notation in formal renditions.

Next, we define a strict order among the levels, <. This strict order is supposed to represent what in the literature is taken to be the relation of “being more specific” holding between different levels:

$$\begin{aligned} L^i < L^j =_{df} \forall d^i_n \forall d^j_m (d^i_n >_S d^j_m) \end{aligned}$$

(6)

In plain English, (6) says that level $L^i$ is more specific than level $L^j$ iff the properties in $L^i$ are further away from the top level. < is provably transitive and asymmetric. Therefore, it is irreflexive. That is, it is a strict order, as desired.¹³ We can then use < among levels to define its counterpart for single determinable/determinate properties:

$$\begin{aligned} d^i_n< d^j_m =_{df} \exists L^i \exists L^j (L^i < L^j) \end{aligned}$$

(7)

That is just to say, unsurprisingly, that a property is more specific than another iff it belongs to a more specific level. The orders induced by D and < are consistent, i.e. (8) and (9) below hold:

$$\begin{aligned}&D(d^i_n, d^j_m) \rightarrow L^i < L^j \end{aligned}$$

(8)

$$\begin{aligned}&L ^i < L^j \rightarrow \lnot (\exists d^j_m \exists d^i_n (D(d^j_m, d^i_n))) \end{aligned}$$

(9)

Taken together, (8) and (9) ensure that the determination relation always hold between two properties such that the former is more specific than the latter. To see that (8) holds, assume the antecedent, that is, assume $D(d^i_n, d^j_m)$. As we saw already, by the construction in (1)-(3), if $D(d^i, d^j)$ holds, then $d^i$ is further away from the top level than $d^j$. Given (6), this entails that $L^i < L^j$ as desired. As for (9), assume $L^i < L^j$. Suppose, for reductio, that there is a $d^j_m$ and a $d^i_n$ such that $D(d^j_m, d^i_n)$. Then, by (8), $L^j < L^i$. But clearly this violates the asymmetry of <.¹⁴

This construction is also able to capture the following facts F$_1$–F$_3$:

F$_1$:

The unique maximally unspecific determinable $d^{\top } \in L^{\top }$ is only a determinable. It is not a determinate. This is because there is no $d^i_n$ such that $D(d^{\top }, d^i_n)$. I am going to assume that for every $d \ne d ^{\top }$ in the same family of properties P, $D(d, d^{\top })$ holds. That is, every property in a given family P is a determinate of $d^{\top }$. This further simplifies the construction for we can always forget the conjunct $D(d, d^{\top })$.¹⁵

F$_2$:

The various maximally specific determinates $d^{\bot }_i \in L^{\bot }$ are only determinates. They are not determinables. This is because there are no $d^i_n$-s such that, for any $d^{\bot } \in L^{\bot }$, $D(d^i_n, d^{\bot })$.

F$_3$:

For any $L^i$ such that $L^{\top } \ne L^i \ne L^{\bot }$, and any $d^i_m \in L^i$, $d^i_m$ is both a determinable and a determinate, for there are $d^j_n$ and $d^k_o$ such that $D(d^j_n, d^i_m)$, and $D(d^i_m, d^k_o)$.¹⁶

Figures 1 and 2 show a general picture of the structure we just discussed, and one paradigmatic example of it, Color.¹⁷

Fig. 1

Determinables and determinates: general structure

Fig. 2

Determinables and determinates: color

2.2 Principles of determination

Once the structure of determinables and determinates is constructed out of the determination relation we can ask about the very logic of such relation. This will give us principles that govern the instantiation of determinables and determinates, not just their relations. As I pointed out already, the principles of determination I want to focus on are Requisite Determination and Unique Determination, for they play the crucial role in the DBA, as I am about to argue.¹⁸ I will give different formal renditions—the reason will be clear in due course. In effect, several variants of those principles can be given. However, I will target only two here: a completely unrestricted one, and one that is restricted to particular $L^i$-s—that is, $L^{\top }$ and $L^{\bot }$. Before I move on, let me say a few words about why I mentioned that there are more variants than the two I target. The reason is that, while in the variants I will consider the antecedent expresses that an object x has the maximally unspecific determinable $d^{\top } \in L^{\top }$, one could substitute $L^{\top }$ with an arbitrary level $L^i \ne L^{\bot }$ in the antecedent instead. This would provide more variants of the same principle indeed. There is a reason why I stick to $d^{\top } \in L^{\top }$, and I will get to it shortly. As of now, let me start with Requisite Determination—once again restricting one’s attention to one relevant family of properties P, and letting d(x) abbreviate “x has determinable-determinate property d”:¹⁹

$$\begin{aligned}&\mathbf{RD } \qquad \qquad d^{\top } (x) \rightarrow (\forall L^i (L^i \ne L^{\top })\exists d^i_n (d^i_n (x))) \end{aligned}$$

(10)

$$\begin{aligned}&\mathbf{RD} ^{\top }_{\bot } \qquad \qquad \qquad \qquad \qquad \quad d^{\top }(x) \rightarrow (\exists d^{\bot }_n (d^{\bot }_n (x))) \end{aligned}$$

(11)

Clearly (10) entails (11) but the converse, in absence of any other principles, does not hold. Now, there is a principle that underwrites the entailment from (11) to (10), thus rendering the two materially equivalent. The principle is the one Wilson (2017b) calls Determinable Inheritance:

• Determinable Inheritance: If x has a determinate $d_1$ of a determinable $d_2$, then x has $d_2$.

In the present context, Determinable Inheritance translates into the following:

$$\begin{aligned} \mathbf{DI}&d^i_n (x) \rightarrow (\forall L^j (L^i < L^j) \exists d^j_m (D(d^i_n, d^j_m) \wedge (d^j_m (x)) \end{aligned}$$

(12)

Let me briefly sketch why, given DI, (11) entails (10).²⁰ Assume (11). Then, any x that has $d^{\top }$ has a maximally specific determinate of it. By DI for any less specific level, x has a determinable of that maximally specific determinate, up to $L^{\top }$. This ensures that the consequent of (10) is satisfied. I will indeed assume DI, so that there is no point in distinguishing between the unrestricted RD and the restricted RD$^{\top }_{\bot }$. Yet, as I will argue, things are different from different principles: in some cases, restricted and unrestricted versions are not materially equivalent. Before I turn to Unique Determination, let me just note that, in the presence of DI, if x has any property whatsoever, it will have its maximally unspecific determinable $d^{\top } \in L^{\top }$. Therefore, any version of Requisite Determination that figures in the antecedent that x has a determinable $d^i \in L^i$ with $L^{\top } \ne L^i \ne L^{\bot }$ will entail the formulations I gave above. This is why I restricted my attention to those formulations.

Let me then move to Unique Determination. I will be focusing on the restricted version of the principle. Its literal formalization is as follows:

$$\begin{aligned} \mathbf{UD ^{\top }_{\bot }}&d^{\top }(x) \rightarrow (\exists d^{\bot }_n (d^{\bot }_n (x)) \wedge \forall d^{\bot }_m (d^{\bot }_m(x) \rightarrow d^{\bot }_n = d^{\bot }_m) \end{aligned}$$

(13)

This literally translates the uniqueness requirement. It is exactly this uniqueness requirement that has been historically considered a feature of determination. There is however a weaker requirement that one may want to impose. This is the requirement that something has at most one determinate rather than a unique determinate. Let me call this At Most One Determinate:

• At Most One Determinate: If x has determinable $d_1$, x has at most one determinate $d_2$ of $d_1$ for each level of determination.

Here is a rendition of the principle, in both unrestricted and restricted versions:

$$\begin{aligned}&\mathbf{AMOD } \qquad d^{\top } (x) \rightarrow \forall L^i ( \forall d^i_n \forall d^i_m (d^i_n (x) \wedge d^i_m (x) \rightarrow d^i_n = d^i_m)) \end{aligned}$$

(14)

$$\begin{aligned}&\mathbf{AMOD ^{\top }_{\bot }} \qquad \quad d^{\top } (x) \rightarrow \forall d^{\bot }_n \forall d^{\bot }_m (d^{\bot }_n (x) \wedge d^{\bot }_m (x) \rightarrow d^{\bot }_n = d^{\bot }_m) \end{aligned}$$

(15)

Principle (14) says that if x has the maximally unspecific determinable, it has at most one determinate for every level of determination. By contrast (15) says that if x has the maximally unspecific determinable, it has at most one of its maximally specific determinates. Now, (14) entails (15), but without any further principle, (15) does not entail (14). In the presence of (15) alone, nothing prevents some x to have a unique maximally specific determinate, but many determinables of that determinate at some level $L^i$ such that $L^{\top } \ne L^i \ne L^{\bot }$.²¹

As of now, I just want to point out something about the restricted principles: (13) entails (15) but the converse, in absence of any other principles, does not hold. The reason behind this overall discussion will be clear in the following sections.

3 The determinable based account of metaphysical indeterminacy

The DBA was first proposed in Wilson (2013), and defended in Wilson (2017). It has been applied to several cases:²² indeterminate boundaries (Wilson 2013, 2017), the open future (Wilson 2017; Mariani and Torrengo 2020), fundamentality (Mariani and Torrengo 2020), and quantum indeterminacy (Bokulich 2014; Wolff 2015; Lewis 2016, Calosi and Wilson (2018, Forthcoming), Wilson (2020), Calosi and Mariani (Forthcoming a, b)). Wilson herself suggests two different characterization of the DBA. She is explicit in that the second formulation is simplified (Wilson 2017: p. 118):

Determinable-based MI$_1$: What it is for a state of affairs to be MI in a given respect R at a time t is for the state of affairs to constitutively involve an object (more generally, entity) O such that (i) O has a determinable property P at t, and (ii) for some level L of determination of P, O does not have a unique level-L determinate of P at t (Wilson 2013: p. 366).

Determinable-based MI$_2$: What it is for a state of affairs to be MI in a given respect R at a time t is for the state of affairs to constitutively involve an object (more generally, entity) O such that (i) O has a determinable property P at t, and (ii) O does not have a unique determinate of P at t (Wilson 2017: p. 107).

I take it that Wilson’s claim about the second characterization being simplified boils down to the fact that there is no mention of different levels of determination. I think that Wilson has put her finger onto something interesting here. This dovetails nicely with both (i) my focus on intermediate levels of determination, and (ii) my discussion of unrestricted and restricted principles of determination in the previous section. In effect, noticing the different levels of determination, and simplifying a little, one may distinguish (at least) three interesting versions of Determinable MI:

• Unrestricted MI: x is a case of Unrestricted MI iff x has a determinable $d^i_n \in L^i$ and, for all levels $L^j$ such that $L^j < L^i$, x lacks a unique determinate $d^j_m \in L^j$.

• Restricted to the Bottom MI: x is a case of Restricted to the Bottom MI iff x has a determinable $d^i_n \in L^i$ and x lacks a unique determinate $d^{\bot }_m \in L^{\bot }$.

• Restricted MI: x is a case of of Restricted MI iff x has a determinable $d^i_n \in L^i$, and there is one level $L^j$, with $L^j < L^i$, such that x lacks a unique determinate $d^j_m \in L^j$.²³

The different versions are given in order of decreasing logical strength: every “downstream” entailment holds, whereas no “upstream” entailment does. In light of this, it should already be clear that cases of Unrestricted, Restricted, and Restricted to the Bottom MI violate different versions of the principles in Sect. 2.2. For instance, in absence of any other principle, a case of Restricted MI might not violate AMOD$^{\top }_{\bot }$. There could be some x that has a unique maximally determinate $d^{\bot }$ that does not have, for some level $L^i$, a unique $d^i_n \in L^i$. This would still violate AMOD.

One should note that I am only claiming that this is a logical possibility, in a broad sense. That is, this case is compatible with the logic of determination, as constructed in Sect. 2.2. The substantive question is now whether one can find examples that are metaphysically significant. This goes beyond the scope of the paper. But it is within the scope of the paper to explore the broadly logical consequences of the possibility of such cases. Note that they will have to be cases of glutty MI: cases in which some x has the determinable $d^{\top }$ and for some level $L^i$ such that $L^{\top } \ne L^i \ne L^{\bot }$, it has more than one determinate $d^i_n, d^i_m \in L^i$.²⁴ This is because there could not be similar cases of gappy MI, i.e. cases in which some x has the maximally unspecific determinable $d^{\top }$, a unique determinate $d^{\bot } \in L^{\bot }$ but, for some $L^i$, no determinate $d^i_n \in L^i$. In the presence of DI, there simply cannot be any such case. Let me defer a more detailed discussion to the next section.

4 Gappy, glutty and principles of determination

As we saw in the introduction, there are different ways an object x can have a determinable and yet fail to have a unique determinate of that determinable, as per gappy and glutty MI. In this section I will present a discussion of somewhat neglected details of gappy and glutty MI. I will also highlight crucial differences between the cases that rest on some results of the previous sections.

Let’s start from gappy. Recall that cases of gappy MI are cases in which an object x has a determinable but no determinate of that determinable. In light of the discussion of Sect. 3 one could distinguish Unrestricted, Restricted to the Bottom, and Restricted gappy cases of MI: just replace “x lacks a unique determinate” with “x has no determinate”. All of the cases violate both RD and RD$^{\top }_{\bot }$, given that they are materially equivalent. One may worry that Restricted gappy cases can violate RD without violating RD$^{\top }_{\bot }$. That would be a case in which some x has a determinate at $L^{\top }$ but no determinate $d^i_n$ for some $L^i$. But, once again, DI rules out such a possibility.

Do gappy cases violate Unique Determination? Here is an informal argument. In (restricted) gappy cases, some object x has no determinate $d^{\bot }_i \in L^ {\bot }$. A fortiori it does not have a unique determinate $d^{\bot }_i \in L^{\bot }$. And this simply constitutes a violation of (restricted) Unique Determination. In effect, the formal renditions in Sect. 2 provide a formal counterpart to such an argument: UD$^{\top }_{\bot }$ entails RD$^{\top }_{\bot }$. And, as we saw already, gappy cases do violate RD$^{\top }_{\bot }$.

But, recall, I introduced yet another principle in Sect. 2.2, namely At Most One Determination. The question is then whether gappy cases of MI violate that. The restricted version of the principle is enough to make my point so I will focus on it. The crucial thing to note is that AMOD$^{\top }_{\bot }$ will not be violated by cases of gappy MI: if an object x has no determinate $d^{\bot }_i \in L^{\bot }$, AMOD$^{\top }_{\bot }$ is trivially satisfied. Once again, the formal rendition shows this immediately: as we saw, UD$^{\top }_{\bot }$ entails RD$^{\top }_{\bot }$. By contrast, AMOD$^{\top }_{\bot }$ does not entail RD$^{\top }_{\bot }$. Naturally enough UD$^{\top }_{\bot }$ and AMOD$^{\top }_{\bot }$ are materially equivalent in the presence of RD$^{\top }_{\bot }$. But cases of gappy MI violate RD$^{\top }_{\bot }$.

The conclusion to draw is the following. Those who endorse gappy MI are committed to violations of UD$^{\top }_{\bot }$ but not to violations of AMOD$^{\top }_{\bot }$. One can probably argue that the “at most one requirement” is close enough in spirit to the “uniqueness requirement”. If so, I simply offer At Most One Determination as the one principle of determination that is close enough in spirit to Unique Determination and is not violated by cases of gappy MI.²⁵

I anticipate the following objection: AMOD$^{\top }_{\bot }$ was never part and parcel of the logic of determination. Thus, it is unclear why we should really care about it, unless we are given independent philosophical motivations in its favor. This is a fair point, but there is a reply. As I pointed out, in the presence of RD$^{\top }_{\bot }$, UD$^{\top }_{\bot }$ and AMOD$^{\top }_{\bot }$ are materially equivalent. So there is little interest in distinguishing them. But, where there is no RD$^{\top }_{\bot }$ there is no equivalence. It is exactly violations of RD$^{\top }_{\bot }$, as we have in gappy cases, that provide motivations for distinguishing between UD$^{\top }_{\bot }$ and AMOD$^{\top }_{\bot }$. Look at it this way. Once this distinction is recognized, there are cases, namely cases in which RD$^{\top }_{\bot }$ is violated, in which the distinction becomes relevant. In those cases, the “at most one” requirement can do most of the job the “uniqueness requirement” was supposed to do. In the end, it does capture the idea that if something has a determinate, then it has only one.

Let’s move to glutty MI. Recall that glutty cases of MI are cases in which some x has a determinable and more than one determinate of that determinable. Let me be crystal clear. Advocates of glutty MI²⁶ are adamant that in glutty cases of MI, the relevant indeterminate objects instantiate different determinates only mediately so to speak, that is, either relative to a given perspective p, or to a certain degree $\delta$, with $0 < \delta \le 1$. I will omit this specification from now on for the sake of readability, but the reader is urged to keep this in mind.

Clearly, the same move I made for gappy cases is available for glutty cases as well. That is, one can distinguish Unrestricted, Restricted to the Bottom, and Restricted glutty cases of MI: just replace “x lacks a unique determinate” with “x has more than one determinate” in the characterization of MI in Sect. 3. The glutty case is more interesting, insofar as Unique Determination and the two versions of At Most one Determinate are not materially equivalent. It is a substantive question which cases of glutty MI violate which version of which principle.²⁷

Let’s start with Unrestricted cases of glutty MI. These are cases in which x, for any level $L^i \ne L^{\top }$, has more than one determinate $d^i_n \in L^i$. These cases violate all principles, UD$^{\bot }_{\top }$, AMOD, and AMOD$^{\top }_{\bot }$. Next, Restricted to the Bottom cases of glutty MI. These are cases in which x has more than one maximally specific determinate $d^{\bot } \in L^{\bot }$. These cases violate all principles as well. Finally, cases of Restricted glutty MI. These are cases in which x has more than one determinate $d^i_n \in L^i$, for some $L^i \ne L^{\top }$. Note however that nothing prevents that $L^i \ne L^{\bot }$. This opens the following possibility: x has a unique maximally specific determinate but more than one $d^i_n \in L^i$ with $L^i \ne L^{\bot }$. These cases–that we did briefly encounter already in the previous section—will violate the unrestricted version AMOD. But they need violate neither AMOD$^{\bot }_{\bot }$ nor UD$^{\bot }_{\top }$.

In the light of the above one could give the following “gloss”: one might think of cases of determinable MI as cases in which Unique Determination is violated. This can happen in two ways: when no determinate is instantiated (gappy) and when more than one determinate is instantiated—albeit in a mediated way (glutty). Alternatively, one can see cases of determinable MI as cases in which either Requisite Determination is violated (gappy), or At Most One Determinate is violated (glutty).

It is instructive to sum up the results of this section—if only by considering the simple cases of Restricted to the Bottom MI, and consequently only the restricted versions of different principles of determination. Table 1 sums up such results—$\checkmark$ indicates which principles of determination can be retained:

Table 1 Principles of determination and metaphysical indeterminacy

The discussion so far suggests several differences between gappy and glutty cases—beside the obvious one to the point that they present counter-examples to different principles of determination, as indicated in Table 1. The first difference is that gappy cases violate both restricted and unrestricted versions of the determination principles they are a counterexample to, for the versions of those principles are materially equivalent. This is not the case for glutty cases. In effect, as we saw there could be a case in wich x has a unique determinate at $L^{\bot }$ but more than one determinate at $L^i$ with $L^{\bot } < L^i$. In Sect. 3 I pointed out there cannot be any gappy counterpart, that is, no case in which x has a unique determinate at $L^{\bot }$ but no determinate for some $L^i$ with $L^i$ with $L^{\bot } < L^i$. Suppose this is the case. Then, by DI, x would have, for any level $L^i < L^{\bot }$, at least a determinate at $L^i$, which clearly contradicts our hypothesis. This highlights yet another difference between gappy and glutty cases. If x is such that, at level $L^i$ it has no determinate $d^i_n \in L^i$, then, for every level $L^j$ than it is more specific than $L^i$, x does not have any determinate $d^j_m \in L^j$ either. This is not the case for glutty. In other words: gappyness trickles all the way down to the bottom level, whereas gluttiness can be confined. This is an interersting feature in and on itself, and it is about to play a role in the next section.

5 Glappy, the third way of metaphysical indeterminacy

One of the most interesting and significant consequences of focusing on intermediate levels of determination is that it opens the possibility of a third way for something to be MI, in between gappy and glutty so to speak—or, to put it differently, a combination of gappy and glutty. Consider the following passage from Calosi and Mariani (Forthcoming, b):

[I]t is clear that there are two ways in which an object can fail to instantiate a unique determinate of a determinable:

• Gappy Metaphysical Indeterminacy. No Determinate of the determinable is instantiated (...)

• Glutty Metaphysical Indeterminacy. More than one determinate of the determinable is instantiated (Calosi and Mariani, Forthcoming: 3-4, italics added).

There is a sense in which the claim is indeed true. If we restrict our attention to one level of determination $L^i$ there are indeed only two ways in which an object can fail to have a unique determinate. One should not infer however that gappy and glutty cases are mutually exclusive in general, in that if some object x constitutes a case of gappy MI, x cannot constitute a case of glutty MI. This, I want to suggest, is false. At least in its full generality. One can have cases of an object x such that x qualifies both as gappy and glutty if only one pays crucial attention to different levels of determination. I shall call these mixed cases glappy. In what follows, I first introduce such cases (Sect. 5.1), and then go on to provide (some) potential applications (Sect. 5.2).

5.1 Introducing glappy MI

Here is a first characterization of glappy MI:

• Glappy MI: x is a case of glappy MI when x has a determinable $d^i_n \in L^i$, and for some levels $L^j, L^k$, such that $L^k< L^j < L^i$, x has more than one determinate $d^j_l, d^j_m \in L^j$—with $d^j_l \ne d^j_m$, and x has no determinate $d^k_i \in L^k$.

Here is another way of phrasing things. Let me introduce:

• Glutty MI at L$^j$: x is a case of glutty MI at level $L^j$ when x has a determinable $d^i \in L^i$ and more than one determinate $d^j_l, d^j_m \in L^j$—with $d^j_l \ne d^j_m$ and $L^j < L^i$.

Note that if x is glutty at only one level $L^i$, it will be a case of Restricted Glutty MI. It will be a case of Restricted to the Bottom Glutty MI iff $L^i = L^{\bot }$. And it will be a case of Unrestricted Glutty MI iff there are only two levels of specification, namely $L^{\top }$ and $L^{\bot }$—note that in this case x will qualify as a case of Restricted to the Bottom Glutty MI as well. Similarly:

• Gappy MI at L$^j$: x is a case of gappy MI at level $L^j$ when x has a determinable $d^i \in L^i$ and no determinate $d^j_l \in L^j$—with $L^j < L ^i$.

What I said for glutty goes for gappy as well. I will not repeat it. Now we can provide an equivalent, perhaps more perspicuous characterization of Glappy MI:

• Glappy MI: x is a case of glappy MI when x is glutty at $L^j$ and gappy at $L^i$, with $L^i < L^j$.

Perhaps the most interesting thing to note is that in both characterizations of Glappy MI, it is explicit that the level at which some x is glutty is less specific than the level at which x is gappy. In effect, given the formal background I set forth, this clause is redundant. The argument is straightforward. Nothing can be both glutty and gappy at the same level of determination. And the case in which some x is glutty at $L^j$ and gappy at $L^i$ with $L^j < L^i$ is ruled out by DI. In the presence of DI, gappiness trickles all the way down to the bottom, as I pointed out already in Sect. 4—from the first level $L^i$ such that x is gappy at $L^i$. We can now phrase the argument differently. Suppose this not the case. That is, suppose there is an x such that x is gappy at $L^i$ and yet x has at least one determinate $d^j_n \in L^j$ with $L^j < L^i$. Then, by DI, x has $d^i_m$ such that $D(d^i_m, d^j_n)$. But $d^i_m \in L^i$, which contradicts the hypothesis that x is gappy at $L^i$.

I submit this might be interesting not only in and on itself. Recently, there have been worries about whether gappy MI does any work in understanding quantum indeterminacy. For instance, Mariani and Torrengo write:

[W]e believe there are good reasons, coming from quantum mechanics, for preferring the “glutty” approach (Mariani and Torrengo 2020: footnote 13).

Now, quantum mechanics seems to provide the best examples of gappy MI. But, if quantum indeterminacy is best understood in glutty terms, this might fuel some skepticism about gappy cases.²⁸ This is where glappy might lend gappy a helping hand. One would need to explore whether some cases of quantum indeterminacy are actually best understood in glappy terms. I consider such potential applications of glappy MI next.

5.2 Potential applications of glappy MI

To conclude I want to suggest a potential application of glappy MI.²⁹ I should be upfront and confess that at this stage, this is just a suggestion rather than a full-fledged argument in favor of glappy MI. It has been recently argued that the DBA provides a satisfactory account of quantum indeterminacy, that is, indeterminacy that arises as a result of the failure of value definiteness for quantum observables.³⁰ One alleged case of quantum indeterminacy comes from quantum entanglement.³¹ To illustrate briefly, consider an entangled system $s_{12}$ composed by $s_1$ and $s_2$ with corresponding Hilbert space $\mathcal {H}_{12}=\mathcal {H}_1\otimes \mathcal {H}_2$. $S_{12}$ might be in an eigenstate $|\omega \rangle$ of $\mathcal {O}_{12}=\mathcal {O}_1 - \mathcal {O}_2$ that is neither an eigenstate of $\mathcal {O}_1$ nor an eigenstate of $\mathcal {O}_2$—with $\mathcal {O}_1$ and $\mathcal {O}_2$ defined on $\mathcal {H}_1$ and on $\mathcal {H}_2$ respectively. Under controversial, yet not implausible assumptions, both $s_1$ and $s_2$ will therefore lack a definite value for the corresponding observables. Let me consider a simplified case in which two quantum systems $s_1$ and $s_2$ are entangled in the position degree of freedom. That is, let me stipulate that $s_{12}$ is in the following state—forgetting normalization constants: $|\psi \rangle _{12} = |r_1\rangle _1 |r_2\rangle _2 + |r_2\rangle _1 |r_1\rangle _2$—where $|r_i\rangle$ is the state “being in region $r_i$”, and $r_1$ and $r_2$ are disjoint. To each region of space $r_i$ we can associate a projection operator $\hat{P}_{r_i}$ that represents the observable $\mathcal {O}_i$ of “being in region r”. In other words states $|r_i\rangle$ are eigenstates of projectors $\hat{P}_{r_i}$.³² Suppose we can divide a given region of space r into a subregions $r_i$ such that the $r_i$-s are pairwise disjoint and their sum is r.³³ One can then argue that each $\hat{P}_{r_i}$ is a determinate of $\hat{P}_r$. For example one may notice that each $\hat{P}_{r_i}$ specify $\hat{P}_r$, by providing a way of being in r. The unique maximally unspecific determinable would then be $\hat{P}_{\Delta }$, where $\Delta$ is just the maximal spatial region, i.e., space itself. Go back now to our system $s_{12}$ in $|\psi \rangle _{12} = |r_1\rangle _1 |r_2\rangle _2 + |r_2\rangle _1 |r_1\rangle _2$. By construction, $\hat{P}_{r_1}$ and $\hat{P}_{r_2}$ are determinates of $\hat{P}_{\Delta }$. Calosi and Wilson (2018) provides an account of glutty MI, according to which $s_1$ is both at $r_1$ and $r_2$ albeit in either a relativized fashion—as e.g., per Everett’s original relative state interpretation, or to a degree $\delta <1$. But now suppose we could divide both $r_1$ and $r_2$ in two disjoint subregions, $r_{1l}$ for “left”, and $r_{1r}$ for “right”. Once again, by construction, $\hat{P}_{r_{1l}}$ and $\hat{P}_{r_{1r}}$ are determinates of $\hat{P}_{r_{1}}$. The determinable structure is depicted in Fig. 3.

Fig. 3

Determinables and Determinates: “Being in a Region r”

Finally, suppose one can argue that $s_1$ is neither in region $r_{1l}$, nor in region $r_{1r}$—for example by arguing that $r_{1l}$ (and $r_{1r}$) is too small a region to contain a quantum system such as $s_1$.³⁴ If so, $s_1$ qualifies as glutty at $L^i$ and gappy at $L^{\bot }$. That is, $s_1$ qualifies as an example of glappy MI. I will admit this example feels convoluted. But it offers a prima facie reason to explore glappy MI further. Yet another example comes from the double-slit experiment. Calosi and Wilson (Forthcoming) argues that the interference pattern in the double-slit experiment can be explained in glutty terms by letting a single electron having both $\hat{P}_{s_1}$ and $\hat{P}_{s_2}$, where $s_1$ and $s_2$ are the regions corresponding to the two-slits. Suppose one goes further and—simplifying only slightly—one endorses the view that the electron in question does not have a more precise location, represented by e.g., $\hat{P}_{s_{1l}}$, $\hat{P}_{s_{1r}}$, $\hat{P}_{s_{2l}}$, and $\hat{P}_{s_{2r}}$, for a suitable partition of $s_1$ and $s_2$—say because quantum systems do not have definite trajectories. Then, an electron in a single run of the double-slit experiment would qualify as a case of glappy MI as well. I admit that a thorough exploration of glappy quantum indeterminacy deserves an independent scrutiny. I hope I said enough to show that such a scrutiny is worth having.