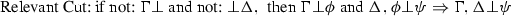Abstract
I discuss Greg Restall’s attempt to generate an account of logical consequence from the incoherence of certain packages of assertions and denials. I take up his justification of the cut rule and argue that, in order to avoid counterexamples to cut, he needs, at least, to introduce a notion of logical form. I then suggest a few problems that will arise for his account if a notion of logical form is assumed. I close by sketching what I take to be the most natural minimal way of distinguishing content and form and suggest further problems arising for this route.
Similar content being viewed by others
Notes
There are, of course, other putative problems, such as the interpretation of the modal ‘necessarily’ (Etchemendy 1990). Note that I do not claim that any of these problems is insoluble—far from it. I am here concerned only to motivate why we might look elsewhere for an account of logical consequence.
See MacFarlane (2004) for a survey of some of the options in this regard.
See Harman (1986) for vigorous argument that logic has little to do with inference.
More on this below. This way of introducing logical operators continues a project begun in Dosen (1989).
See also our discussion of the sort of explanatory account that is necessary to motivate these types of inconsistency.
Ian Rumfitt, in a piece brought to my attention recently, briefly makes a similar objection to Restall’s account (Rumfitt 2008). He there argues that ‘There is a god’ is not entailed by ‘There could never be sufficient evidence to accept or deny ‘There is a god”. Again, I am obviously sympathetic. Thanks to Catharine Diehl for discussion of both objections.
See Baker and Woods (2015) for an approach to this and initial worries.
And, in particular, because we can assert we are not denying something while not asserting it either. Thanks to an anonymous referee for discussion.
Here and below, I will use ‘structural constraints’ and ‘structural features’ as opposed to ‘structural rules’ since for Restall these are features of the consequence relation, not rules of inference in any reasonable sense.
Restall’s actual formulation is in terms of multiple conclusions, but showing trouble for the single-conclusion case is sufficient for my purposes.
Note that Restall is explicit that his background picture does not require commitment to either asserting or denying every statement. But it is difficult to see how to justify Cut without this commitment, as we will see.
The ‘explicitly’ is important here. Withholding has to be an action, otherwise we would conflate taking no stand on something, being lethargic, and having never heard of something.
Again, the ‘explicitly’ is important as there is nothing wrong with asserting \(\gamma \) without having heard of \(\beta \). We need ‘denial’ to be something we do, but if it is, then asserting \(\gamma \) and denying \(\beta \) is incoherent.
I switch here from ‘evidence’ to ‘reason’ for purposes of naturalness. Nothing hangs on it.
Such attempts at universal logics have become more popular recently. See, for example, Field (2008). One expects that Restall would also want to be able to capture such logics within his framework. And some of these logics, such as Field’s, accept Cut.
Thanks to a helpful referee for suggesting this direct version of the problem.
And mutatis mutandis for all the above examples.
For more on this point, see below.
This can be spelled out in a roughly Tarskian fashion even in the context of assertion and denial. Note that capturing the particularly pragmatic aspect of my above examples is harder—see Baker and Woods (2015) for both points in more detail.
Nothing I have said here shows that there is not some restricted version of Cut which can be justified in the way Restall suggests. Consider:
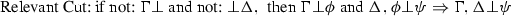
That is, if asserting all of \(\Gamma \) is not antecedently incoherent, and likewise with denying \(\Delta \), then Cut goes through. This is a cut rule reminiscent of Tennant’s favored one (Tennant 1987); this restricted cut rule generates a form of relevance logic. But Restall’s ambition is greater; he is trying to describe and justify logics far stronger than Tennant’s.
Another option is to start off the process of recapturing consequence for a suitably restricted language where contents like the ones above do not appear. The problem is that a general account of consequence would require us to either introduce notions we either introduce notions of evidence, reasonableness, warrant, and the like, which can form part of our basic content, without reintroducing our counterexamples—which is rather implausible; claim that cut holds only for this fragmentary language—an unpalatable result; or somehow build such notions out of a combination of more basic materials and logical consequence in a fashion which does not give rise to our counterexamples—a daunting task.
Thanks to a referee for stressing this worry.
Note, though, that it is still useful to develop a form/content distinction like the one that I sketch below since we might have reason to use Restall’s definition instrumentally in contexts where we do not lexicalize ‘is asserted’ and ‘is denied’.
Note that these worries do not spread to the referee’s example of \(\lnot D\phi \bot \phi \) which is still obviously incoherent—as is its cognate \(\phi \bot A\phi \).
That is most plausible on the assumption that such operators can be iterated. One might deny this. See Ripley (2014) for a discussion of embedding denial and a useful discussion of paradoxes arising from a denial operator.
Worries about the nature and general legitimacy of terms like ‘is asserted’ and ‘is denied’ are part of why I have included four counterexamples to Restall’s justification of Cut. We need operators occurring in our language, F and G, which do not necessarily take a stand on every content \(\phi \)—i.e. where it is coherent for some content \(\phi \) to assert \(\lnot F\phi \) while denying \(G\phi \), but not coherent to assert \(\lnot F\phi \) while denying \(\phi \) nor to assert \(\phi \) while denying \(G \phi \). That this holds for any of the four can be questioned. But it would be too costly to revise our intuitive grasp of the terms in all four examples (and the many more that could be found.)
An interesting alternative account of logical form is articulated in Sagi (2014). On Sagi’s view, logical form is a relative notion tied to a more basic type of semantic constraint. There can be pragmatic reasons for choosing what semantic constraints will count as the form of a sentence. The discussion here can be seen as giving a pragmatic reason—the loss of Cut and the collapse of pragmatic and semantic inconsistency—to avoid a too expansive notion of logical form once we accept something like Restall’s account as our foundation. Thanks to Nissim Francez for discussion.
This is an expository simplification. A fully rigorous treatment would distinguish, say, between propositional constants and propositional variables. But, as such technicalities would distract here and the point is clear enough, I simply take quantification over propositional constants for granted.
Conjunction is about the most reasonable logical concept to take for granted. It is singularly well-behaved: for example, the rules for introduction and elimination of conjunctions uniquely specify the meaning on any way of defining the connection between proof rules and meanings. It is the only non-trivial constant having this property. It is so close to fundamental to understanding assertion that John Stuart Mill once quipped that to call a conjunction a compound proposition was tantamount to calling a street a compound house.
Note that this is to read the structural comma as conjunctive on both sides. One of Restall’s nice points is noticing that in the presence of a basic notion of denial, we do not need to read the structural comma on the right disjunctively. This avoids many problems, not least of which is that alternation does not seem to be a basic feature of assertions and denials.
The problem is not that we cannot generate this idealized notion out of assertion, denial, definitions, and quantification over statements. We can. Rather, the problem is that the notion generated in this way diverges from our intuitive grasp of the constants since it is intuitively not incoherent to believe inconsistencies.
Note that I am not claiming that no similar problem arises for the Tarskian account conjoined with other theses about assertion and belief. Just that the Tarskian account by itself is insufficient as a source of this kind of problem. Of course, the Tarskian account, like any formal representation of an intuitive notion, faces the distinct problem of explaining how the formal account represents the intuitive one. See Sher (1991) for a useful formal and philosophical overview of the Tarskian approach and Hanson (2002) for some worries about Sher’s approach.
This problem is related, of course, to the sort of worry that Quine raises against Carnap in Quine (1936). Quine’s point is that you need logic to get logic from definitions. Our point is that you may need logic to understand how we can quantify over statements in such a way as to recognize patterns of any old form.
Note, in defense of this approach, that attempting to give a rule-based justification of quantification or generality is quite difficult; see Garson (2013) for details in the case of model-theoretic inferentialism. Similar worries apply to Restall’s sort of approach.
The logical status of ‘is asserted’ is less clear. If such expressions are logical, then Restall’s account faces more troubles than my simple form/content distinction can finesse. See above discussion.
References
Baker, D., & Woods, J. (2015). How expressivists can and should explain inconsistency. Ethics, 125(2), 391–424.
Dosen, K. (1989). Logical constants as punctuation marks. Notre Dame Journal of Formal Logic, 30(3), 362–381.
Etchemendy, J. (1990). The concept of logical consequence. Cambridge: Harvard University Press.
Field, H. (2008). Saving truth from paradox. Oxford: Oxford University Press.
Garson, J. W. (2013). What logic means: From proof theory to model-theoretic semantics. Cambridge: Cambridge University Press.
Gentzen, G. (1934). Untersuchungen über das logische Schliessen. Mathematische Zeitschrift, 39, 176–210.
Hanson, W. H. (2002). The formal-structural view of logical consequence: A reply to Gila Sher. The Philosophical Review, 111(2), 243–258.
Harman, G. (1986). Change in view. Cambridge: MIT Press.
Hjortland, O. T. (2010). The structure of logical consequence: Proof-theoretic conceptions. Ph.D. Thesis, University of St. Andrews
Lycan, W. G. (1989). Logical constants and the glory of truth-conditional semantics. Notre Dame Journal of Formal Logic, 30(3), 390–400.
MacFarlane, J. (2004). In what sense (if any) is logic normative for thought. Presented at American Philosophical Association Central Division meeting.
Priest, G. (2006). Doubt truth to be a liar. Oxford: Oxford University Press.
Quine, W. V. O. (1936). Truth by convention. In O. H. Lee (Ed.), Philosophical essays for A. N. Whitehead (90–124). London: Longmans, Green & Co.
Restall, G. (2005). Multiple conclusions. In P. Hájek, L. Valdes Villanueva, & D. Westerståhl (Eds.), Logic, methodology and philosophy of science: Proceedings of the twelfth international congress (189–205). London: Kings College Publications.
Ripley, D. (2014). Embedding denial. In O. T. Hjortland & C. Caret (Eds.), Foundations of logical consequence. Oxford: Oxford University Press.
Rumfitt, I. (2008). Knowledge by deduction. Grazer Philosophische Studien, 77(1), 61–84.
Sagi, G. (2014). Formality in logic: From logical terms to semantic constraints. Logique et Analyse, 227, 259–276.
Sher, G. (1991). The bounds of logic: A generalized viewpoint. Cambridge: MIT Press.
Tennant, N. (1987). Anti-realism and logic: Truth as eternal. Oxford: Clarendon Press.
Acknowledgments
Thanks to Catharine Diehl, Nissim Francez, Nick Stang, and two anonymous (and helpful!) referees for useful discussion. Thanks also to John Burgess for remarks on a distant ancestor of this paper and Jimmy Martin for a conversation about it that I reckon he’s forgotten.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Woods, J. Assertion, denial, content, and (logical) form. Synthese 193, 1667–1680 (2016). https://doi.org/10.1007/s11229-015-0797-y
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11229-015-0797-y