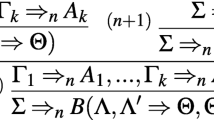Abstract
This paper studies the relationship between Argumentation Logic (AL), a recently defined logic based on the study of argumentation in AI, and classical Propositional Logic (PL). In particular, it shows that AL and PL are logically equivalent in that they have the same entailment relation from any given classically consistent theory. This equivalence follows from a correspondence between the non-acceptability of (arguments for) sentences in AL and Natural Deduction (ND) proofs of the complement of these sentences. The proof of this equivalence uses a restricted form of ND proofs, where hypotheses in the application of the Reductio of Absurdum inference rule are required to be “relevant” to the absurdity derived in the rule. The paper also discusses how the argumentative re-interpretation of PL could help control the application of ex-falso quodlibet in the presence of inconsistencies.
Article PDF
Similar content being viewed by others
References
Amgoud, L., Postulates for logic-based argumentation systems, International Journal of Approximate Reasoning 55(9): 2028–2048, 2014.
Besnard, P., and A. Hunter, Elements of Argumentation, MIT Press, Cambridge, 2008.
Da Costa, N. C. A., Sistemas Formais Inconsistentes, Universidade Federal do Parana, Parana, 1963. Thesis.
D’Agostino, M., and S. Modgil, A rational account of classical logic argumentation for real-world agents in G. A. Kaminka, M. Fox, P. Bouquet, E. Hüllermeier, V. Dignum, F. Dignum and F. van Harmelen (eds.), ECAI 2016: 22nd European Conference on Artificial Intelligence, vol. 285 of Frontiers in Artificial Intelligence and Applications, IOS Press, 2016, pp. 141–149.
De Castro, M. A., and I. M. L. D’Ottaviano, Natural deduction for paraconsistent logic, Logica Trianguli 4: 3–24, 2000.
Dimopoulos, Y., B. Nebel, and F. Toni, On the computational complexity of assumption-based argumentation for default reasoning, Artificial Intelligence 141 (1/2): 57–78, 2002.
D’Ottaviano, I. M. L., On the development of paraconsistent logic and Da Costa’s work, The Journal of Non-Classical Logic 7: 89–152, 1990.
Dung, P. M., On the acceptability of arguments and its fundamental role in nonmonotonic reasoning, logic programming and n-person games, Artificial Intelligence 77(2): 321–358, 1995.
Dung, P. M., A. C. Kakas, and P. Mancarella, Negation as failure revisited, in Technical Report, University of Pisa, 1992.
Dunne, P. E., The computational complexity of ideal semantics, Artificial Intelligence 173(18): 1559–1591, 2009.
Gentzen, G., Untersuchungen über das Logische Schliessen, Mathematische Zeitschrift 39(1): 176–210, 1935.
Jaśkowski, S., On the rules of suppositions in formal logic, Studia Logica 1: 232–258, 1934.
Kakas, A., and L. Michael, Cognitive systems: argument and cognition, IEEE Intelligent Informatics Bulletin 17(1): 4–20, 2016.
Kakas, A. C., P. Mancarella, and P. M. Dung, The acceptability semantics for logic programs, in ICLP, 1994, pp. 504–519.
Kakas, A. C., and F. Toni, Computing argumentation in logic programming, Journal of Logic and Computation 9: 515–562, 1999.
Kakas, A., and P. Mancarella, On the semantics of abstract argumentation, Journal of Logic and Computation 23: 991–1015, 2013.
Kakas, A., L. Michael, and F. Toni, Argumentation: reconciling human and automated reasoning, in Second Workshop on Bridging the Gap Between Human and Automated Reasoning, IJCAI-16, 2016.
Kakas, A. C., and P. Mancarella, On the semantics of abstract argumentation, Journal of Logic and Computation 23(5): 991–1015, 2013.
Kakas, A. C., F. Toni, and P. Mancarella, Argumentation logic, in S. Parsons, N. Oren, C. Reed and F. Cerutti (eds.), Computational Models of Argument: Proceedings of COMMA 2014, vol. 266 of Frontiers in Artificial Intelligence and Applications, IOS Press, 2014, pp. 345–356.
Prawitz, D., Natural Deduction: A Proof Theoretical Study, Almqvist and Wiksell, Stockholm, 1965.
Priest, G., Minimally inconsistent LP, Studia Logica 50: 321–331, 1991.
Priest, G., R. Routley, and J. Norman, Paraconsistent Logic: Essays on the Inconsistent, Philosophia Verlag, 1989.
Rahwan, I., and G. R. Simari, Argumentation in Artificial Intelligence, Springer, New York, 2009.
Tennant, N., A proof-theoretic approach to entailment, Philosophical Logic 9(2): 185–209, 1980.
Tennant, N., Perfect validity, entailment and paraconsistency, Studia Logica 43: 179–198, 1984.
Tennant, N., Natural deduction and sequent calculus for intuitionistic relevant logic, Symbolic Logic 52(3): 665–680, 1987.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
Open Access This article is distributed under the terms of the Creative Commons Attribution 4.0 International License (http://creativecommons.org/licenses/by/4.0/), which permits unrestricted use, distribution, and reproduction in any medium, provided you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made.
About this article
Cite this article
Kakas, A.C., Mancarella, P. & Toni, F. On Argumentation Logic and Propositional Logic. Stud Logica 106, 237–279 (2018). https://doi.org/10.1007/s11225-017-9736-x
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11225-017-9736-x