Abstract
A Triple Helix (TH) network of bi- and trilateral relations among universities, industries, and governments can be considered as an ecosystem in which uncertainty can be reduced when functions become synergetic. The functions are based on correlations among distributions of relations, and therefore latent. The correlations span a vector space in which two vectors (P and Q) can be used to represent forward “sending” and reflexive “receiving,” respectively. These two vectors can also be understood in terms of the generation versus reduction of uncertainty in the communication field that results from interactions among the three bi-lateral channels of communication. We specify a system of Lotka–Volterra equations between the vectors that can be solved. Redundancy generation can then be simulated and the results can be decomposed in terms of the TH components. Furthermore, we show that the strength and frequency of the relations are independent parameters in the model. Redundancy generation in TH arrangements can be decomposed using Fourier analysis of the time-series of empirical studies. As an example, the case of co-authorship relations in Japan is re-analyzed. The model allows us to interpret the sinusoidal functions of the Fourier analysis as representing redundancies.










Similar content being viewed by others
Notes
In the case of weak coupling among the three dimensions, one can use perturbation theory for an approximate solution. By writing the solution in terms of a formal power or perturbation series, one can formulate for relatively small parameters \( W^{2} \) (≪1), as follows:
$$ R =\, \propto_{0} + \propto_{1} W^{2} + \propto_{2} (W^{2} )^{2} + \cdots $$(4a)Since raising the power of W 2 further leads to increasingly smaller values, one can approximate the perturbation solution by using the first two terms:
$$ R =\, \propto_{0} + \propto_{1} W^{2} $$or, disregarding the constant term, as:
$$ R \sim W^{2} $$(4b)Q.e.d.
In a next step, one would be able to differentiate among different coupling coefficients in the three (bilateral) communication channels.
One can generate the network graph from the attribution matrix by multiplication of the latter with its transposed.
The second law of thermodynamics holds equally for probabilistic (Shannon) entropy, since S = k B H and k B is a constant (the Boltzmann constant). Because of the constant, the development of S over time is a function of the development of H, and vice versa.
“Frequently the messages have meaning; that is they refer to or are correlated according to some system with certain physical or conceptual entities. These semantic aspects of communication are irrelevant to the engineering problem.” (Shannon 1948, p. 379).
References
Abramson, N. (1963). Information Theory and Coding. New York: McGraw-Hill.
Bateson, G. (1972). Steps to an ecology of mind. New York: Ballantine.
Biggiero, L. (1998). Italian industrial districts: A Triple Helix pattern of problem solving. Industry and Higher Education, 12(4), 227–234.
Biggiero, L. (2001). Self-organizing processes in building entrepreneurial networks: A theoretical and empirical investigation. Human Systems Management, 20(3), 209–222.
Burt, R. S. (2000). The network structure of social capital. Research in Organizational Behavior, 22, 345–423.
Dubois, D. M. (1998). Computing anticipatory systems with incursion and hyperincursion. In D. M. Dubois (Ed.), Computing anticipatory systems, CASYS-first international conference (Vol. 437, pp. 3–29). Woodbury, NY: American Institute of Physics.
Etzkowitz, H., & Leydesdorff, L. (2000). The dynamics of innovation: from national systems and ‘Mode 2’ to a Triple Helix of university–industry–government relations. Research Policy, 29(2), 109–123.
Kamke, E. (1971). Differentialgleichungen: Lösungsmethoden und Lösungen (Vol. 1, p. 1). New York: American Mathematical Society.
Kemp, R., Schot, J., & Hoogma, R. (1998). Regime shifts to sustainability through processes of niche formation. The approach of strategic niche management. Technology Analysis & Strategic Management, 10(2), 175–195.
Krippendorff, K. (1980). Q: an interpretation of the information theoretical Q-measures. In R. Trappl, G. J. Klir, & F. Pichler (Eds.), Progress in cybernetics and systems research (Vol. VIII, pp. 63–67). New York: Hemisphere.
Krippendorff, K. (2009a). W. Ross Ashby’s information theory: A bit of history, some solutions to problems, and what we face today. International Journal of General Systems, 38(2), 189–212.
Krippendorff, K. (2009b). Information of interactions in complex systems. International Journal of General Systems, 38(6), 669–680.
Kwon, K. S., Park, H. W., So, M., & Leydesdorff, L. (2012). Has globalization strengthened South Korea’s national research system? National and international dynamics of the Triple Helix of scientific co-authorship relationships in South Korea. Scientometrics, 90(1), 163–175. doi:10.1007/s11192-011-0512-9.
Leydesdorff, L. (1994). Epilogue. In L. Leydesdorff & P. van den Besselaar (Eds.), Evolutionary economics and chaos theory: New directions for technology studies (pp. 180–192). London: Pinter.
Leydesdorff, L. (2010). The communication of meaning and the structuration of expectations: Giddens’ “structuration theory” and Luhmann’s “self-organization”. Journal of the American Society for Information Science and Technology, 61(10), 2138–2150.
Leydesdorff, L. (2011). “Meaning” as a sociological concept: a review of the modeling, mapping, and simulation of the communication of knowledge and meaning. Social Science Information, 50(3–4), 1–23.
Leydesdorff, L. (2012). Radical constructivism and radical constructedness: Luhmann’s sociology of semantics, organizations, and self-organization. Constructivist Foundations, 8(1), 85–92.
Leydesdorff, L. (2014). Science visualization and discursive knowledge. In B. Cronin & C. Sugimoto (Eds.), Beyond bibliometrics: Harnessing multidimensional indicators of scholarly impact. Cambridge, MA: MIT Press.
Leydesdorff, L., & Franse, S. (2009). The communication of meaning in social systems. Systems Research and Behavioral Science, 26(1), 109–117.
Leydesdorff, L. & Ivanova, I. A. (2014) Mutual redundancies in inter-human communication systems: Steps towards a calculus of processing meaning. Journal of the Association for Information Science and Technology, 65(2), 386–389.
Leydesdorff, L., & Strand, Ø. (2013). The Swedish system of innovation: Regional synergies in a knowledge-based economy. Journal of the American Society for Information Science and Technology, 64(9), 1890–1902. doi:10.1002/asi.22895.
Leydesdorff, L., & Sun, Y. (2009). National and international dimensions of the Triple Helix in Japan: University–industry–government versus international co-authorship relations. Journal of the American Society for Information Science and Technology, 60(4), 778–788.
Luhmann, N. (1986). The autopoiesis of social systems. In F. Geyer & J. V. D. Zouwen (Eds.), Sociocybernetic paradoxes (pp. 172–192). London: Sage.
Luhmann, N. (1995). Social systems. Stanford, CA: Stanford University Press.
Maturana, H. R., & Varela, F. (1980). Autopoiesis and cognition: The realization of the living. Boston: Reidel.
McGill, W. J. (1954). Multivariate information transmission. Psychometrika, 19(2), 97–116.
Padgett, J. F., & Powell, W. W. (2012). The emergence of organizations and markets. Princeton, NJ: Princeton University Press.
Parsons, T. (1951). The social system. New York: The Free Press.
Shannon, C. E. (1948). A mathematical theory of communication. Bell System Technical Journal, 27, 379–423; 623–656.
Strand, Ø., & Leydesdorff, L. (2013). Where is synergy in the Norwegian innovation system indicated? Triple Helix relations among technology, organization, and geography. Technological Forecasting and Social Change, 80(3), 471–484.
Strydom, P. (1999). Triple contingency: The theoretical problem of the public in communication societies. Philosophy & Social Criticism, 25(2), 1–25.
Ulanowicz, R. E. (2008). Autocatalysis. In S. E. Joergensen & B. D. Fath (Eds.), Systems ecology. Volume 1 of encyclopedia of ecology (Vol. 1, pp. 288–290). Oxford: Elsevier.
Ulanowicz, R. E. (2009a). The dual nature of ecosystem dynamics. Ecological Modelling, 220(16), 1886–1892.
Ulanowicz, R. E. (2009b). A third window: Natural life beyond Newton and Darwin. West Conshohocken, PA: Templeton Foundation Press.
Yeung, R. W. (2008). Information theory and network coding. New York, NY: Springer.
Author information
Authors and Affiliations
Corresponding author
Appendices
Appendix 1
Communication of information and meaning in a TH dynamic symmetry model
In a TH dynamic symmetry model, communication field W is described by the following equation:
Here i, j = 0,1; \( \partial_{0} = \partial_{t} \); \( \partial_{1} = - \partial_{x} \); \( \partial^{0} = \partial_{t} \); \( \partial^{1} = \partial_{x} \); summing on repeating indexes j is implied. Eq. (A.1) can be rewritten in a more explicit form:
W ij is defined by the formula:
W i , W j are three-component vectors in TH internal symmetry space, and two component vectors in (x, t) space. We can define these as: \( W_{i} = \left( {P,Q} \right) \); \( W^{i} = \left( { - P,Q} \right) \); P, Q are three-component vectors in the case of university–industry–government (TH) relations. Eq. (A.3) can be expressed in components:
and substituting this into (A.2) produces:
Setting: \( P = P\left( \xi \right) \); \( Q = Q\left( \xi \right) \); \( \xi = x - t \), so that \( P_{x} = - P_{t} = P_{\xi } \); \( Q_{x} = - Q_{t} = Q_{\xi } \), summing first and second equations in (A.5), and assuming that: \( Q \times Q_{t} = gQ \times \left( {P \times Q} \right) \); \( P \times P_{x} = gP \times \left( {P \times Q} \right) \), we can rewrite Eq. (A.5) in the form:
This can be interpreted as describing two fields: P, Q propagating in a positive direction of the x axes.
We can show that Eq. (A.6) can be coupled to the equation describing these two fields P, Q propagating in a negative direction of the x axes (or propagating in a positive direction but reversed in time). Setting:
where P, Q are vectors: \( P = \left( {\begin{array}{*{20}c} {P_{1} } \\ {P_{2} } \\ {P_{3} } \\ \end{array} } \right) \); \( Q = \left( {\begin{array}{*{20}c} {Q_{1} } \\ {Q_{2} } \\ {Q_{3} } \\ \end{array} } \right) \), and the right sides of (A.7) are cross-products. Alongside the “time” version of Eq. (A.7) can be introduced the “space” version of this equation:
Each pair of Eqs. (A.7) and (A.8) describes the evolution of functions P and Q in time and space. We can obtain a combined space–time equation. Differentiating the first and second equations of the system (A.8) by t and taking into consideration (A.7) produces:
Setting further: \( P = P\left( \xi \right) \); \( Q = Q\left( \xi \right) \); \( \xi = x + t \), so that \( P_{x} = P_{t} = P_{\xi } \); \( Q_{x} = Q_{t} = Q_{\xi } \), and subtracting in (A.9) the second equation from the first, we obtain:
Equation (A.10) coincides in full with Eq. (A.6) if we set \( \beta = \delta = g \), but refers to solutions which evolve reversed in time.
Appendix 2
Analytical solution for a TH communication field
Defining the functions
We can write a system of equations for a TH communication field (coupling constant 2g in the right-hand side of systems (B.2), (B.3) are not mentioned)
from system (2) we obtain:
Expressing Q in terms of P from system (B.3) and substituting the result into system (B.2), we get:
Here: \( a = - 2g\alpha \); \( b = - 2g\beta \); \( c = - 2g\gamma \). System (B.4) can be solved analytically and has a well-known solution (Kamke 1971)
Here: \( r^{2} = a^{2} + b^{2} + c^{2} \); \( aC_{1} + bC_{2} + cC_{3} = 0. \) From the equation:
we get after integration:
and from the equation:
we also get:
This means that integral curves are situated simultaneously on the sphere surface. The same equation holds for \( Q \):
From (B.5) we get:
So that:
Constants C 1, C 2, C 3 can be calculated from system (B.5):
Rights and permissions
About this article
Cite this article
Ivanova, I.A., Leydesdorff, L. A simulation model of the Triple Helix of university–industry–government relations and the decomposition of the redundancy. Scientometrics 99, 927–948 (2014). https://doi.org/10.1007/s11192-014-1241-7
Received:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11192-014-1241-7




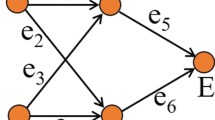
 . However, one can add minus signs to the tails of the arrows in this figure, and thus obtain the trade-off between evolutionary auto-catalysis (“self-organization”) versus historical organization as in Fig.
. However, one can add minus signs to the tails of the arrows in this figure, and thus obtain the trade-off between evolutionary auto-catalysis (“self-organization”) versus historical organization as in Fig.