Abstract
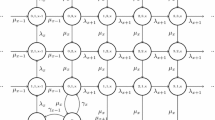
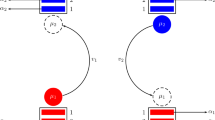
In this paper we consider a single-server, cyclic polling system with switch-over times. A distinguishing feature of the model is that the rates of the Poisson arrival processes at the various queues depend on the server location. For this model we study the joint queue length distribution at polling epochs and at the server’s departure epochs. We also study the marginal queue length distribution at arrival epochs, as well as at arbitrary epochs (which is not the same in general, since we cannot use the PASTA property). A generalised version of the distributional form of Little’s law is applied to the joint queue length distribution at customer’s departure epochs in order to find the waiting time distribution for each customer type. We also provide an alternative, more efficient way to determine the mean queue lengths and mean waiting times, using Mean Value Analysis. Furthermore, we show that under certain conditions a Pseudo-Conservation Law for the total amount of work in the system holds. Finally, typical features of the model under consideration are demonstrated in several numerical examples.
Article PDF
Similar content being viewed by others
Avoid common mistakes on your manuscript.
References
Boon, M.A.A.: A polling model with reneging at polling instants. Ann. Oper. Res. (2010, to appear). doi:10.1007/s10479-010-0758-2
Boon, M.A.A., Adan, I.J.B.F.: Mixed gated/exhaustive service in a polling model with priorities. Queueing Syst. 63, 383–399 (2009)
Borst, S.C.: Polling Systems. CWI Tracts, vol. 115 (1996)
Borst, S.C., Boxma, O.J.: Polling models with and without switchover times. Oper. Res. 45(4), 536–543 (1997)
Boxma, O.J.: Polling systems. In: From Universal Morphisms to Megabytes: A Baayen Space Odyssey. Liber Amicorum for P.C. Baayen, pp. 215–230. CWI, Amsterdam (1994)
Boxma, O.J., Groenendijk, W.P.: Pseudo-conservation laws in cyclic-service systems. J. Appl. Probab. 24(4), 949–964 (1987)
Boxma, O.J., Kelbert, M.: Stochastic bounds for a polling system. Ann. Oper. Res. 48, 295–310 (1994)
Boxma, O.J., Weststrate, J.A., Yechiali, U.: A globally gated polling system with server interruptions, and applications to the repairman problem. Probab. Eng. Inf. Sci. 7, 187–208 (1993)
Boxma, O.J., van Wijk, A.C.C., Adan, I.J.B.F.: Polling systems with a gated-exhaustive discipline. In: ValueTools 2008 (Third International Conference on Performance Evaluation Methodologies and Tools, Athens, Greece, October 20–24, 2008)
Boxma, O.J., Bruin, J., Fralix, B.H.: Waiting times in polling systems with various service disciplines. Perform. Eval. 66, 621–639 (2009)
Boxma, O.J., Ivanovs, J., Kosiński, K., Mandjes, M.: Lévy-driven polling systems and continuous-state branching processes. Eurandom report 2009-026, Eurandom (2009)
Cohen, J.W.: The Single Server Queue, revised edn. North-Holland, Amsterdam (1982)
Eisenberg, M.: Queues with periodic service and changeover time. Oper. Res. 20(2), 440–451 (1972)
Fuhrmann, S.W.: Performance analysis of a class of cyclic schedules. Technical memorandum 81-59531-1, Bell Laboratories (March 1981)
Fuhrmann, S.W., Cooper, R.B.: Stochastic decompositions in the M/G/1 queue with generalized vacations. Oper. Res. 33(5), 1117–1129 (1985)
Gong, Y., de Koster, R.: A polling-based dynamic order picking system for online retailers. IIE Trans. 40, 1070–1082 (2008)
Ibe, O.C., Trivedi, K.S.: Two queues with alternating service and server breakdown. Queueing Syst. 7, 253–268 (1990)
Jain, M., Jain, A.: Working vacations queueing model with multiple types of server breakdowns. Appl. Math. Model. 34(1), 1–13 (2010)
Keilson, J., Servi, L.D.: The distributional form of Little’s Law and the Fuhrmann–Cooper decomposition. Oper. Res. Lett. 9(4), 239–247 (1990)
Kofman, D., Yechiali, U.: Polling systems with station breakdowns. Perform. Eval. 27–28, 647–672 (1996)
Levy, H., Sidi, M.: Polling systems: applications, modeling, and optimization. IEEE Trans. Commun. 38, 1750–1760 (1990)
Mandelbaum, A., Yechiali, U.: Optimal entering rules for a customer with wait option at an M/G/1 queue. Manag. Sci. 29(2), 174–187 (1983)
Nakdimon, O., Yechiali, U.: Polling systems with breakdowns and repairs. Eur. J. Oper. Res. 149, 588–613 (2003)
Resing, J.A.C.: Polling systems and multitype branching processes. Queueing Syst. 13, 409–426 (1993)
Shanthikumar, J.G.: On stochastic decomposition in M/G/1 type queues with generalized server vacations. Oper. Res. 36(4), 566–569 (1988)
Shogan, A.W.: A single server queue with arrival rate dependent on server breakdowns. Nav. Res. Logist. Q. 26(3), 487–497 (1979)
Takagi, H.: Queuing analysis of polling models. ACM Comput. Surv. (CSUR) 20, 5–28 (1988)
van der Mei, R.D., Resing, J.A.C.: Analysis of polling systems with two-stage gated service: fairness versus efficiency. In: Mason, L., Drwiega, T., Yan, J. (eds.) Proc. ICT-20—Managing Traffic Performance in Converged Networks: The Interplay Between Convergent and Divergent Forces, pp. 544–555. Springer, Berlin (2007)
Vishnevskii, V.M., Semenova, O.V.: Mathematical methods to study the polling systems. Autom. Remote Control 67(2), 173–220 (2006)
Winands, E.M.M.: Polling, Production & Priorities. PhD thesis, Eindhoven University of Technology (2007)
Winands, E.M.M., Adan, I.J.B.F., van Houtum, G.-J.: Mean value analysis for polling systems. Queueing Syst. 54, 35–44 (2006)
Wolff, R.: Stochastic Modeling and the Theory of Queues. Prentice-Hall, Englewood Cliffs (1989)
Author information
Authors and Affiliations
Corresponding author
Additional information
The research was done in the framework of the BSIK/BRICKS project, and of the European Network of Excellence Euro-NF.
Rights and permissions
Open Access This is an open access article distributed under the terms of the Creative Commons Attribution Noncommercial License (https://creativecommons.org/licenses/by-nc/2.0), which permits any noncommercial use, distribution, and reproduction in any medium, provided the original author(s) and source are credited.
About this article
Cite this article
Boon, M.A.A., van Wijk, A.C.C., Adan, I.J.B.F. et al. A polling model with smart customers. Queueing Syst 66, 239–274 (2010). https://doi.org/10.1007/s11134-010-9191-0
Received:
Revised:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11134-010-9191-0