Abstract
Direct state measurement (DSM) is a tomography method that allows for retrieving quantum states’ wave functions directly. However, a shortcoming of current studies on the DSM is that it does not provide access to noisy quantum systems. Here, we attempt to fill the gap by investigating the DSM measurement precision that undergoes the state-preparation-and-measurement (SPAM) errors. We manipulate a quantum controlled measurement framework with various configurations and compare the efficiency between them. Under such SPAM errors, the state to be measured lightly deviates from the true state, and the measurement error in the postselection process results in less accurate in the tomography. Our study could provide a reliable tool for SPAM errors tomography and contribute to understanding and resolving an urgent demand for current quantum technologies.






Similar content being viewed by others
References
Paris, M., Rehacek, J. (eds.): Quantum State Estimation. Lecture Notes in Physics, Springer, Berlin (2004)
Helsen, J., Xue, X., Vandersypen, L.M.K., Wehner, S.: A new class of efficient randomized benchmarking protocols. npj Quantum Inf. 5(1), 71 (2019). https://doi.org/10.1038/s41534-019-0182-7
Frank, F., Unden, T., Zoller, J., Said, R.S., Calarco, T., Montangero, S., Naydenov, B., Jelezko, F.: Autonomous calibration of single spin qubit operations. npj Quantum Inf. 3(1), 48 (2017). https://doi.org/10.1038/s41534-017-0049-8
Gheorghiu, A., Kapourniotis, T., Kashefi, E.: Verification of quantum computation: an overview of existing approaches. Theory Comput. Syst. 63(4), 715 (2019). https://doi.org/10.1007/s00224-018-9872-3
Preskill, J.: Quantum computing in the NISQ era and beyond. Quantum 2, 79 (2018). https://doi.org/10.22331/q-2018-08-06-79
Schlosshauer, M.: Decoherence and the Quantum-to-Classical Transition. Springer, Berlin (2007)
Jackson, C., van Enk, S.J.: Detecting correlated errors in state-preparation-and-measurement tomography. Phys. Rev. A 92, 042312 (2015). https://doi.org/10.1103/PhysRevA.92.042312
Kosaka, H., Inagaki, T., Rikitake, Y., Imamura, H., Mitsumori, Y., Edamatsu, K.: Spin state tomography of optically injected electrons in a semiconductor. Nature 457(7230), 702 (2009). https://doi.org/10.1038/nature07729
Vanner, M.R., Hofer, J., Cole, G.D., Aspelmeyer, M.: Cooling-by-measurement and mechanical state tomography via pulsed optomechanics. Nat. Commun. 4(1), 2295 (2013). https://doi.org/10.1038/ncomms3295
Lu, D., Xin, T., Yu, N., Ji, Z., Chen, J., Long, G., Baugh, J., Peng, X., Zeng, B., Laflamme, R.: Tomography is necessary for universal entanglement detection with single-copy observables. Phys. Rev. Lett. 116, 230501 (2016). https://doi.org/10.1103/PhysRevLett.116.230501
Baldwin, C.H., Deutsch, I.H., Kalev, A.: Strictly-complete measurements for bounded-rank quantum-state tomography. Phys. Rev. A 93, 052105 (2016). https://doi.org/10.1103/PhysRevA.93.052105
Lundeen, J.S., Sutherland, B., Patel, A., Stewart, C., Bamber, C.: Direct measurement of the quantum wavefunction. Nature 474(7350), 188 (2011). https://doi.org/10.1038/nature10120
Lundeen, J.S., Bamber, C.: Procedure for direct measurement of general quantum states using weak measurement. Phys. Rev. Lett. 108, 070402 (2012). https://doi.org/10.1103/PhysRevLett.108.070402
Shi, Z., Mirhosseini, M., Margiewicz, J., Malik, M., Rivera, F., Zhu, Z., Boyd, R.W.: Scan-free direct measurement of an extremely high-dimensional photonic state. Optica 2(4), 388 (2015)
Mirhosseini, M., Magaña Loaiza, O..S., Hashemi Rafsanjani, S..M., Boyd, R.W.: Compressive direct measurement of the quantum wave function. Phys. Rev. Lett 113, 090402 (2014). https://doi.org/10.1103/PhysRevLett.113.090402
Malik, M., Mirhosseini, M., Lavery, M.P.J., Leach, J., Padgett, M.J., Boyd, R.W.: Direct measurement of a 27-dimensional orbital-angular-momentum state vector. Nat. Commun. 5(1), 3115 (2014). https://doi.org/10.1038/ncomms4115
Bolduc, E., Gariepy, G., Leach, J.: Direct measurement of large-scale quantum states via expectation values of non-Hermitian matrices. Nat. Commun. 7(1), 10439 (2016). https://doi.org/10.1038/ncomms10439
Knarr, S.H., Lum, D.J., Schneeloch, J., Howell, J.C.: Compressive direct imaging of a billion-dimensional optical phase space. Phys. Rev. A023854 98, 023854 (2018). https://doi.org/10.1103/PhysRevA.98.023854
Thekkadath, G.S., Giner, L., Chalich, Y., Horton, M.J., Banker, J., Lundeen, J.S.: Direct measurement of the density matrix of a quantum system. Phys. Rev. Lett. 117, 120401 (2016). https://doi.org/10.1103/PhysRevLett.117.120401
Calderaro, L., Foletto, G., Dequal, D., Villoresi, P., Vallone, G.: Direct reconstruction of the quantum density matrix by strong measurements. Phys. Rev. Lett. 121, 230501 (2018). https://doi.org/10.1103/PhysRevLett.121.230501
Ho, L.B.: Improving direct state measurements by using rebits in real enlarged Hilbert spaces. Phys. Lett. A 383(4), 289 (2019). https://doi.org/10.1016/j.physleta.2018.10.047
Pan, W.W., Xu, X.Y., Kedem, Y., Wang, Q.Q., Chen, Z., Jan, M., Sun, K., Xu, J.S., Han, Y.J., Li, C.F., Guo, G.C.: Direct measurement of a nonlocal entangled quantum state. Phys. Rev. Lett. 123, 150402 (2019). https://doi.org/10.1103/PhysRevLett.123.150402
Gross, J.A., Dangniam, N., Ferrie, C., Caves, C.M.: Novelty, efficacy, and significance of weak measurements for quantum tomography. Phys. Rev. A 92, 062133 (2015). https://doi.org/10.1103/PhysRevA.92.062133
Sainz, I., Klimov, A.B.: Error estimation in the direct state tomography. EPL (Europhysics Lett.) 116(1), 10002 (2016). https://doi.org/10.1209/0295-5075/116/10002
Pang, S., Alonso, J.R.G., Brun, T.A., Jordan, A.N.: Protecting weak measurements against systematic errors. Phys. Rev. A 94, 012329 (2016). https://doi.org/10.1103/PhysRevA.94.012329
Ho, L.B.: Systematic errors in direct state measurements with quantum controlled measurements. J. Phys. B: Atom. Mol. Opt. Phys. 53(11), 115501 (2020). https://doi.org/10.1088/1361-6455/ab7881
von Neumann, J.: Mathematical Foundations of Quantum Mechanics. New Princeton University Press, Princeton (2018)
Hofmann, H.F.: Sequential measurements of non-commuting observables with quantum controlled interactions. New J. Phys. 16(6), 063056 (2014). https://doi.org/10.1088/1367-2630/16/6/063056
Ogawa, K., Yasuhiko, O., Kobayashi, H., Nakanishi, T., Tomita, A.: A framework for measuring weak values without weak interactions and its diagrammatic representation. New J. Phys. 21(4), 043013 (2019). https://doi.org/10.1088/1367-2630/ab0773
Shikano, Y., Hosoya, A.: Weak values with decoherence. J. Phys. A: Math. Theor. 43(2), 025304 (2009). https://doi.org/10.1088/1751-8113/43/2/025304
Weiss, T., Romero-Isart, O.: Quantum motional state tomography with nonquadratic potentials and neural networks. Phys. Rev. Res. 1, 033157 (2019). https://doi.org/10.1103/PhysRevResearch.1.033157
Palmieri, A.M., Kovlakov, E., Bianchi, F., Yudin, D., Straupe, S., Biamonte, J.D., Kulik, S.: Experimental neural network enhanced quantum tomography. npj Quantum Inf. 6(1), 20 (2020). https://doi.org/10.1038/s41534-020-0248-6
Vallone, G., Dequal, D.: Strong measurements give a better direct measurement of the quantum wave function. Phys. Rev. Lett. 116, 040502 (2016). https://doi.org/10.1103/PhysRevLett.116.040502
Nielsen, M.A., Chuang, I.L.: Quantum Computation and Quantum Information. Cambridge University Press, Cambridge (2000)
Maccone, L., Rusconi, C.C.: State estimation: a comparison between direct state measurement and tomography. Phys. Rev. A 89, 022122 (2014). https://doi.org/10.1103/PhysRevA.89.022122
Ho, L.B., Tuan, K.Q., Nguyen, H.Q.: tqix: A toolbox for quantum in X: X: quantum measurement, quantum tomography, quantum metrology, and others. Computer Phys. Commun. (2021). https://doi.org/10.1016/j.cpc.2021.107902
Mezzadri, F.: How to generate random matrices from the classical compact groups. Not. Am. Math. Soc. 54(5), 592 (2007)
Carvacho, G., Graffitti, F., D’Ambrosio, V., Hiesmayr, B.C., Sciarrino, F.: Experimental investigation on the geometry of GHZ states. Sci. Rep. 7(1), 13265 (2017). https://doi.org/10.1038/s41598-017-13124-6
Li, D.X., Zheng, T.Y., Shao, X.Q.: Adiabatic preparation of multipartite GHZ states via Rydberg ground-state blockade. Opt. Express 27(15), 20874 (2019). https://doi.org/10.1364/OE.27.020874
Paris, M.G.A.: Quantum estimation for quantum technology. Int. J. Quantum Inf. 07(supp01), 125 (2009). https://doi.org/10.1142/S0219749909004839
Nielsen, M.A., Caves, C.M., Schumacher, B., Barnum, H.: Information-theoretic approach to quantum error correction and reversible measurement. Proc. R. Soc. Lond. Ser. A: Math. Phys. Eng. Sci. 454, 277 (1998). https://doi.org/10.1098/rspa.1998.0160
Watanabe, Y., Sagawa, T., Ueda, M.: Optimal measurement on noisy quantum systems. Phys. Rev. Lett. 104, 020401 (2010). https://doi.org/10.1103/PhysRevLett.104.020401
Yang, M., Xiao, Y., Liao, Y.W., Liu, Z.H., Xu, X.Y., Xu, J.S., Li, C.F., Guo, G.C.: Zonal reconstruction of photonic wavefunction via momentum weak measurement. Laser Photonics Rev. 14(5), 1900251 (2020). https://doi.org/10.1002/lpor.201900251
Acknowledgements
This work was supported by JSPS KAKENHI Grant Number 20F20021 and the Vietnam National University under Grant Number QG.20.17. LBH would like to thank Shikano for pointing out Ref. [30].
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Appendices
An example of noisy quantum state preparation
We provide an example for preparing the quantum state GHZ\(_3\) that contains noise. Consider a quantum circuit as shown in Fig. 7 (inset). Therein, three qubits \(q_0, q_1\), and \(q_2\) are prepared in the ground state, i.e., \(|000\rangle \). Applying a sequence of Hadamard (H) gate onto \(q_0\), control-NOT (CNOT) gate onto \(q_0, q_1\), and control-NOT gate onto \(q_0, q_2\), as shown in the inset figure, respectively, we obtain the output state as
which is the GHZ\(_3\) state. We simulate this state in Fig. 7 (left) by using the IBM Qiskis package. It can be seen that the amplitudes of \(|000\rangle \) and \(|111\rangle \) are the same and equal to \(1/\sqrt{2}\).
Inset: Quantum circuit for generating GHZ\(_3\) state. Initially, three qubits \(q_0, q_1\), and \(q_2\) are prepared in the ground state, i.e., \(|000\rangle \). Quantum Hadamard gate and Control-NOT gates are applied to transform the initial state to the desired state. See detailed in Appendix A. Left: the amplitudes of the components in the GHZ\(_3\) state after applying H and CNOT gates onto the initial state \(|000\rangle \). Right: the amplitudes of the components in the GHZ\(_3\) state under the imperfection of the Hadamard gate. These amplitudes are lightly deviated from the true values in the left figure
Now, let us assume the imperfection in the Hadamard gate as follows. We first decompose the Hadamard gate into the two rotations: \(\pi /2\) about the Y-axis, and \(\pi \) about the Z-axis, such that
where the rotation matrices are given as
Under the imperfection, assume that the rotation angles will deviate from their true values, such as \(\pi /2 + \alpha \), and \(\pi + \beta \), where \(\alpha \) and \(\beta \) are small angles. Without loss of generality, we can choose \(\beta = 0\) since the operation of \(R_z(\theta )\) does not affect the amplitudes of the quantum state. As a result, the Hadamard gate becomes
Therefore, the final state becomes
which slightly different from the true GHZ\(_3\) state. Here, \(\frac{1}{2}(|a|^2 + |b|^2) = 1\). This is an example of a noisy state-preparation process. In Fig. 7 (right), we simulate the GHZ\(_3\) state assuming that the Hadamard gate is under the imperfection at \(\alpha = 0.2\) as an example. Under this noisy state-preparation, the two components \(|000\rangle \) and \(|111\rangle \) are deviated from \(1/\sqrt{2}\). In general, all the components will be deviated from their values as we have modeled from Eq. 3 in the main text.
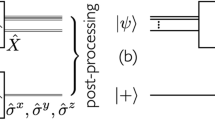
Quantum controlled measurements for pure states
In this appendix, we closely follow the quantum controlled measurement framework introduced by Ogawa et al. [29] for pure states, and we derive it under the noise using our denotation to make the work self-consistency.
We consider the initial joint state of the target system and the control qubit probe as \(|\varPsi \rangle = |\psi '\rangle \otimes |\xi \rangle \), where
where \(\mathcal {N}\) is the normalization factor, \(\psi '_n = \frac{\psi _n+\delta _n}{\mathcal {N}}\), and \(\delta _n = x_1 + ix_2\) is a complex random number, where \(x_1\) and \(x_2\) are random numbers that follow the normal distribution \(f(x) = \frac{1}{\sigma \sqrt{2\pi }} \exp [-\frac{1}{2}(\frac{x}{\sigma })^2]\).
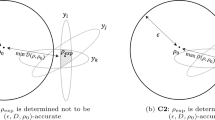
1.1 For C1
For C1, following Ogawa et al. [29], we consider the interaction as
After the interaction, the joint state becomes
We postselect the target system onto the conjugate basis
where \(\mathcal {M}\) is the normalization factor, and \(\mathfrak {c}_m = \frac{1+\kappa _m}{\mathcal {M}}\). Here, \(\kappa _m\) is a real random number and distributes according to the normal distribution. The final control qubit state is given by
where \(\varGamma = \sum _{m=0}^{d-1} \mathfrak {c}_{m}\psi '_{m}\).
Finally, we measure the control qubit probe in the Pauli basis \(|j\rangle \in \{ |0\rangle , |1\rangle , |+\rangle , |-\rangle , |L\rangle , |R\rangle \}\), where \(|\pm \rangle = \frac{1}{\sqrt{2}}\bigl (|0\rangle \pm |1\rangle \bigr ), |L\rangle = \frac{1}{\sqrt{2}}\bigl (|0\rangle + i|1\rangle \bigr ), |R\rangle = \frac{1}{\sqrt{2}}\bigl (|0\rangle - i|1\rangle \bigr )\). The probability for measuring \(|j\rangle \langle j|\) is \(P_j = |\langle j|\eta \rangle |^2\) explicitly give
As a result, the real and imaginary parts of the amplitude \(\psi '_n\) are reproduced as
1.2 For C2
For C2, the interaction is given by [29]
The joint state after the interaction is given by
After the interaction, the target system is postselected onto \(|n\rangle \) while the remaining state of the control qubit probe is given as
Measuring the control qubit probe in the Pauli basis as above, we obtain
Then, we have
Quantum controlled measurements for mixed states
We consider the joint state \(\varLambda \) as following
1.1 For C1
The interaction operator is given the same as above:
After the interaction, the joint state evolves to
which is explicitly written as
Here, c.c stands for ‘complex conjugate.’ After postselecting this state onto
the final state of the control qubit probe becomes
Explicitly,
Using Fourier transformation on \(\varLambda ''_{10}(n,k)\), we obtain
To get \(\varLambda _{10}''(n,k)\) and \(\varLambda ''_{11}(n,k)\), the control qubit is measured as follows:
where \(P_j = \mathrm{Tr}[|j\rangle \langle j|\varLambda '']\) is the probability when measuring the control qubit probe in the element j of the Pauli basis.
1.2 For C2
In this case, the interaction is
After applying this interaction U, the initial joint state becomes
which is explicitly given as
Next, we postselect this state onto \(|n\rangle \langle n|\) and get
Explicitly,
Using Fourier transformation on \(\varLambda _{01}''(n,k)\), we obtain:
where \(\varLambda _{01}''(n,k)\) is obtained by measuring the control qubit probe as follows:
Quantum fisher information
In this section, we show how to calculate the total quantum Fisher information (QFI) for \(|\psi '\rangle \) state:
where \(\psi _n\) is unknown. The normalization constant is
Here, note that we consider both \(\psi _n\) and \(\delta _n\) are real for simplicity. First, we calculate \(\partial _{\psi _n}|\psi '\rangle \), where we are using \(\partial _{\psi _n}\) as shorthand for \(\partial /\partial {\psi _n}\). We have
The Quantum Fisher Information (QFI) is given by
Then, the total QFI is
Rights and permissions
About this article
Cite this article
Tuan, K.Q., Nguyen, H.Q. & Ho, L.B. Direct state measurements under state-preparation-and-measurement errors. Quantum Inf Process 20, 197 (2021). https://doi.org/10.1007/s11128-021-03144-7
Received:
Accepted:
Published:
DOI: https://doi.org/10.1007/s11128-021-03144-7