Skip to main content
SpringerLink
Log in

Self-protected quantum algorithms based on quantum state tomography

  • Published:
Quantum Information Processing Aims and scope Submit manuscript

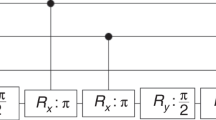
Abstract

Only a few classes of quantum algorithms are known which provide a speed-up over classical algorithms. However, these and any new quantum algorithms provide important motivation for the development of quantum computers. In this article new quantum algorithms are given which are based on quantum state tomography. These include an algorithm for the calculation of several quantum mechanical expectation values and an algorithm for the determination of polynomial factors. These quantum algorithms are important in their own right. However, it is remarkable that these quantum algorithms are immune to a large class of errors. We describe these algorithms and provide conditions for immunity.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Shor P.W.: Why haven’t more quantum algorithms been found. J. ACM 50, 87–90 (2003)

    Article  MathSciNet  Google Scholar 

  2. Shor P.: Progress in quantum algorithms. Quantum Inf. Process. 3, 5–13 (2004)

    Article  MATH  MathSciNet  Google Scholar 

  3. Shor P.W.: Polynomial-time algorithms for prime factorization and discrete logarithms on a quantum computer. SIAM J. Comp. 26, 1484–1509 (1997)

    Article  MATH  MathSciNet  Google Scholar 

  4. Grover, L.K.: A fast quantum mechanical algorithm for database search. In: Proceedings of the 28th Annual ACM Symposium on the Theory of Computing, pp. 212–219. ACM, New York, NY (1996)

  5. Feynman R.P.: Simulating physics with computers. Int. J. Theor. Phys. 21, 467–488 (1982)

    Article  MathSciNet  Google Scholar 

  6. Lloyd S.: Universal quantum simulators. Science 273, 1073–1078 (1996)

    Article  ADS  MathSciNet  Google Scholar 

  7. Meyer D.A.: Quantum mechanics of lattice gas automata: one-particle plane waves and potentials. Phys. Rev. E 55, 5261–5269 (1997)

    Article  ADS  Google Scholar 

  8. Boghosian B.M., Taylor W.: Quantum lattice-gas models for the many-body Schrodinger equation in d dimensions. Phys. Rev. E 57, 54–66 (1998)

    Article  MathSciNet  ADS  Google Scholar 

  9. Zalka C.: Simulating quantum systems on a quantum computer. Proc. R. Soc. Lond. Ser. A 454, 313–322 (1998)

    MATH  ADS  Google Scholar 

  10. Abrams D.S., Lloyd S.: Simulation of many-body fermi systems on a universal quantum computer. Phys. Rev. Lett. 79, 2586–2589 (1997)

    Article  ADS  Google Scholar 

  11. Terhal B.M.: Bell inequalities and the separability criterion. Phys. Lett. A 271, 319–326 (2000)

    Article  MATH  ADS  MathSciNet  Google Scholar 

  12. Freedman M.H., Kitaev A., Wang Z.: Simulation of topological field theoriesby quantum computers. Commun. Math. Phys. 227, 587–603 (2002)

    Article  MATH  ADS  MathSciNet  Google Scholar 

  13. Lidar D.A., Wang H.: Calculating the thermal rate constant with exponential speed- up on a quantum computer. Phys. Rev. E 59, 2429–2438 (1999)

    Article  ADS  Google Scholar 

  14. Ortiz G., Gubernatis J.E., Knill E., Laflamme R.: Quantum algorithms for fermionic simulations. Phys. Rev. A 64, 022319-1–022319-14 (2001)

    Article  ADS  Google Scholar 

  15. Wu L.-A., Byrd M.S., Lidar D.A.: Polynomial-time simulation of the BCS Hamiltonian. Phys. Rev. Lett. 89, 057904-1–057904-4 (2002)

    ADS  MathSciNet  Google Scholar 

  16. Jane E., Vidal G., Dür W., Zoller P., Cirac J.I.: Simulation of quantum dynamics with quantum optical systems. Quantum Inf. Comp. 3, 015–037 (2003)

    Google Scholar 

  17. Shor P.W.: Scheme for reducing decoherence in quantum memory. Phys. Rev. A 52, R2493–R2496 (1995)

    Article  ADS  Google Scholar 

  18. Steane A.: Quantum computing. Rep. Prog. Phys. 61, 117 (1998)

    Article  MathSciNet  ADS  Google Scholar 

  19. Calderbank A.R., Shor P.W.: Good quantum error correcting codes exist. Phys. Rev. A 54, 1098–1105 (1996)

    Article  ADS  Google Scholar 

  20. Gottesman, D.: Stabilizer Codes and Quantum Error Correction. Ph.D. thesis, California Institute of Technology, Pasadena, CA (1997). Eprint quant-ph/9705052

  21. Zanardi P., Rasetti M.: Noiseless quantum codes. Phys. Rev. Lett. 79, 3306–3309 (1997)

    Article  ADS  Google Scholar 

  22. Duan L.-M., Guo G.-C.: Reducing decoherence in quantum-computer memory with all quantum bits coupling to the same environment. Phys. Rev. A 57, 737–741 (1998)

    Article  ADS  Google Scholar 

  23. Lidar D.A., Chuang I.L., Whaley K.B.: Decoherence free subspaces for quantum computation. Phys. Rev. Lett. 81, 2594–2597 (1998)

    Article  ADS  Google Scholar 

  24. Knill E., Laflamme R., Viola L.: Theory of quantum error correction for general noise. Phys. Rev. Lett. 84, 2525–2528 (2000)

    Article  MATH  ADS  MathSciNet  Google Scholar 

  25. Zanardi P., Rasetti M.: Holonomic quantum computation. Phys. Lett. A 264, 94–99 (1999)

    Article  MATH  ADS  MathSciNet  Google Scholar 

  26. Pachos J., Zanardi P., Rasetti M.: Non-Abelian Berry connections for quantum computation. Phys. Rev. A 61, 010305(R)-1–010305(R)-4 (1999)

    Article  MathSciNet  Google Scholar 

  27. Vogel K., Risken H.: Determination of quasiprobability distributions in terms of probability distributions for the rotated quadrature phase. Phys. Rev. A 40, 2847–2849 (1989)

    Article  ADS  Google Scholar 

  28. Somma R., Ortiz G., Gubernatis J.E., Knill E., Laflamme R.: Simulating physical phenomena by quantum networks. Phys. Rev. A 65, 042323-1–042323-14 (2002)

    Article  ADS  Google Scholar 

  29. Paz J.P., Roncaglia A.: Quantum gate arrays can be programmed to evaluate the expectation value of any operator. Phys. Rev. A 68, 052316-1–052316-5 (2003)

    Article  ADS  Google Scholar 

  30. Alves C.M., Horodecki P., Oi D.K.L., Kwek L.C., Ekert A.K.: Direct estimation of functionals of density operators by local operations and classical communication. Phys. Rev. A 68, 032306-1–032306-4 (2003)

    Article  ADS  Google Scholar 

  31. D’Ariano G.M., Macchiavello C., Perinotti P.: Optimal phase estimation for qubit mixed states. Phys. Rev. A 72, 042327-1–042327-4 (2005)

    ADS  Google Scholar 

  32. Kitaev, A.: Quantum measurements and the Abelian Stabilizer Problem (1995) (quant-ph/9511026)

  33. Cleve R., Ekert A., Macchiavello C., Mosca M.: Quantum algorithms revisited. Proc. R. Soc. Lond. Ser. A 454, 339–354 (1998)

    Article  MATH  MathSciNet  ADS  Google Scholar 

  34. Abrams D.S., Lloyd S.: Quantum algorithm providing exponential speed increase for finding eigenvalues and eigenvectors. Phys. Rev. Lett. 83, 5162–5165 (1999)

    Article  ADS  Google Scholar 

  35. Nielsen M.A., Chuang I.L.: Quantum Computation and Quantum Information. Cambridge University Press, Cambridge, UK (2000)

    MATH  Google Scholar 

  36. Emerson J., Weinstein Y.S., Saraceno M., Lloyd S., Cory D.G.: Pseudo-random unitary operators for quantum information processing. Science 302, 2098–2100 (2003)

    Article  ADS  MathSciNet  Google Scholar 

  37. Mohseni M., Lidar D.A.: Direct characterization of quantum dynamics. Phys. Rev. Lett. 97, 170501-1–170501-4 (2006)

    Article  ADS  Google Scholar 

  38. Braunstein S.L.: Some limits to precision phase measurement. Phys. Rev. A 49, 69–75 (1994)

    Article  ADS  Google Scholar 

  39. Giovannetti V., Lloyd S., Maccone L.: Quantum-enhanced measurements: beating the standard quantum limit. Science 306, 1330–1336 (2004)

    Article  ADS  Google Scholar 

  40. Brown K.R., Clark R.J., Chuang I.L.: Limitations of quantum simulation examined by simulating a pairing Hamiltonian using nuclear magnetic resonance. Phys. Rev. Lett. 97, 050504-1–050504-4 (2006)

    Article  ADS  Google Scholar 

  41. Jordan P., Wigner E.: Über das Paulische Äquivalenzverbot. Z. Phys. 47, 631–651 (1928)

    Article  ADS  Google Scholar 

  42. Wu L.-A., Lidar D.A.: Qubits as parafermions. J. Math. Phys. 43, 4506–4525 (2002)

    Article  MATH  ADS  MathSciNet  Google Scholar 

  43. Mahler G., Weberruss V.A.: Quantum Networks: Dynamics of Open Nanostructures, 2nd edn. Springer, Berlin (1998)

    Google Scholar 

  44. Jakóbczyk L., Siennicki M.: Geometry of Bloch vectors in two-qubit system. Phys. Lett. A 286, 383–390 (2001)

    Article  MATH  ADS  MathSciNet  Google Scholar 

  45. Byrd M.S., Khaneja N.: Characterization of the positivity of the density matrix in terms of the coherence vector representation. Phys. Rev. A 68, 062322-1–062322-13 (2003)

    Article  ADS  MathSciNet  Google Scholar 

  46. Kimura G.: The bloch vector for N-level systems. Phys. Lett. A 314, 339–349 (2003)

    Article  MATH  ADS  MathSciNet  Google Scholar 

  47. Byrd M.S., Wu L.-A., Lidar D.A.: Overview of quantum error prevention and leakage elimination. J. Mod. Opt. 51, 2449–2460 (2004)

    Article  MATH  ADS  Google Scholar 

  48. Byrd M.S., Lidar D.A.: Empirical determination of Bang–Bang Operations. Phys. Rev. A 67, 012324-1–012324-14 (2003)

    Article  ADS  Google Scholar 

Download references

Author information

Author notes

  1. Lian-Ao Wu

    Present address: Theoretical Physics and History of Science, University of the Basque Country, P.O. Box 644, 48080, Bilbao, Spain

Authors and Affiliations

  1. Department of Chemistry, Chemical Physics Theory Group, Center for Quantum Information and Quantum Control, University of Toronto, 80 St. George Street, Toronto, ON, Canada, M5S 3H6

    Lian-Ao Wu

  2. Department of Physics and Department of Computer Science, Southern Illinois University, Carbondale, IL, 62901, USA

    Mark S. Byrd

Authors
  1. Lian-Ao Wu
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Mark S. Byrd
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Mark S. Byrd.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Wu, LA., Byrd, M.S. Self-protected quantum algorithms based on quantum state tomography. Quantum Inf Process 8, 1–12 (2009). https://doi.org/10.1007/s11128-008-0090-9

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11128-008-0090-9

Keywords

PACS

Search

Navigation