Skip to main content
SpringerLink
Log in

An Obstacle Problem for Nonlocal Equations in Perforated Domains

  • Published:
Potential Analysis Aims and scope Submit manuscript

Abstract

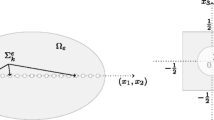
In this paper we analyze the behavior of solutions to a nonlocal equation of the form J ∗ u (x) − u (x) = f (x) in a perforated domain Ω ∖ A 𝜖 with u = 0 in \(A^{\epsilon } \cup {\Omega }^{c}\) and an obstacle constraint, u ≥ ψ in Ω ∖ A 𝜖. We show that, assuming that the characteristic function of the domain Ω ∖ A 𝜖 verifies \(\chi _{\epsilon } \rightharpoonup \mathcal {X}\) weakly ∗ in \(L^{\infty }({\Omega })\), there exists a weak limit of the solutions u 𝜖 and we find the limit problem that is satisfied in the limit. When \(\mathcal {X} \not \equiv 1\) in this limit problem an extra term appears in the equation as well as a modification of the obstacle constraint inside the domain.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Andreu-Vaillo, F., Mazón, J.M., Rossi, J.D., Toledo, J.: Nonlocal Diffusion Problems. Mathematical Surveys and Monographs, vol. 165. AMS (2010)

  2. Barles, G., Chasseigne, E., Imbert, C.: On the Dirichlet problem for second-order elliptic integro-differential equations. Indiana Univ. Math. J. 57(1), 213–246 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  3. Caffarelli, L.A., Mellet, A.: Random homogenization of an obstacle problem. Ann. Inst. H. Poincaré Anal. Non Linéaire 26(2), 375–395 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  4. Caffarelli, L.A., Mellet, A.: Random homogenization of fractional obstacle problems. Netw. Heterog. Media 3(3), 523–554 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  5. Calvo-Jurado, C., Casado-Díaz, J., Luna-Laynez, M.: Homogenization of nonlinear Dirichlet problems in random perforated domains. Nonlinear Anal. 133, 250–274 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  6. Cioranescu, D., Murat, F.: A strange term coming from nowhere. Progress Nonl. Diff. Eq. Their Appl. 31, 45–93 (1997)

    MATH  Google Scholar 

  7. Cioranescu, D., Damlamian, A., Donato, P., Griso, G., Zaki, R.: The periodic unfolding method in domains with holes. SIAM J. Math. Anal. 44(2), 718–760 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  8. Cioranescu, D., Donato, P.: An Introduction to Homogenization. Oxford Lecture Series in Mathematics and its Applications, vol. 17. Oxford University Press (1999)

  9. Cioranescu, D., Saint Jean Paulin, J.: Homogenization in open sets with holes. J. Math. Anal. Appl. 71(2), 590–607 (1979)

    Article  MathSciNet  MATH  Google Scholar 

  10. Chasseigne, E., Felmer, P., Rossi, J.D., Topp, E.: Fractional decay bounds for nonlocal zero order heat equations. Bull. Lond. Math. Soc. 46(5), 943–952 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  11. Cortazar, C., Elgueta, M., Rossi, J.D.: Nonlocal diffusion problems that approximate the heat equation with Dirichlet boundary conditions. Israel J. Math. 170(1), 53–60 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  12. Cortazar, C., Elgueta, M., Rossi, J.D., Wolanski, N.: How to approximate the heat equation with Neumann boundary conditions by nonlocal diffusion problems. Arch. Ration. Mech. Anal. 187(1), 137–156 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  13. Courant, R., Hilbert, D.: Methods of Mathematical Physics, vol. I. Interscience, New York (1953)

    MATH  Google Scholar 

  14. Du, Q., Gunzburger, M., Lehoucq, R.B., Zhou, K.: A nonlocal vector calculus, nonlocal volume-constrained problems, and nonlocal balance laws. Math. Models Methods Appl. Sci. 23(3), 493–540 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  15. Felmer, P., Topp, E.: Uniform equicontinuity for a family of zero order operators approaching the fractional Laplacian. Comm. Partial Differential Equations 40(9), 1591–1618 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  16. Friedman, A.: Variational Principles and Free-boundary Problems. Wiley (1982)

  17. García Melián, J., Rossi, J.D.: On the principal eigenvalue of some nonlocal diffusion problems. J. Differential Equations. 246(1), 21–38 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  18. Lehoucq, R.B., Silling, S.A.: Force flux and the peridynamic stress tensor. J. Mech. Phys. Solids 56(4), 1566–1577 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  19. Necas, J.: Les Méthodes Directes En Théorie Des Équations Elliptiques. Masson, Paris (1967)

    MATH  Google Scholar 

  20. Pereira, M.C., Rossi J.D.: Nonlocal problems in perforated domains. Preprint. Available at http://mate.dm.uba.ar/~jrossi/PD_final_version.pdf

  21. Rauch, J., Taylor, M.: Potential and scattering theory on wildly perturbed domains. J. Funct. Anal. 18, 27–59 (1975)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

The first author (MCP) is partially supported by CNPq 302960/2014-7 and 471210/2013-7, FAPESP 2015/17702-3 (Brazil) and the second author (JDR) by MINCYT grant MTM2016-68210 (Spain).

Author information

Authors and Affiliations

  1. Dpto. de Matemática Aplicada, IME, Universidade de São Paulo, Rua do Matão 1010, São Paulo, SP, Brazil

    Marcone C. Pereira

  2. Dpto. de Matemáticas, FCEyN, Universidad de Buenos Aires, Ciudad Universitaria Pab 1, 1428, Buenos Aires, Argentina

    Julio D. Rossi

Authors
  1. Marcone C. Pereira
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Julio D. Rossi
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Marcone C. Pereira.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Pereira, M.C., Rossi, J.D. An Obstacle Problem for Nonlocal Equations in Perforated Domains. Potential Anal 48, 361–373 (2018). https://doi.org/10.1007/s11118-017-9639-5

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11118-017-9639-5

Keywords

Mathematics Subject Classification (2010)

Search

Navigation