Skip to main content
SpringerLink
Log in

Finite Element Method and Discontinuous Galerkin Method for Stochastic Scattering Problem of Helmholtz Type in ℝd (d = 2, 3)

  • Published:
Potential Analysis Aims and scope Submit manuscript

Abstract

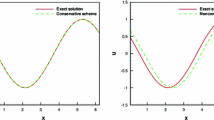
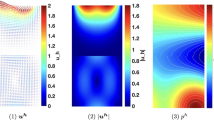
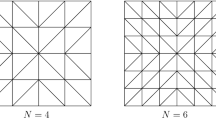
In this paper, we address the finite element method and discontinuous Galerkin method for the stochastic scattering problem of Helmholtz type in ℝd (d = 2, 3). Convergence analysis and error estimates are presented for the numerical solutions. The effects of the noises on the accuracy of the approximations are illustrated. Results of the numerical experiments are provided to examine our theoretical results.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Allen, E.J., Novosel, S.J., Zhang, Z.: Difference approximation of some linear stochastic partial differential equations. Stochastics Stochastics Rep. 64, 117–142 (1998)

    MathSciNet  MATH  Google Scholar 

  2. Alvarez, G.B., Loula, A.F.D., Dutra, E.G., Rochinha, F.A.: A discontinuous finite element formulation for Helmholtz equation. Comput. Methods Appl. Mech. Engrg. 195(33–36), 4018–4035 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  3. Babuska, I., Tempone, R., Zouraris, G.: Galerkin fnite element approximations of stochastic elliptic partial differential equations. SIAM J. Numer. Anal. 42, 800–825 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  4. Brenner, S., Scott, L.R.: The Mathematical Theory of Finite Element Methods. Springer, Berlin Heidelberg New York (1994)

    MATH  Google Scholar 

  5. Buckdahn, R., Pardoux, E.: Monotonicity methods for white noise driven quasi-linear PDEs. Progr. Probab. 22, 219–233 (1990)

    MathSciNet  Google Scholar 

  6. Cao, Y.Z., Yang, H.T., Yin, L.: Finite element methods for semilinear elliptic stochastic partial differential equations. Numer. Math. 106(2), 181–198 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  7. Chen, Z.M., Liu, X.Z.: An adaptive perfectly matched layer technique for time-harmonic scattering problems. SIAM J. Numer. Anal. 43(2), 645–671 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  8. Colton, D., Kress, R.: Integral Equation Methods in Scattering Theory. Wiley, New York (1983)

    MATH  Google Scholar 

  9. Du, Q., Zhang, T.Y.: Numerical approximation of some linear stochastic partial differential equations driven by special additive noise. SIAM J. Numer. Anal. 4, 1421–1445 (2002)

    Article  MathSciNet  Google Scholar 

  10. Gyöngy, I.: Lattice approximations for stochastic quasi-linear parabolic partial differential equations driven by space-time white noise II. Potential Anal. 11, 1–37 (1999)

    Article  MathSciNet  MATH  Google Scholar 

  11. Hausenblas, E.: Error analysis for approximation of stochastic differential equations driven by Poisson random measures. SIAM J. Numer. Anal. 40, 87–113 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  12. Johnson, C.: Numerical Solution of Partial Differential Equations by the Finite Element Method. Cambridge University Press, Cambridge (1994)

    Google Scholar 

  13. Keese, A., Matthies, H.: Parallel computation of stochastic groundwater flow. In: Wolf, D., Munster, G., Kremer, M. (eds.) NIC Symposium 2004, Proceedings, NIC series, vol. 20, pp. 399–408. John von Neumann Institute for Computing, Julich (2003)

  14. Kloeden, P., Platen, E.: Numerical Solution of Stochastic Differential Equations. Springer, New York (1992)

    MATH  Google Scholar 

  15. Lin, Y.P., Zhang, K., Zou, J.: Studies on some perfectly matched layers for one-dimensional time-dependent systems. Adv. Comput. Math. (2008, in press)

  16. Martínez, T., Sanz-Solé, M.: A lattice scheme for stochastic partial differential equations of elliptic type in dimension d≥4. Appl. Math. Optim. 54(3), 343–368 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  17. McLean, W.: Strongly Elliptic Systems and Boundary Integral Equations. Cambridge University Press, Cambridge (2000)

    MATH  Google Scholar 

  18. Melenk, J.M.: On generalized finite element methods. Ph.D. thesis, University of Maryland at College Park (http://www.anum.tuwien.ac.at/~melenk/) (1995)

  19. Milstein, G.N., Platen, E., Schurz, H.: Balanced implicit methods for stochastic systems. SIAM J. Numer. Anal. 35, 1010–1019 (1998)

    Article  MathSciNet  MATH  Google Scholar 

  20. Mitchell, A.R., Griffiths, D.F.: The Finite Difference Method in Partial Differential Equations. Wiley, Chichester (1980)

    MATH  Google Scholar 

  21. Perugia, I., Schötzau, D.: An hp-analysis of the local discontinuous Galerkin method for diffusion problems. J. Sci. Comput. 17, 561–571 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  22. Walsh, J.B.: An Introduction to Stochastic Partial Differential Equations. Lecture Notes in Mathematics, vol. 1180, pp. 265–439. Springer, Berlin Heidelberg New York (1986)

    Google Scholar 

  23. Zhang, K., Zhang, R., Yin, Y.G., Yu, S.: Domain decomposition methods for linear and semi linear elliptic stochastic partial differential equations. Appl. Math. Comput. (2008 in press)

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, Florida A&M, University, Tallahassee, FL, 32307, USA

    Yanzhao Cao

  2. Department of Mathematics, Jilin University, Changchun, Jilin, 130023, People’s Republic of China

    Ran Zhang & Kai Zhang

Authors
  1. Yanzhao Cao
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Ran Zhang
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Kai Zhang
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Kai Zhang.

Additional information

The first author is supported by NSF under grand number 0609918 and AFOSR under grant numbers FA9550-06-1-0234 and FA9550-07-1-0154, the second author is supported by NSFC(10671082, 10626026, 10471054), and the third author is supported by NNSF (No. 10701039 of China) and 985 program of Jilin University.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Cao, Y., Zhang, R. & Zhang, K. Finite Element Method and Discontinuous Galerkin Method for Stochastic Scattering Problem of Helmholtz Type in ℝd (d = 2, 3). Potential Anal 28, 301–319 (2008). https://doi.org/10.1007/s11118-008-9078-4

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11118-008-9078-4

Keywords

Mathematics Subject Classifications (2000)

Search

Navigation