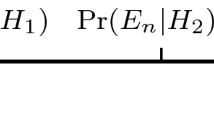Abstract
Jonathan Weisberg has argued that Jeffrey Conditioning is inherently “anti-holistic” By this he means, inter alia, that JC does not allow us to take proper account of after-the-fact defeaters for our beliefs. His central example concerns the discovery that the lighting in a room is red-tinted and the relationship of that discovery to the belief that a jelly bean in the room is red. Weisberg’s argument that the rigidity required for JC blocks the defeating role of the red-tinted light rests on the strong assumption that all posteriors within the distribution in this example are rigid on a partition over the proposition that the jelly bean is actually red. But individual JC updates of propositions do not require such a broad rigidity assumption. Jeffrey conditionalizers should consider the advantages of a modest project of targeted updating focused on particular propositions rather than seeking to update the entire distribution using one obvious partition. Although Weisberg’s example fails to show JC to be irrelevant or useless, other problems he raises for JC (the commutativity and inputs problems) remain and actually become more pressing when we recognize the important role of background information.
Similar content being viewed by others
Notes
Of course, from both a foundationalist and a holist perspective, no experience could really make one certain of F, so imagining our "finding out that" F and treating this as being "given" F at probability 1 is an idealization.
Weisberg's argument also applies if F is negatively relevant to E in q but does not completely remove the support for E in q.
I am silently changing Weisberg's notation for negation.
Note that here and in what follows, in phrases such as "two or more propositions" and "another directly affected proposition," I am not speaking of the negation of a proposition as "another" directly affected proposition. So, for example, E and F as described above are different "directly affected propositions" from one another as that concept is being used here. It is necessarily true that if E is directly affected, its negation is as well. What is less intuitive is that E and F are both directly affected.
Once we realize that the posteriors for F on E are not rigid, we can see that there are other posteriors for propositions influenced epistemically by F that are also not rigid on {E, ~E}, because they are indirectly affected by the experience via F—for example, the posteriors for T Someone has been tampering with the light in the room.
It could be argued that the holist should regard F′ as a rebutting defeater of E′, in Weisberg's terminology, rather than an undercutting defeater (2009, p. 807). If we can give any meaning to the notion of my being a poor judge of my color experiences (and a foundationalist will have serious doubts about this), it would seem to have some such consequence as that I may "judge" that I am having any of a very large number of different color experiences on any given occasion. This would seem to reduce the probability before the fact of my judging that I am having a red-jelly-bean experience when I enter the room, since on ordinary background information (without the proposed defeater), my expectations before the fact about jelly bean appearances will be related instead to my information about the actual colors of jelly beans, which are more limited in number. Thus, it would seem that F′, if it is taken in the way that the holist wants it to be taken, should be negatively relevant to E′ even in p. I am waiving this point, however, for purposes of the main discussion, since Weisberg apparently intends F′ to be treated as an undercutting defeater of E′.
If the thoroughgoing holist maintains that for any partition proposed there is always some defeater proposition that has non-rigid posteriors over that partition (see Weisberg 2009, p. 797), it seems that the holist may be envisaging a distribution with an infinite number of distinct propositions, which is psychologically implausible. It would be interesting to see strong holists address this question.
Since we are now discussing a partition constructed using more than one proposition and hence made up of members which are conjunctions, it would be a good idea to define the concept of "including" a proposition in a partition. For purposes of this discussion, a proposition or a negation of a proposition is included in a partition just in case either it is a member of the partition or is equivalent to the disjunction of all the members of a partition in which it appears as a conjunct. My thanks to a reviewer for Philosophical Studies for raising this question and to Timothy McGrew for helping me to refine the definition.
It is not necessary, for purposes of using the JC formula, that the uncertain evidence used as a partition be in some sense "as close as possible" to the experience. As long as P(H|E) and P(H|~E) are rigid in the transition induced by the experience, the uncertain evidence used as a partition can be much "higher up" in one's evidential framework than a simple sentence like "The jelly bean is red." This point about "closeness" to the experience has been emphasized by James Hawthorne (personal communication). See also Hawthorne's discusson of a basis (2004, p. 93). This point is crucial to the argument in McGrew and McGrew (2008).
Zynda's interest is in seeing how the notion of confirmation is affected by the possibility that one proposition in the partition made up of directly affected propositions is positively relevant to some H while another is negatively relevant—in other words, where one portion of the partition seems to confirm H while another seems to disconfirm it (Zynda 1995, p. 77).
This objection was raised by a reviewer.
This objection was raised by a reviewer.
For a full discussion of this proposal concerning rigidity, see McGrew (2010).
Someone who wished to use the JC formula could avoid the commutativity problem by treating JC as an analytical tool while assuming an underlying structure of true Bayesian conditioning on certainties. That approach would, of course, be contrary to thoroughgoing holism in any event, thus confirming Weisberg's point that commutativity and thoroughgoing holism as he defines it are incompatible.
References
Christensen, D. (1992). Confirmational holism and bayesian epistemology. Philosophy of Science, 59, 540–557.
Field, H. (1978). A note on Jeffrey conditionalization. Philosophy of Science, 45, 361–367.
Hawthorne, J. (2004). Three models of sequential belief updating on uncertain evidence. Journal of Philosophical Logic, 33, 89–123.
Jeffrey, R. (1965). The logic of decision. Chicago: University of Chicago Press.
McGrew, L. (2010). Probability kinematics and probability dynamics. Journal of Philosophical Research, 35, 89–105.
McGrew, T., & McGrew, L. (2008). Foundationalism, probability, and mutual support. Erkenntnis, 68, 55–77.
Weisberg, J. (2009). Commutativity or holism? A dilemma for Jeffrey conditionalizers. British Journal for the Philosophy of Science, 60, 793–812.
Zynda, L. (1995). Old evidence and new theories. Philosophical Studies, 77, 67–95.
Acknowledgments
I wish to thank James Hawthorne, Timothy McGrew, and an anonymous reviewer for Philosophical Studies for comments on this paper.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
McGrew, L. Jeffrey conditioning, rigidity, and the defeasible red jelly bean. Philos Stud 168, 569–582 (2014). https://doi.org/10.1007/s11098-013-0145-3
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11098-013-0145-3