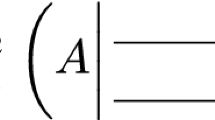Abstract
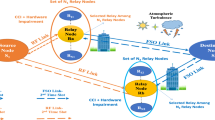
In this research work, coded cooperation protocol has been opted for combating impact of interference on a combined network of radio frequency (RF) and free space optical (FSO) systems called the mixed RF/FSO relaying system. The fading on the RF link has been considered to follow \(\alpha -\mu\) distribution, whereas the atmospheric turbulence on the FSO link has been considered to follow double generalized Gamma turbulence model. With a purpose of introducing practicality to the system, it is considered that the relay node operates in the vicinity of multiple co-channel interferers. For the proposed system model, closed-form expressions for outage probability and ergodic capacity have been derived. The exact analysis for outage probability has been taken forward to derive asymptotic expression in terms of elementary functions. The findings of the research work suggest that coded cooperation can be utilized for combating the impact of interference at the relay node. Importantly, for mixed RF/FSO transmissions, coded cooperation outperforms decode-and-forward relaying strategy for the considered system model.




Similar content being viewed by others
Data availability
Data sharing not applicable to this article as no datasets were generated or analyzed during the current study
References
Al-Eryani, Y.F., Salhab, A.M., Zummo, S.A., Alouini, M.-S.: Two-way multiuser mixed RF/FSO relaying: performance analysis and power allocation. IEEE/OSA J. Opt. Commun. Networking 10(4), 396–408 (2018)
AlQuwaiee, H., Ansari, I.S., Alouini, M.-S.: On the performance of free-space optical communication systems over double generalized gamma channel. IEEE J. on Selec. Areas Commun. 33(9), 1829–1840 (2015)
Aminikashani, M., Uysal, M., Kavehrad, M.: A novel statistical channel model for turbulence-induced fading in free-space optical systems. CoRR arXiv:1502.00348 (2015)
Annamalai, A., Palat, R.C., Matyjas, J.: Estimating ergodic capacity of cooperative analog relaying under different adaptive source transmission techniques. 2010 IEEE Sarnoff Symposium , 1–5 (2010)
Ansari, I.S., Yilmaz, F., Alouini, M.-S.: Impact of pointing errors on the performance of mixed RF/FSO dual-hop transmission systems. IEEE Wireless Communications Letters 2(3), 351–354 (2013)
Ansari, I.S., Yilmaz, F., Alouini, M.-S.: Performance analysis of free-space optical links over \({\cal{M}}\acute{a}\)laga (\({\cal{M}}\)) turbulence channels with pointing errors. IEEE Trans. Wireless Commun. 15(1), 91–102 (2016)
Chergui, H., Benjillali, M., Alouini, M.S.: Rician K-factor-based analysis of XLOS service probability in 5G outdoor ultra-dense networks. IEEE Wirel. Commun. Lett. (2018)
Dahrouj, H., Douik, A., Rayal, F., Al-Naffouri, T.Y., Alouini, M.-S.: Cost-effective Hybrid RF/FSO Backhaul solution for next generation wireless systems. IEEE Wirel. Commun. 22(5), 98–104 (2015)
Dias, U. S., Yacoub, M. D.: On the \(\alpha -\mu\) autocorrelation and power spectrum functions: Field trials and validation, GLOBECOM 2009 - 2009 IEEE, Global Telecommunications Conference (2009), 1, 1–6 (2009)
Djordjevic, G., Petkovic, M., Cvetkovic, A., Karagiannidis, G.: Mixed RF/FSO relaying with outdated channel state information. IEEE J. Sel. Areas Commun. 33(9), 1935–1948 (2015)
El-Malek, A.H.A., Salhab, A.M., Zummo, S.A., Alouini, M.-S.: Effect of RF interference on the security-reliability tradeoff analysis of multiuser mixed RF/FSO relay networks with power allocation. J. Lightwave Technol. 35(9), 1490–1505 (2017)
Filho, J.C.S.S., Yacoub, M.D.: Nakagami-m approximation to the sum of M non-identical independent Nakagami-m variates. Electron. Lett. 40(15), 951–952 (2004)
Gradshteyn, I.S., Ryzhik, I.M.: Table of integrals, series and products, New York, NY. Academic, USA (2000)
Heidarpour, A.R., Ardakani, M., Tellambura, C.: Network-coded cooperation with outdated CSI. IEEE Commun. Lett. 22(8), 1720–1723 (2018)
Hunter, T.E., Nosratinia, A.: Diversity through coded cooperation. IEEE Trans. Wireless Commun. 5(2), 283–289 (2006)
Jiang, B., Yang, S., Bao, J., Liu, C., Tang, X., Zhu, F.: Optimized polar coded selective relay cooperation with iterative threshold decision of pseudo posterior probability, IEEE Access, 7,53 078 (2019)
Kashani, M.A., Uysal, M., Kavehrad, M.: A novel statistical channel model for turbulence-induced fading in free-space optical systems. IEEE/OSA J. Lightw. Technol. 33(11), 2303–2312 (2015)
Kilbas, A.: H-transforms: Theory and applications. analytical methods and special functions. Taylor and Francis, NewYork (2004)
Lapidoth, A., Moser, S.M., Wigger, M.A.: On the capacity of free space optical intensity channels. IEEE Trans. Inf. Theory 55(10), 4449–4461 (2009)
Lee, E., Park, J., Han, D., Yoon, G.: Performance analysis of the asymmetric dual-hop relay transmission with mixed RF/FSO links. IEEE Photonics Technol. Lett. 23(21), 1642–1644 (2011)
Mittal, P., Gupta, K.: An integral involving generalized function of two variables, Proceedings of the Indian Academy of Sciences - Section A, 75, 117–123 (1972)
Olver, F.W.J., Lozier, D.W., C. W. C. Boisvert, R. F.: NIST handbook of mathematical functions, 1st edn. Cambridge Univ. Press, New York, NY, USA (2010)
Petkovic, M. I., Cvetkovic, A. M., Djordjevic, G. T., Karagiannidis, G. K.: Outage performance of the mixed RF/FSO relaying channel in the presence of interference, Wireless Pers Commun., 96, 2999–3014, (2017)
Saber, M.J., Mazloum, J., Sazdar, A.M., Keshavarz, A., Piran, M.J.: On secure mixed RF-FSO decode-and-forward relaying systems with energy harvesting. IEEE Systems Journal , 1–4 (2019)
Soleimani-Nasab, E., Uysal, M.: Generalized performance analysis of mixed RF/FSO cooperative systems. IEEE Trans. Wireless Commun. 15(1), 714–727 (2016)
Varshney, N., Puri, P.: Performance analysis of decode-and-forward-based mixed MIMO-RF/FSO cooperative systems with source mobility and imperfect csi. J. Lightwave Technol. 35(11), 2070–2077 (2017)
Varshney, N., Jagannatham, A. K.: Cognitive decode-and-forward MIMO-RF/FSO cooperative relay networks, IEEE Commun. Lett, 21, 893–896, (2017)
Wolfram, I.: Wolfram, research, mathematica edition: Version 10.0. champaign, Wolfram Research, Inc. (2010)
Yang, L., Hasna, M.O., Ansari, I.S.: Unified performance analysis for multiuser mixed \(\eta\) - \(\mu\) and \({\cal{M}}\) - distribution dual-hop RF/FSO systems. IEEE Trans. Commun. 65(8), 3601–3613 (2017)
Zedini, E., Ansari, I.S., Alouini, M.-S.: Performance analysis of mixed Nakagami-m and Gamma-Gamma dual-hop FSO transmission systems. IEEE Photonics J. 7(1), 1–20 (2015)
Acknowledgements
The author acknowledges the editor and anonymous reviewer for providing inputs that improved the quality of the manuscript.
Funding
No funding agency is involved in the research work.
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
This article is part of the Topical Collection on Optical and Quantum Sciences in Africa, Guest edited by Salah Obayya, Alex Quandt, Andrew Forbes, Malik Maaza, Abdelmajid Belafhal and Mohamed Farhat.
Appendix
Appendix
A. Derivation of Eq. ( 15 )
In this section, the derivation steps of of Eq. (15) are elaborated. Noting the fact that from (14) and (6), it can be formulated as
With the aid of Wolfram (2010), the Meijer-G can be expressed in terms of complex integral as
Similarly, \(f_{\gamma _{{sd}}}(x)\) can be expressed as
From (35), (36) and (34), it can be established that
The above expression can be simplified as
Comparing the Mellin Barnes type double complex contour integral with [Mittal and Gupta (1972), Eq. (1.1)], the closed-form expression in (18) can be obtained to complete the proof.
B. Derivation of Eq. ( 20 )
Using the definition of Fox’s H-function in [Mittal and Gupta (1972), Eq. (1.1)], we have
Now, consider the following complex integral
With the aid of [Kilbas (2004), Eq. (1.2), (1.3), (1.132)], \({\mathbf {h}}_1\) can be expressed as follows
This completes the proof for derivation of (20).
Rights and permissions
About this article
Cite this article
Upadhya, A. Coded cooperation for combating interference in mixed RF/FSO cooperative relays. Opt Quant Electron 53, 577 (2021). https://doi.org/10.1007/s11082-021-03193-3
Received:
Accepted:
Published:
DOI: https://doi.org/10.1007/s11082-021-03193-3