Abstract
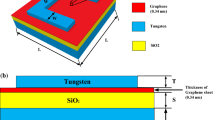
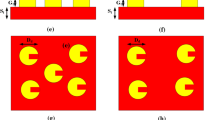
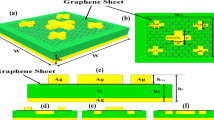
We present graphene metasurface based efficient and broadband solar absorber composed of a periodically arranged array of C-shaped metasurface placed above the dielectric layer. The proposed broadband graphene-based solar absorber is analyzed in terms of absorption characteristic with 82.7% absorption in the infrared region (280–380 THz), 86.5% absorption in the visible region (430–770 THz) and 92.99% absorption in Ultraviolet region (780–1000 THz). The simulation results are analyzed from 100 to 1200 THz in terms of absorption and reflection response with and without multiple graphene strips placed above the dielectric layer. The wide absorption band can be flexibly tuned from low-frequency band to high-frequency band by adjusting different design parameters of the proposed absorber. The proposed absorber design will be used as a building block for designing graphene-based sensors, optoelectronic devices, energy harvesting devices, and photovoltaic devices.












Similar content being viewed by others
References
Akimov, Y.A., Koh, W.S.: Resonant and nonresonant plasmonic nanoparticle enhancement for thin-film silicon solar cells. Nanotechnology 21(23), 235201 (2010). https://doi.org/10.1088/0957-4484/21/23/235201
Arik, K., Abdollahramezani, S., Farajollahi, S., Khavasi, A., Rejaei, B.: Design of mid-infrared ultra-wideband metallic absorber based on circuit theory. Optics Commun. 381, 309–313 (2016). https://doi.org/10.1016/j.optcom.2016.07.014
Arik, K., AbdollahRamezani, S., Khavasi, A.: Polarization insensitive and broadband terahertz absorber using graphene disks. Plasmonics 12(2), 393–398 (2017). https://doi.org/10.1007/s11468-016-0276-4
Cao, C., Cheng, Y.: Quad-band plasmonic perfect absorber for visible light with a patchwork of silicon nanorod resonators. Materials 11(10), 1954 (2018). https://doi.org/10.3390/ma11101954
Cao, C., Cheng, Y.: A broadband plasmonic light absorber based on a tungsten meander-ring-resonator in the visible region. Appl. Phys. A 125(1), 15 (2019). https://doi.org/10.1007/s00339-018-2310-1
Charola, S., Patel, S.K., Parmar, J., Ladumor, M., Vigneshwaran, D.: Broadband graphene-based metasurface solar absorber. Microw. Optic. Technol. Lett. (2019). https://doi.org/10.1002/mop.32156
Chen, M., Sun, W., Cai, J., Chang, L., Xiao, X.: Frequency-tunable terahertz absorbers based on graphene metasurface. Optics Commun. 382, 144–150 (2017). https://doi.org/10.1016/j.optcom.2016.07.077
Chen, F., Cheng, Y., Luo, H.: A broadband tunable terahertz metamaterial absorber based on single-layer complementary gammadion-shaped graphene. Materials 13(4), 860 (2020). https://doi.org/10.3390/ma13040860
Cheng, Y., Du, C.: Broadband plasmonic absorber based on all silicon nanostructure resonators in visible region. Opt. Mater. 98, 109441 (2019). https://doi.org/10.1016/j.optmat.2019.109441
Cheng, Y., Mao, X.S., Wu, C., Wu, L., Gong, R.: Infrared non-planar plasmonic perfect absorber for enhanced sensitive refractive index sensing. Opt. Mater. 53, 195–200 (2016). https://doi.org/10.1016/j.optmat.2016.01.053
Cheng, Y., Zhang, H., Mao, X.S., Gong, R.: Dual-band plasmonic perfect absorber based on all-metal nanostructure for refractive index sensing application. Mater. Lett. 219, 123–126 (2018). https://doi.org/10.1016/j.matlet.2018.02.078
Cheng, Y., Luo, H., Chen, F., Gong, R.: Triple narrow-band plasmonic perfect absorber for refractive index sensing applications of optical frequency. OSA Contin. 2(7), 2113–2122 (2019). https://doi.org/10.1364/OSAC.2.002113
Dave, V., Sorathiya, V., Guo, T., Patel, S.K.: Graphene-based tunable broadband far-infrared absorber. Superlattices Microstruct. 124, 113–120 (2018). https://doi.org/10.1016/j.spmi.2018.10.013
Deng, H., Mathai, C.J., Gangopadhyay, S., Gao, J., Yang, X.: Ultra-broadband infrared absorption by tapered hyperbolic multilayer waveguides. Opt. Express 26(5), 6360–6370 (2018). https://doi.org/10.1364/OE.26.006360
Gao, H., Peng, W., Chu, S., Cui, W., Liu, Z., Yu, L., Jing, Z.: Refractory ultra-broadband perfect absorber from visible to near-infrared. Nanomaterials 8(12), 1038 (2018). https://doi.org/10.3390/nano8121038
Guo, T., Argyropoulos, C.: Broadband polarizers based on graphene metasurfaces. Opt. Lett. 41(23), 5592–5595 (2016). https://doi.org/10.1364/OL.41.005592
Heidari, M.H., Sedighy, S.H.: Broadband wide-angle polarization-insensitive metasurface solar absorber. JOSA A 35(4), 522–525 (2018). https://doi.org/10.1364/JOSAA.35.000522
Huang, M., Cheng, Y., Cheng, Z., Chen, H., Mao, X., Gong, R.: Based on graphene tunable dual-band terahertz metamaterial absorber with wide-angle. Optics Commun. 415, 194–201 (2018a). https://doi.org/10.1016/j.optcom.2018.01.051
Huang, M.L., Cheng, Y.Z., Cheng, Z.Z., Chen, H.R., Mao, X.S., Gong, R.Z.: Design of a broadband tunable terahertz metamaterial absorber based on complementary structural graphene. Materials 11(4), 540 (2018b). https://doi.org/10.3390/ma11040540
Katrodiya, D., Jani, C., Sorathiya, V., Patel, S.K.: Metasurface based broadband solar absorber. Opt. Mater. 89, 34–41 (2019). https://doi.org/10.1016/j.optmat.2018.12.057
Landy, N.I., Sajuyigbe, S., Mock, J.J., Smith, D.R., Padilla, W.J.: Perfect metamaterial absorber. Phys. Rev. Lett. 100(20), 207402 (2008). https://doi.org/10.1103/PhysRevLett.100.207402
Liang, Q., Wang, T., Lu, Z., Sun, Q., Fu, Y., Yu, W.: Metamaterial-based two-dimensional plasmonic subwavelength structures offer the broadest waveband light harvesting. Adv. Optic. Mater. 1(1), 43–49 (2013). https://doi.org/10.1002/adom.201200009
Liu, X., Starr, T., Starr, A.F., Padilla, W.J.: Infrared spatial and frequency selective metamaterial with near-unity absorbance. Phys. Rev. Lett. 104(20), 207403 (2010). https://doi.org/10.1103/PhysRevLett.104.207403
Liu, B., Tang, C., Chen, J., Xie, N., Tang, H., Zhu, X., Park, G.S.: Multiband and broadband absorption enhancement of monolayer graphene at optical frequencies from multiple magnetic dipole resonances in metamaterials. Nanoscale Res. Lett. 13(1), 153 (2018). https://doi.org/10.1186/s11671-018-2569-3
Niu, X., Qi, D., Wang, X., Cheng, Y., Chen, F., Li, B., Gong, R.: Improved broadband spectral selectivity of absorbers/emitters for solar thermophotovoltaics based on 2D photonic crystal heterostructures. JOSA A 35(11), 1832–1838 (2018). https://doi.org/10.1364/JOSAA.35.001832
Ogawa, S., Kimata, M.: Metal-insulator-metal-based plasmonic metamaterial absorbers at visible and infrared wavelengths: a review. Materials 11(3), 458 (2018). https://doi.org/10.3390/ma11030458
Palik, E. D. (Ed.). (1998). Handbook of optical constants of solids (Vol. 3). Academic Pres, Cambridge.
Patel, S.K., Ladumor, M., Sorathiya, V., Guo, T.: Graphene-based tunable grating structure. Mater. Res. Express 6(2), 025602 (2018). https://doi.org/10.1088/2053-1591/aaea9a
Patel, S.K., Shah, K.H., Kosta, Y.P.: Frequency-reconfigurable and high-gain metamaterial microstrip-radiating structure. Waves Random Complex Media 29(3), 523–539 (2019a). https://doi.org/10.1080/17455030.2018.1452309
Patel, S., Charola, S., Parmar, J., Ladumor, M.: Broadband metasurface solar absorber in the visible and near-infrared region. Mater Res Express 6(8), 086213 (2019b). https://doi.org/10.1088/2053-1591/ab207d
Patel, S.K., Charola, S., Jani, C., Ladumor, M., Parmar, J., Guo, T.: Graphene-based highly efficient and broadband solar absorber. Opt. Mater. 96, 109330 (2019c). https://doi.org/10.1016/j.optmat.2019.109330
Rana, A.S., Mehmood, M.Q., Jeong, H., Kim, I., Rho, J.: Tungsten-based ultrathin absorber for the visible regime. Sci. Rep. 8(1), 2443 (2018). https://doi.org/10.1038/s41598-018-20748-9
Rufangura, P., Sabah, C.: Graphene-based wideband metamaterial absorber for solar cells application. J. Nanophotonics 11(3), 036008 (2017). https://doi.org/10.1117/1.JNP.11.036008
Sang, T., Gao, J., Yin, X., Qi, H., Wang, L., Jiao, H.: Angle-insensitive broadband bbsorption enhancement of graphene using a multi-grooved metasurface. Nanoscale Res. Lett. 14(1), 105 (2019). https://doi.org/10.1186/s11671-019-2937-7
Smith, D.R., Pendry, J.B., Wiltshire, M.C.: Metamaterials and negative refractive index. Science 305(5685), 788–792 (2004). https://doi.org/10.1126/science.1096796
Sorathiya, V., Patel, S.K., Katrodiya, D.: Tunable graphene-silica hybrid metasurface for far-infrared frequency. Opt. Mater. 91, 155–170 (2019). https://doi.org/10.1016/j.optmat.2019.02.053
Thomas, L., Sorathiya, V., Patel, S.K., Guo, T.: Graphene-based tunable near-infrared absorber. Microw. Optic. Technol. Lett. 61(5), 1161–1165 (2019). https://doi.org/10.1002/mop.31712
Xu, J., Li, R., Wang, S., Han, T.: Ultra-broadband linear polarization converter based on anisotropic metasurface. Opt. Express 26(20), 26235–26241 (2018). https://doi.org/10.1364/OE.26.026235
Yao, G., Ling, F., Yue, J., Luo, C., Ji, J., Yao, J.: Dual-band tunable perfect metamaterial absorber in the THz range. Opt. Express 24(2), 1518–1527 (2016). https://doi.org/10.1364/OE.24.001518
Zhang, H., Zhang, H., Yang, J., Liu, J.: Ultra-broadband infrared metasurface absorber. Opt. Express 27(4), 5346–5350 (2019). https://doi.org/10.1364/OE.27.005346
Acknowledgements
The authors would like to express their sincere thanks to Prof. Dr. Truong Khang Nguyen, Division of Computational Physics, Institute for Computational Science, Ton Duc Thang University, Ho Chi Minh City, Vietnam for giving his value suggestion, comments and support to complete this work as effective.
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
About this article
Cite this article
Patel, S.K., Charola, S., Parmar, J. et al. Broadband and efficient graphene solar absorber using periodical array of C-shaped metasurface. Opt Quant Electron 52, 250 (2020). https://doi.org/10.1007/s11082-020-02379-5
Received:
Accepted:
Published:
DOI: https://doi.org/10.1007/s11082-020-02379-5