Skip to main content
SpringerLink
Log in

Efficient high-order exponential time differencing methods for nonlinear fractional differential models

  • Original Paper
  • Published:
Numerical Algorithms Aims and scope Submit manuscript

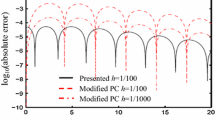
Abstract

Exponential integrators, due to their robust stability properties, have been considered as reliable schemes for numerical solutions of stiff systems. In this paper, we propose generalized exponential time differencing (GETD) schemes for nonlinear fractional differential equations of order α ∈ (0,1). First, we improve the suboptimal performance of the multistep GETD schemes. Using graded mesh, uniform optimal convergence rates under no additional smoothness requirements are obtained. Second, we develop and analyze novel second-order and third-order accurate predictor-corrector type GETD schemes. Using linear stability analysis and numerical illustrations, we demonstrate that the newly introduced schemes have better stability properties than the multistep GETD schemes. Partial fraction decompositions of global Padé approximations for Mittag-Leffler function are used for efficient implementation. Numerical examples involving nonlinear scalar equations and stiff systems are provided to illustrate the theoretical findings.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5

Similar content being viewed by others

References

  1. Ahmed, E., El-Sayed, A.M.A., El-Saka, H.A.A.: Equilibrium points, stability and numerical solutions of fractional-order predator–prey and rabies models. J. Math. Anal. Appl. 325(1), 542–553 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  2. Baffet, D., Hesthaven, J.S.: High-order accurate local schemes for fractional differential equations. J. Sci. Comput. 70, 355–385 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  3. Beylkin, G., Keiser, J.M., Vozovoi, L.: A new class of time discretization schemes for the solution of nonlinear PDEs. J. Comput. Phys. 147(2), 362–387 (1998)

    Article  MathSciNet  MATH  Google Scholar 

  4. Brunner, H.: The numerical solution of weakly singular Volterra integral equations by collocation on graded meshes. Math. Comput. 45, 417–437 (1985)

    Article  MathSciNet  MATH  Google Scholar 

  5. Cao, W., Zeng, F., Zhang, Z., Karniadakis, G.E.: Implicit-explicit difference schemes for nonlinear fractional differential equations with nonsmooth solutions. SIAM J. Sci. Comput. 38(5), A3070–A3093 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  6. Cox, S.M., Matthews, P.C.: Exponential time differencing for stiff systems. J. Comput. Phys. 176(2), 430–455 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  7. Diethelm, K., Ford, N.J., Freed, A.D.: A predictor-corrector approach for the numerical solution of fractional differential equations. Nonlinear Dynam. 29(1–4), 3–22 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  8. Diethelm, K., Ford, N.J., Freed, A.D.: Detailed error analysis for a fractional Adams method. Numerical Algorithms 36(1), 31–52 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  9. Furati, K.M., Sarumi, I.O., Khaliq, A.Q.M.: Fractional model for the spread of covid-19 subject to government intervention and public perception. Appl. Math. Model. 95, 89–105 (2021)

    Article  MathSciNet  MATH  Google Scholar 

  10. Furati, K.M., Yousuf, M., Khaliq, A.: Fourth-order methods for space fractional reaction–diffusion equations with non-smooth data. Int. J. Comput. Math. 95(6-7), 1240–1256 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  11. Garrappa, R.: On linear stability of predictor-corrector algorithms for fractional differential equations. Int. J. Comput. Math. 87(10), 2281–2290 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  12. Garrappa, R.: Trapezoidal methods for fractional differential equations: theoretical and computational aspects. Math. Comput. Simul. 110, 96–112 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  13. Garrappa, R., Popolizio, M.: Generalized exponential time differencing methods for fractional order problems. Comput. Math. Appl. 62, 876–890 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  14. Gorenflo, R., Kilbas, A.A., Mainardi, F., Rogosin, S. V.: Mittag-leffler functions. Related topics and applications. Springer (2014)

  15. Hochbruck, M., Ostermann, A.: Explicit exponential Runge Kutta methods for semilinear parabolic problems. SIAM J. Numer. Anal. 43(3), 1069–1090 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  16. Hochbruck, M., Ostermann, A.: Exponential integrators. Acta Numerica 19, 209–286 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  17. Gao, G., Sun, Z., Zhang, H.: A new fractional numerical differentiation formula to approximate the Caputo fractional derivative and its applications. J. Comput. Phys. 259, 33–50 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  18. Iserles, A.: A first course in the numerical analysis of differential equations, Cambridge texts in applied mathematics. Cambridge University Press, Cambridge, second edn. (2009)

  19. Jin, B., Li, B., Zhou, Z.: Numerical analysis of nonlinear subdiffusion equations. SIAM J. Numer. Anal. 56(1), 1–23 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  20. Kassam, A.-K., Trefethen, L.N.: Fourth-order time-stepping for stiff pdes. SIAM J. Sci. Comput. 26(4), 1214–1233 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  21. LeVeque, R.J.: Finite difference methods for ordinary and partial differential equations. SIAM (2007)

  22. Li, C., Yi, Q., Chen, A.: Finite difference methods with non-uniform meshes for nonlinear fractional differential equations. J. Comput. Phys. 316, 614–631 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  23. Liu, Y., Roberts, J., Yan, Y.: Detailed error analysis for a fractional Adams method with graded meshes. Numerical Algorithms 78(4), 1195–1216 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  24. Lubich, C.: Runge-kutta theory for volterra and abel integral equations of the second kind. Math. Comput. 41(163), 87–102 (1983)

    Article  MathSciNet  MATH  Google Scholar 

  25. Lubich, C.: Discretized fractional calculus. SIAM J. Math. Anal. 17(3), 704–719 (1986)

    Article  MathSciNet  MATH  Google Scholar 

  26. Lubich, C.: A stability analysis of convolution quadratures for abel-volterra integral equations. IMA J. Numer. Anal. 6(1), 87–101 (1986)

    Article  MathSciNet  MATH  Google Scholar 

  27. McLean, W., Mustapha, K.: A second-order accurate numerical method for a fractional wave equation. Numer. Math. 105(3), 481–510 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  28. Minchev, B., Wright, W.: A review of exponential integrators for first order semi-linear problems. Technical report 2/05 department of mathematics, NTNU (2005)

  29. Mustapha, K., Knio, O.M., Le Maître, O. P.: A second-order accurate numerical scheme for a time-fractional Fokker-Planck equation. IMA J. Numer. Anal., 2022 (to appear)

  30. Mustapha, K., McLean, W.: Superconvergence of a discontinuous Galerkin method for fractional diffusion and wave equations. SIAM J. Numer. Anal. 51(1), 491–515 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  31. Mustapha, K., Ryan, J.K.: Post-processing discontinuous Galerkin solutions to Volterra integro-differential equations: analysis and simulations. J. Comput. Appl. Math. 253, 89–103 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  32. Ford, M. R., Neville J., Luísa Morgado, M.: Nonpolynomial collocation approximation of solutions to fractional differential equations. Fract. Calc. Appl. Anal. 16, 874–891 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  33. Nguyen, T.B., Jang, B.: A high-order predictor-corrector method for solving nonlinear differential equations of fractional order. Fract. Calc. Appl. Anal. 20(2), 447–476 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  34. Perdikaris, P., Karniadakis, G.E.: Fractional-order viscoelasticity in one-dimensional blood flow models. Ann. Biomed. Eng. 42 (5), 1012–1023 (2014)

    Article  Google Scholar 

  35. Sardar, T., Rana, S., Bhattacharya, S., Al-Khaled, K., Chattopadhyay, J.: A generic model for a single strain mosquito-transmitted disease with memory on the host and the vector. Math. Biosci. 263, 18–36 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  36. Sarumi, I. O., Furati, K. M., Khaliq, A. Q. M.: Highly accurate global Padé approximations of generalized Mittag–Leffler function and its inverse. J. Sci. Comput., vol. 82(46) (2020)

  37. Sarumi, I. O., Furati, K. M., Khaliq, A. Q. M., Mustapha, K.: Generalized exponential time differencing schemes for stiff fractional systems with nonsmooth source term. J. Sci. Comput., vol. 86(23) (2021)

  38. Yanzhao Cao, Y.X., Herdman, Terry: A hybrid collocation method for Volterra integral equations with weakly singular kernels. SIAM J. Numer. Anal. 40(1), 364–381 (2004)

    MathSciNet  MATH  Google Scholar 

  39. Zeng, F.: Second-order stable finite difference schemes for the time-fractional diffusion-wave equation. J. Sci. Comput. 65(1), 411–430 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  40. Zhou, Y., Suzuki, J.L., Zhang, C., Zayernouri, M.: Implicit-explicit time integration of nonlinear fractional differential equations. Appl. Numer. Math. 156, 555–583 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  41. Zhu, L., Ju, L., Zhao, W.: Fast high-order compact exponential time differencing Runge–Kutta methods for second-order semilinear parabolic equations. J. Sci. Comput. 67, 1043–1065 (2016)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

The authors would like to acknowledge the support provided by King Fahd University of Petroleum & Minerals via the project SB191001. We thank the reviewer for the constructive comments, which helped improve the presentation of this paper.

Author information

Authors and Affiliations

  1. Department of Mathematics, King Fahd University of Petroleum & Minerals, Dhahran, 31261, Saudi Arabia

    Ibrahim O. Sarumi, Khaled M. Furati & Kassem Mustapha

  2. Department of Mathematical Sciences, Middle Tennessee State University, Murfreesboro, TN 37132-0001, USA

    Abdul Q. M. Khaliq

Authors
  1. Ibrahim O. Sarumi
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Khaled M. Furati
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Kassem Mustapha
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Abdul Q. M. Khaliq
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Khaled M. Furati.

Ethics declarations

The authors declare no competing interests.

Additional information

Data availability

Data sharing not applicable to this article as no datasets were generated or analyzed during the current study.

Publisher’s note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Sarumi, I.O., Furati, K.M., Mustapha, K. et al. Efficient high-order exponential time differencing methods for nonlinear fractional differential models. Numer Algor 92, 1261–1288 (2023). https://doi.org/10.1007/s11075-022-01339-2

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11075-022-01339-2

Keywords

Search

Navigation